area between curves y = f (x) between x = a and x = b, integrate y = f (x) between the limits of a and b. Areas under the x-axis will come out negative and areas above the x-axis will be positive.
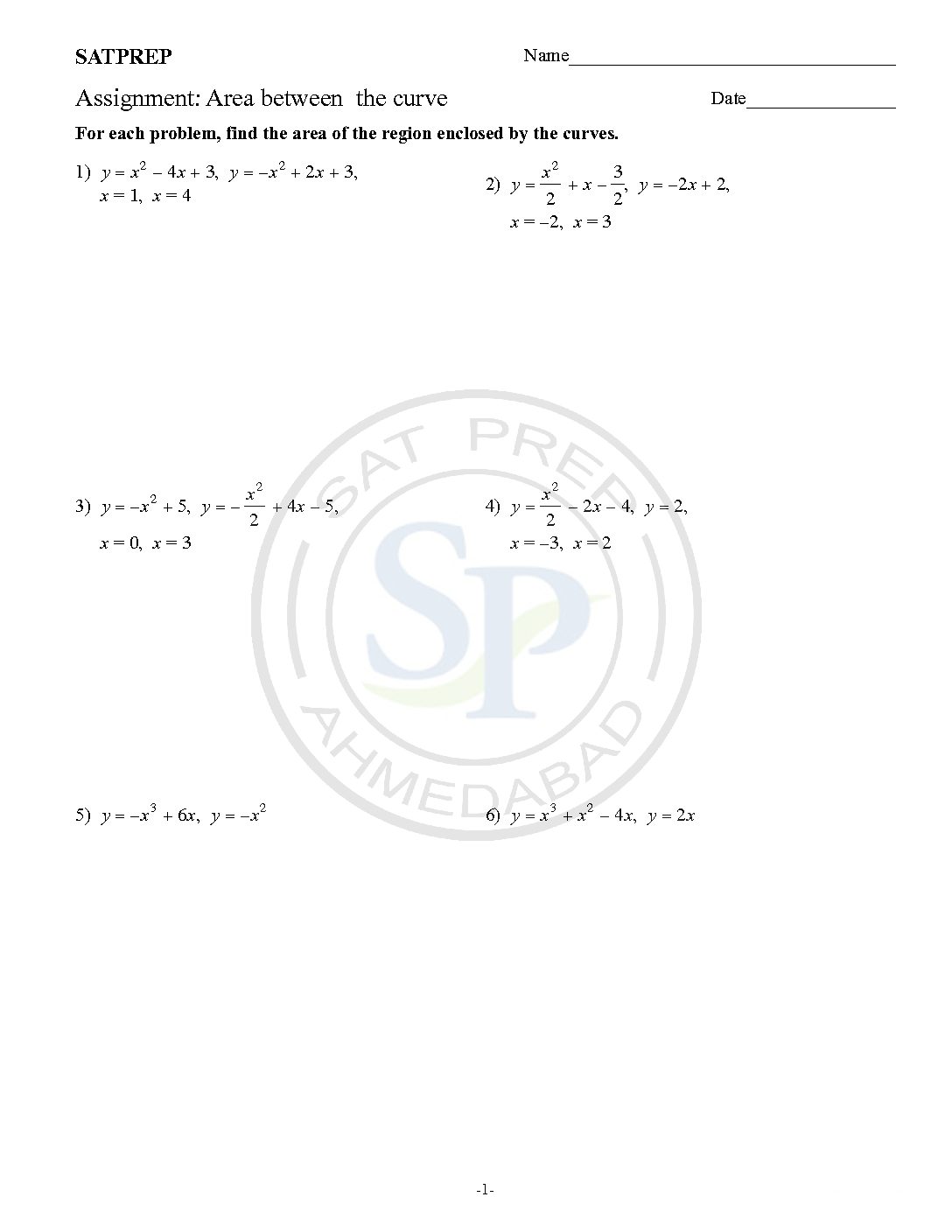
area between curves y = f (x) between x = a and x = b, integrate y = f (x) between the limits of a and b. Areas under the x-axis will come out negative and areas above the x-axis will be positive.
A Definite Integral has start and end values. In other words there is an interval [a, b]. Hence , definite integral gives particular solution. Definite Integration
To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution Volume of Revolution
This post about Area under a Curve. The area between the graph of y = f ( x ) and the x -axis is given by the definite integral. This formula gives a positive result for a graph above the x -axis, and a negative result for a graph below the x -axis. Because of enclosed region by limit. Hence , we use definite integration. Similarly for volume […]