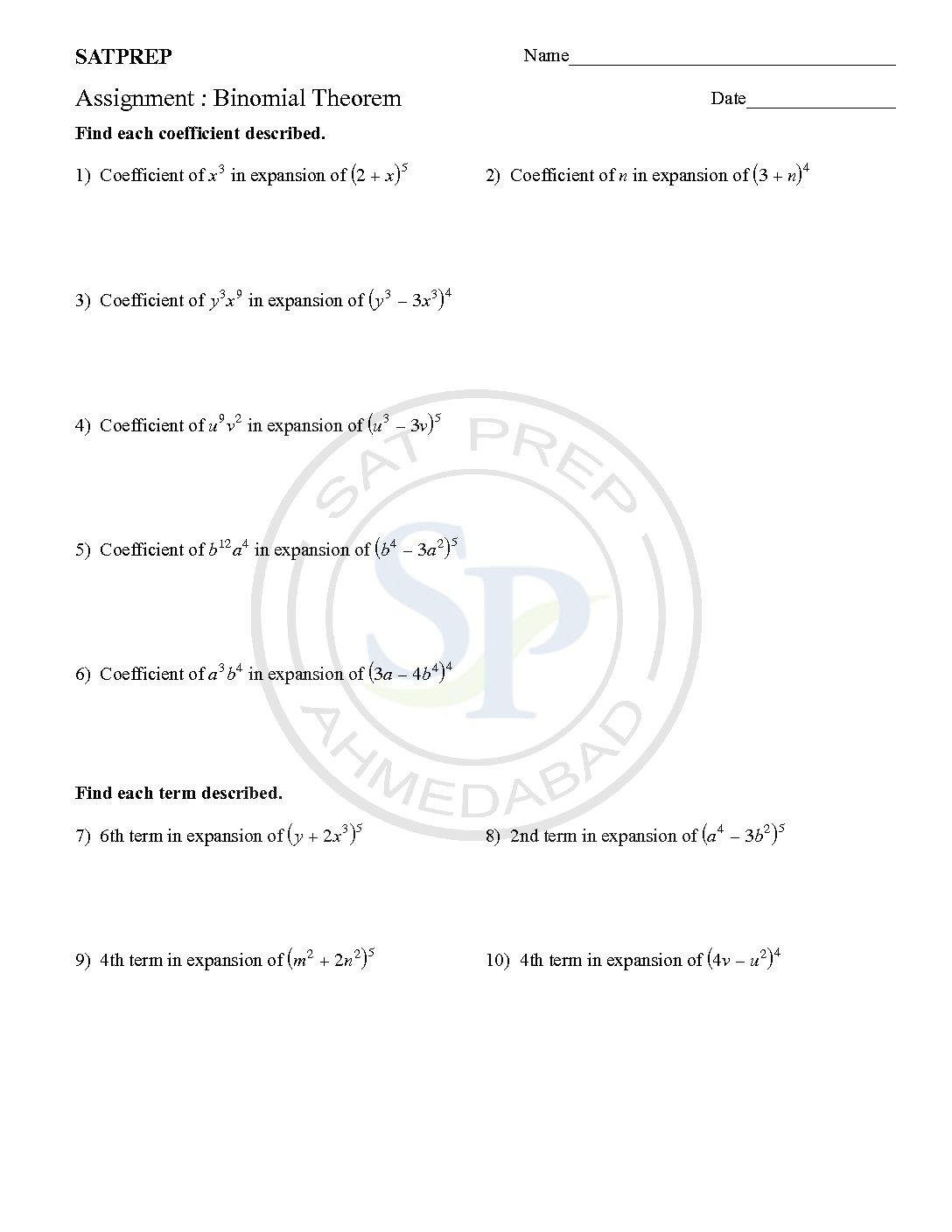
Binomial theorems is another ways of expansion of two terms. Another way it is generalised form of expansion. Due to expansion of two term it is binomial. “What are the binomial coefficients?” . It shows how to calculate the coefficients in the expansion of (a + b) n. The symbol for a binomial coefficient nCr. As well as pascal […]
You are browsing archives for
Category: Expansions
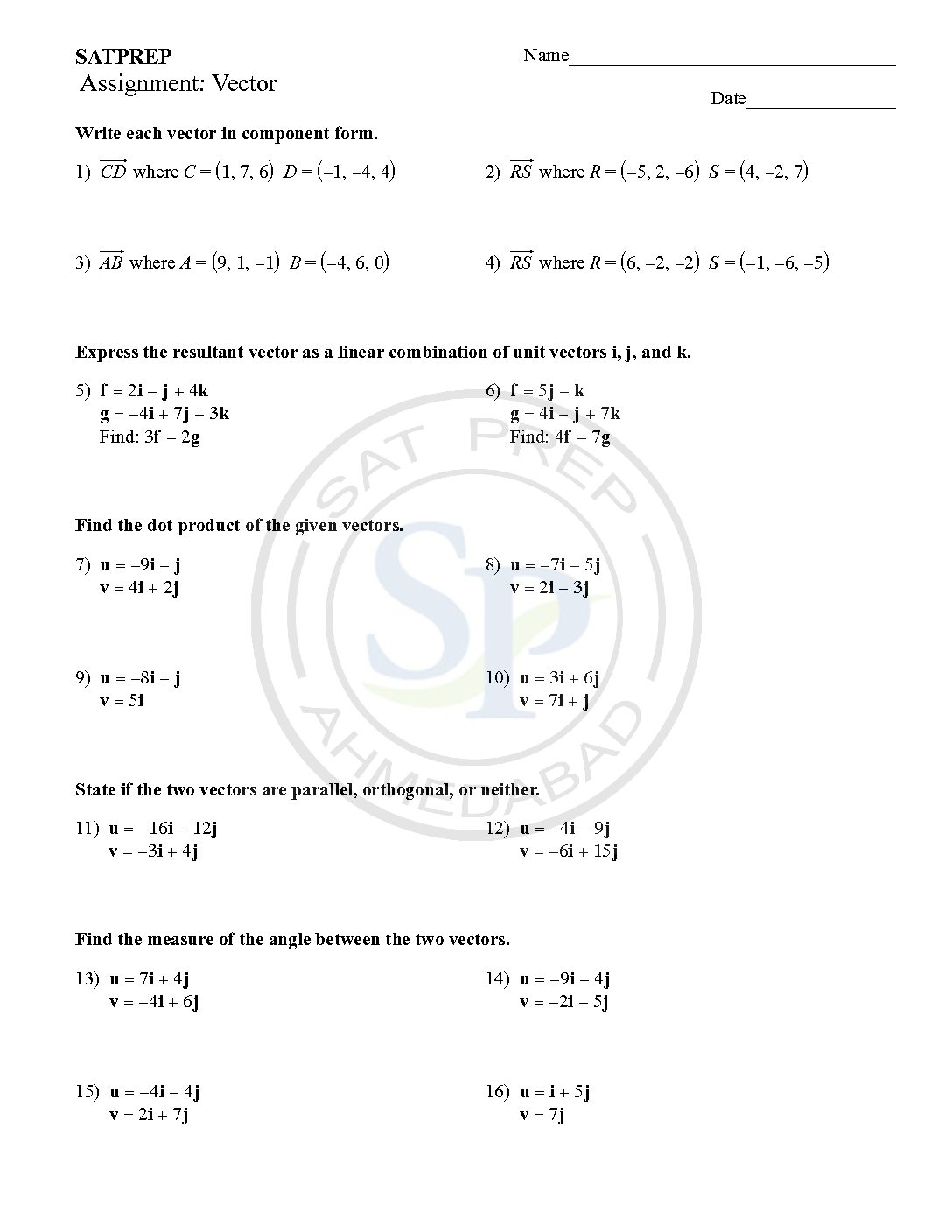
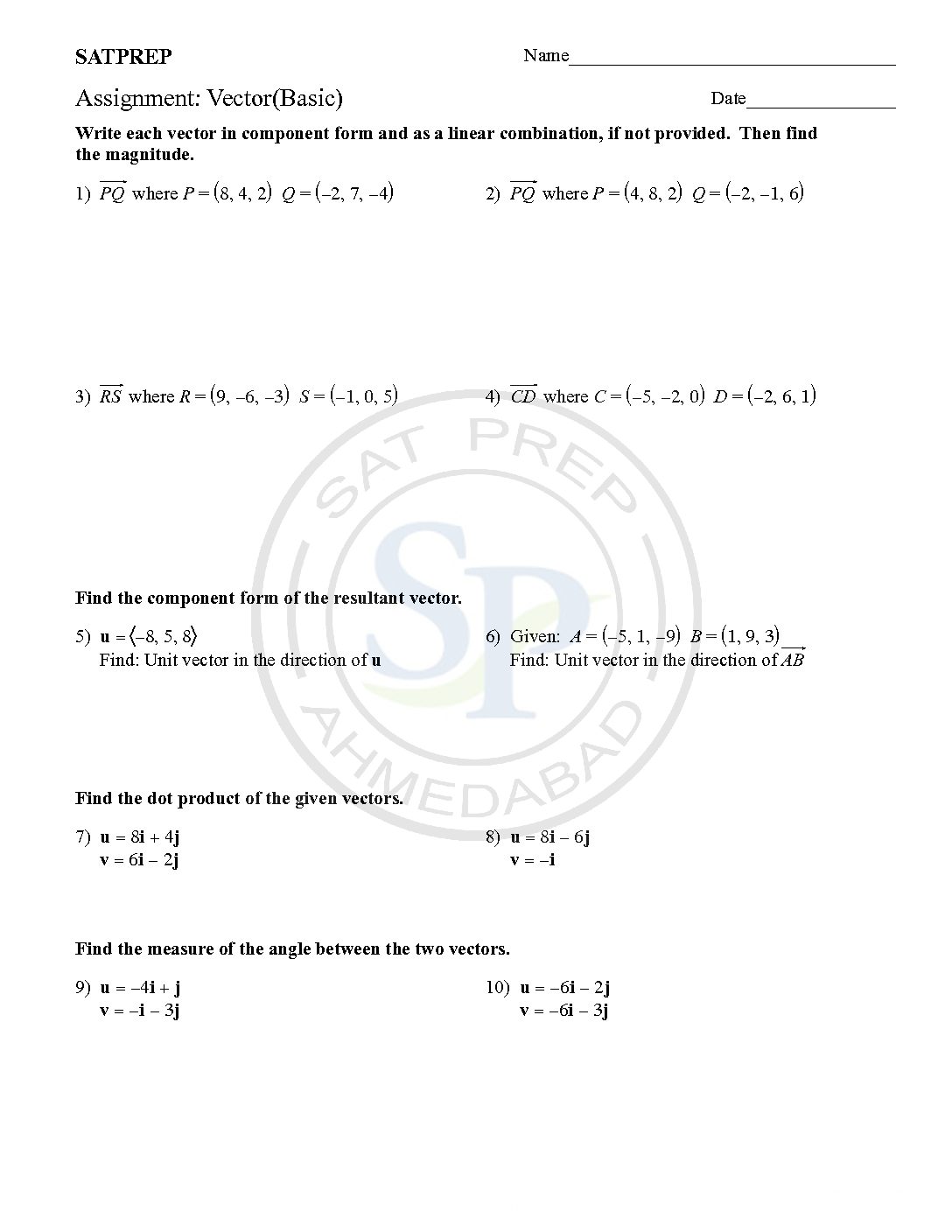
Vectors and scalar product
Scalar product of two vectors is multiply and obtain a scalar quantity. It represent on ‘dot’. Another name is dot product. vector has magnitude and direction. It start from one point to another point. The length of vector is called magnitude. Scalar product and vector
Vector and Scalar product.
vector has magnitude and direction. It start from one point to another point. The length of vector is called magnitude. The scalar product of two vectors is multiply and obtain a scalar quantity. It represent on ‘dot’. Another name is dot product. Vector and scalar product
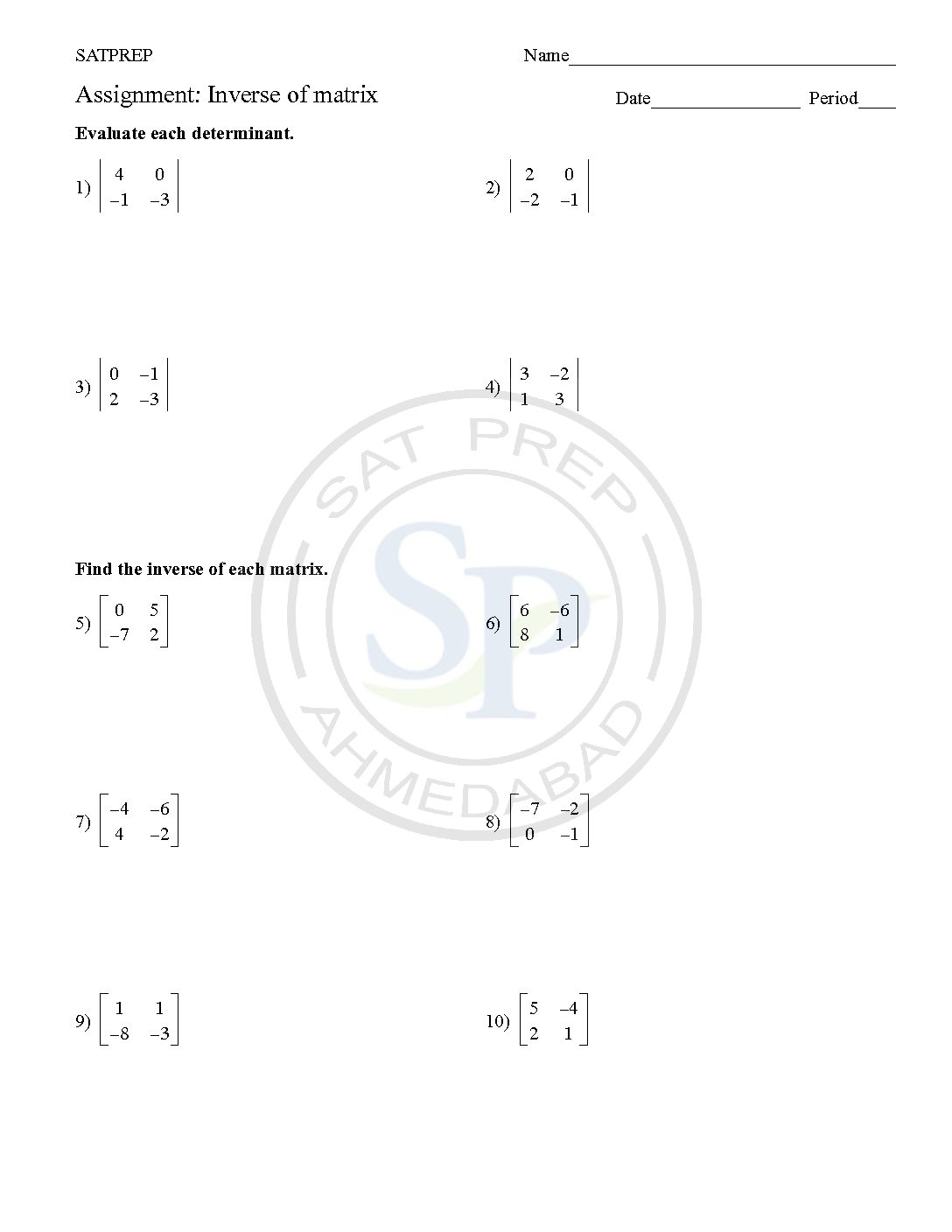
Inverse of Matrix
Matrix Inverses Multiplicative Inverse of a Matrix. For a square matrix A, the inverse is written A-1. When A is multiplied by A-1 get identity matrix. When det(A) is zero inverse not exists. Hence singular matrix has no inverse. Inverse of Matrix
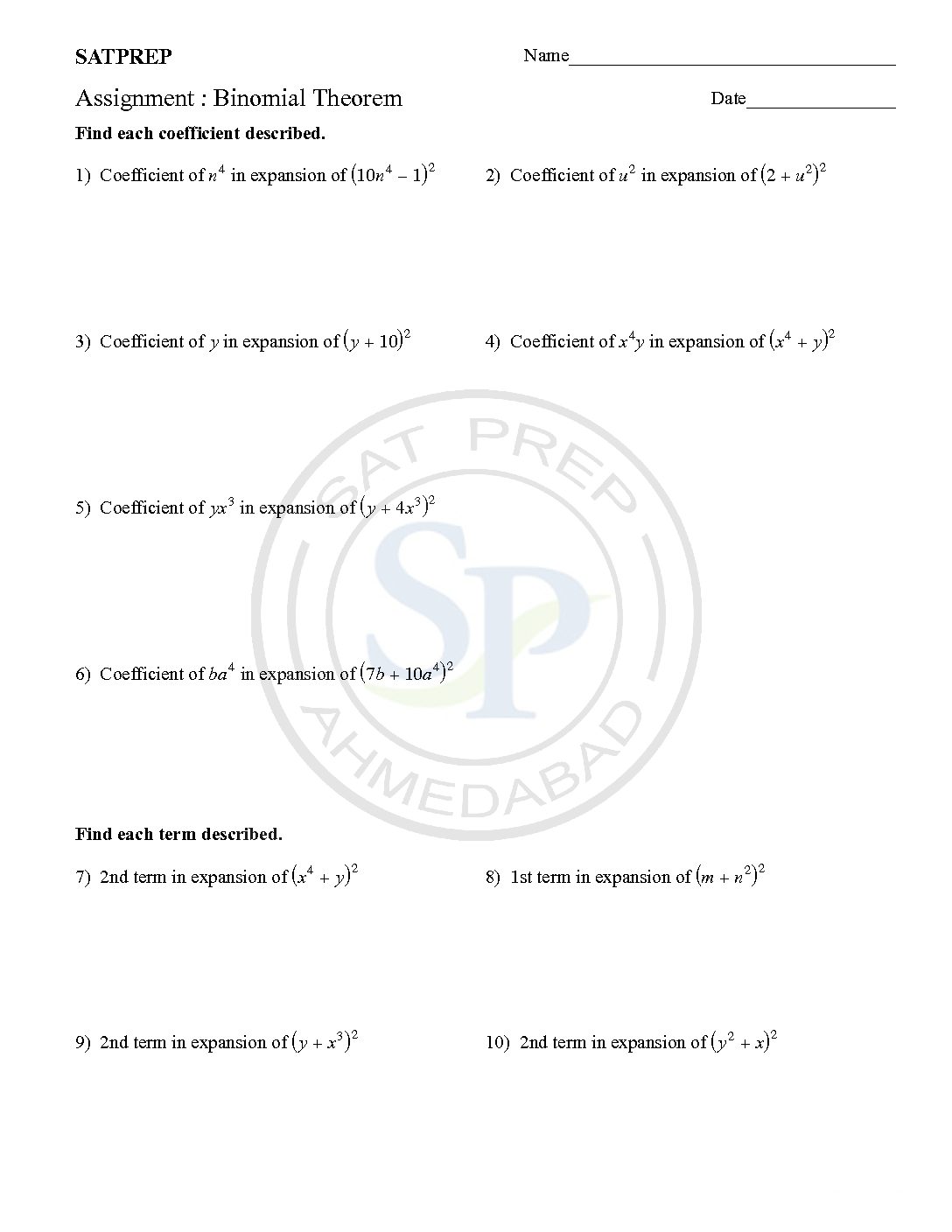
Binomial theorem
The expansion of two terms is know as Binomial theorems . Another way it is generalised form of expansion. Due to expansion of two term it is binomial. “What are the binomial coefficients?” . It shows how to calculate the coefficients in the expansion of (a + b) n. The symbol for a binomial coefficient nCr. As well as […]