This post about Slope of lines Eq. of line is part of coordinate geometry. coordinate geometry
You are browsing archives for
Category: Graphs
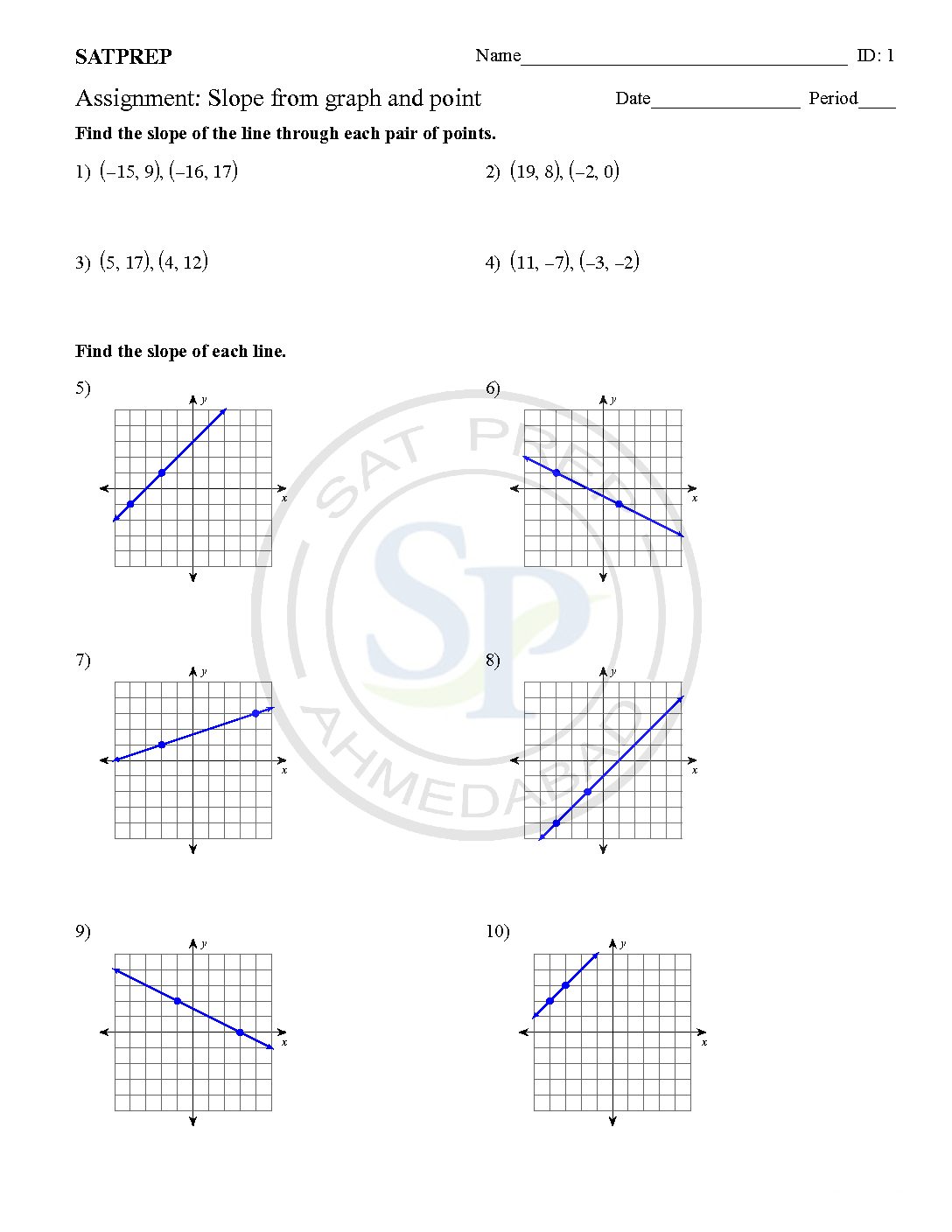
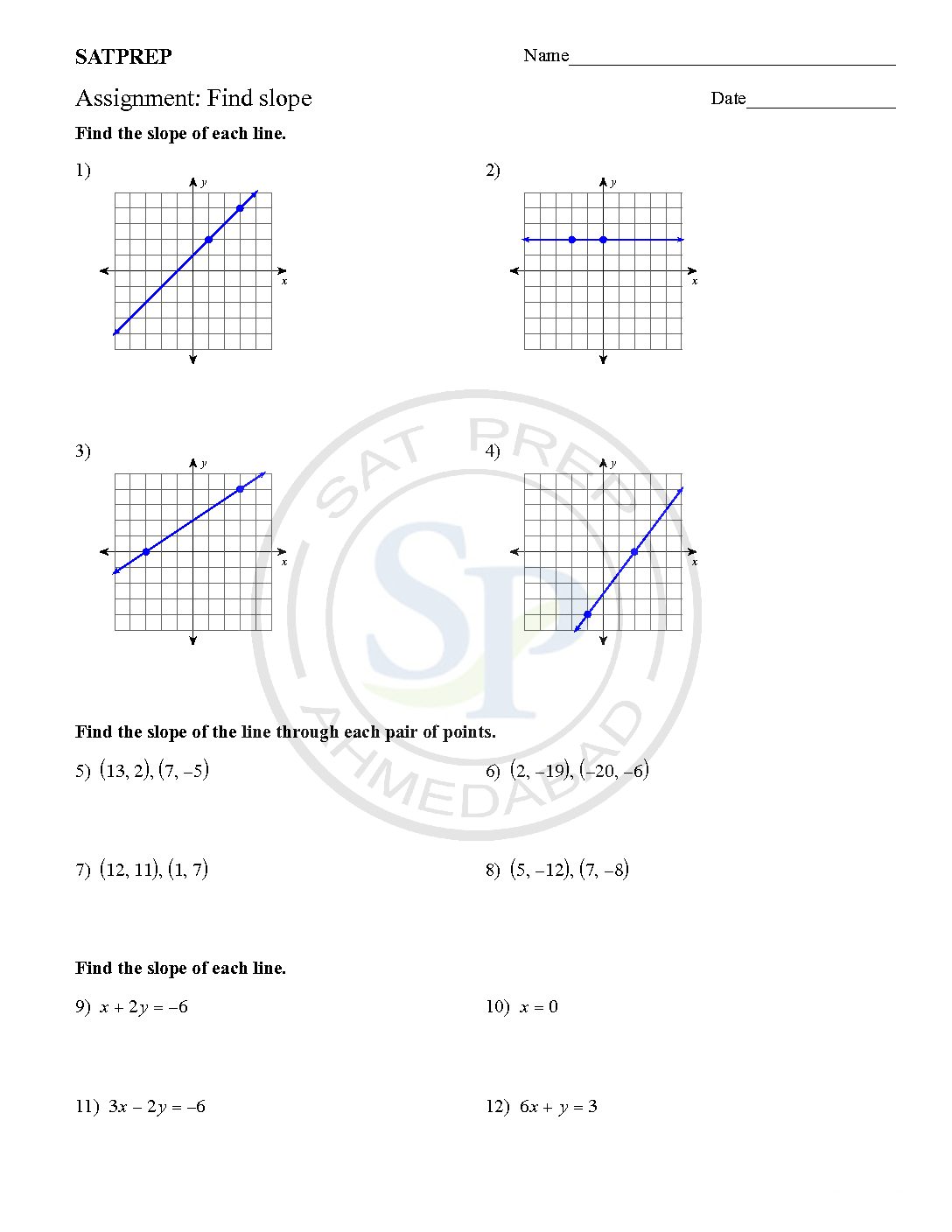
Find slope
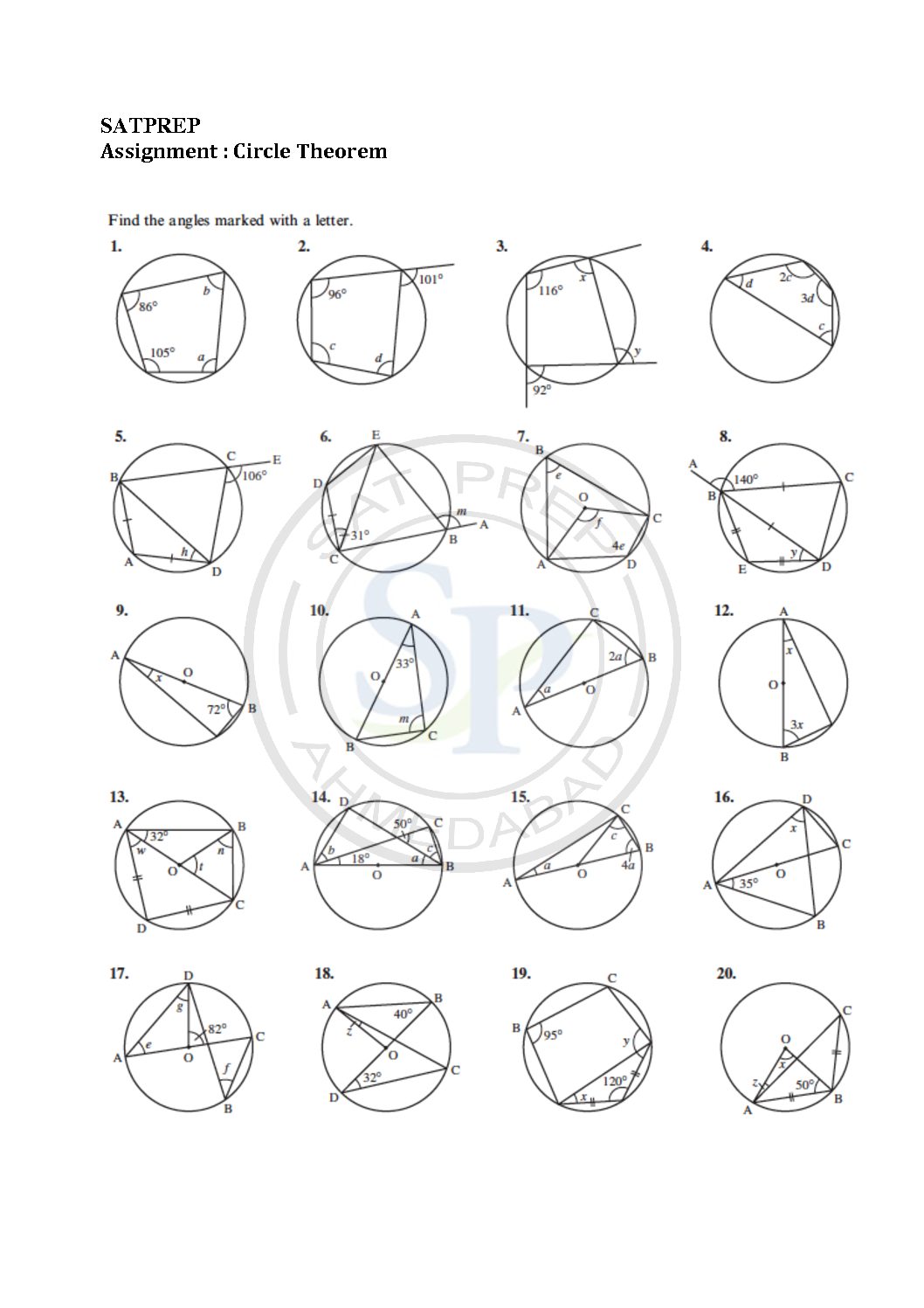
Circle Geometry
This post about is the Circle theorems. Formulas for the radius of a circle, the diameter of a circle, the circumference (perimeter) of a circle. Therefore It also include the length of chord of a circle. Because of length of chord angle also varies. Similarly other properties also involve. Explore, prove, and apply important properties of circles that have […]
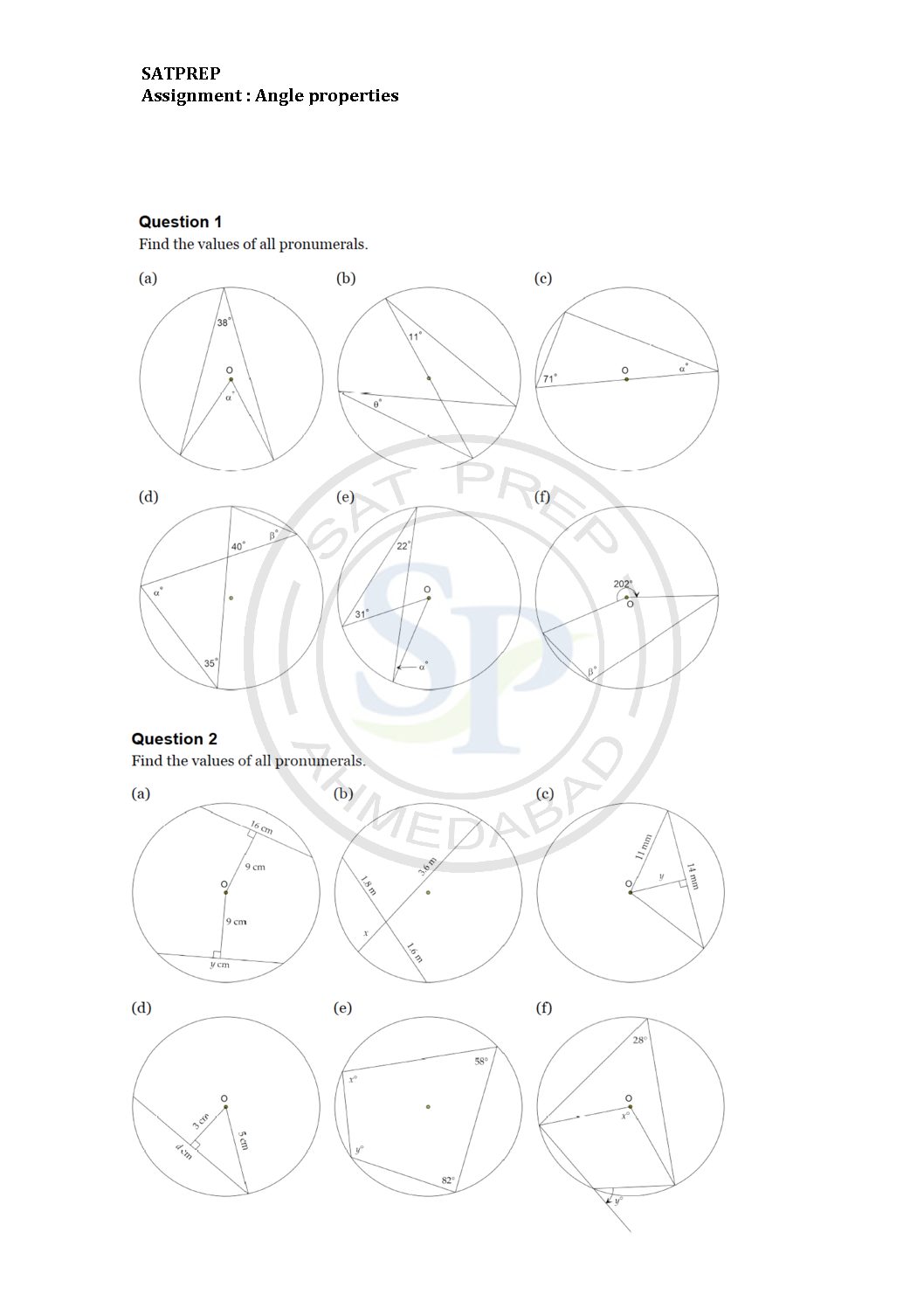
Angles properties
This post about is the the Angle tangent properties. Formulas for the radius of a circle, the diameter of a circle, the circumference (perimeter) of a circle. Therefore It also include the length of chord of a circle. Because of length of chord angles also varies. Similarly other properties also involve. Explore, prove, and apply important properties of circles […]
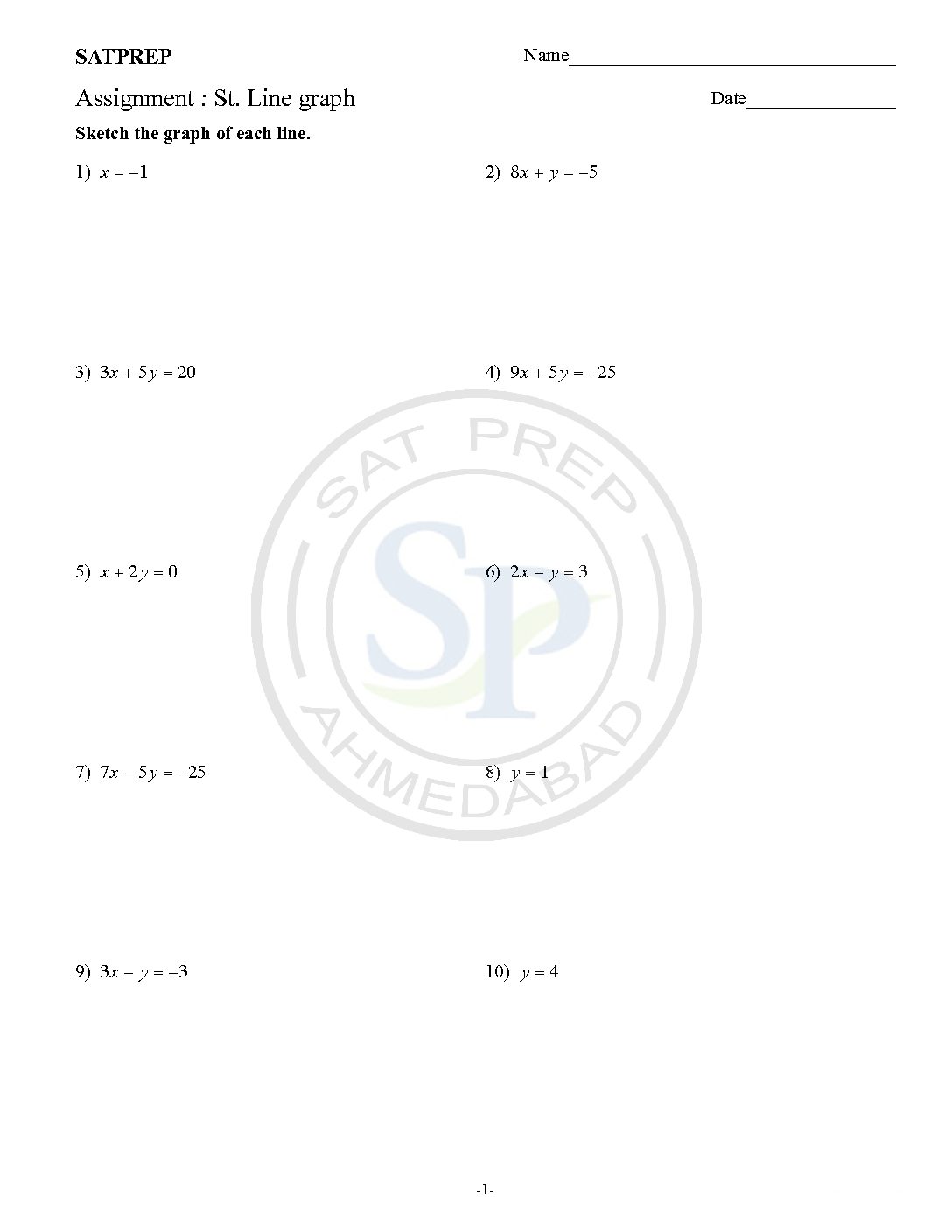
St. Line Graph
Straight line graphs. The graph of each of equations is a straight line: If an equation can be rearranged into the form y = mx +c, then its graph will be a straight line. ax +by= c can be rearranged as (which can be re-written as y = mx+c). Therefore vertical lines have equations have slope undefined form x =k . Hence graph depend on […]
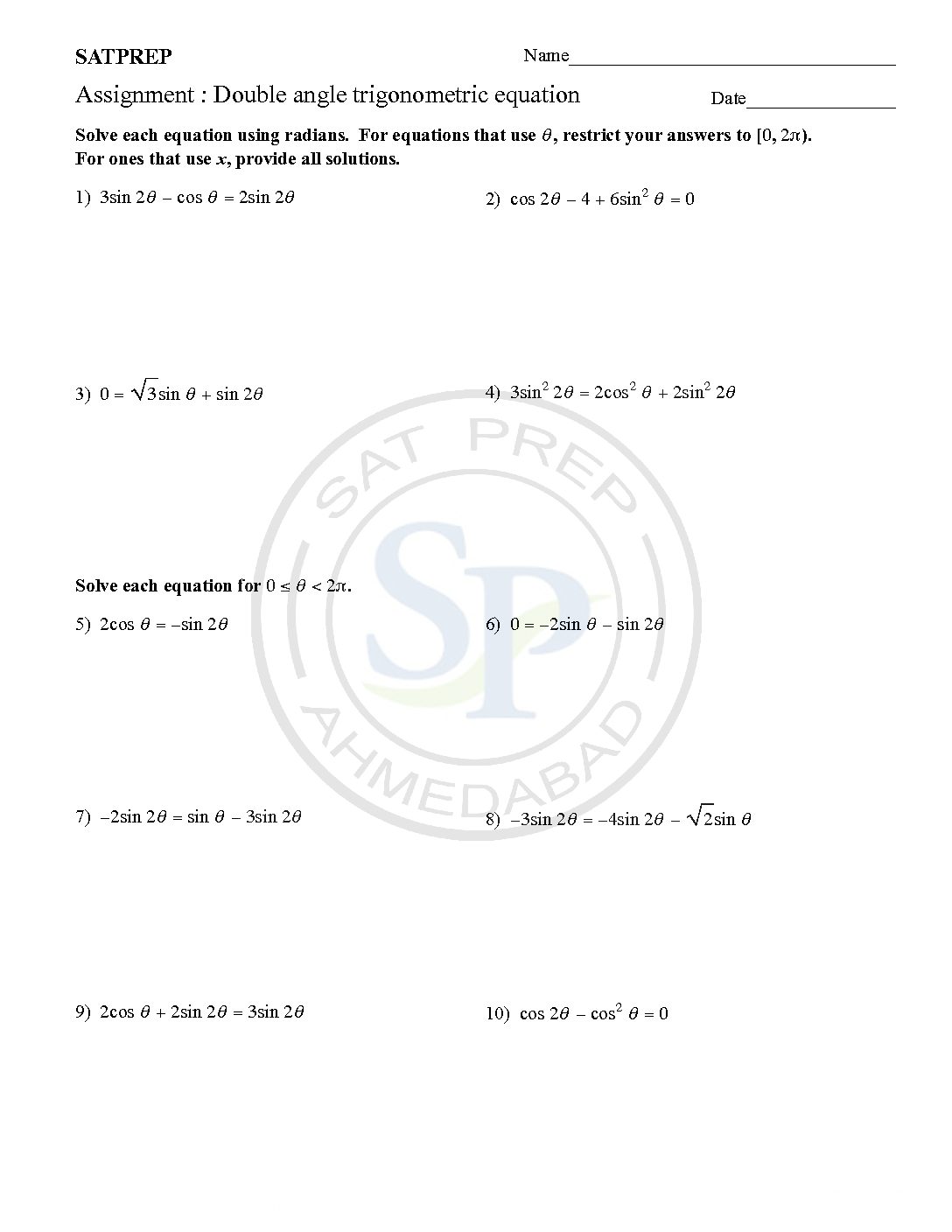
Double angle trigonometric equation
Double angle equations are allowing the expression of trigonometric functions of angles equal to 2u in terms of u. The double angle formulas can simplify the functions and gives ease to perform more complex calculations. The double angle formulas are useful for finding the values of unknown trigonometric functions. Therefore in double angle equation we need to consider two rotation. […]
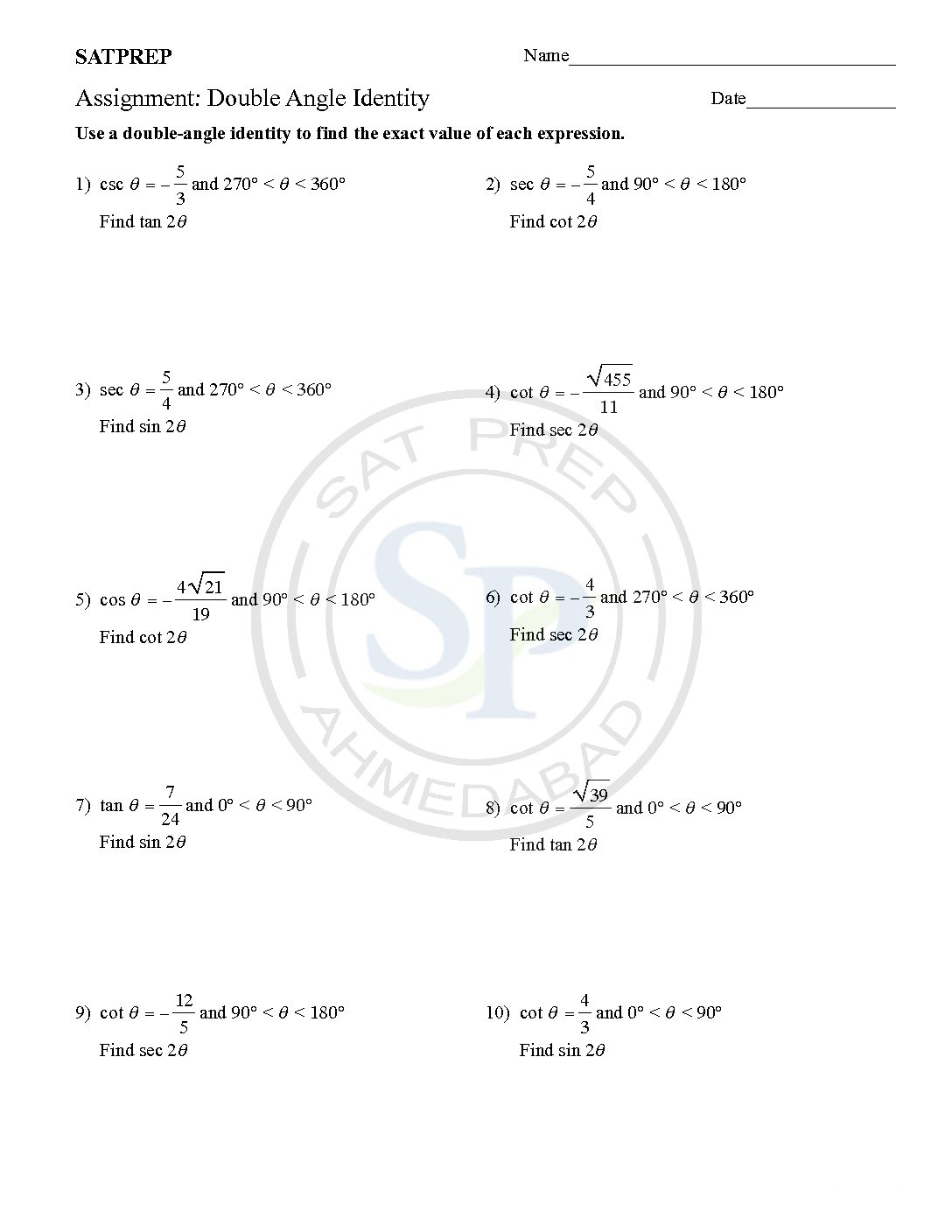
A Double angles identity
A Double angles identity is written2θ, for example, as sin 2θ, cos 2α, or tan 2x, where 2θ, 2α, and 2x. The angle measures and the assumption is that you mean sin(2θ), cos(2α), or tan(2x). Because tangent is equal to the ratio of sine and cosine . Therefor its identity comes from their double-angle identities. double angle identity
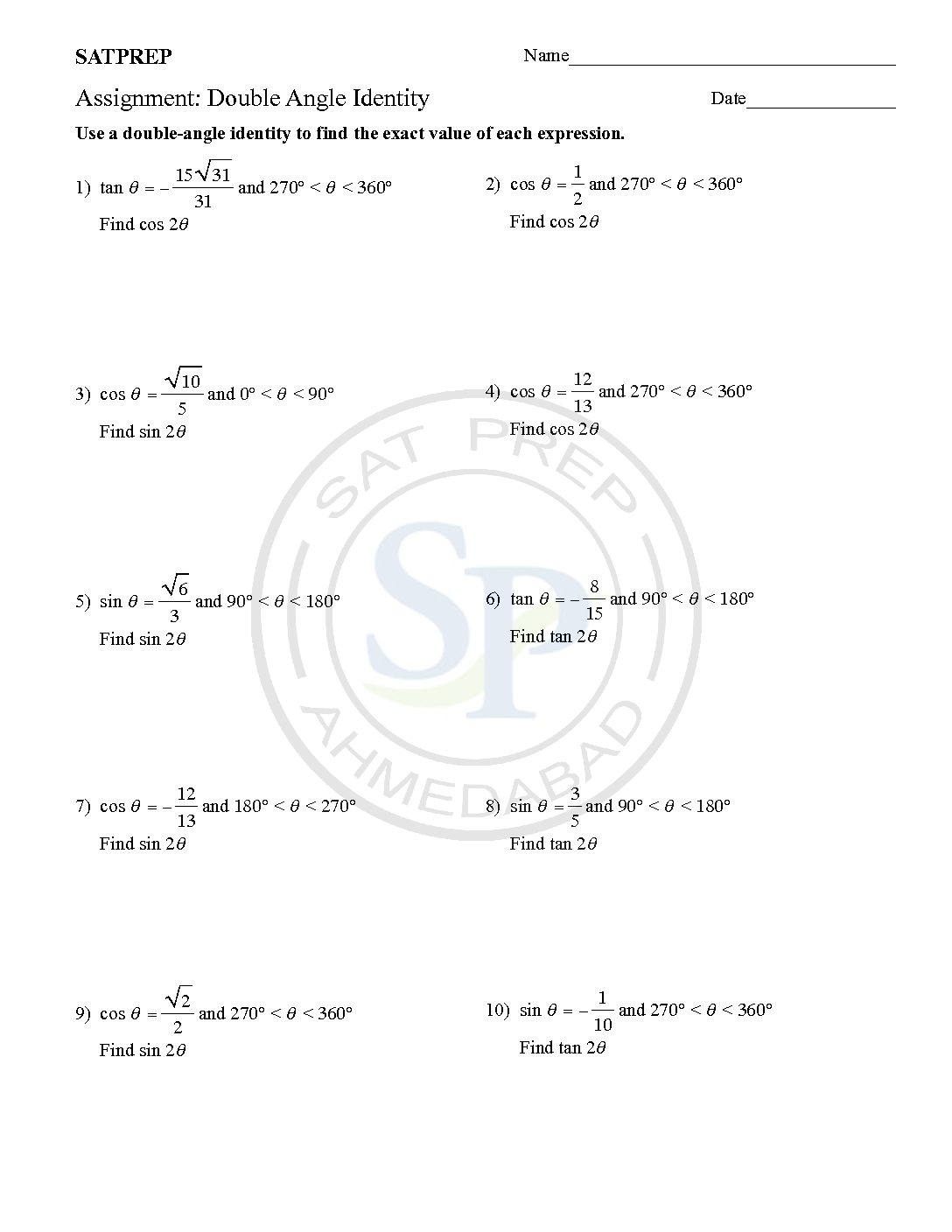
Double Angle trigonometric Identity
A Double angles identity is written2θ, for example, as sin 2θ, cos 2α, or tan 2x, where 2θ, 2α, and 2x. The angle measures and the assumption is that you mean sin(2θ), cos(2α), or tan(2x). Because tangent is equal to the ratio of sine and cosine . Therefor its identity comes from their double-angle identities. Double angle
Trigonometric equations
Trigonometric equations use both the reference angles and trigonometric identities The general method of solving an equation is to convert it into the form of one ratio only. Hence, we can obtain solutions. Trigonometric Equation
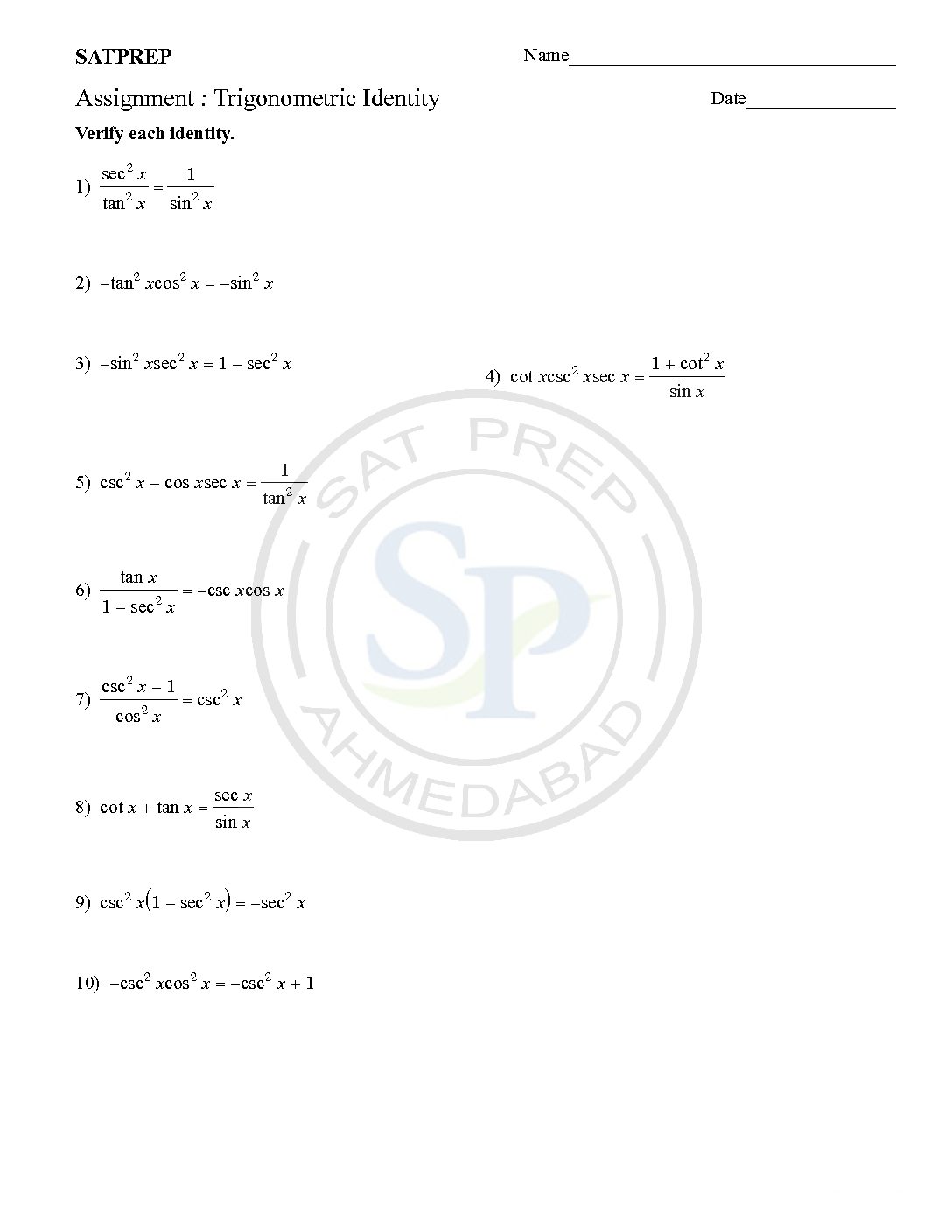
Trigonometric Identity
The trigonometric identity showing that the identity is always true, no matter what value of x or θ is used. Because it has to hold true for all values of x, we cannot simply substitute in a few values of x to “show” that they are equal. We have to use logical steps to show […]