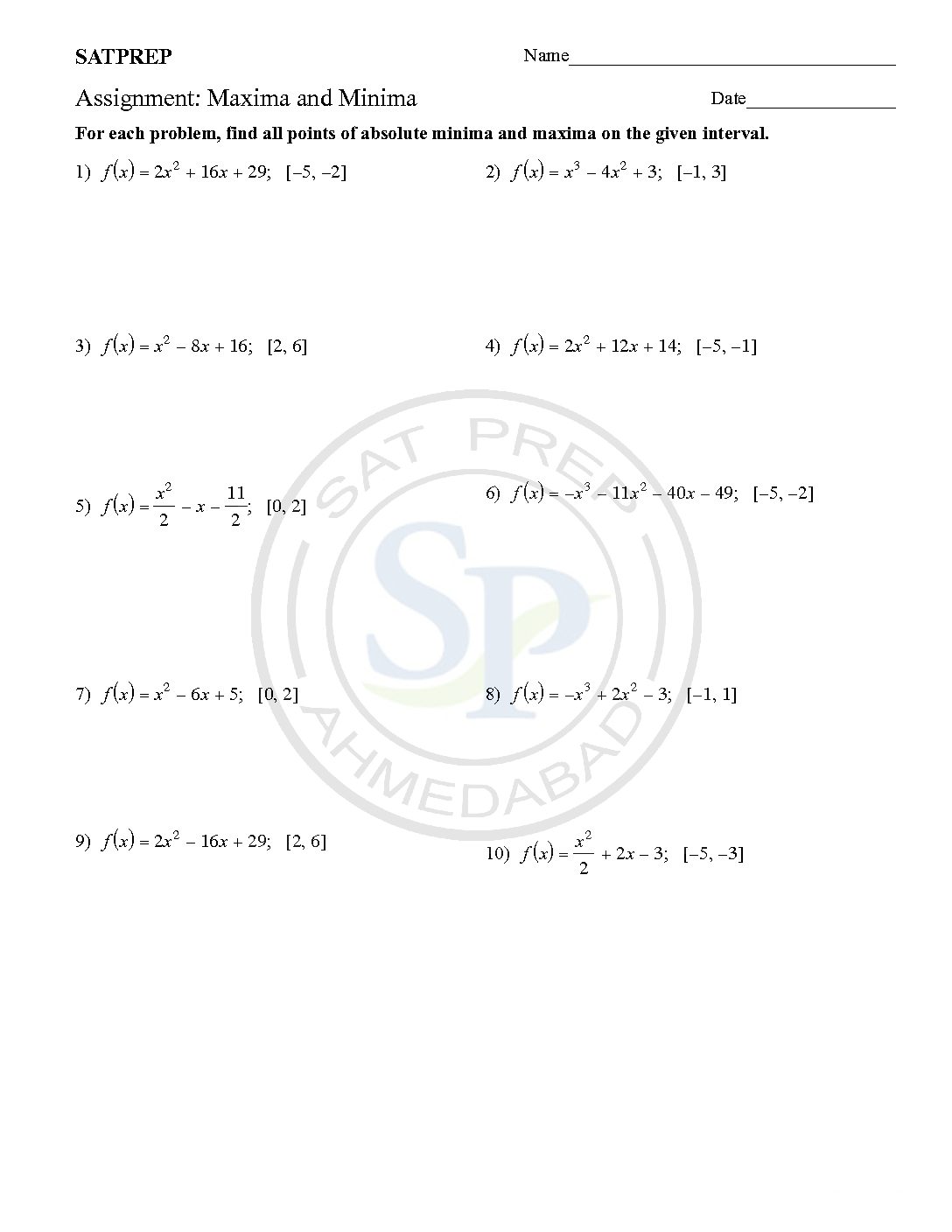
Maximum and Minima of Points of Inflection. The value f ‘(x) is the gradient at any point but often we want to find the Turning or Stationary Point (Maximum and Minimum points) or Point of Inflection These happen where the gradient is zero, f ‘(x) = 0. Critical Points include Turning points and Points where f ‘ (x) does not exist. […]
You are browsing archives for
Category: Additional Maths
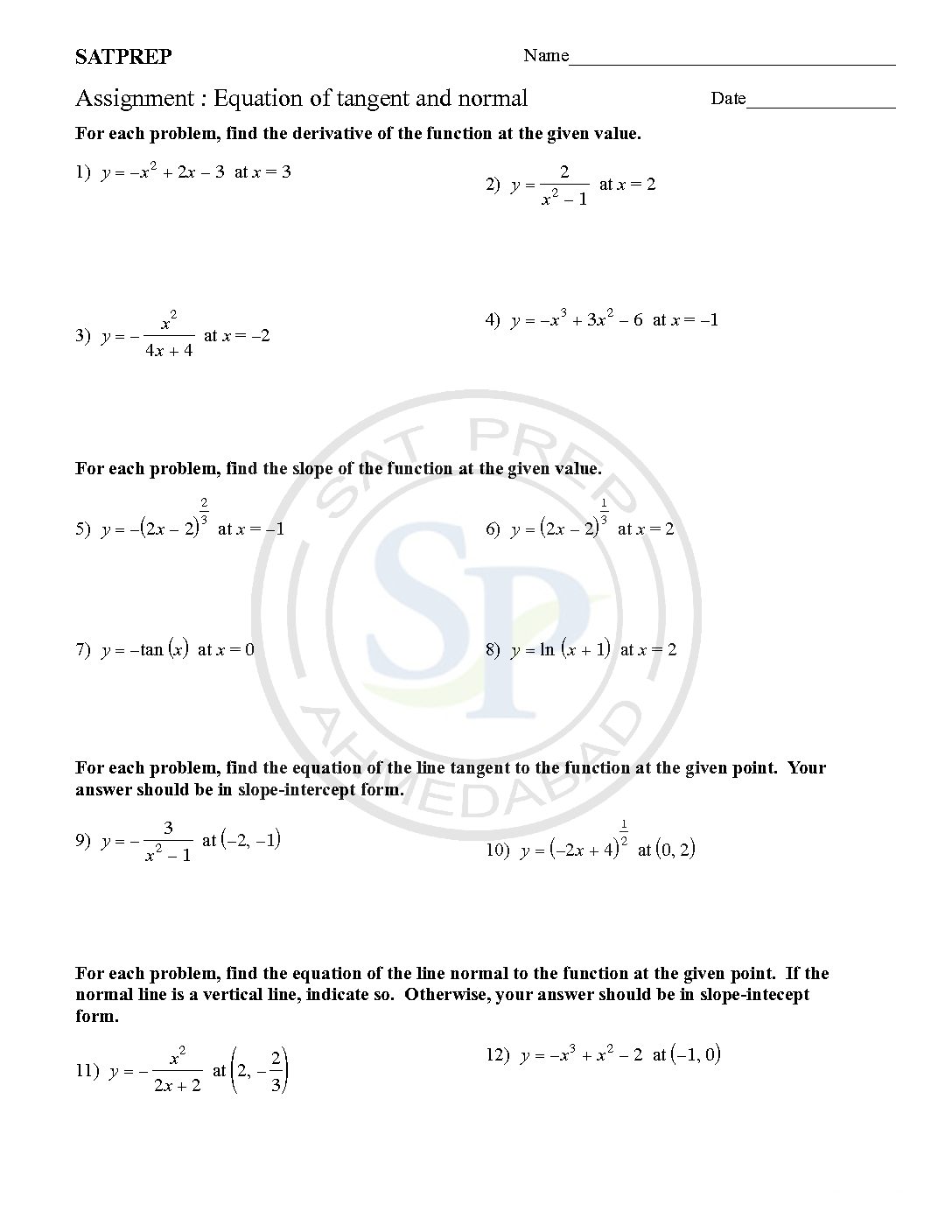
Equation of Tangent and Normal
A tangent to a curves are a line that touches the curve at one point and has the same slope as the curve at that point. A normal to a curve is a line perpendicular to a tangent to the curve. Tangent and normal
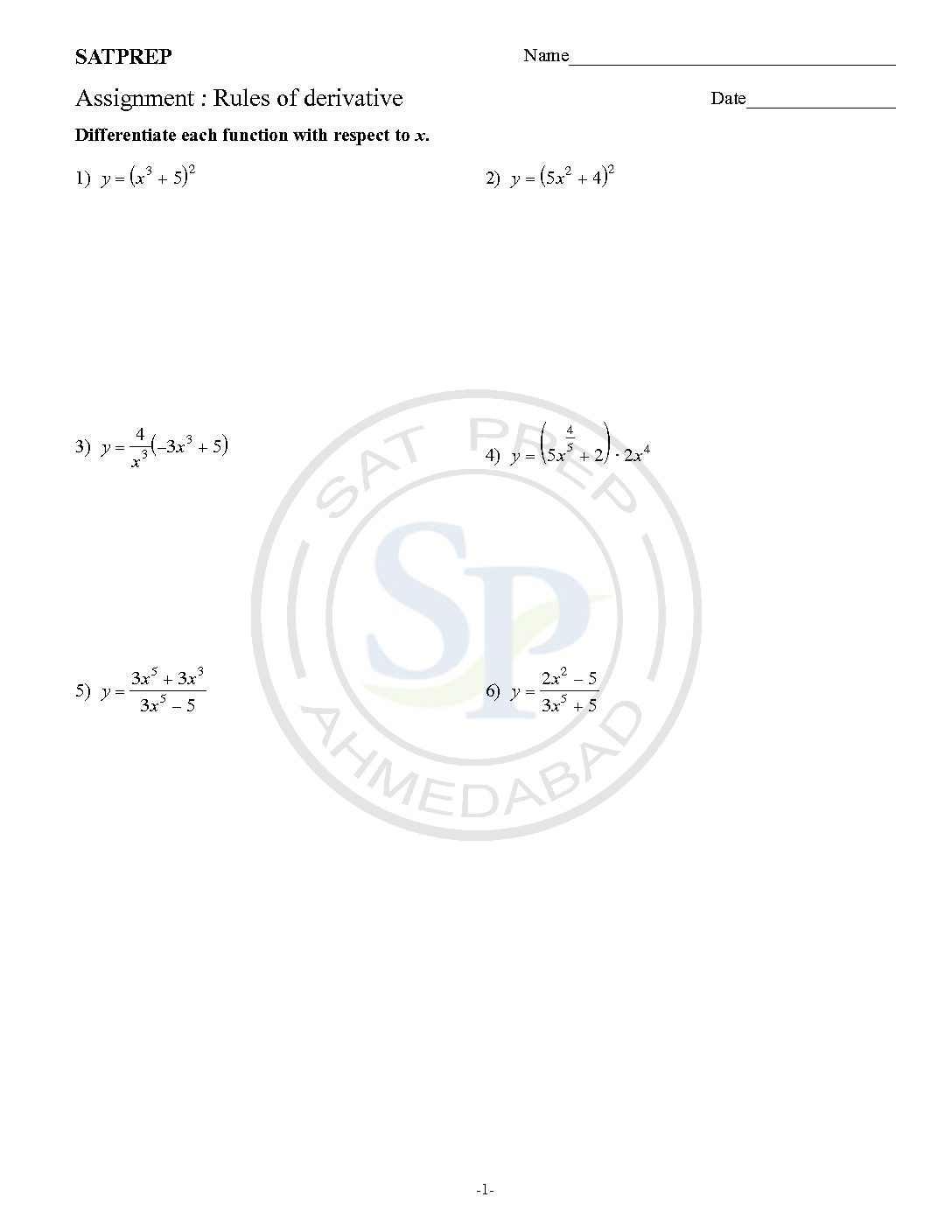
Rules of derivative
Rules for derivatives. Rules for derivatives. Sum rule: The derivative of the sum or difference of two functions is the sum or difference of their derivatives. (u + v)’ = u’ + v’ Constant multiple: The derivative of a constant times a function is the constant times the derivative of the function. (ku)’ = ku’ Rules for derivative
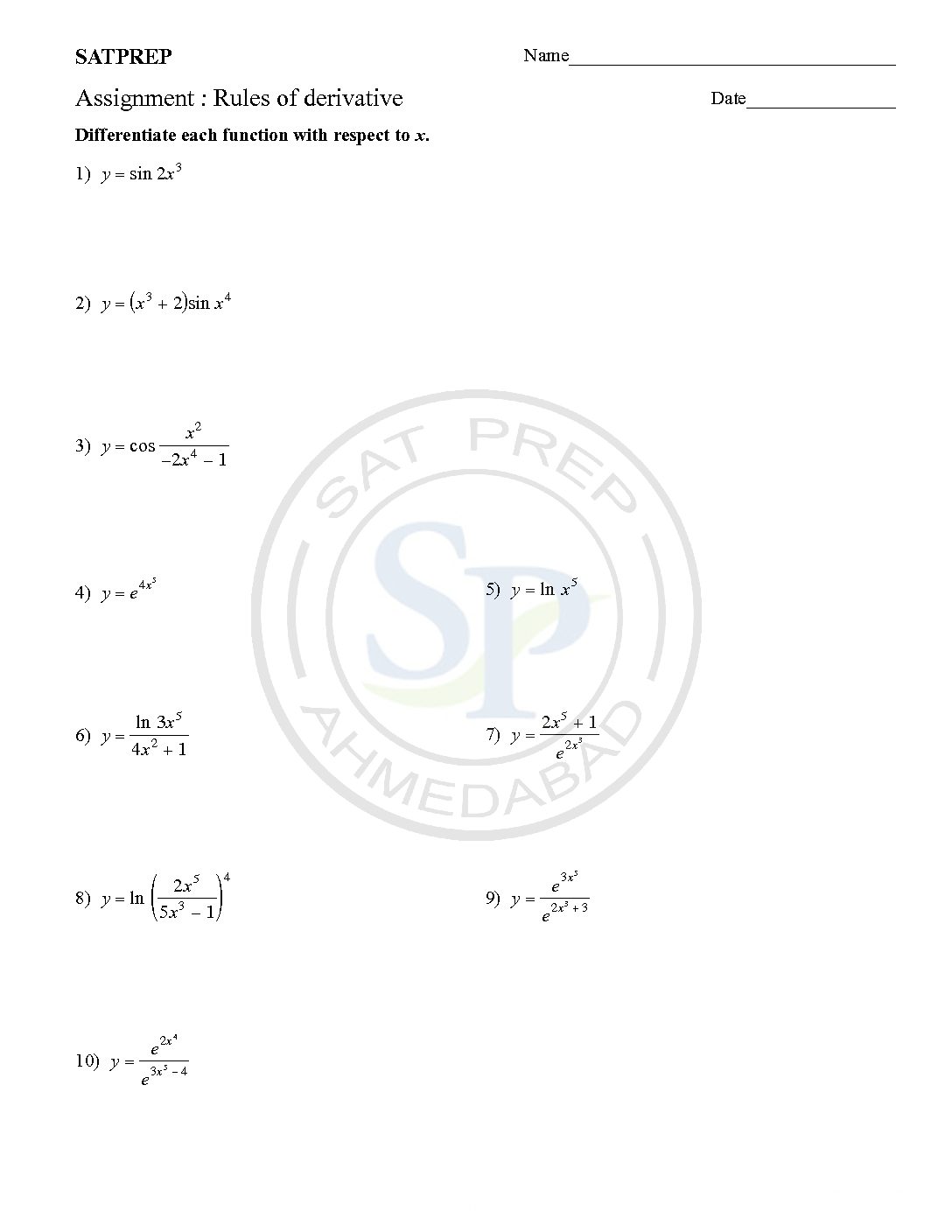
Rules of derivative
Rule for derivatives. Rules for derivatives. Sum rule: The derivative of the sum or difference of two functions is the sum or difference of their derivatives. (u + v)’ = u’ + v’ Constant multiple: The derivative of a constant times a function is the constant times the derivative of the function. (ku)’ = ku’ Rules of derivative
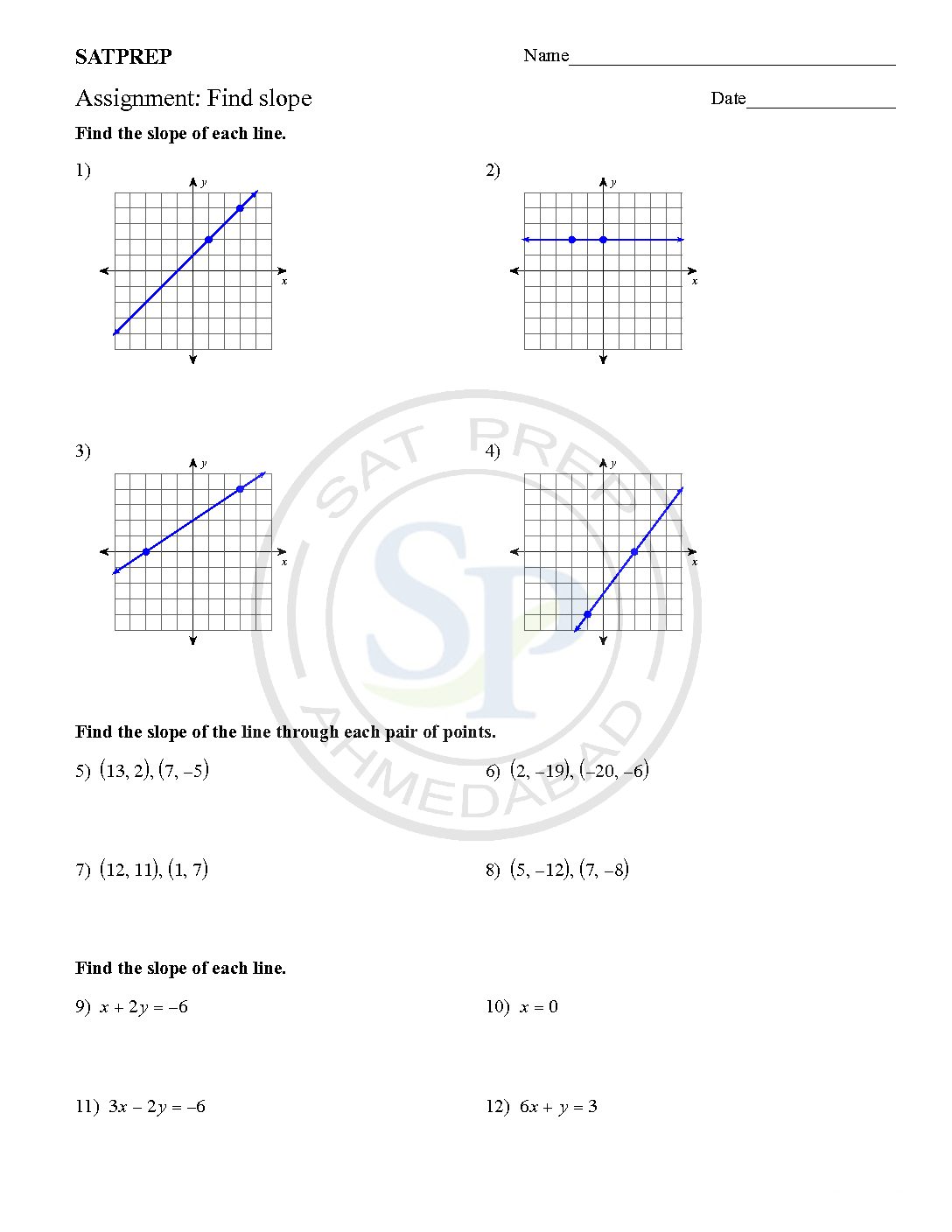
Find slope
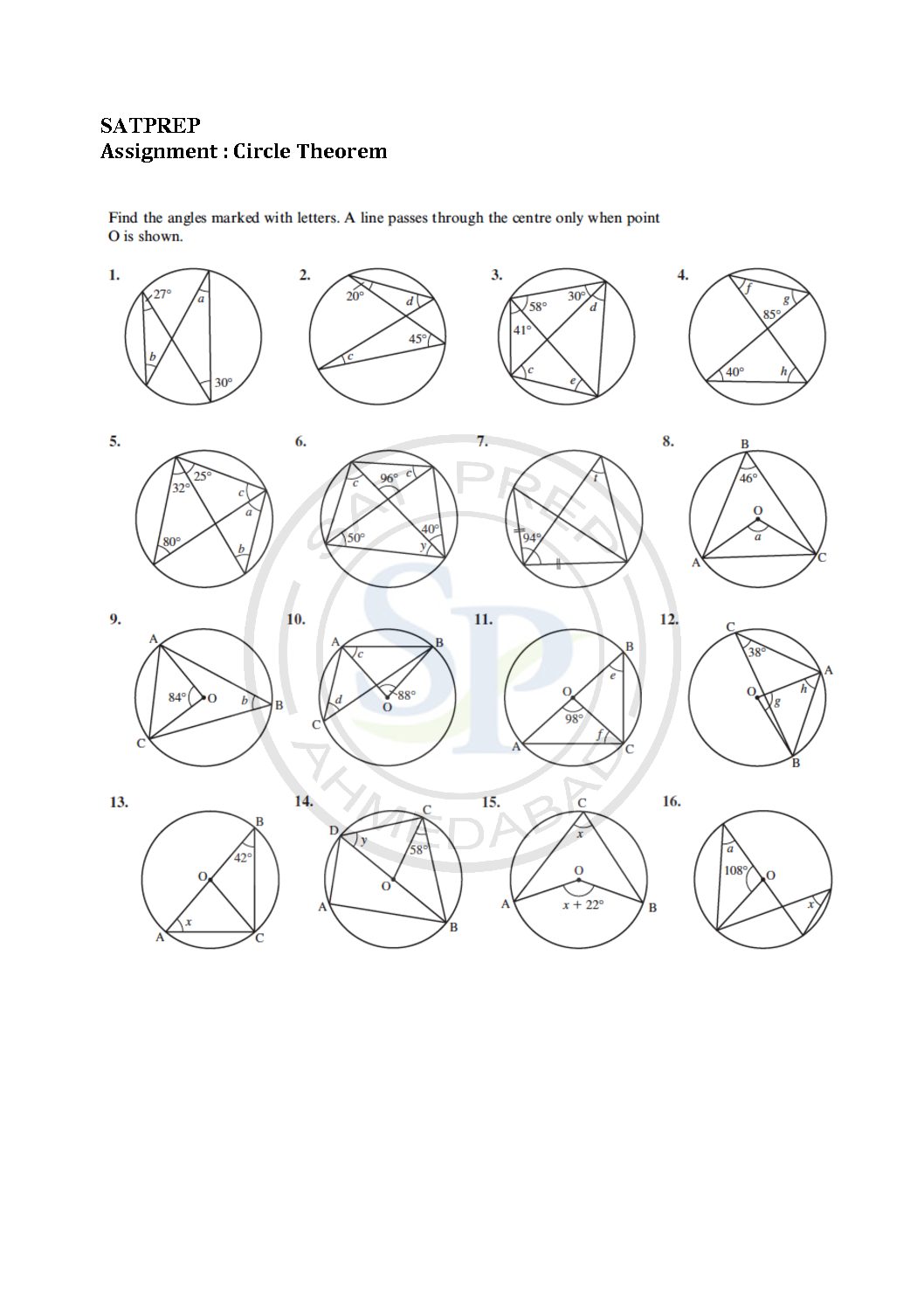
Circle Theorem
This post about is the Circle theorems. Formulas for the radius of a circle, the diameter of a circle, the circumference (perimeter) of a circle. Therefore It also include the length of chord of a circle. Because of length of chord angle also varies. Similarly other properties also involve. Explore, prove, and apply important properties of circles that have […]
Circle Geometry
This post about is the Circle theorems. Formulas for the radius of a circle, the diameter of a circle, the circumference (perimeter) of a circle. Therefore It also include the length of chord of a circle. Because of length of chord angle also varies. Similarly other properties also involve. Explore, prove, and apply important properties of circles that have […]
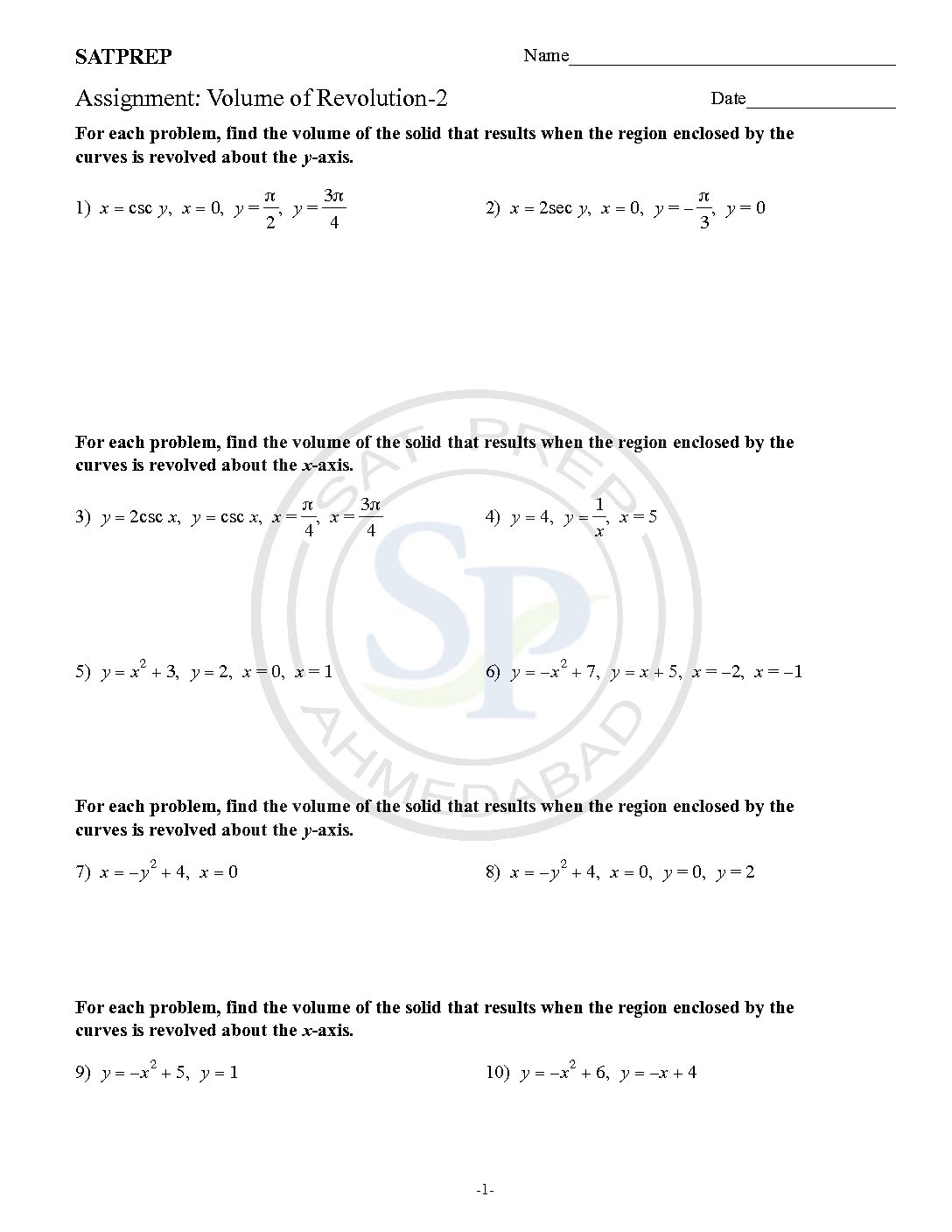
Volume of revolution of solid
Volume of revolution. To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. For purposes of this discussion let’s rotate the curve about the x -axis, although it could be any vertical […]
Volume of revolution of solid
Volume with Rings. To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. For purposes of this discussion let’s rotate the curve about the x -axis, although it could be any vertical or […]
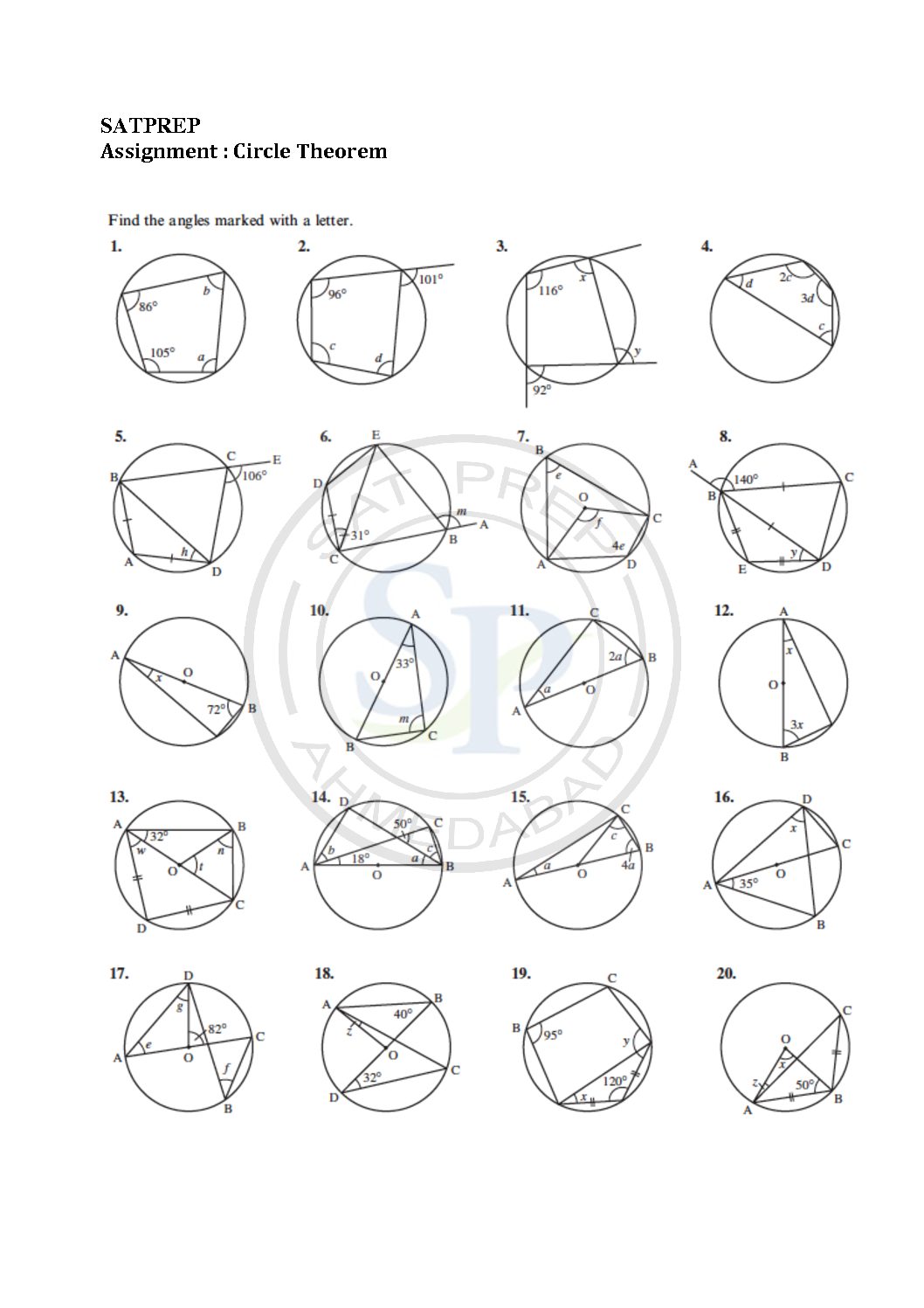
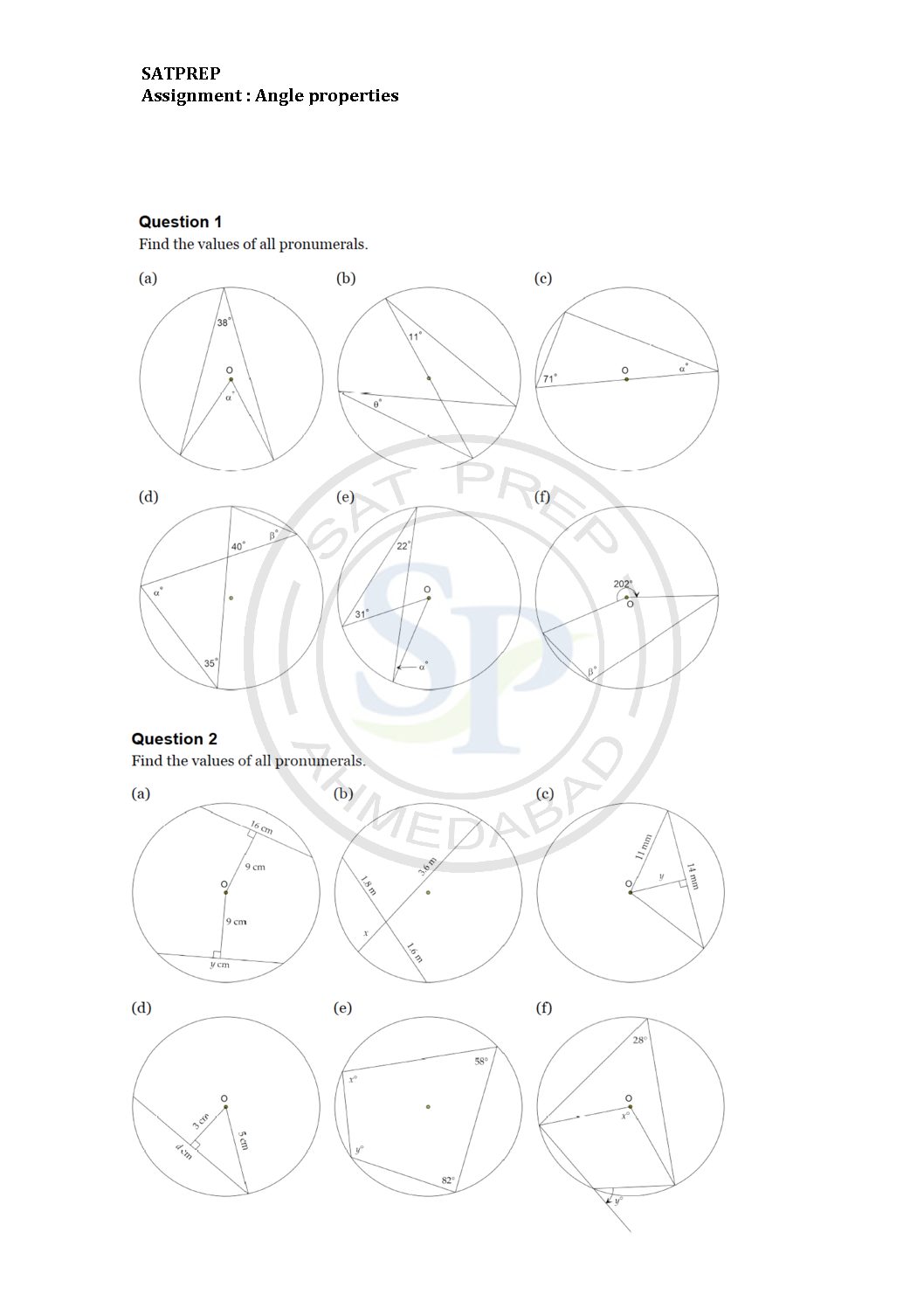
Angles properties
This post about is the the Angle tangent properties. Formulas for the radius of a circle, the diameter of a circle, the circumference (perimeter) of a circle. Therefore It also include the length of chord of a circle. Because of length of chord angles also varies. Similarly other properties also involve. Explore, prove, and apply important properties of circles […]