First of all differentiation and Integration are process of calculus. Due to differentiation we get derivative, while integration of derivative we get function back. Integration also called derivative. Differentiation and Integration
You are browsing archives for
Category: Derivative
Derivative of implicit and inverse trigo...
In calculus, a methods of implicit differentiation, Makes use of the chain rule to differentiate implicitly defined functions. To differentiate an implicit function y ( x ), defined by an equation R ( x, y) = 0, it is not generally possible to solve it explicitly for y and then differentiate. The derivatives of inverse trig functions we’ll need the formula from the last section […]
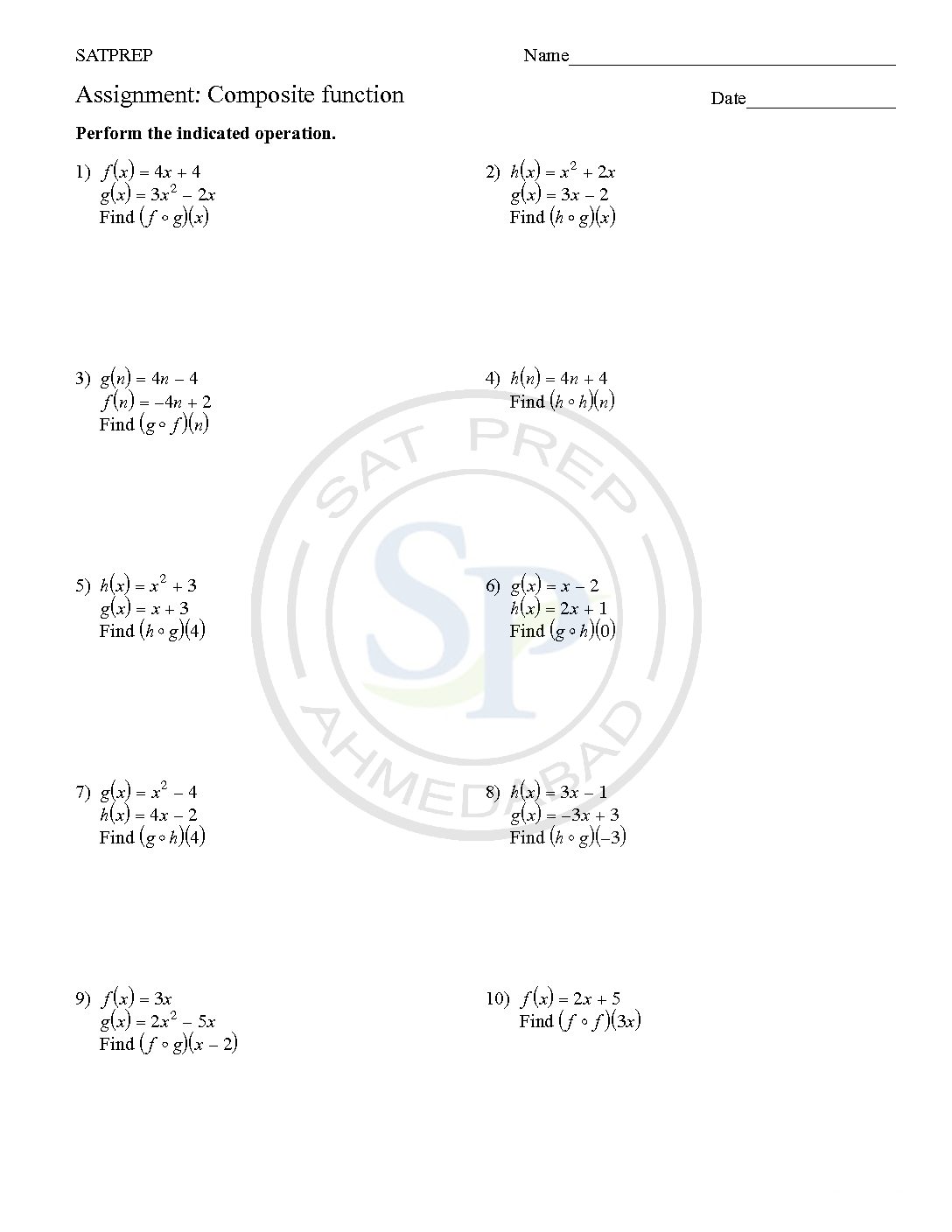
Composite Function
Composite functions are defined as one function defined inside another function. Like ff(x)) or f(g(x)) etc. Another function composition is an operation that takes two functions f and g and produces a function h such that h(x) = g(f(x)) Composite function
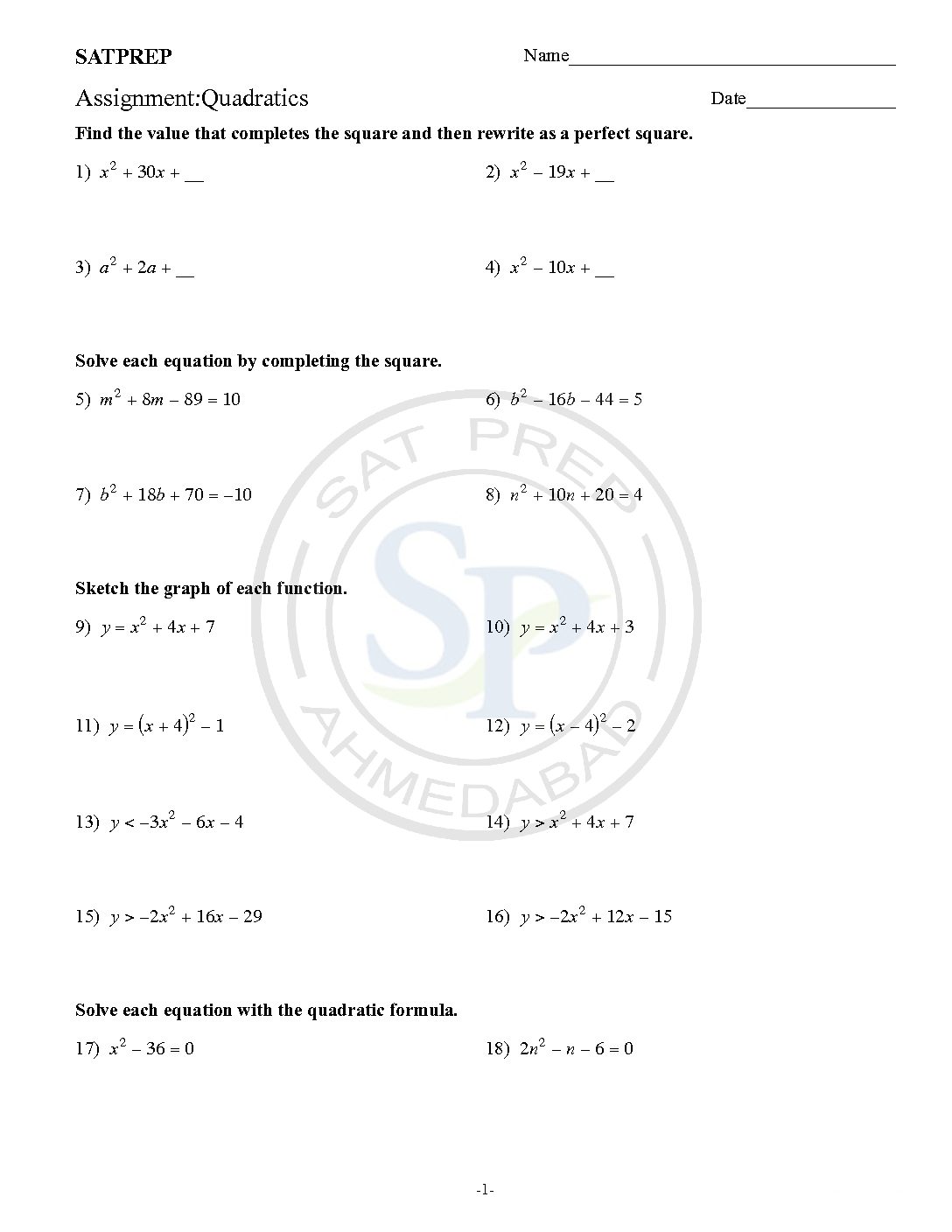
Quadratics
First of all this post consists of questions of factorisation of quadratics . The method use to find solutions are splitting middle term and another method is formula . We also find the nature of roots of equation. The method is use to find nature is discriminant (D= b2-4ac). Discriminant is also used in formula […]
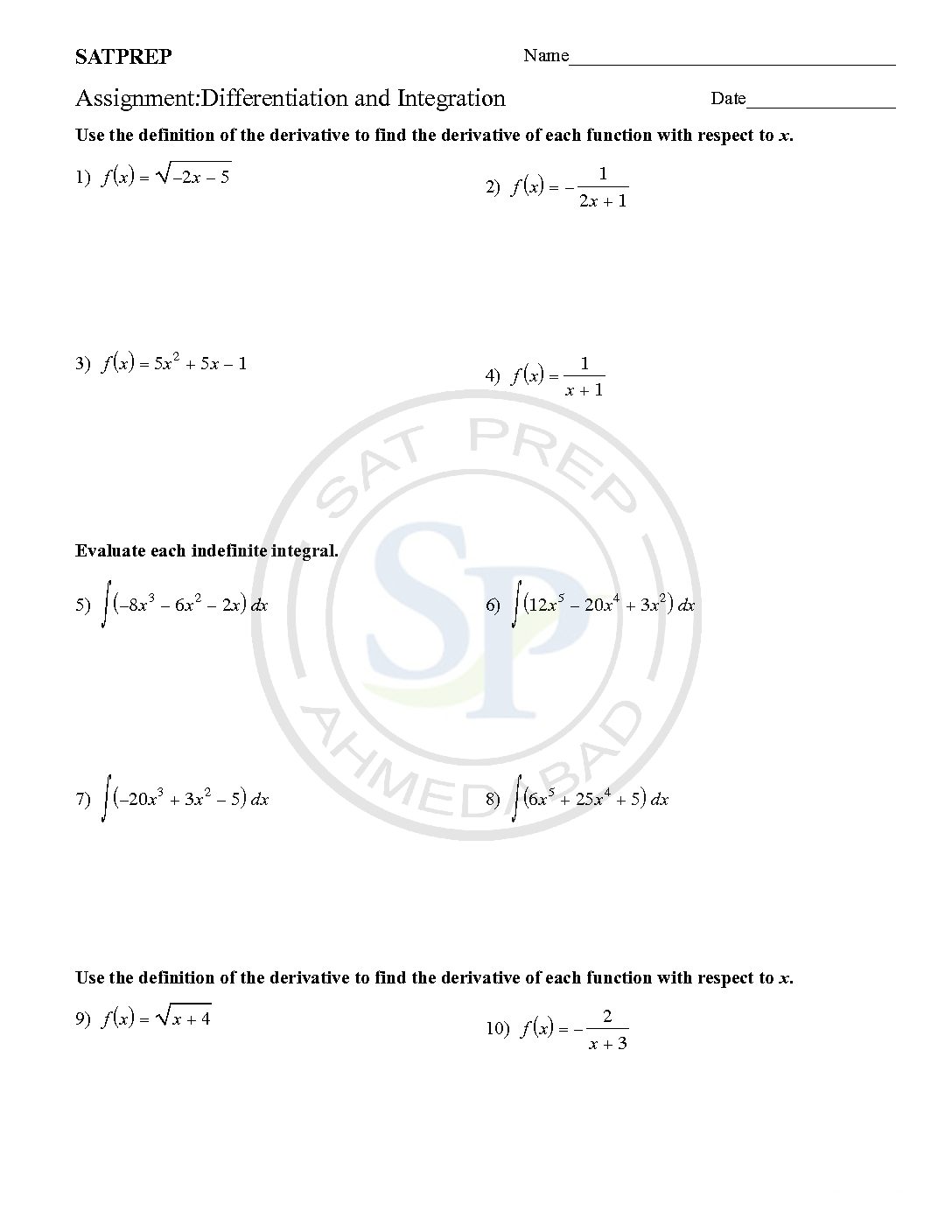
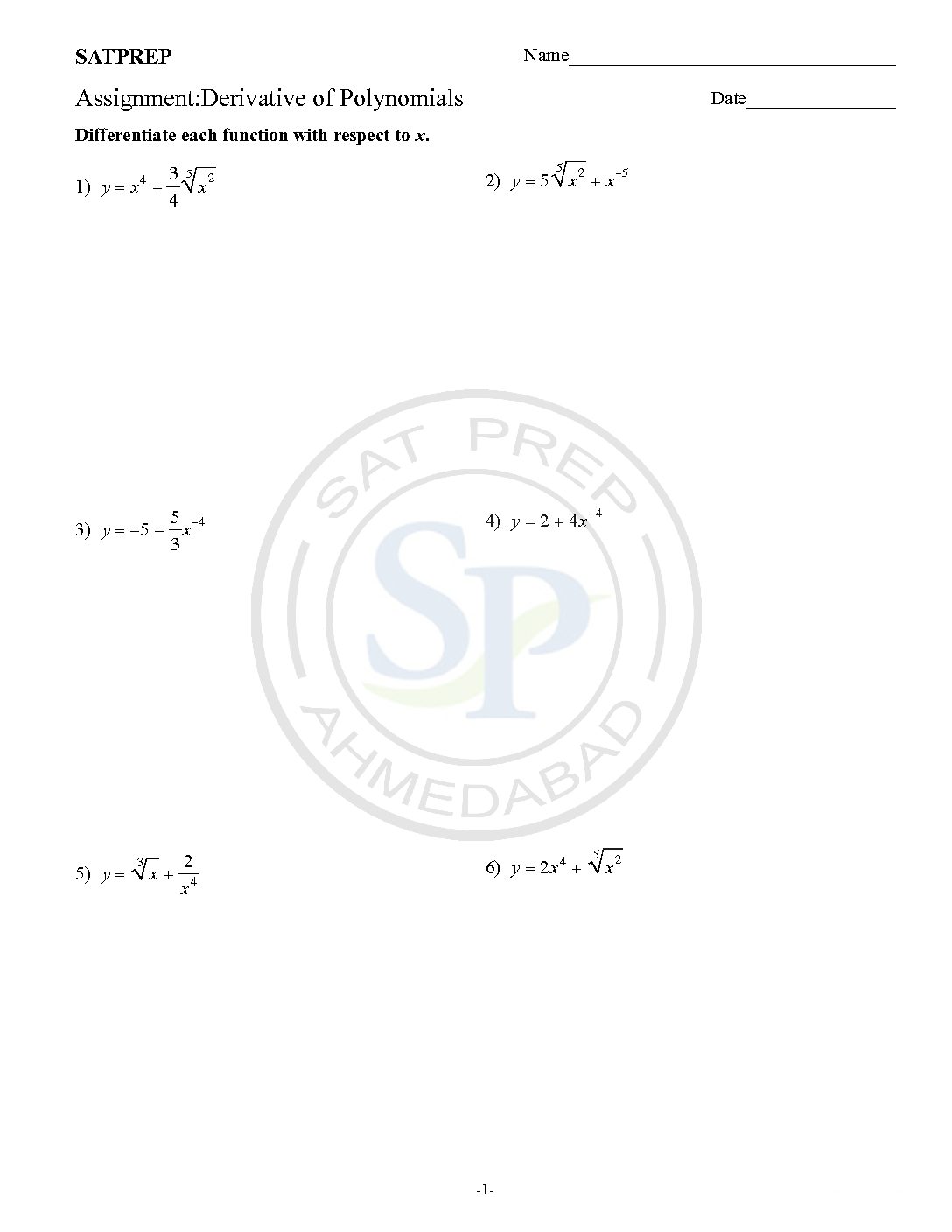
Differentiation of polynomial
Differentiation is process of getting derivative. Differentiation has applications to nearly all quantitative disciplines. For example, in physics, the derivative of the displacement of a moving body with respect to time is the velocity of the body, and the derivative of velocity with respect to time is acceleration. Similarly in chemistry as well as Economics also derivative
Derivative
The derivative of a function of a single variable at a chosen input value. Derivative is the slope of the tangent line to the graph of the function at that point. Hence derivative of a function y = f(x) of a variable x is a measure of the rate at which the value y of the function changes with respect to the change […]
Rolle’s and MVT
Rolle’s Theorem. Then there is a number c such that a<c<b and f′(c)=0. Or, in other words f(x) has a critical point in (a,b). To see the proof of Rolle’s Theorem see the Proofs From Derivative Applications . Roll’s theorem
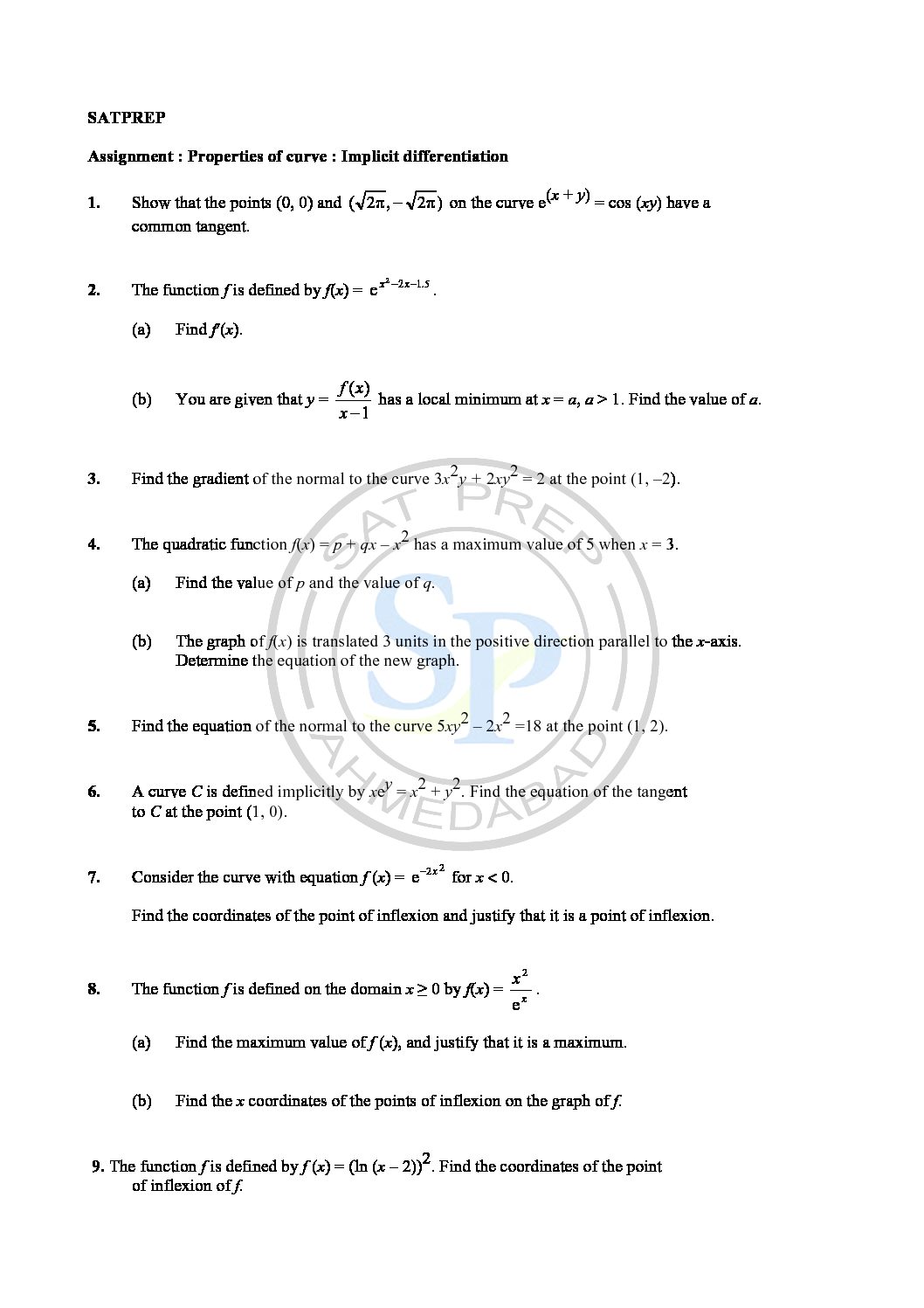
Properties of curve :Implicit
Makes use of the chain rule to differentiate implicitly defined functions. To differentiate an implicit function y ( x ), defined by an equation R ( x, y) = 0, it is not generally possible to solve it explicitly for y and then differentiate. The derivatives of inverse trig functions we’ll need the formula from the last section relating the derivatives of inverse functions. properties […]
Differentiation of Implicit and Inverse ...
In calculus, a method of implicit differentiation, Makes use of the chain rule to differentiate implicitly defined functions. To differentiate an implicit function y ( x ), defined by an equation R ( x, y) = 0, it is not generally possible to solve it explicitly for y and then differentiate. The derivatives of inverse trig functions we’ll need the formula from the last section […]
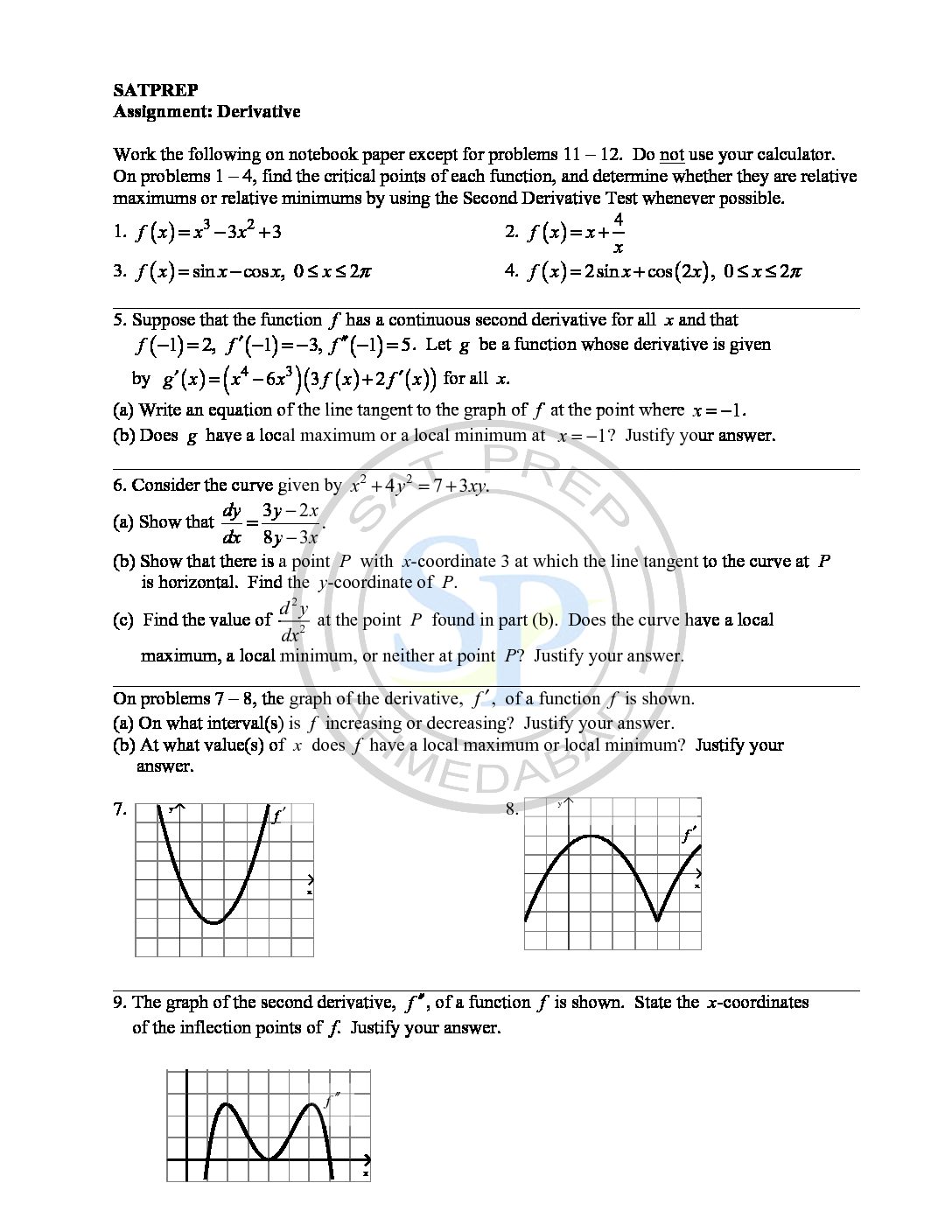
Derivative test
First Derivative Test for Local Extrema. If the derivative changes from positive (increasing function) to negative (decreasing function), the function has a local (relative) maximum at the critical point. Second Derivative Test. 1. If , then has a local minimum at . 2. If , then has a local maximum at . The extremum test gives slightly more general conditions under which […]