Process of optimisation means optimal value of function at turning point (maximum or minimum ) value of the curve. Therefore second derivative use to find greatest or least value . Also it show greatest value. Optimization
You are browsing archives for
Category: Derivatives
Related Rates -2
First of all related rate problems are applications of derivative . One of the hardest calculus problems that students have trouble . Because each application question has a different approach in solving the problem. Hence called rate of change. Related rates
Kinematics
Describes the motion of points, objects and systems of groups of objects, without reference to the causes of motion. The symbol a stands for the acceleration of the object. And the symbol v stands for the instantaneous velocity of the object. The derivative of displacement with time is velocity ( v = ds/dt ). The […]
Optimisation
Process of calculating optimal value of function at turning point (maximum or minimum )value of the curve. Hence we use second derivative. It also give greatest or least value of curve. Optimisation
Related Rates
Rate of change is also related rate problems are applications of derivative . One of the hardest calculus problems that students have trouble . Because each application question has a different approach in solving the problem. Ralated rates
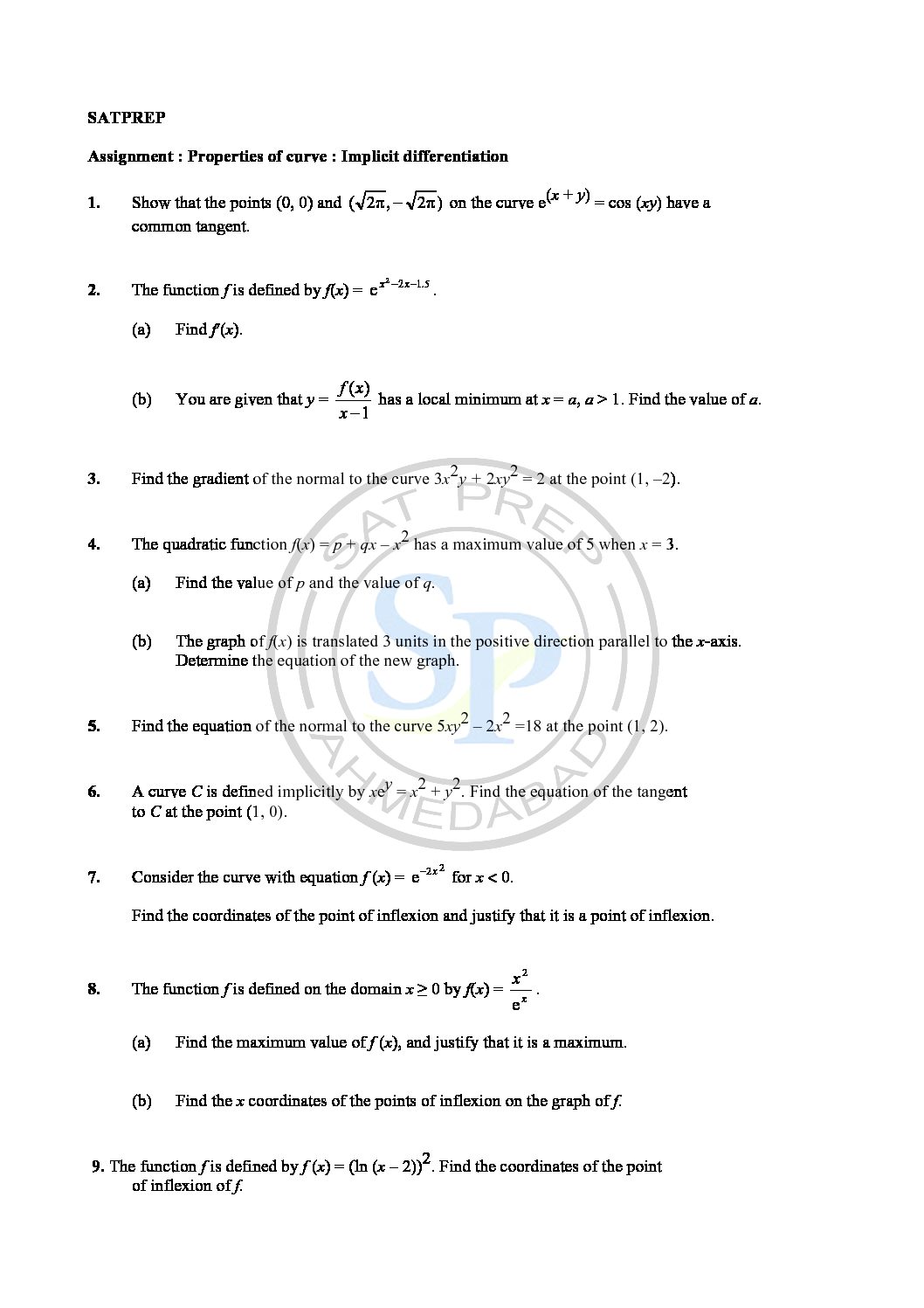
Properties of curve :Implicit
Makes use of the chain rule to differentiate implicitly defined functions. To differentiate an implicit function y ( x ), defined by an equation R ( x, y) = 0, it is not generally possible to solve it explicitly for y and then differentiate. The derivatives of inverse trig functions we’ll need the formula from the last section relating the derivatives of inverse functions. properties […]
Properties of curves
properties of curves follow from its definition and the assumptions upon which indifference curve approach is based. Indifference curves have a negative slope. Also zero slope at turning point. Hence tangent is horizontal. Further also point of inflexion. Properties of curve
Differentiation of Implicit and Inverse ...
In calculus, a method of implicit differentiation, Makes use of the chain rule to differentiate implicitly defined functions. To differentiate an implicit function y ( x ), defined by an equation R ( x, y) = 0, it is not generally possible to solve it explicitly for y and then differentiate. The derivatives of inverse trig functions we’ll need the formula from the last section […]
Derivative test
First Derivative Test for Local Extrema. If the derivative changes from positive (increasing function) to negative (decreasing function), the function has a local (relative) maximum at the critical point. Second Derivative Test. 1. If , then has a local minimum at . 2. If , then has a local maximum at . The extremum test gives slightly more general conditions under which […]