You are browsing archives for
Category: Limits
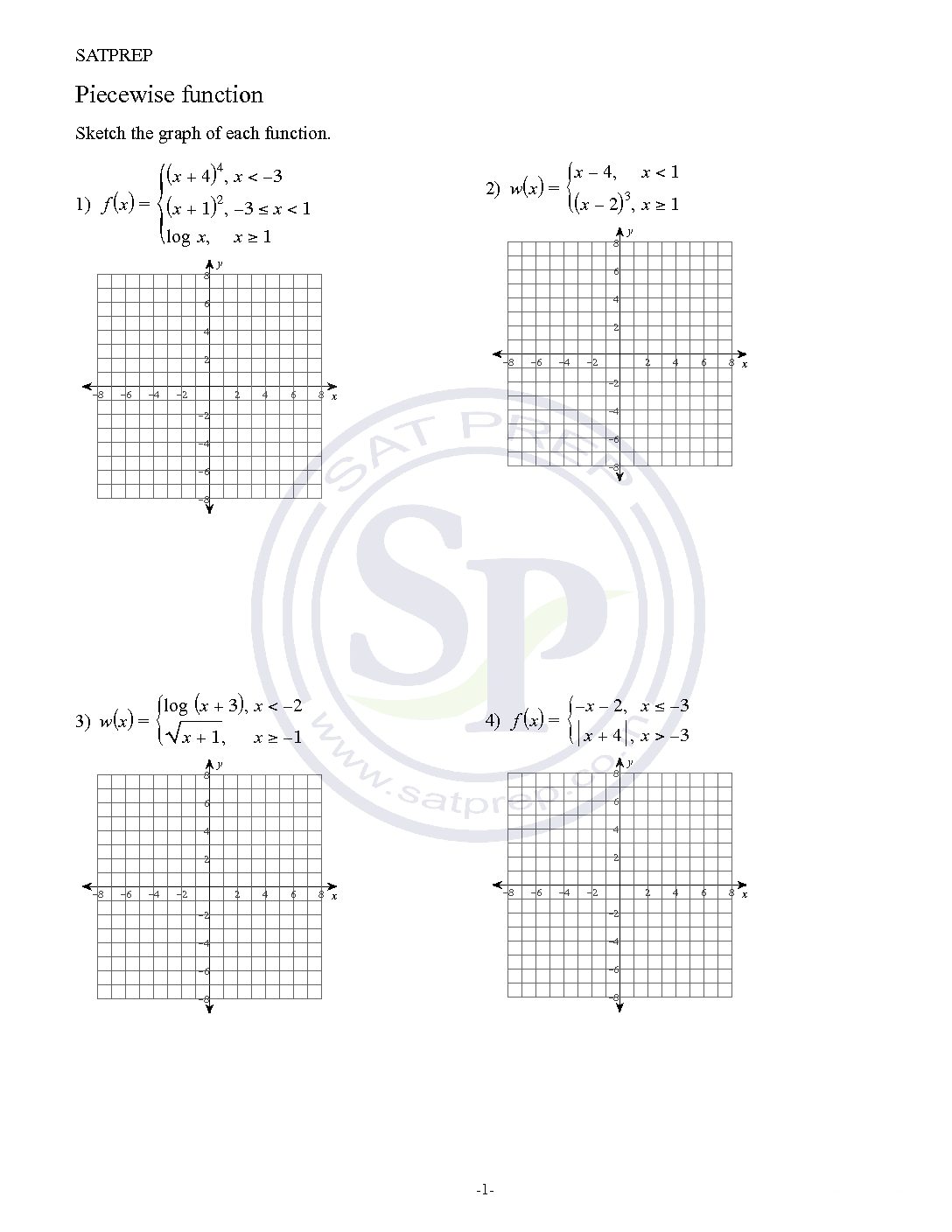
Piecewise Function
Functions that behave differently based on the input (x) value. Piecewise Function. A function made up of 3 pieces as well as two pieces. Hence in some case piecewise function give discontinuous graph. Piecewise
Trigonometric limit
The trigonometric functions sine and cosine function. Limits and Continuity of Functions. Although we can use both radians and degrees, 𝑟𝑎𝑑𝑖𝑎𝑛𝑠radians are a more natural measurement because they are related directly to the unit circle, a circle with radius 1. trigonometric limit
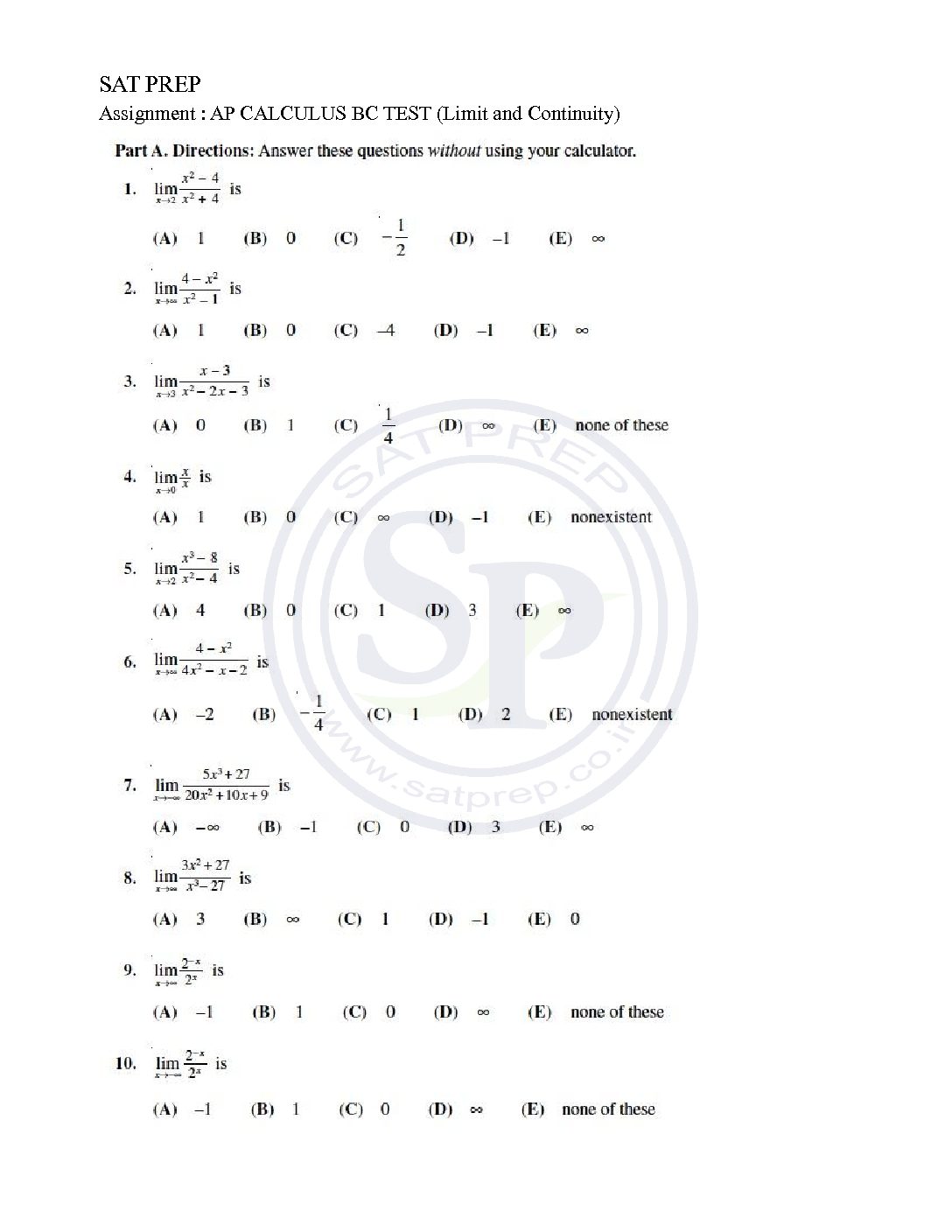
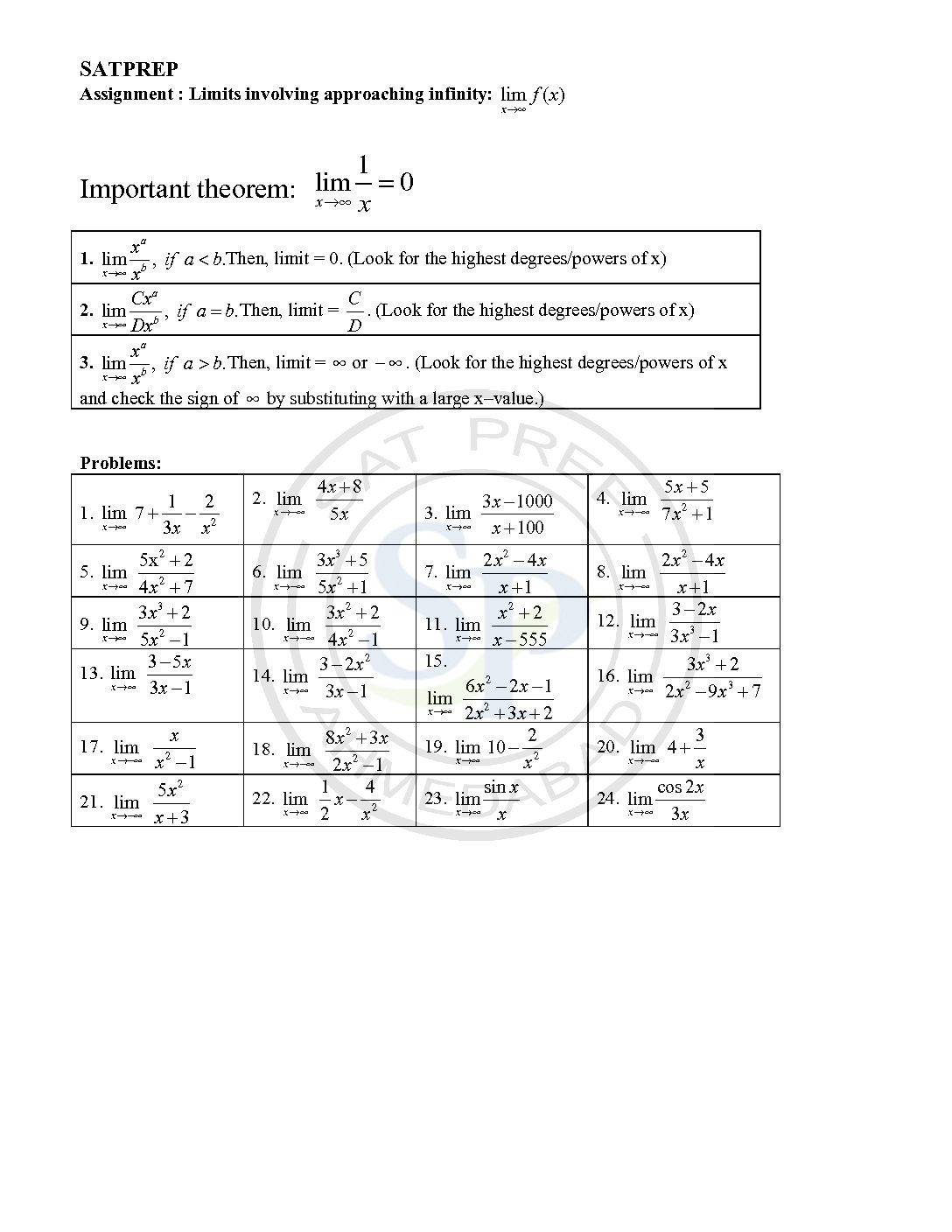
Limit
Limits are the concept of function. So, we have to solve function for given limits. Due to different type of limits , function cannot solve. limit
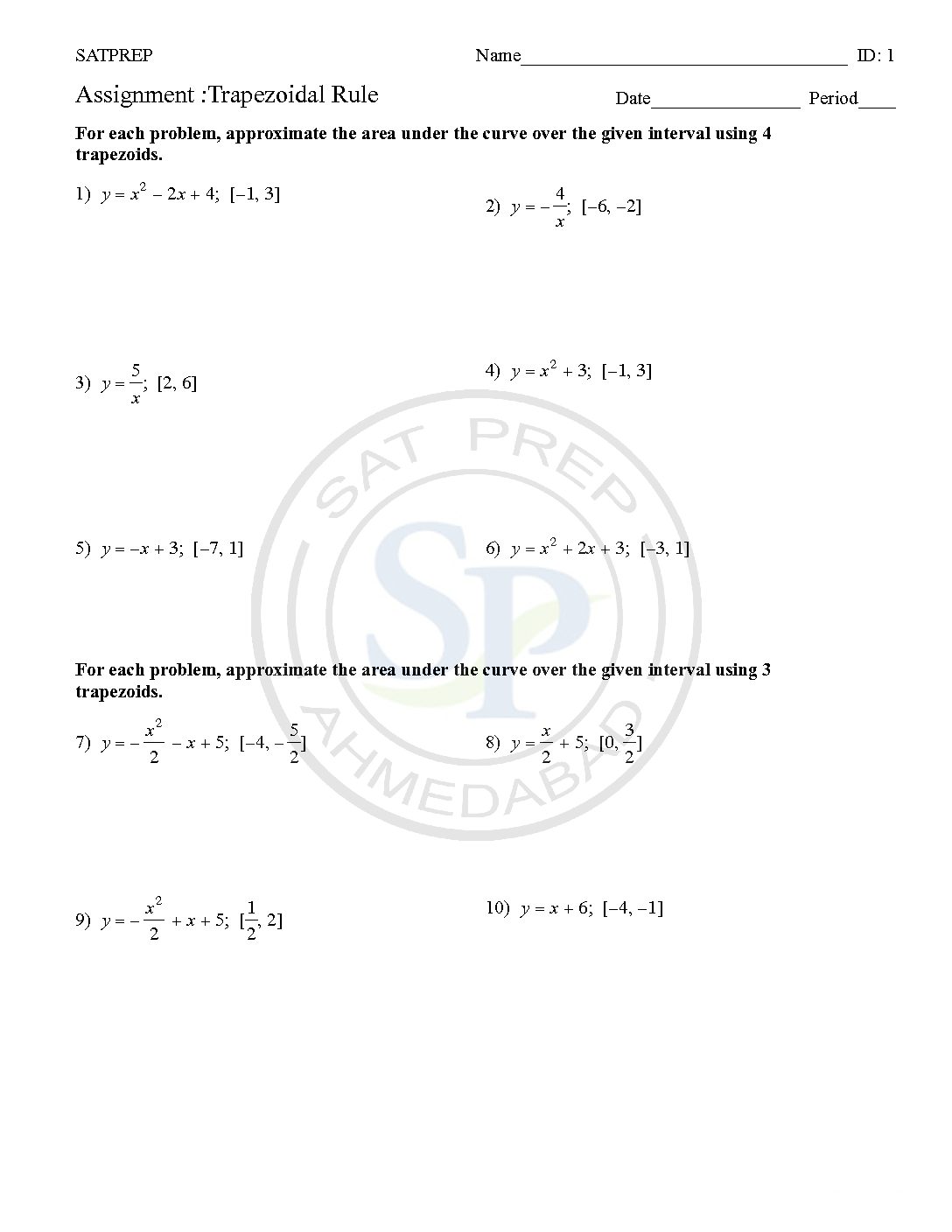
Trapezoidal Rule-2
trapezium rules is a way of estimating the area under a curve. so the trapezium rule gives a method of estimating integrals. Due to this we get approximate answer . Also we can get area using integration. trapezium rule
Trapezoidal Rule
The trapezium rules is a way of estimating the area under a curve. so the trapezium rule gives a method of estimating integrals. Due to this we get approximate answer . Also we can get area using integration. trapezium Rule
Riemann sum( table)
A Riemann sums is an approximation of a region’s area. It obtained by adding up the areas of multiple simplified slices of the region. It is applied in calculus to determine the area of a region. Hence it give approximate area of region. It is also give right and left sum. Riemann sum
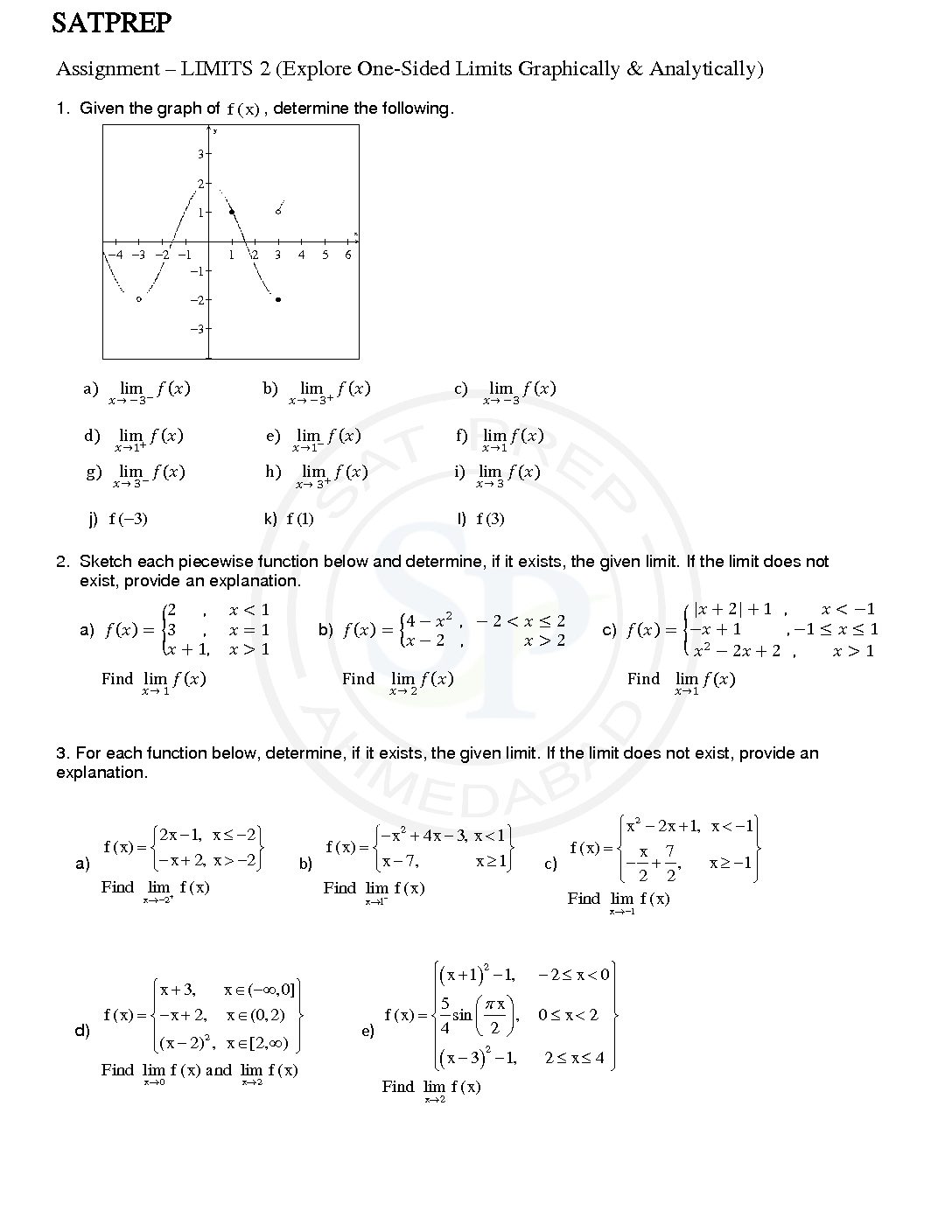
LIMITS (One-Sided Limits )
LHL and RHL Limits are the concept of function. So, we have to solve function for given limits. Due to left side it is LHL and right side RHL Limit( One side limit)
Continuity
Continuity Theorems : a function is continuous if function is LHL = f(x) = RHL the function f (x) is continuous at that point. continuous
Limits – Algebraic
LHL and RHL are the limit concept of function. So, we have to solve function for given limits. Due to different type of limits , function cannot solve. Limits