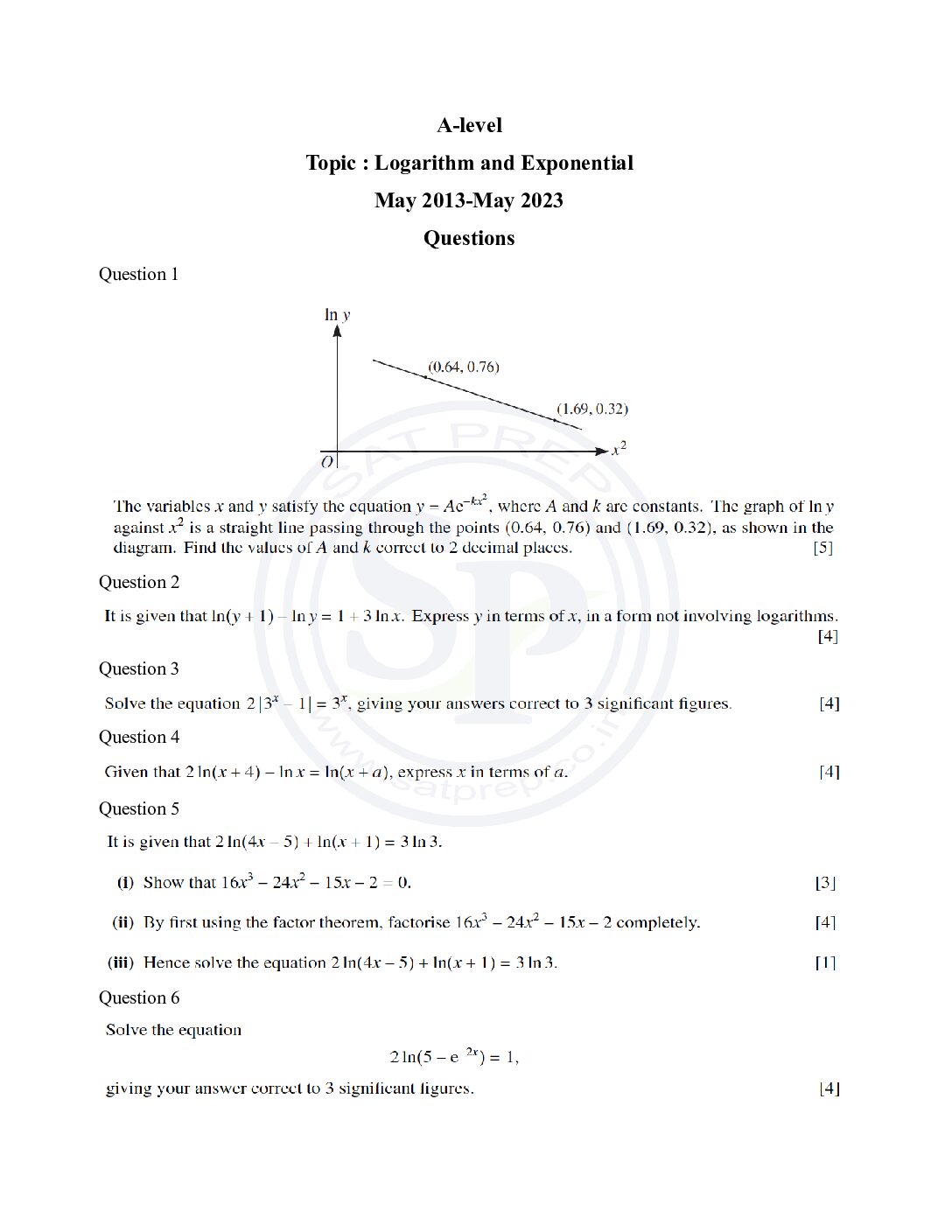
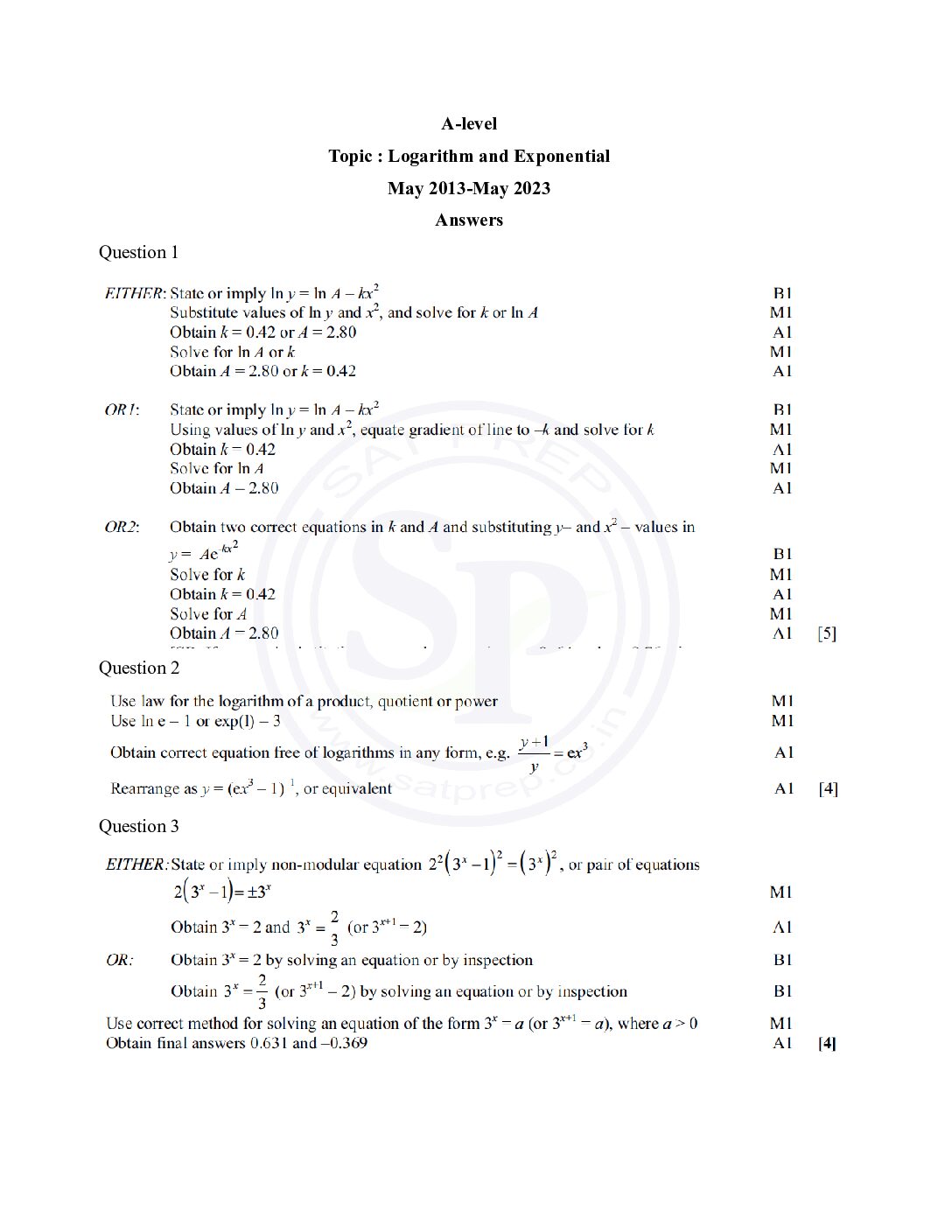
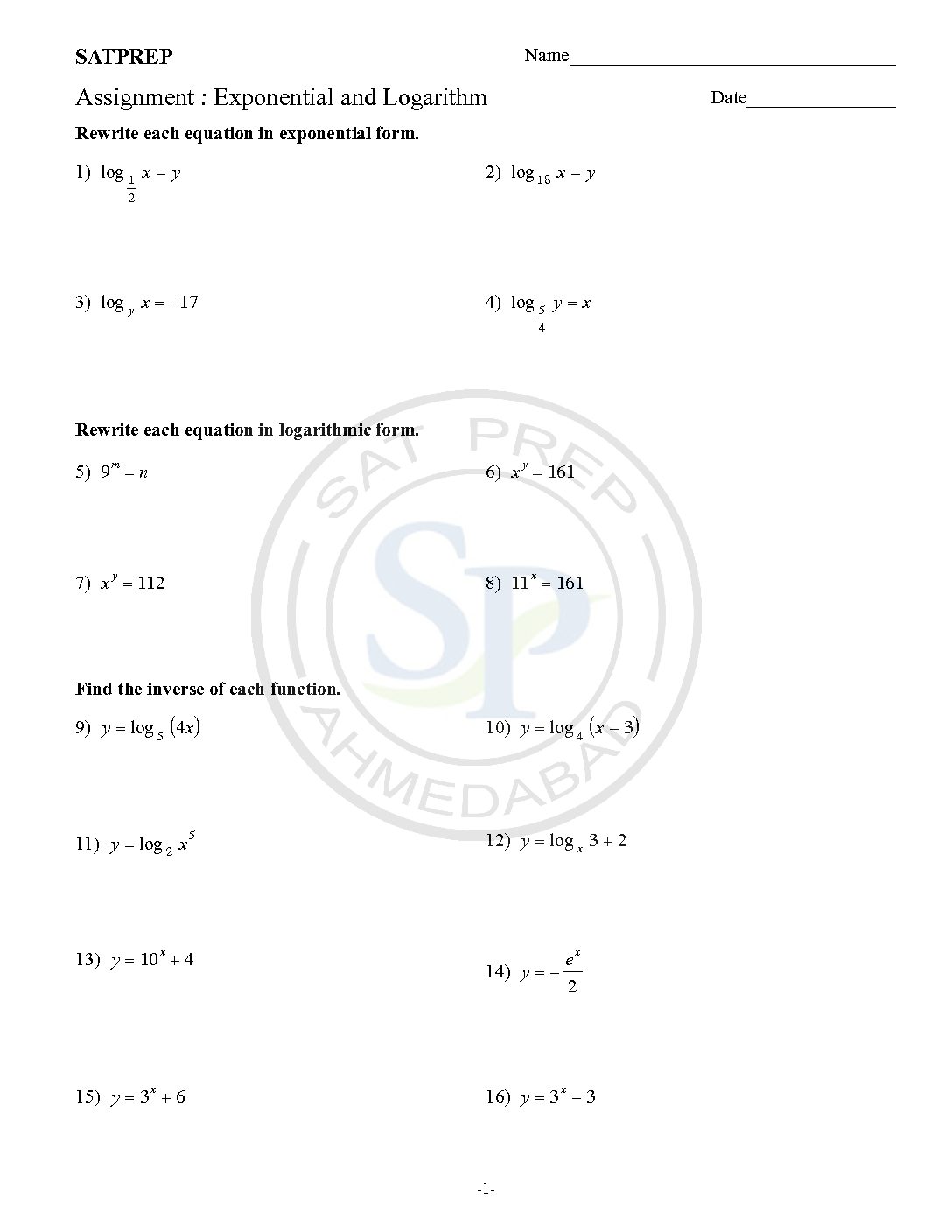
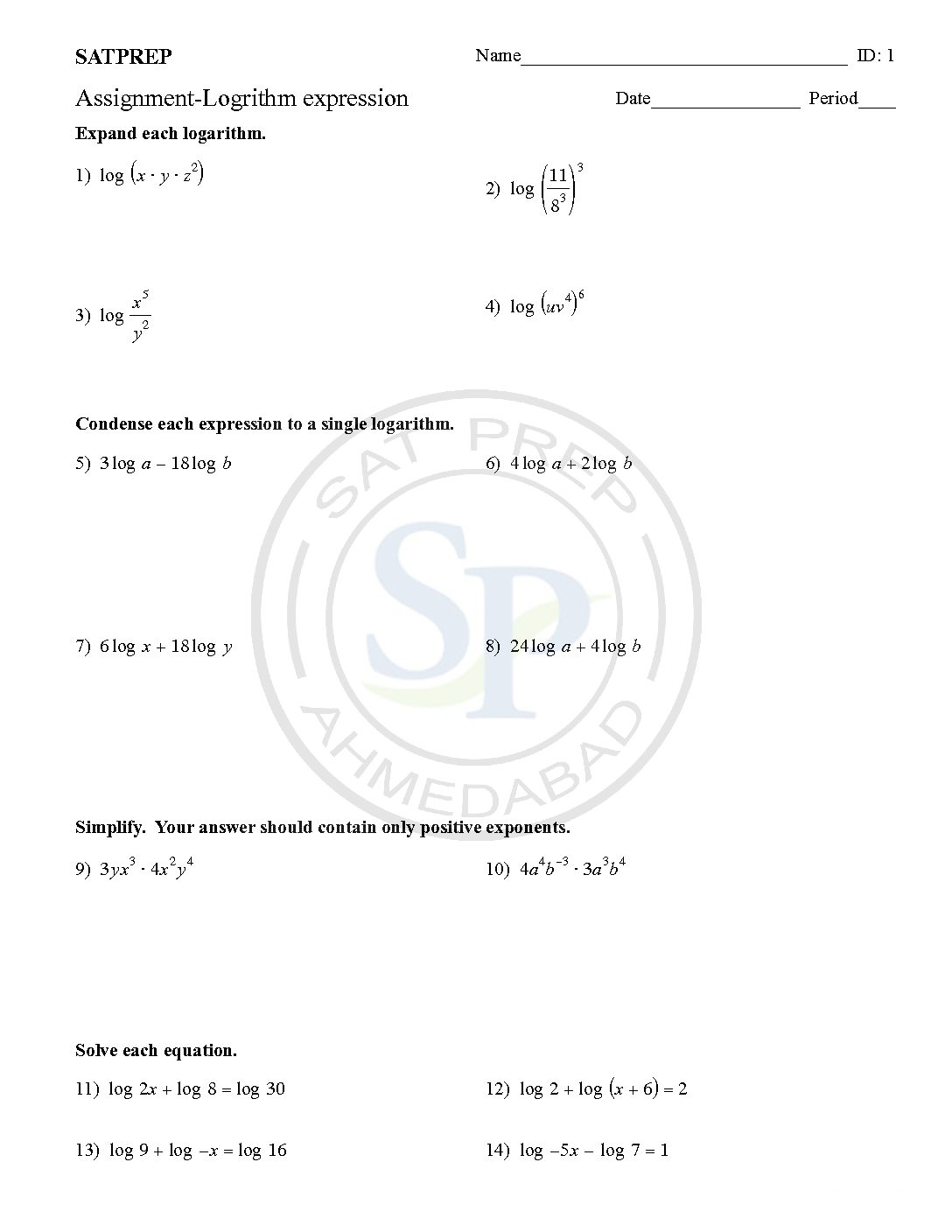
You are browsing archives for
Category: Logirthmic and Exponential
Topic wise A-Level Pure Math Past Paper ...
Function-Exponent and logarithm
This post is about Exponential and Logarithm function . These function are inverse to each other. Therefore graph function are reflection about line y = x . The questions are to change exponent to log and reverse. Exponential and Logarithm P P
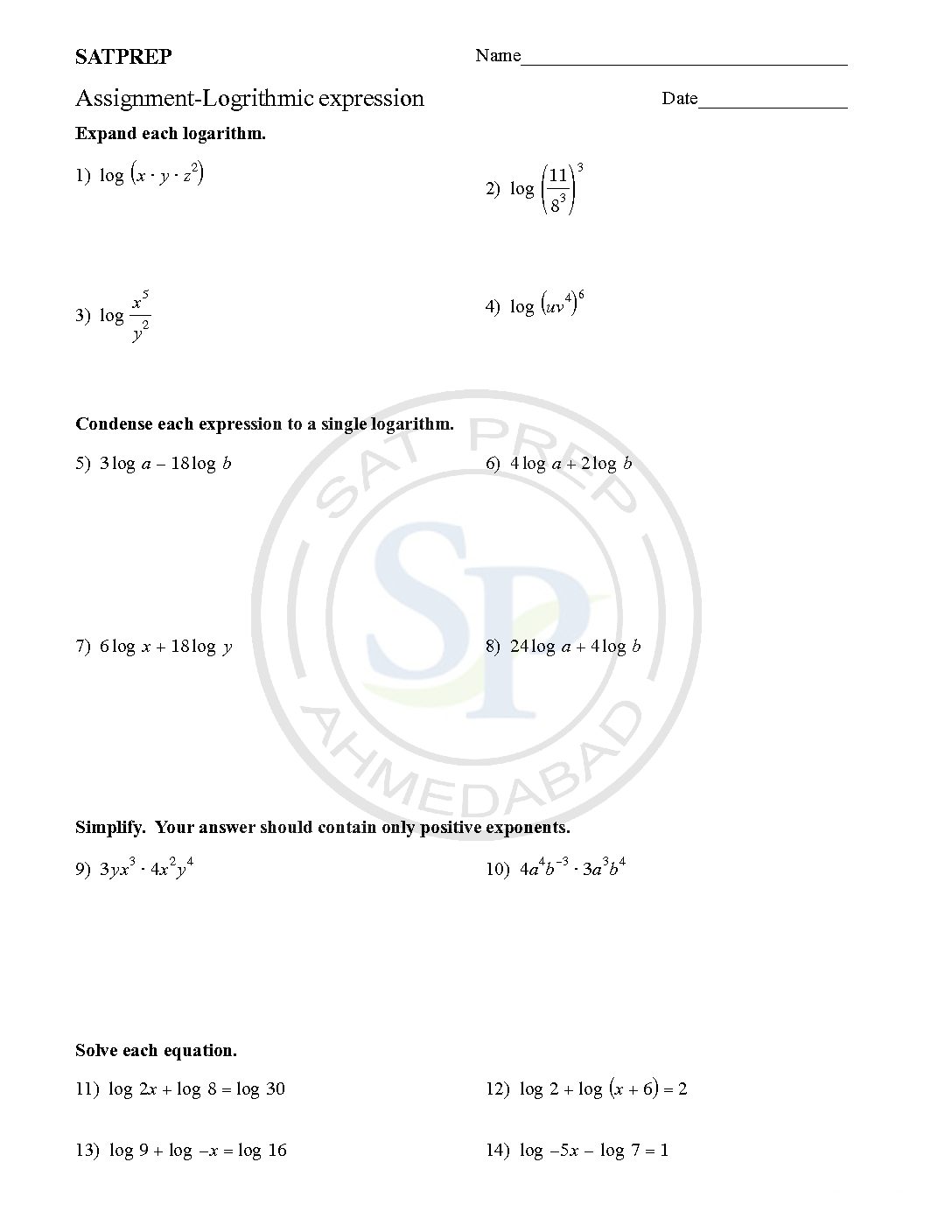
Logarithmic Expression
Logarithmic Expressions are simplify by Rules or Laws of Logarithms and solve by inverse of exponentiation. It is expressed by using the abbreviation “log”. Because of different base of number log notation are also different. lg for base 10 , ln for base e . Logarithm quotient rule The logarithm of the division of x and y is the difference of logarithm of x […]
Logarithmic Expression
Solve the logarithmic equation. This problem involves the use of the symbol “ln” instead of “log” to mean logarithm. Logarithmic expression
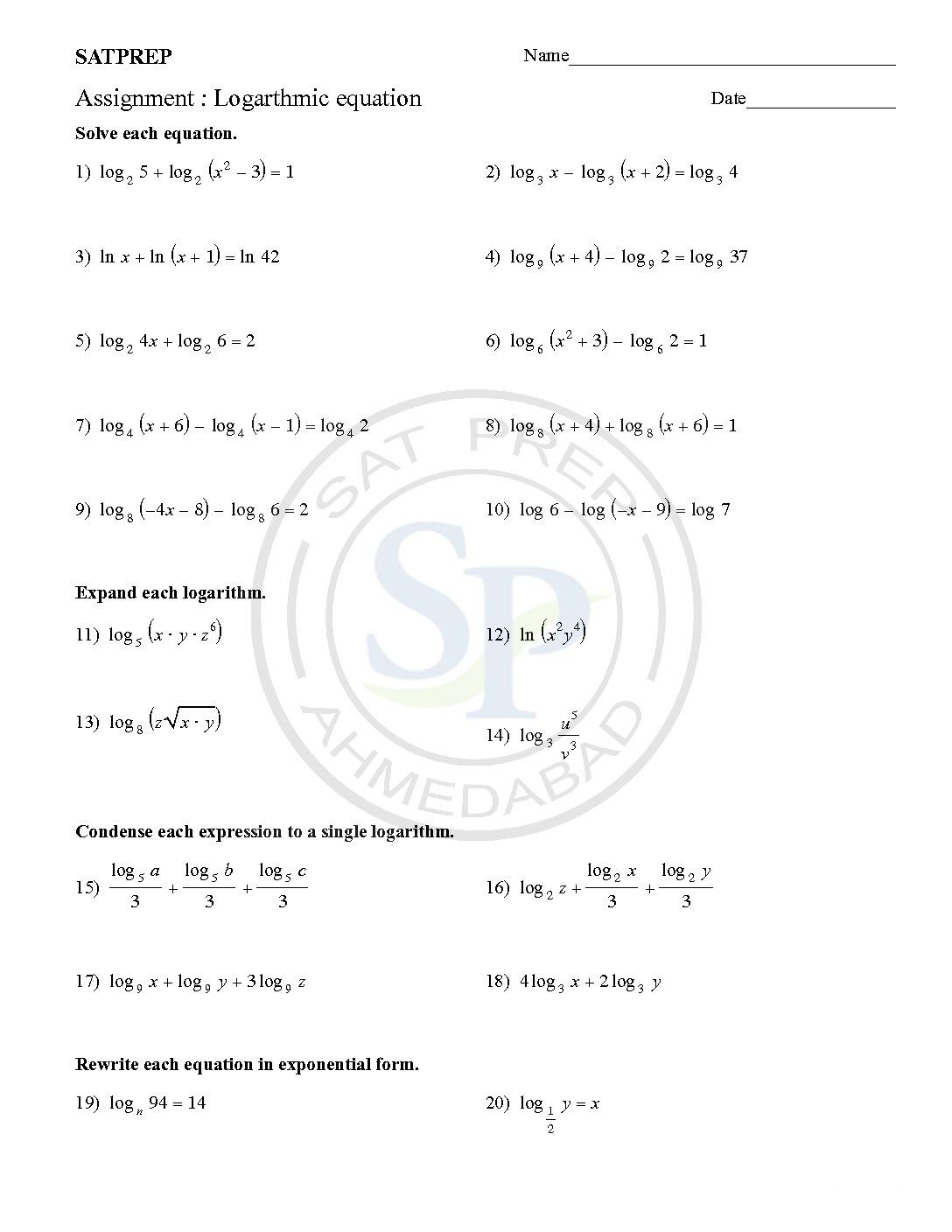
Logarthmic Equation
Logarithms equation can be solved using the properties of logarithms. So equation either are solve by log or exponent. Because both inverse of each other hence are either way exponent or log.In both apply properties of log or exponent. Also we need to factorised to solve. Logarithmic eq
Logarithmic and exponential Function
The logarithmic function y = logax is defined to be equivalent to the exponential equation x = ay. y = logax only under the following conditions: x = ay, a > 0, and a≠1. Logarithmic functions are the inverses of exponential functions. The inverse of the exponential function y = ax is x = ay. […]
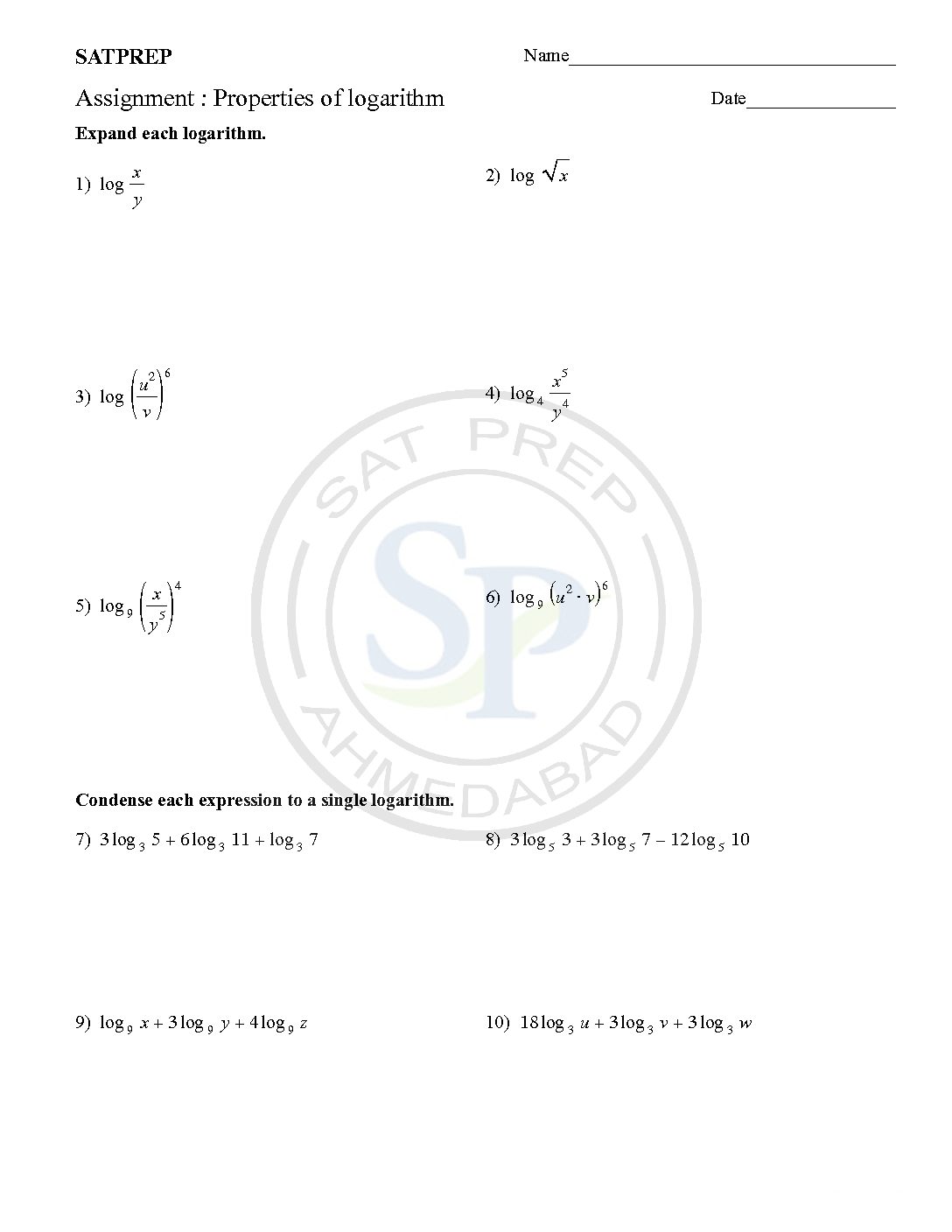
Properties of logarithm
Properties of logarithms are use to evaluate or rewrite logarithmic expressions. Product Rule : ln x+ ln y = ln xy Quotient Rule : ln x – ln y = ln xy Power Rule : ln yx = x ln y Properties of logarithm
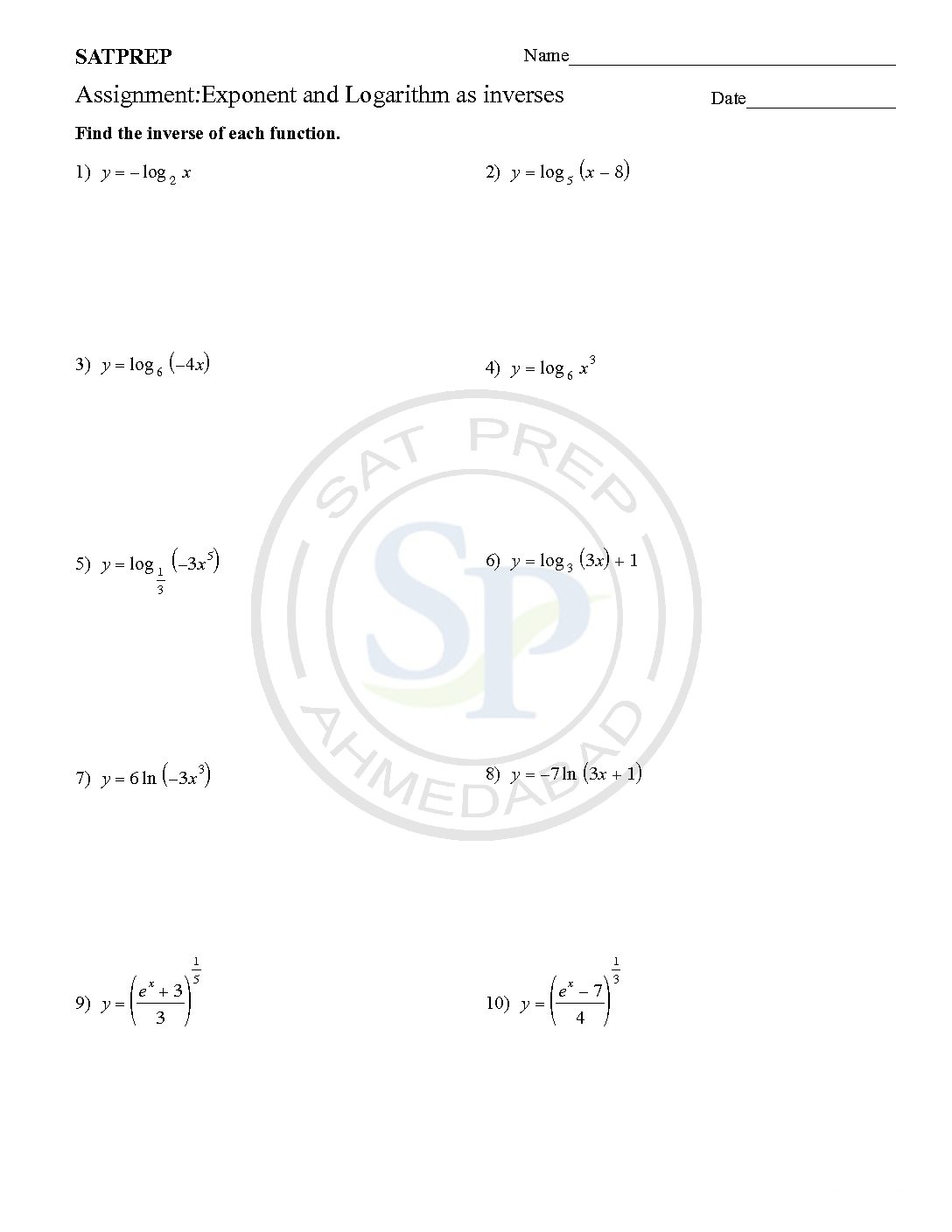
Exponent and Logarithm inverses
The inverse of the exponential function y = ax is x = ay. The logarithmic function y = logax is defined to be equivalent to the exponential equation x = ay. y = logax only under the following conditions: x = ay, a > 0, and a≠1. It is called the logarithmic function with base […]
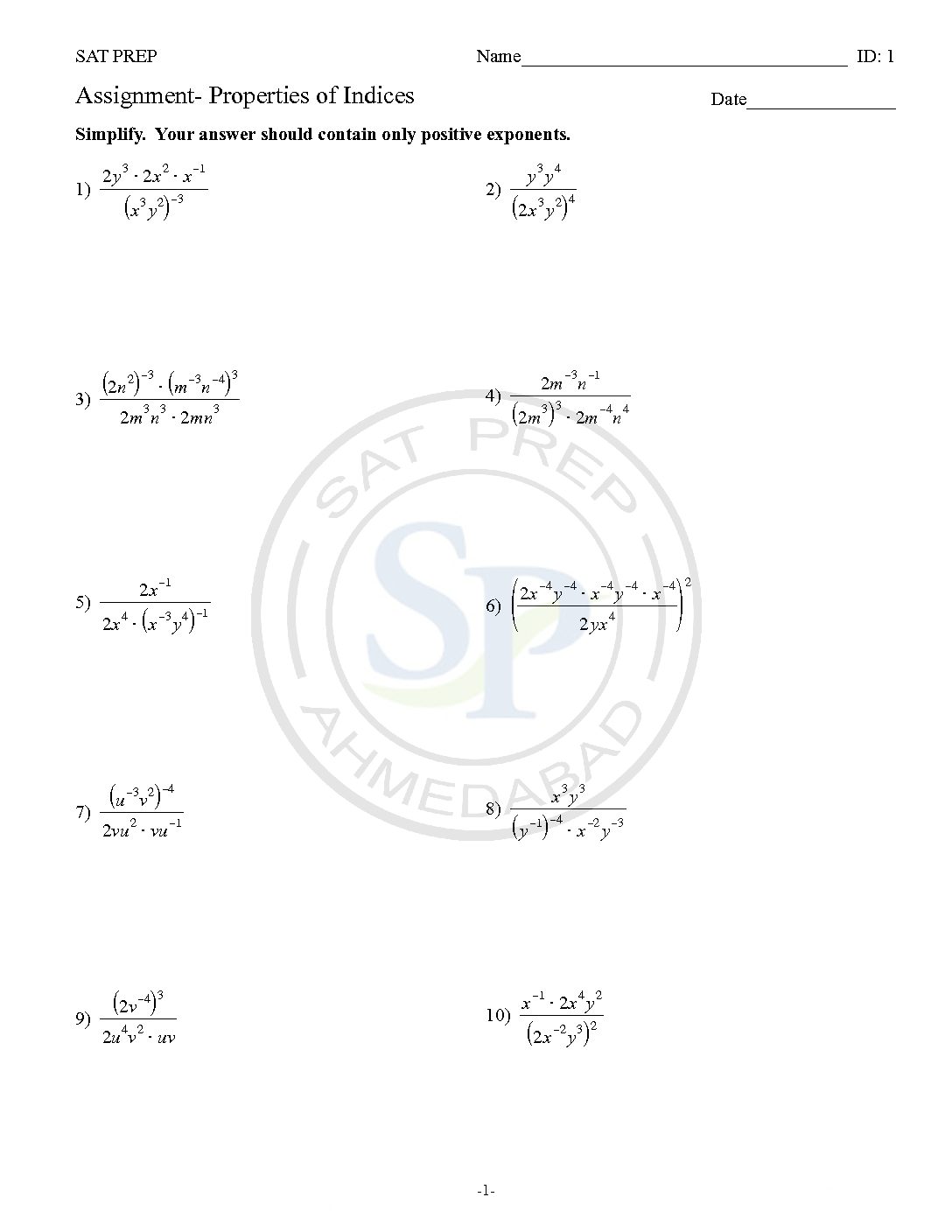
Properties of indices
Laws of Exponents. Exponents are also called Powers or Indices. The exponent of a number says how many times to use the number in a multiplication. Law of Indices. To manipulate expressions, we can consider using the Law of Indices. These laws only apply to expressions with the same base, for example, 3 4 and 3 2 can be manipulated […]