Cross Products. A vector has magnitude (how long it is) and direction: Two vectors can be multiplied using the “Cross Product” (also see Dot Product) The Cross Product a × b of two vectors is another vector that is at right angles to both: Cross product
You are browsing archives for
Category: Vectors
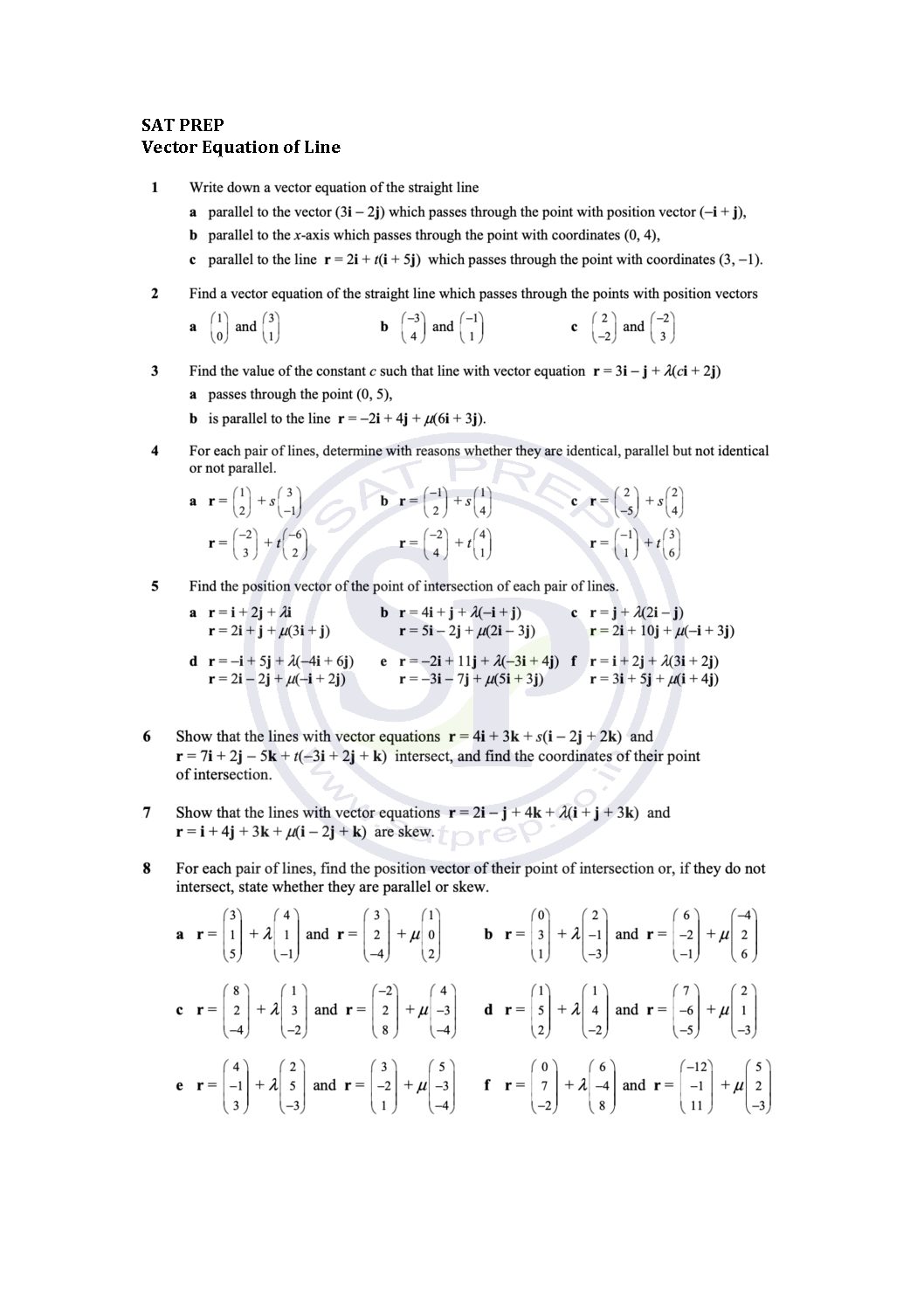
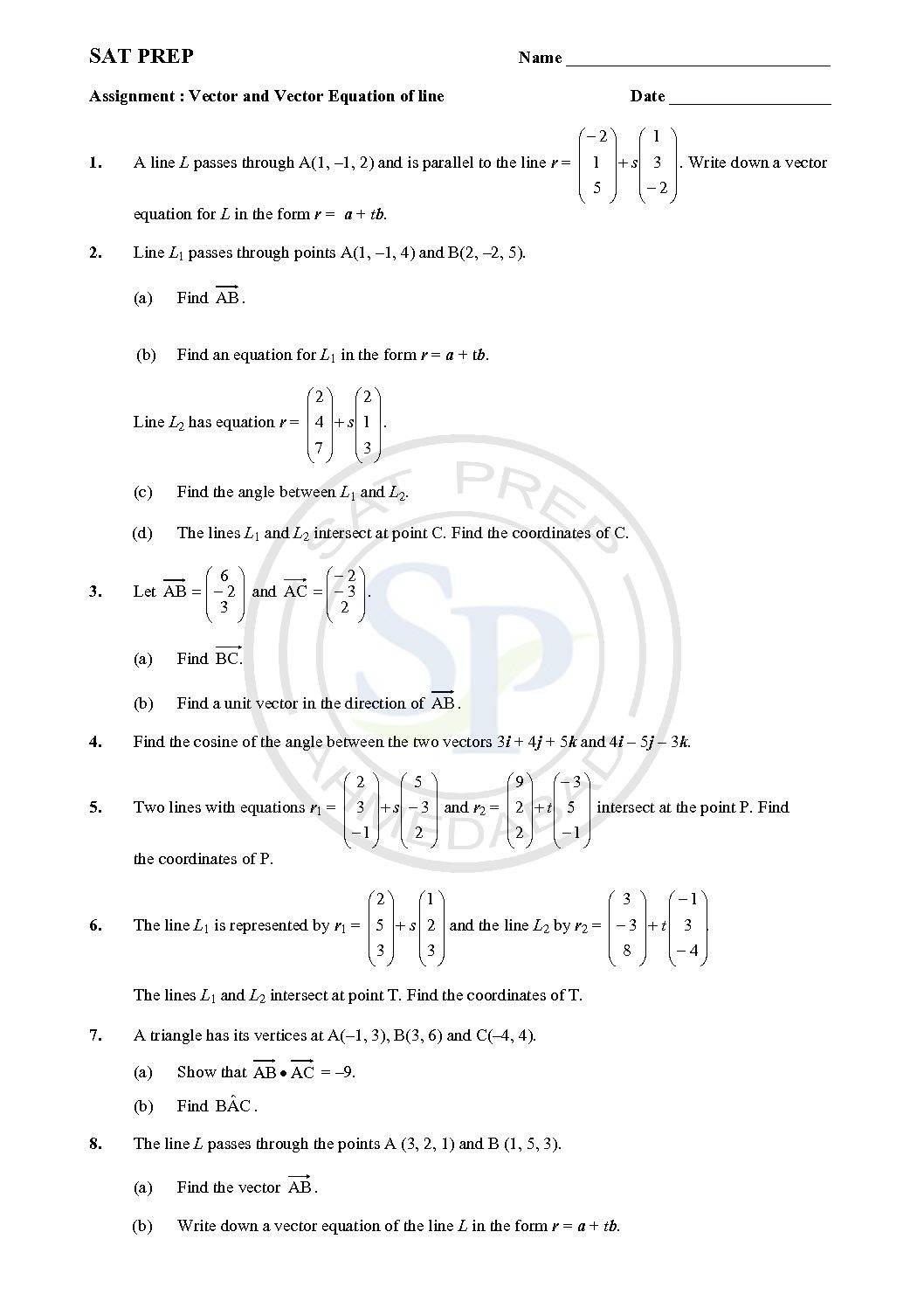
Vector Equation of Line and applications
Vector equation of line and planes
This post about worksheet of vector and planes. This worksheet consist of Vector equation of line and planes questions. www.ibo.org
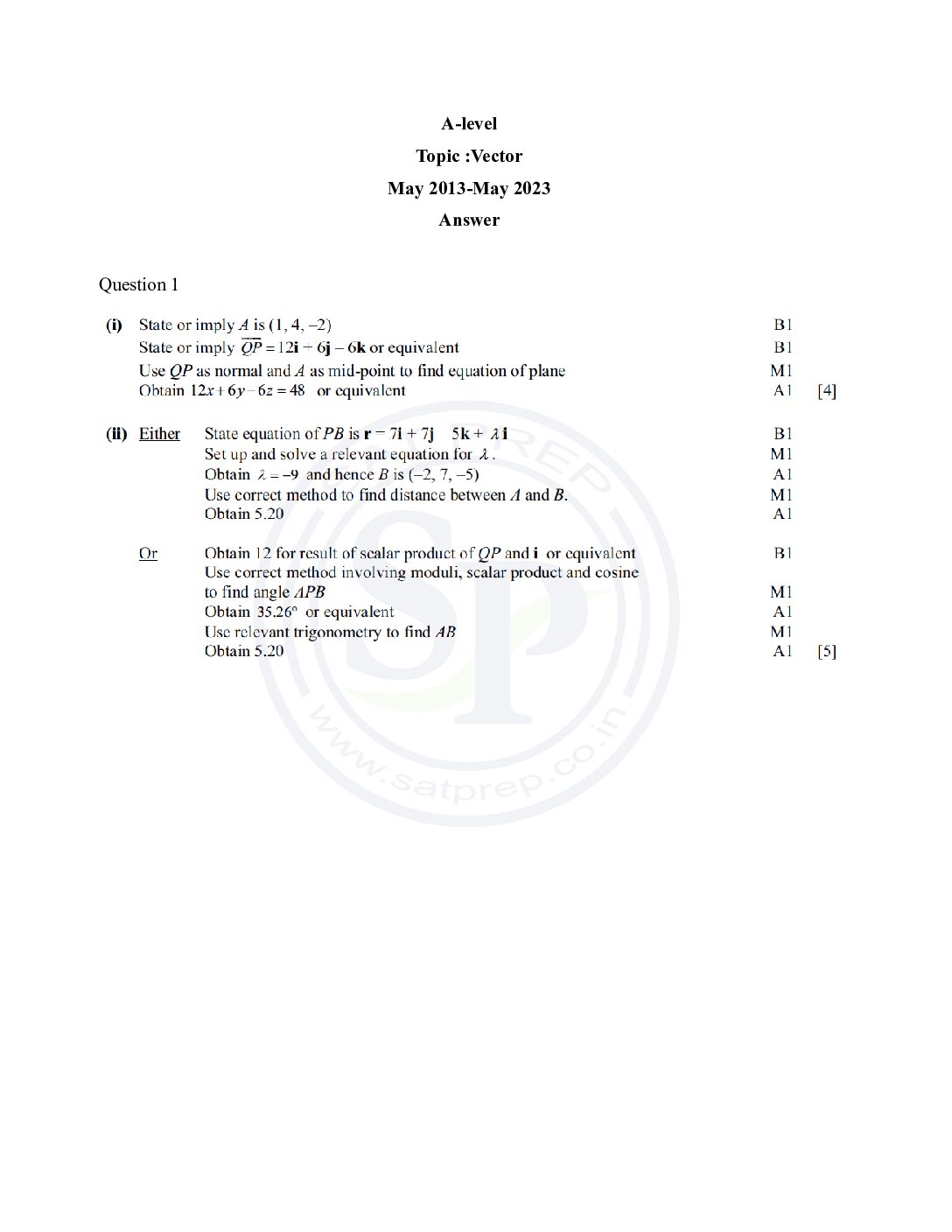
Topic wise A-Level Math Past Paper Vect...
This post is about solution of question of vector from A-level Math past paper. In this post questions are from different paper from May 2013 to May 2023. By this post students will come to know variety of questions asked in previous year papers. The questions type in this post is calculator . www.cie.org.uk
Topic wise A-Level Math Past Paper Vecto...
This post is about question of vector from A-level Math past paper. In this post questions are from different paper from May 2013 to May 2022. By this post students will come to know variety of questions asked in previous year papers. The questions type in this post is calculator . www.cie.org.uk
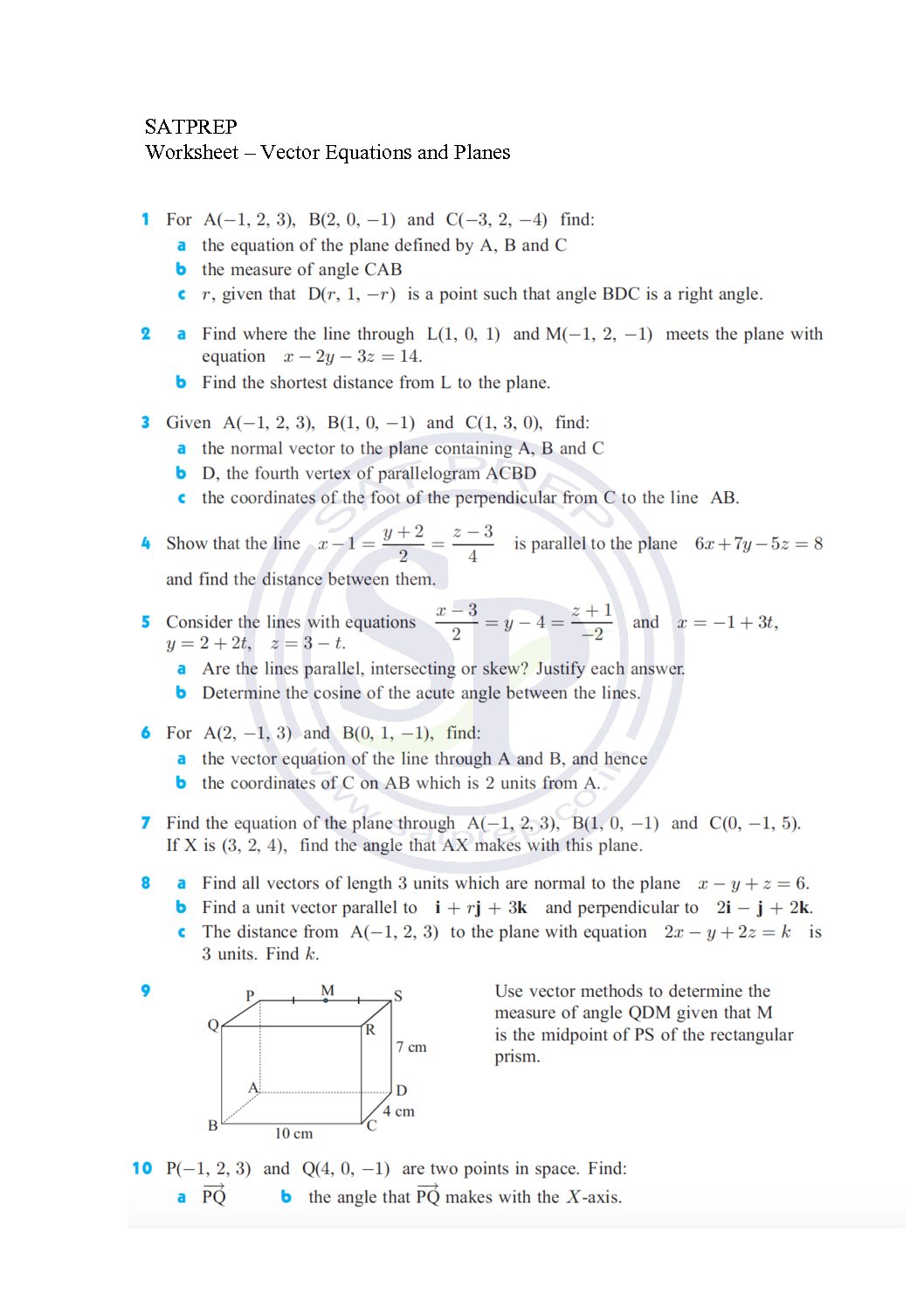
Equation of plane and applications
A plane is determined by a point P in the plane and a normal vector n perpendicular to the plane. Since each vector in the plane must be orthogonal to the normal vector n. Equations is called a vector equation of the plane. Vector equation of a plane. a plane may be characterized by a point contained in the plane and a vector that is perpendicular, or normal, to the plane. Therefore equation plane also called cartesian equation. Plane […]
Vector and vector equation of line
The vector is direction one point to another point. The vector equation of a line is r = a + tb. In this equation, “a” represents position vector and “b” represents a direction vector of the line. Moreover “r” represents the vector of any general point on the line and “t” is constant. Hence it is similar to equation of line vector
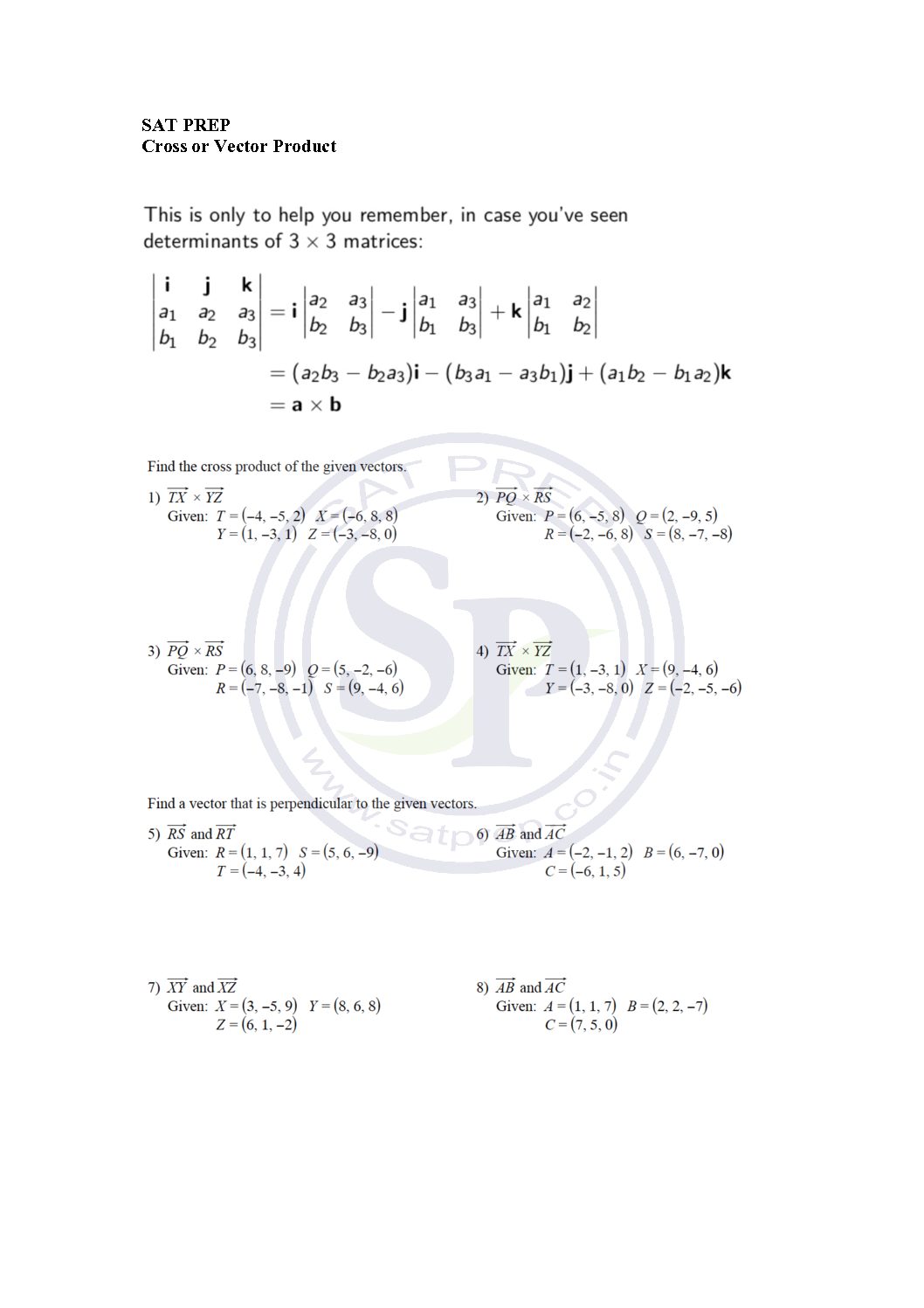
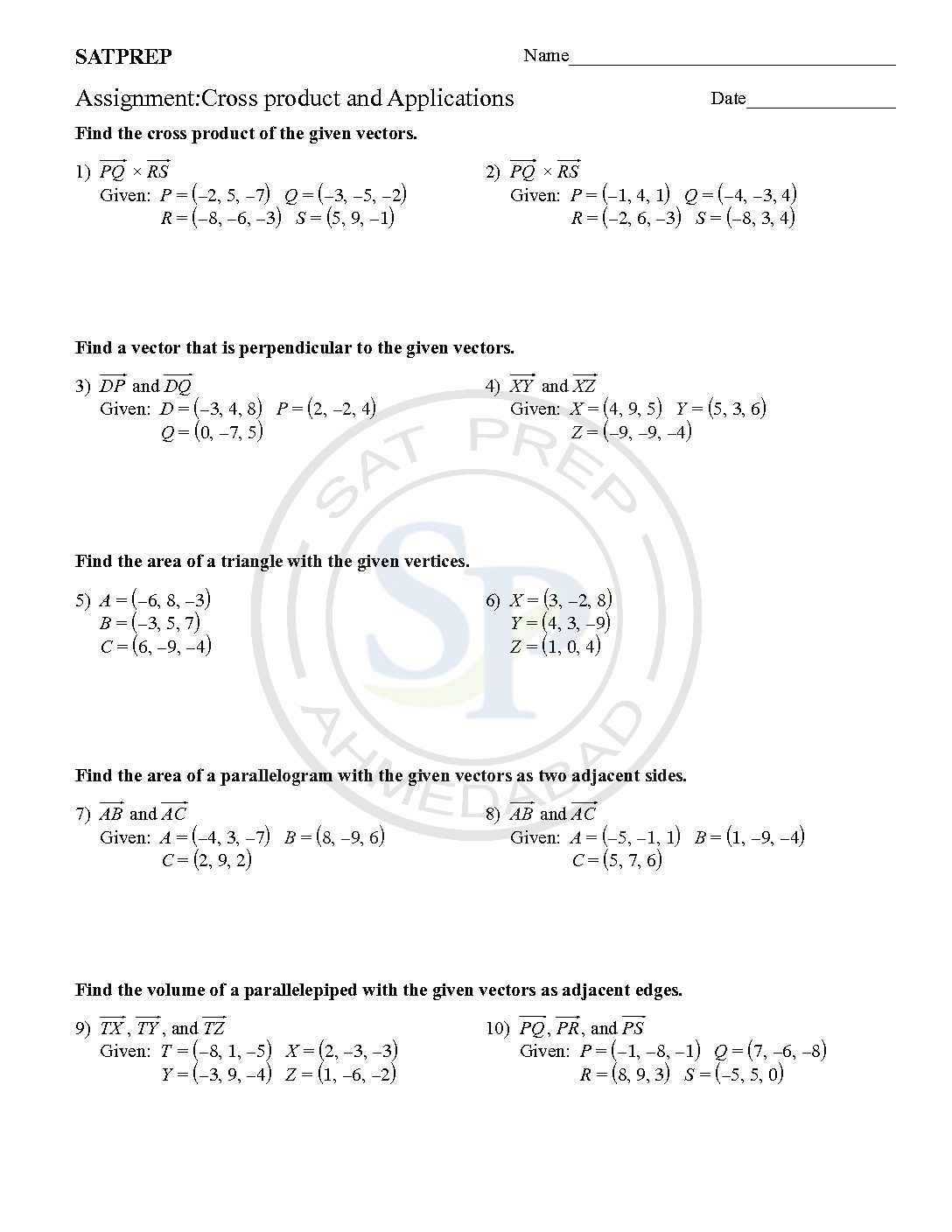
Cross product and Applications
Cross Product vs Dot Product . The significant difference between finding a dot product and cross product is the result. The dot product of any two vectors is a number (scalar), whereas the cross product of any two vectors is a vector. Cross product and application
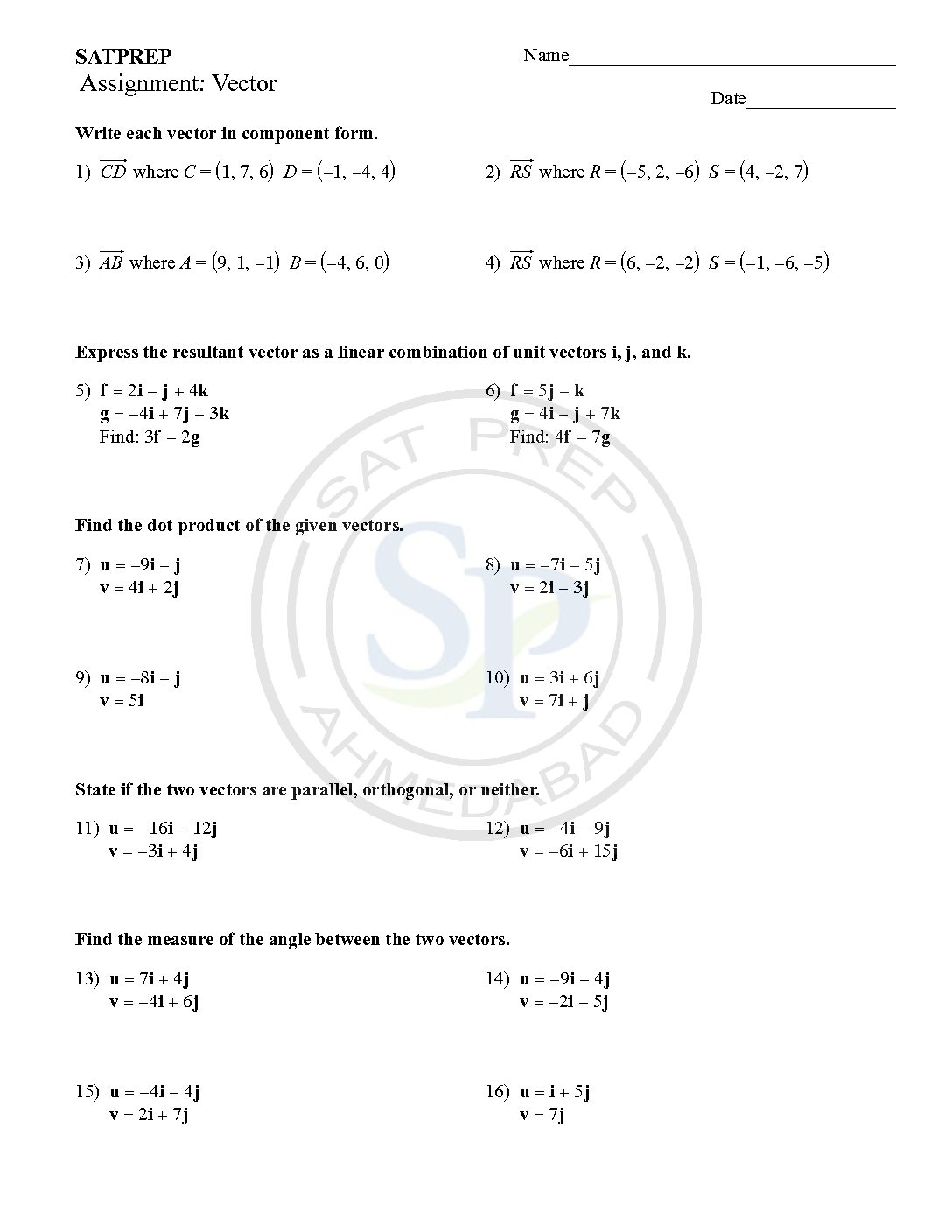
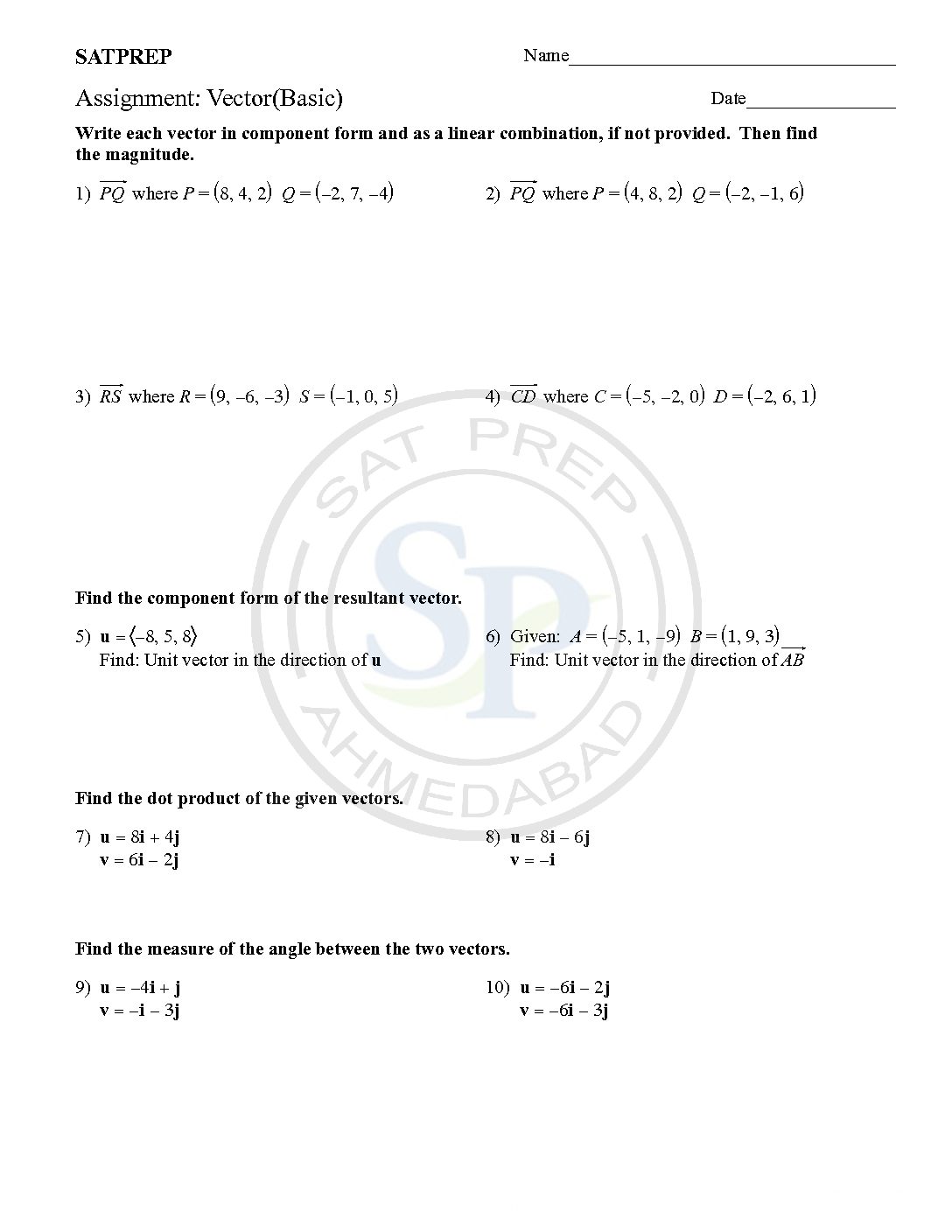
Vectors and scalar product
Scalar product of two vectors is multiply and obtain a scalar quantity. It represent on ‘dot’. Another name is dot product. vector has magnitude and direction. It start from one point to another point. The length of vector is called magnitude. Scalar product and vector
Vector and Scalar product.
vector has magnitude and direction. It start from one point to another point. The length of vector is called magnitude. The scalar product of two vectors is multiply and obtain a scalar quantity. It represent on ‘dot’. Another name is dot product. Vector and scalar product