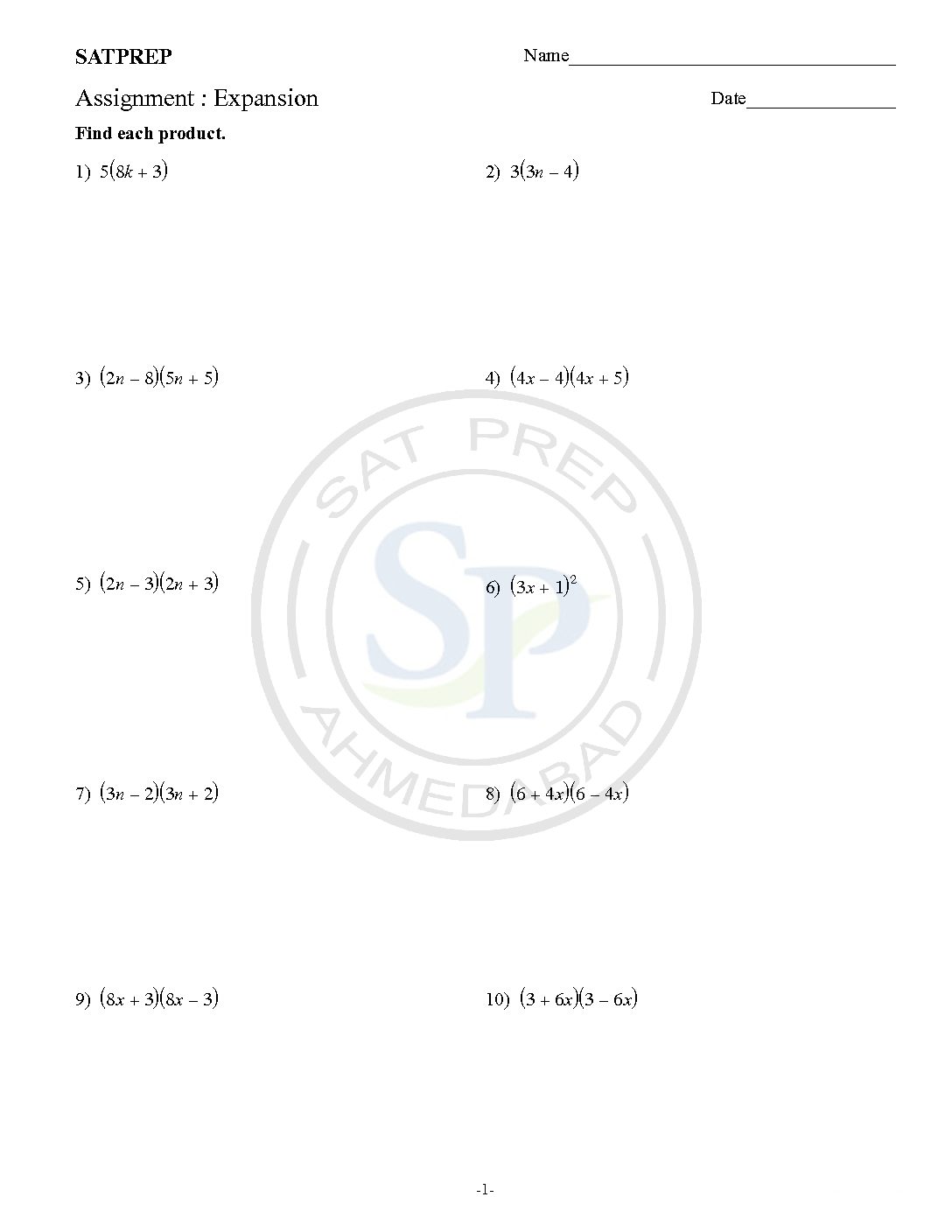
Expansions of Algebraic Expressions The formula: a(b+c) = ab + ac Algebraic Expansion. (a+b)(c+d)=ac+ad+bc+bd and (a+b)(a-b)= a ² – b² difference of square (a+b)² = a² + 2ab + b² perfect square (a-b) ²=a²-2ab+b² Expansion
You are browsing archives for
Category: Cambridge Maths A
Factorisation
Factorisation process we applied bracket to take common term out. Also we apply difference of square of variable. Hence we reduce no of terms in expression. Therefore factorisation and expansion are reverse process. Factorisation
Further Probability
to calculate Further Probability. Further Probability. An event ( E) is a subset of the sample space. That is, an event is a subset of all possible outcomes. We refer to this subset of outcomes as favourable outcomes. further probabilty
Differential Eq
Differential Equation is a function and one or more of its derivatives. Hence it solve by variable seperable and linear differential eq method. Also it solve by homogeneous. Differential Equation
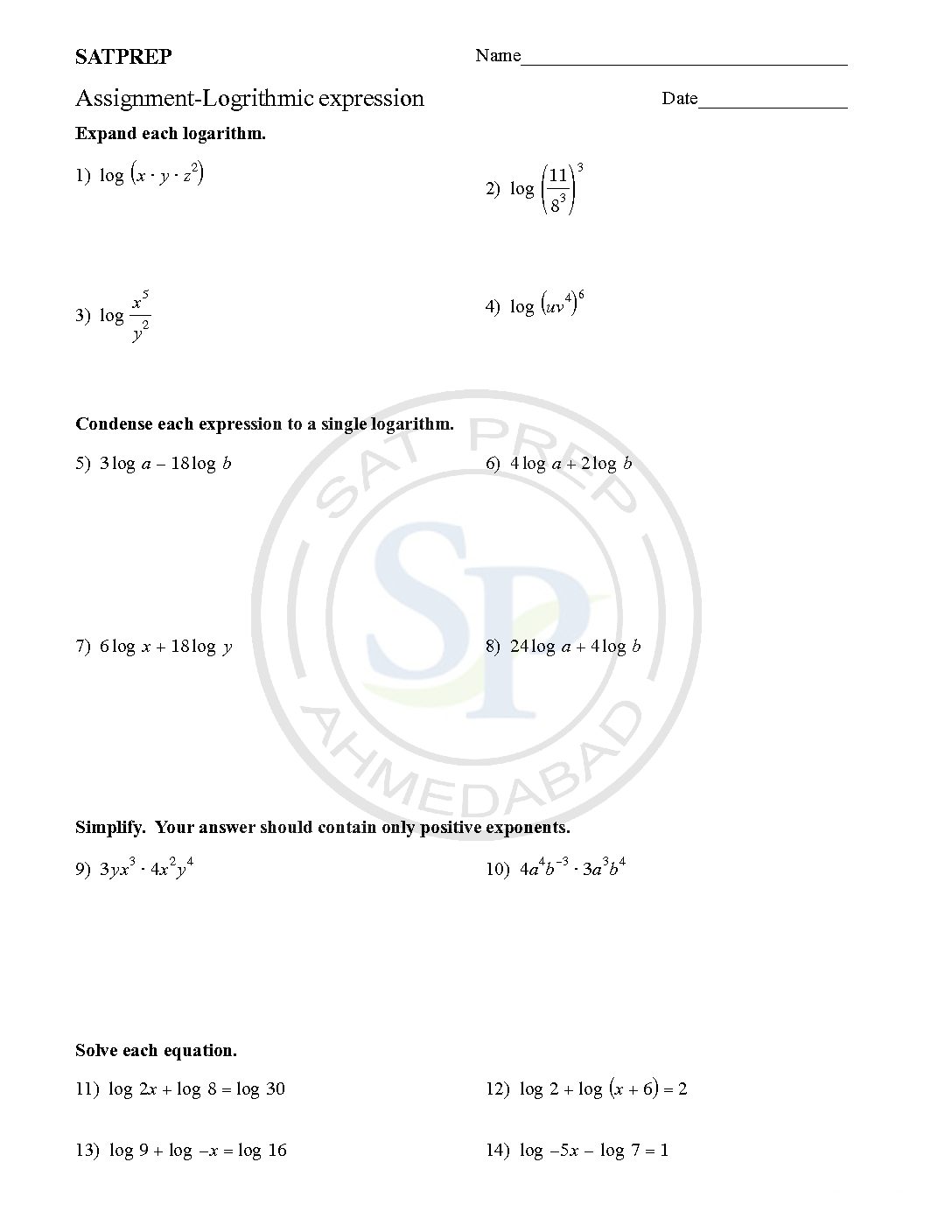
Logarithmic Expression
Solve the logarithmic equation. This problem involves the use of the symbol “ln” instead of “log” to mean logarithm. Logarithmic expression
Complex Number
Conversion from Polar to Rectangular Form Complex and vice versa. r cos Θ = x , r sin Θ = y Conversion of polar to Rectangular
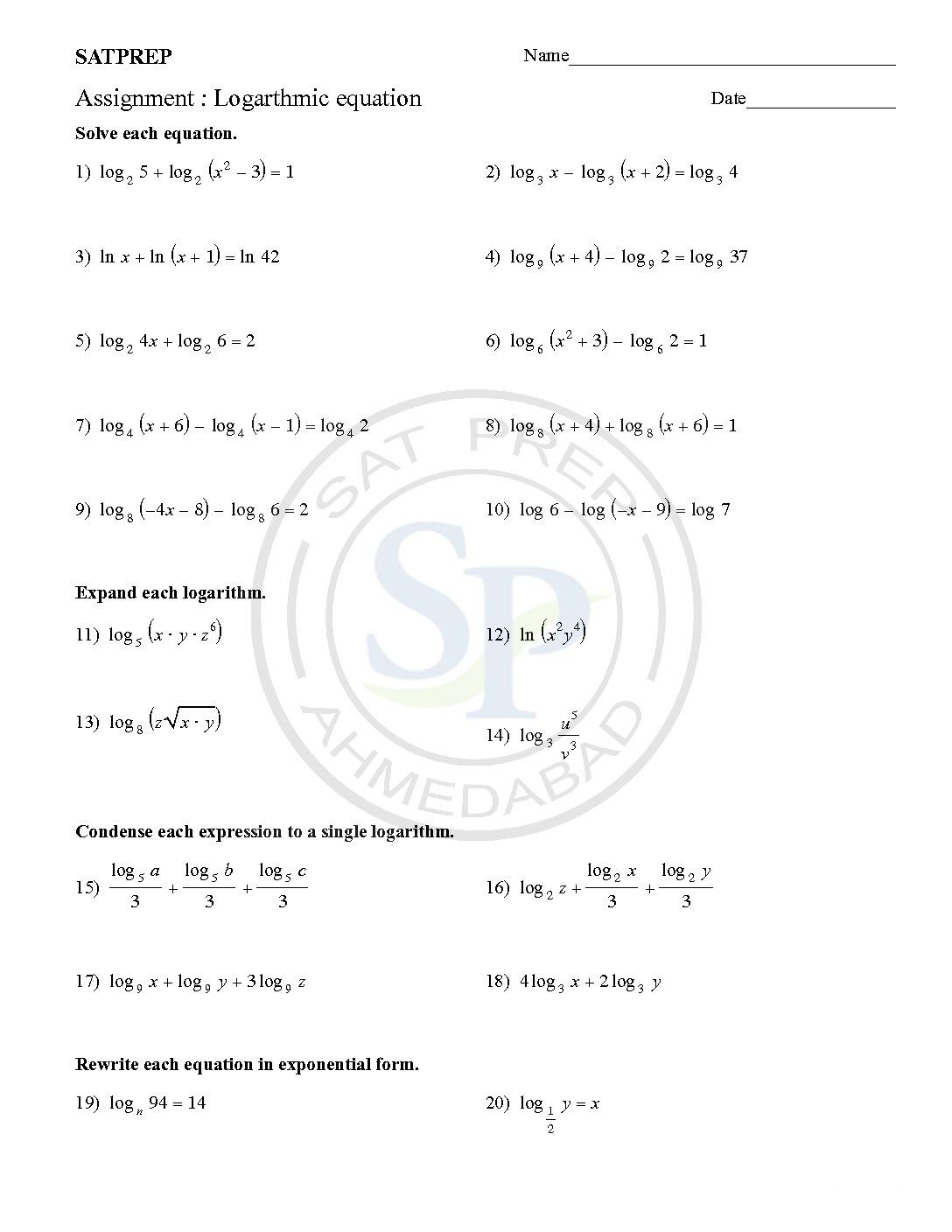
Logarthmic Equation
Logarithms equation can be solved using the properties of logarithms. So equation either are solve by log or exponent. Because both inverse of each other hence are either way exponent or log.In both apply properties of log or exponent. Also we need to factorised to solve. Logarithmic eq
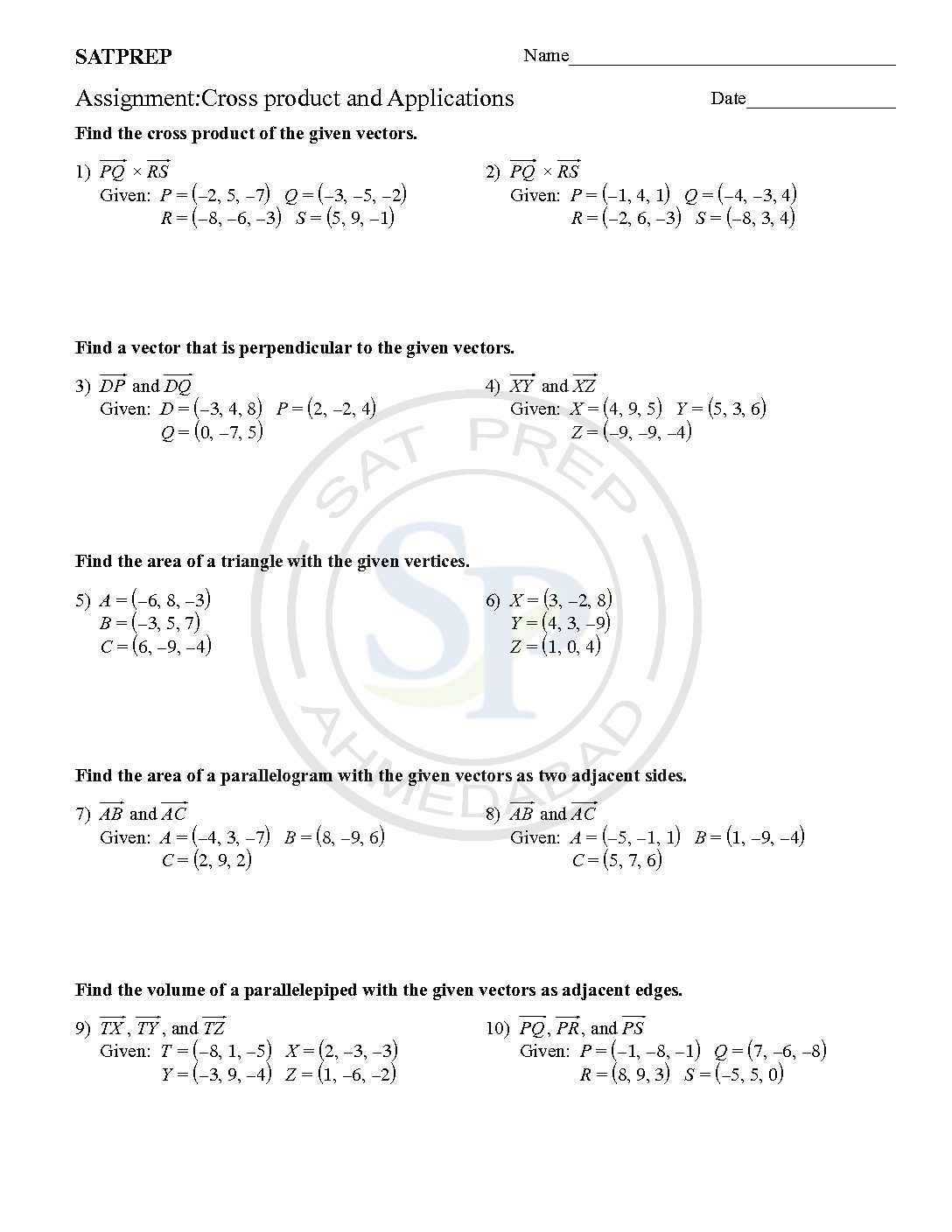
Cross product and Applications
Cross Product vs Dot Product . The significant difference between finding a dot product and cross product is the result. The dot product of any two vectors is a number (scalar), whereas the cross product of any two vectors is a vector. Cross product and application
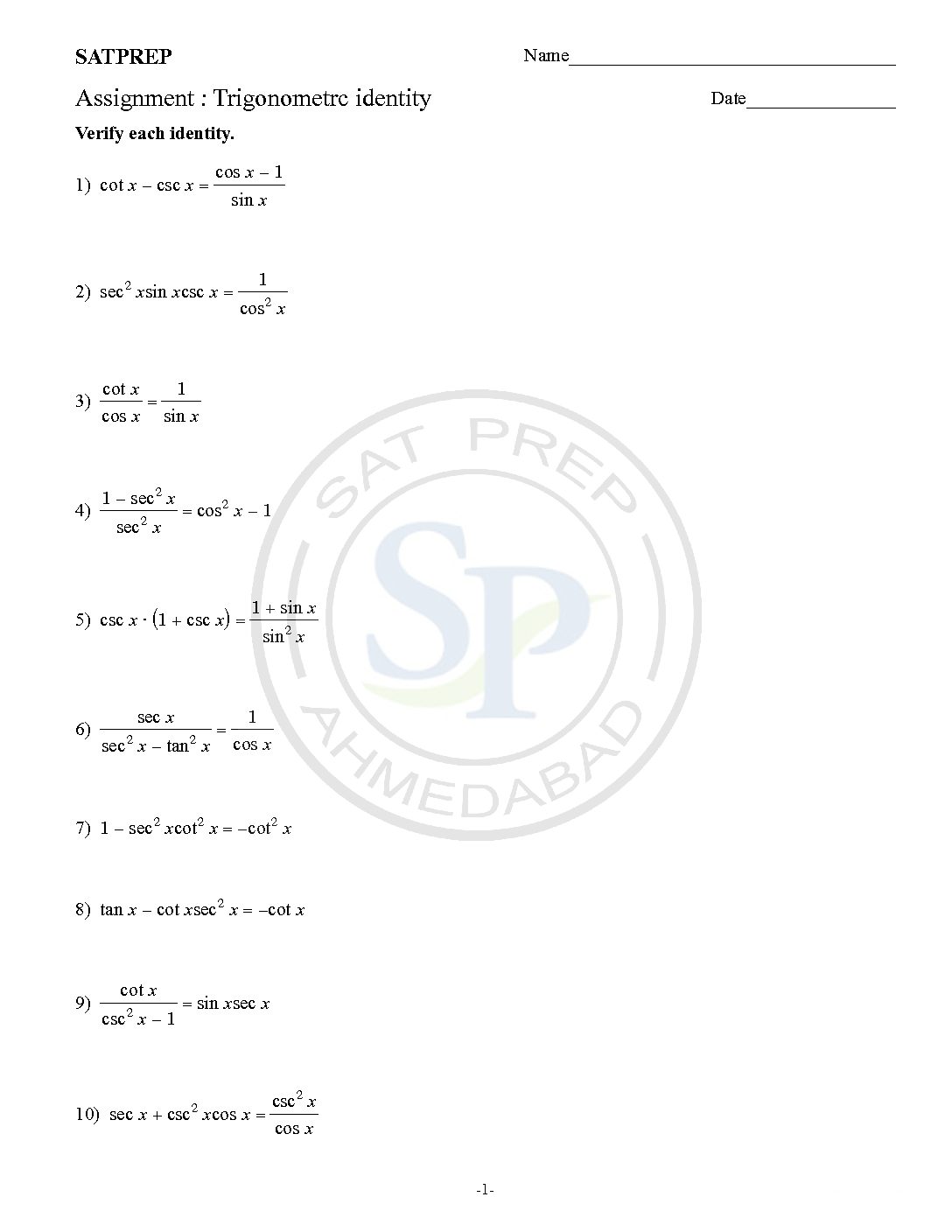
Trigonometric identity
Trig identity is equations that are true for Right Angled Triangles. D1: sin 2A+ cos 2A=1 D2: 1+ tan 2A= sec 2A D3: 1+ cot 2A= csc 2A Hence trigonometric identities are prove by above D1,D2 and D3. Similarly also using reciprocal identity. Trigonometric Identity
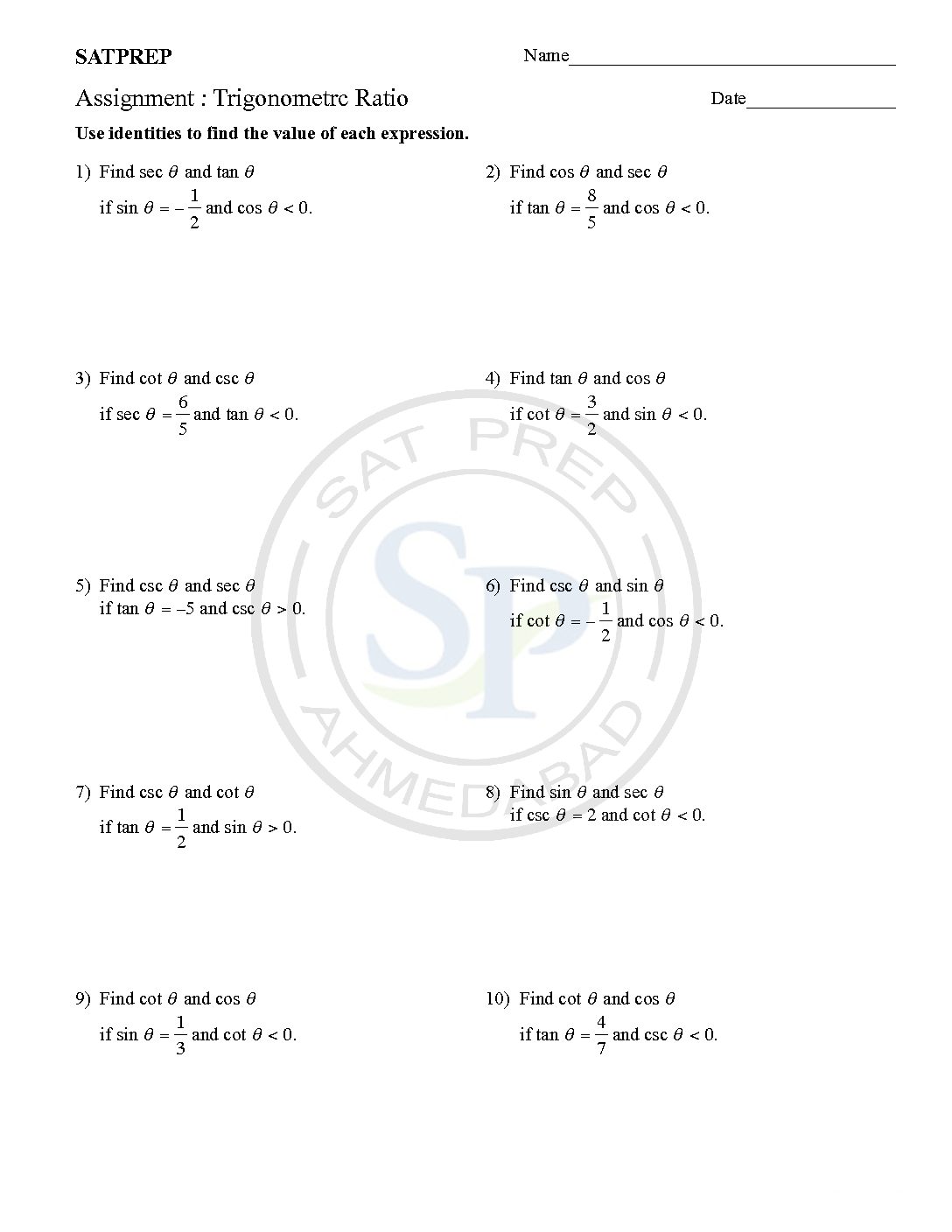
Trigonometric ratio-2
The ratios of the sides of a right triangle are called trigonometric ratios. Sine, Cosine and Tangent are main ratio while rest three reciprocal. Hence Sine and Cosine are the trigonometric ratios, whose values are less that 1 for an acute angle. Because they are periodic. www.kutasoftware.com