First of all related rate problems are applications of derivative . One of the hardest calculus problems that students have trouble . Because each application question has a different approach in solving the problem. Hence called rate of change. Related rates
You are browsing archives for
Category: Calculus
Kinematics
Describes the motion of points, objects and systems of groups of objects, without reference to the causes of motion. The symbol a stands for the acceleration of the object. And the symbol v stands for the instantaneous velocity of the object. The derivative of displacement with time is velocity ( v = ds/dt ). The […]
Optimisation
Process of calculating optimal value of function at turning point (maximum or minimum )value of the curve. Hence we use second derivative. It also give greatest or least value of curve. Optimisation
Related Rates
Rate of change is also related rate problems are applications of derivative . One of the hardest calculus problems that students have trouble . Because each application question has a different approach in solving the problem. Ralated rates
Calculus
This formula sheet help the students for preparing precalculus and calculus. Further this sheet also help in academic math. This can also be use in Pre-calculus. Calculus
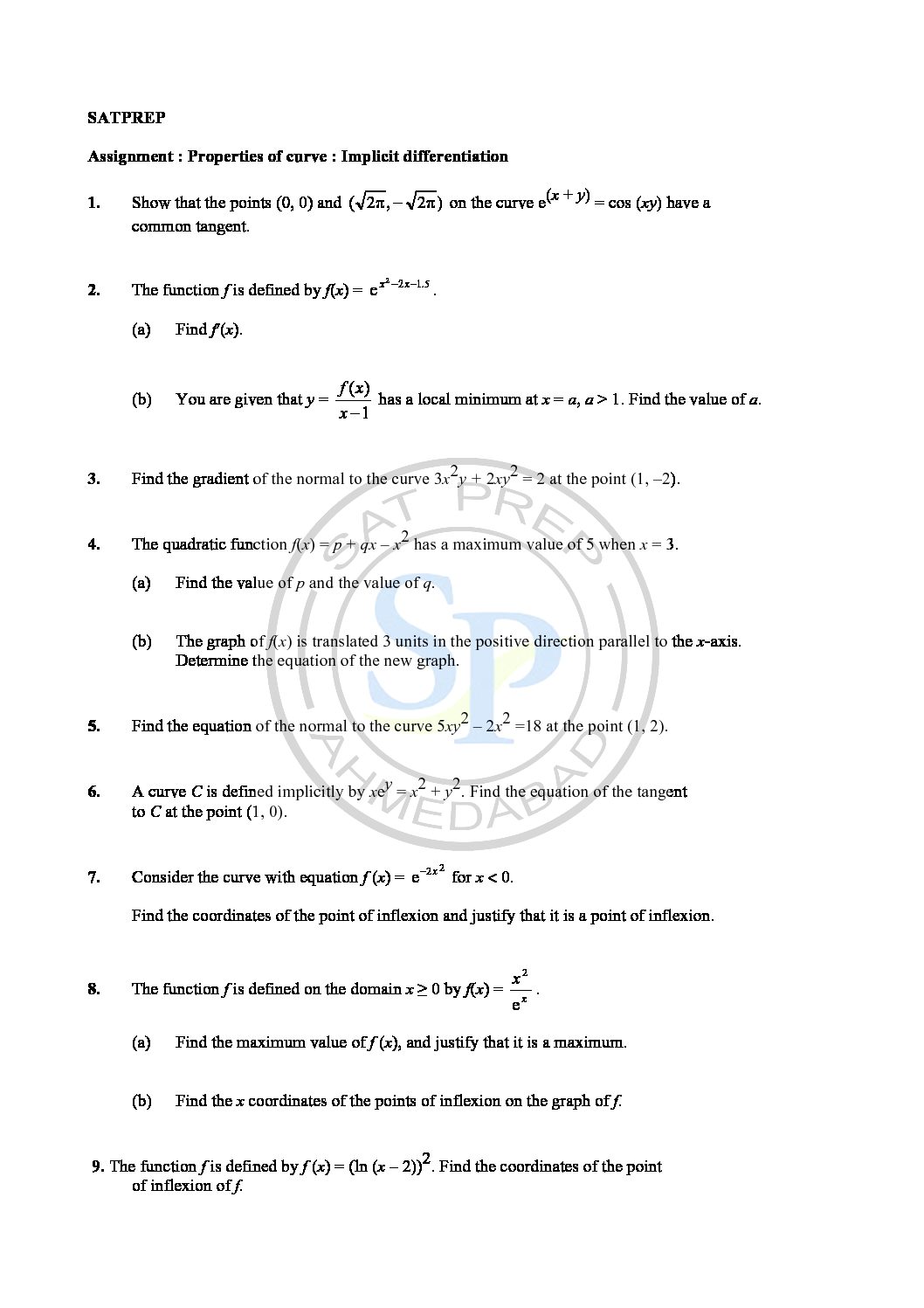
Properties of curve :Implicit
Makes use of the chain rule to differentiate implicitly defined functions. To differentiate an implicit function y ( x ), defined by an equation R ( x, y) = 0, it is not generally possible to solve it explicitly for y and then differentiate. The derivatives of inverse trig functions we’ll need the formula from the last section relating the derivatives of inverse functions. properties […]
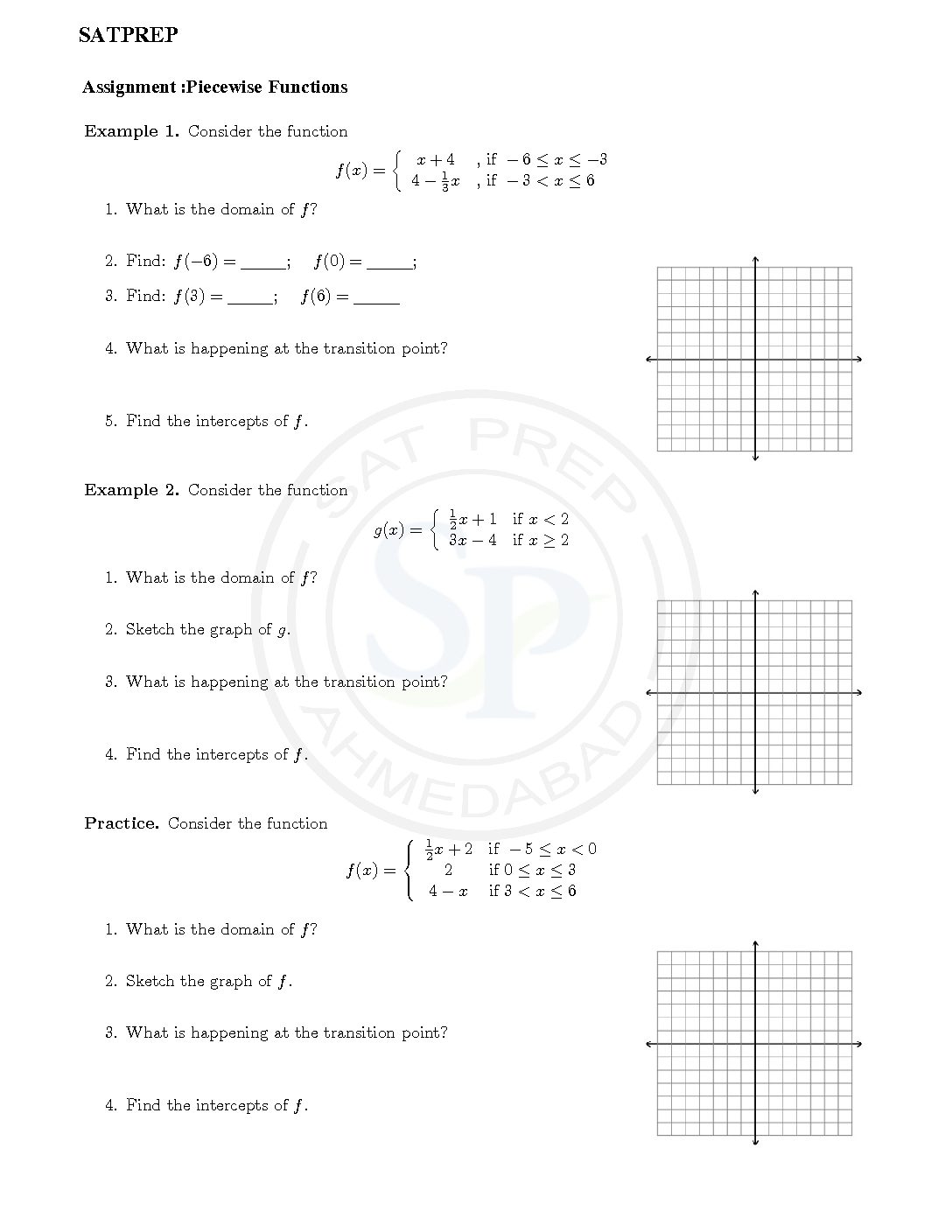
Piece wise function
Piecewise Functions. A Function Can be in Pieces. We can create functions that behave differently based on the input (x) value. Piece wise function
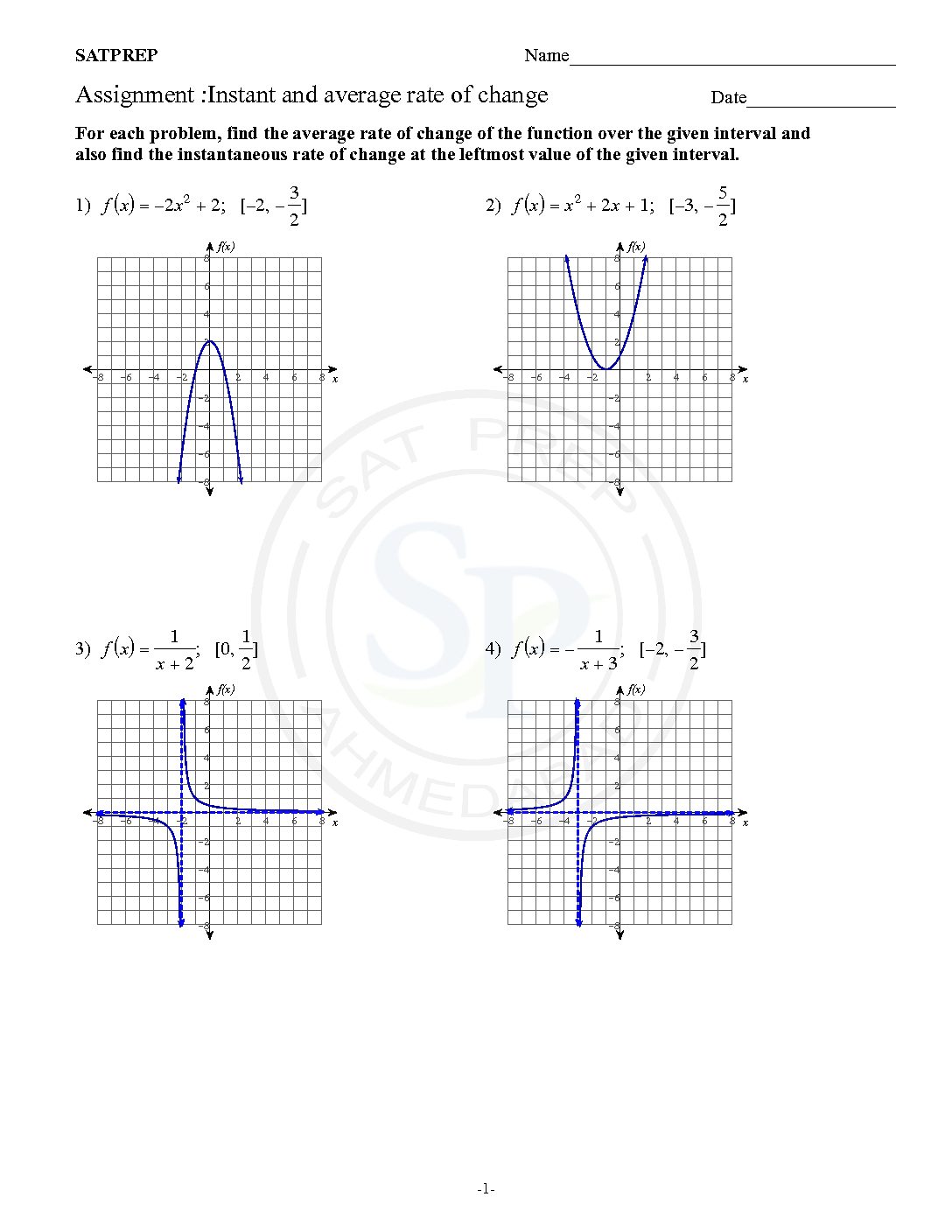
Average and Instantaneous rate of chan...
This rate of change is not the same as the average rate of change. Average rate of change instantaneous rate of change change in quantity change in time limits. We want to talk about instantaneous rate of change, instantaneous rate of change is a lot like instantaneous velocity only it’s a little more general Instant and Average rate of change
Continuity
Continuity Theorems : a function is continuous if function is LHL = f(x) = RHL the function f (x) is continuous at that point. continuous
Derivative test
First Derivative Test for Local Extrema. If the derivative changes from positive (increasing function) to negative (decreasing function), the function has a local (relative) maximum at the critical point. Second Derivative Test. 1. If , then has a local minimum at . 2. If , then has a local maximum at . The extremum test gives slightly more general conditions under which […]