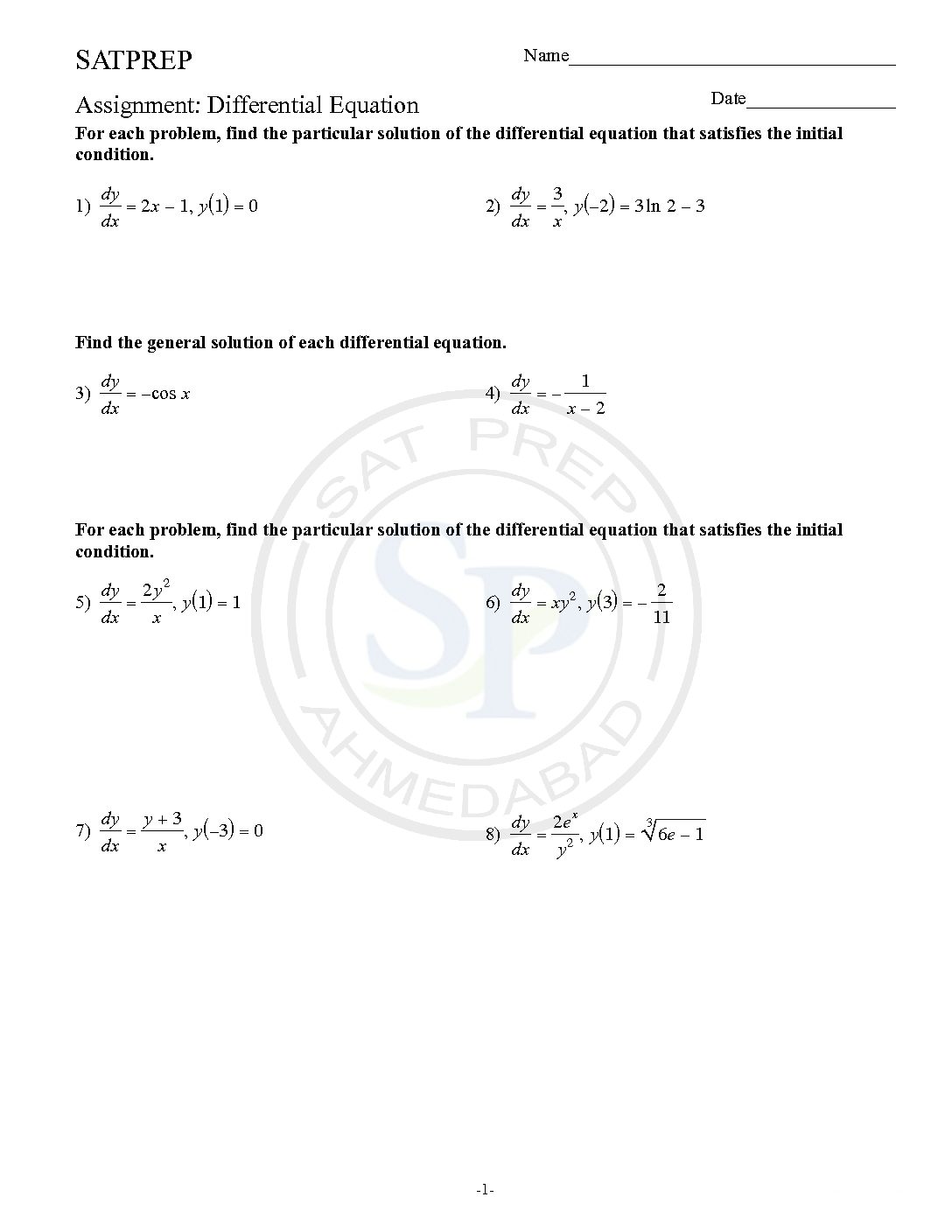
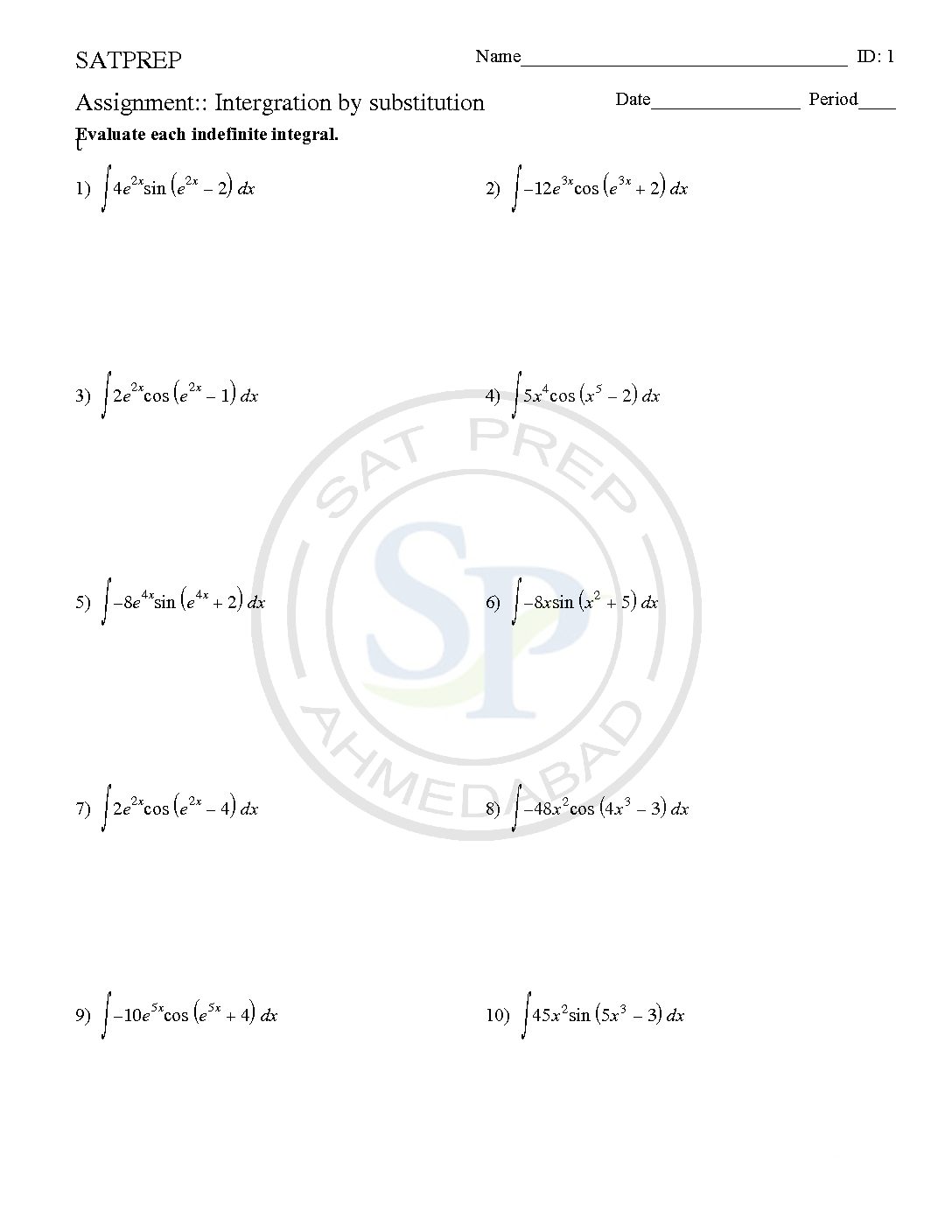
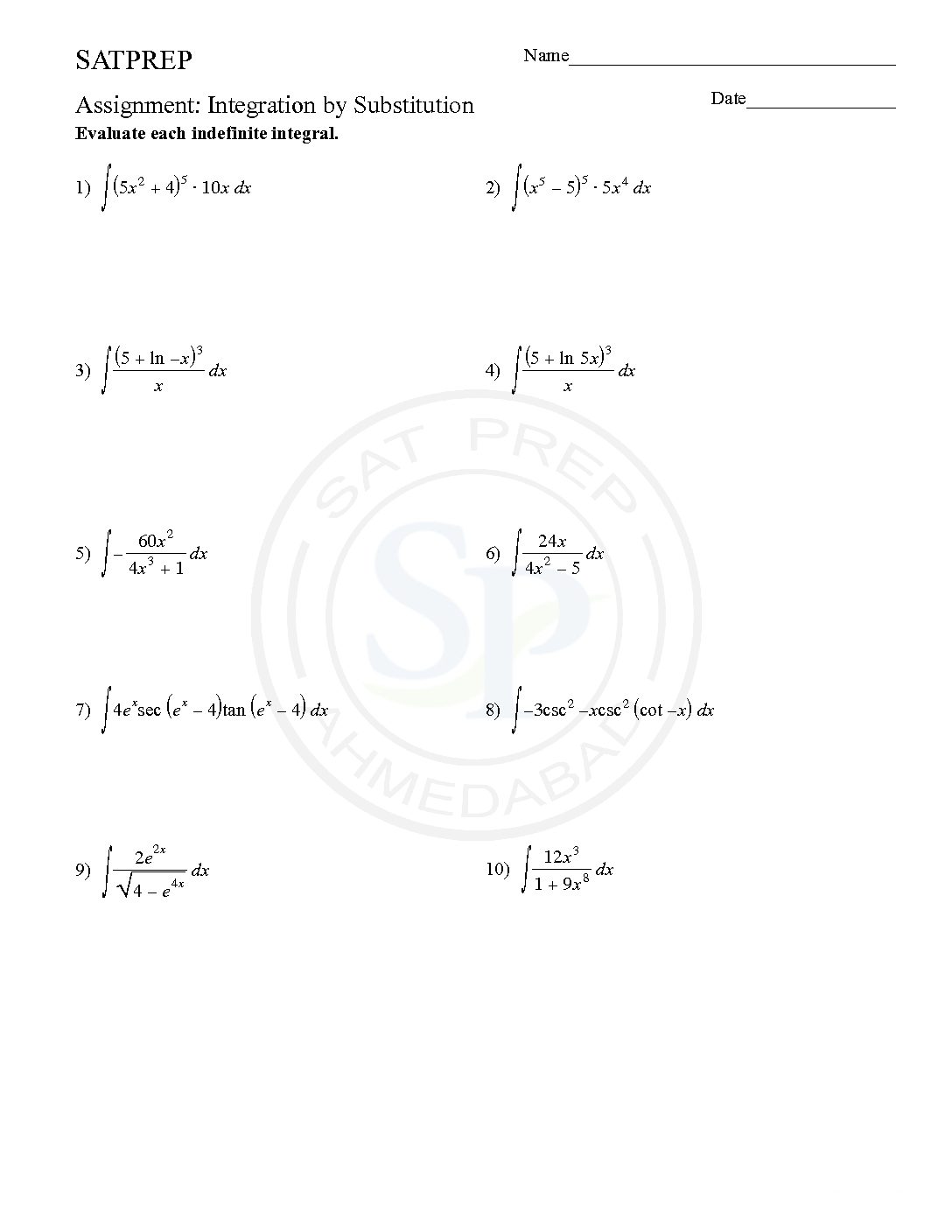
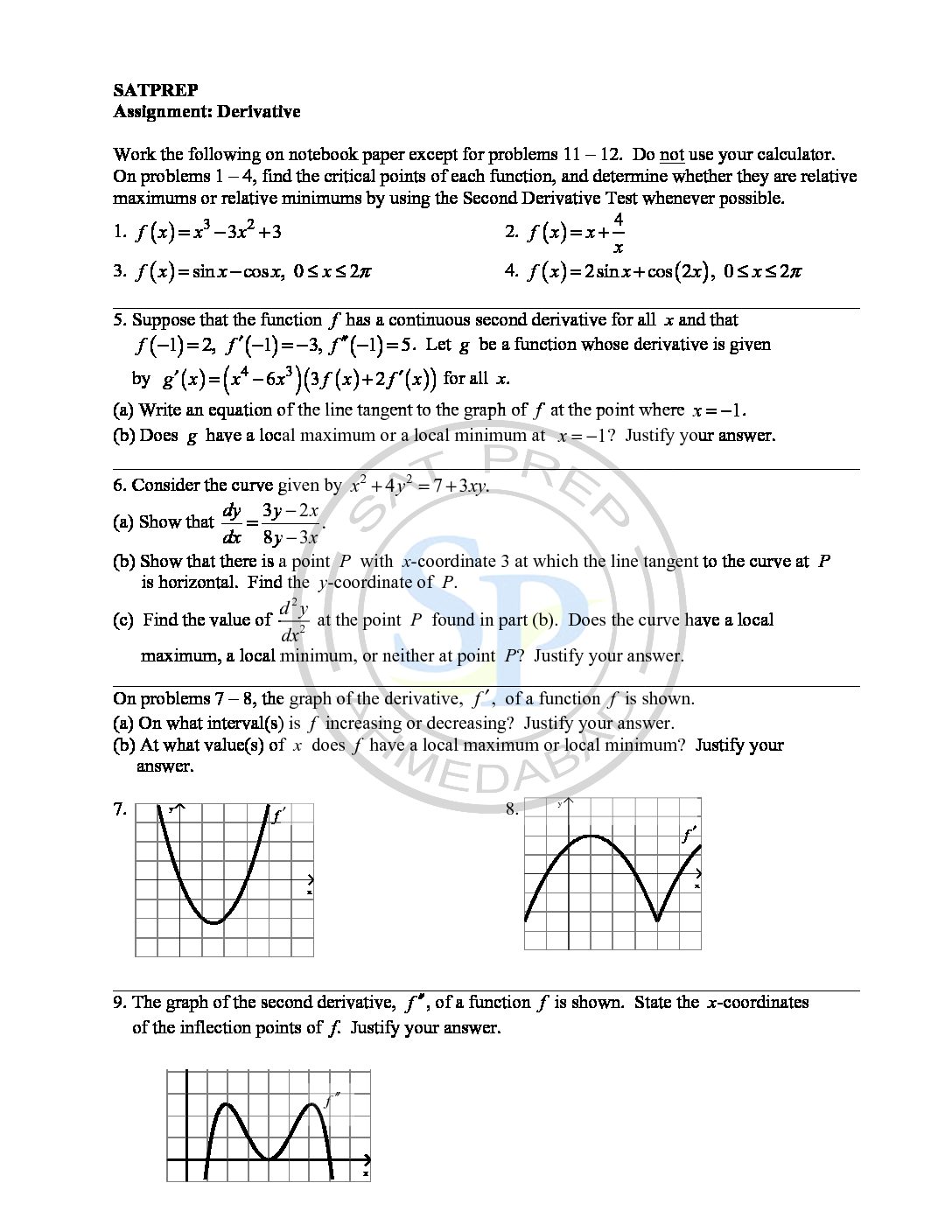
A Differential Equation is a function and one or more of its derivatives. Hence it solve by variable seperable and linear differential eq method. Also it solve by homogeneous. Differential Eq
You are browsing archives for
Category: IB Maths HL
Integration by substitution -1
This post is about worksheet of Integration by exponential substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by exponential substitution
Integration by substitution -2
This post is about worksheet of integration by logarithmic substitution. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by logarithmic substitutions
Integration (Basic)
Integration is summation of function. Integration is a way of adding functions to find the sum of functions, also we find equation of curve. As well as Applications are area under the curve and volume. It can be used to find areas, volumes, central points and many useful things. it help you practice by showing you […]
Probability (Basic)
It is the measure of the likelihood that an event will occur. A number between zero and one that shows how likely a certain event. Another probability of event A is the number of ways event A can occur divided by the total number of possible outcomes. Also expressed by the ratio of the number of actual […]
Basics of Permutation and Combination
Combinations and permutation and is a very important topic of mathematics as well as the quantitative aptitude section. In permutation we arrange object while in combination we select items. Permutation and combination
Derivative
The derivative of a function of a single variable at a chosen input value. Derivative is the slope of the tangent line to the graph of the function at that point. Hence derivative of a function y = f(x) of a variable x is a measure of the rate at which the value y of the function changes with respect to the change […]
Curve sketching
Curve Sketching. If f (-x) = -f (x) for all x in the domain, then f is odd and symmetric about the origin. d) Asymptotes: Find the asymptotes of the function using the methods described above. First attempt to find the vertical and horizontal asymptotes of the function. Curve sketching
Curve Sketching -2
the sketching of curve though coordinate of turning and axes intercepts . So equation of the curve is given. Curve sketching
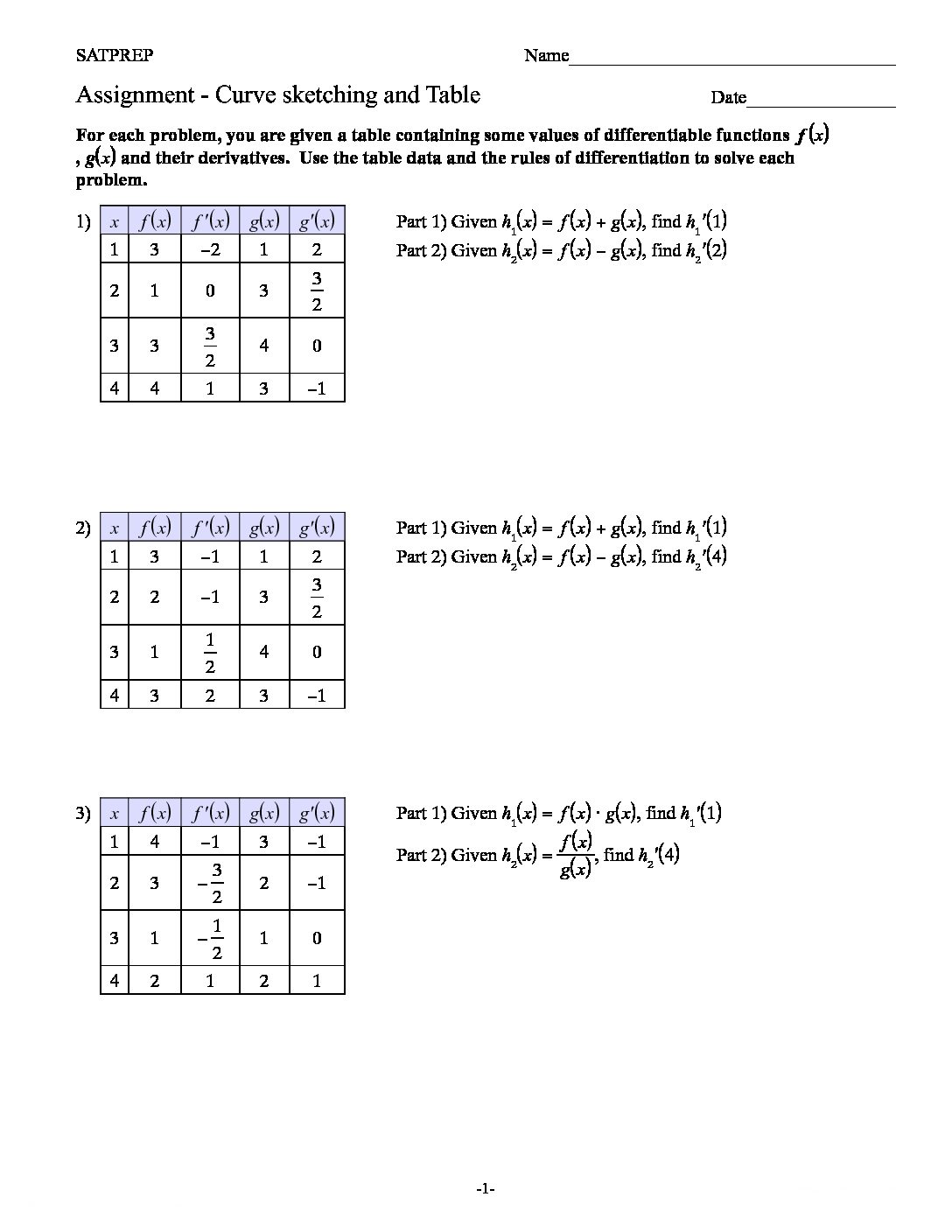
Curve sketching
Coordinate of turning point and axes intercepts for the sketching of curves though . So equation of the curve is given. Curve sketching