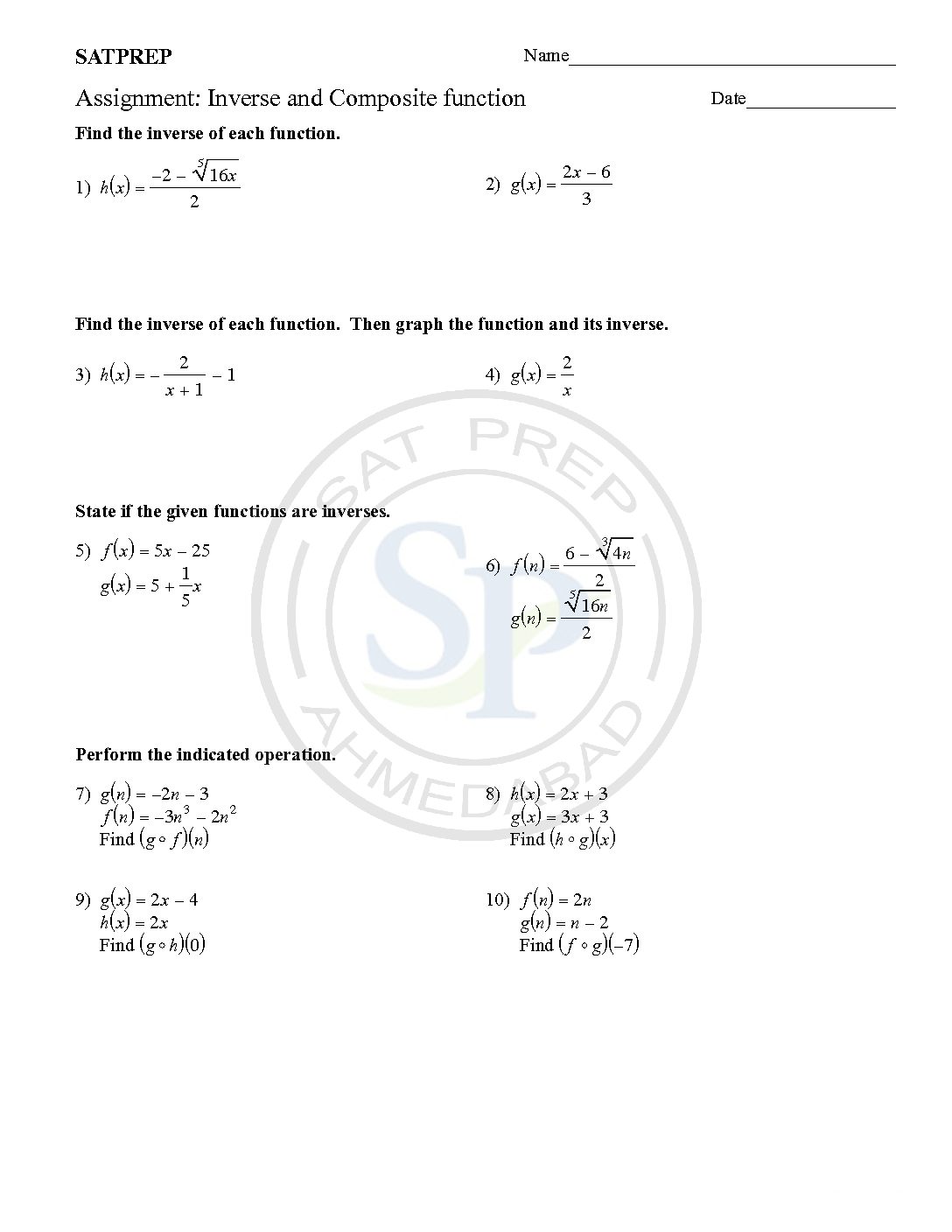
Inverses and Composites are two important concept of functions. Composite function is defined as one function defined inside another function. Like ff(x)) or f(g(x)) etc. Concept of inverse is interchange of variables . Inverse notations are f-1(x),g-1(x) etc. Hence interchange is inverse. Therefore function and its inverse is reflection about y = x. Inverse and composite
You are browsing archives for
Category: IB Maths HL
Inverse and composite function
Inverses and Composite are two important concept of functions. Composite function is defined as one function defined inside another function. Like ff(x)) or f(g(x)) etc. Concept of inverse is interchange of variables . Inverse notations are f-1(x),g-1(x) etc. Hence interchange is inverse. Therefore function and its inverse is reflection about y = x. inverse and […]
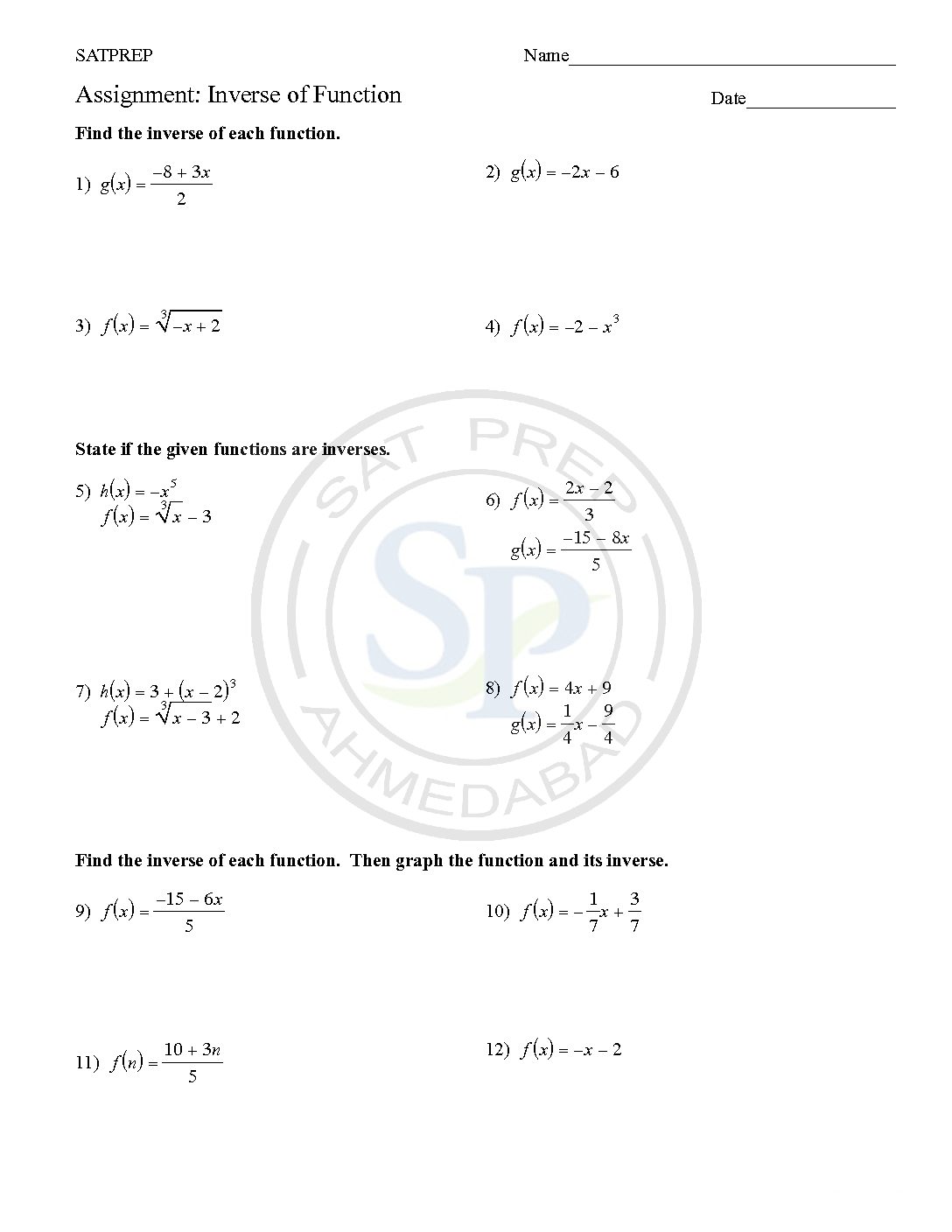
Inverse of function
Inverse of functions is an important concept . Domain values and Range values gets interchange . Due to these values of functions, continuity of function also change. Graph of inverse function also change and become y-axis oriented. Therefore graph of inverse is reflection of function graph about y = x. Inverse of Function
Rational Function
Rational functions is a function that is the quotient of two polynomials. Also polynomial a function that is the quotient of two polynomials. Rational functions and the properties of their graphs such as domain, vertical and horizontal asymptotes, x and y intercepts . Rational function graph is discontinuous graph at asymptotes. Rational function
Rational and Reciprocal functions
A rational functions is a function of the form f(x)=p(x)/q(x), where p(x) and q(x) are polynomials and q(x)≠0 ,q(x)≠0 . The domain of a rational function consists of all the real numbers x=0 except those for which the denominator is x= 0 . reciprocal for a number x, denoted by 1/x or x −1, is a number which when multiplied by x yields the multiplicative identity, 1.. The […]
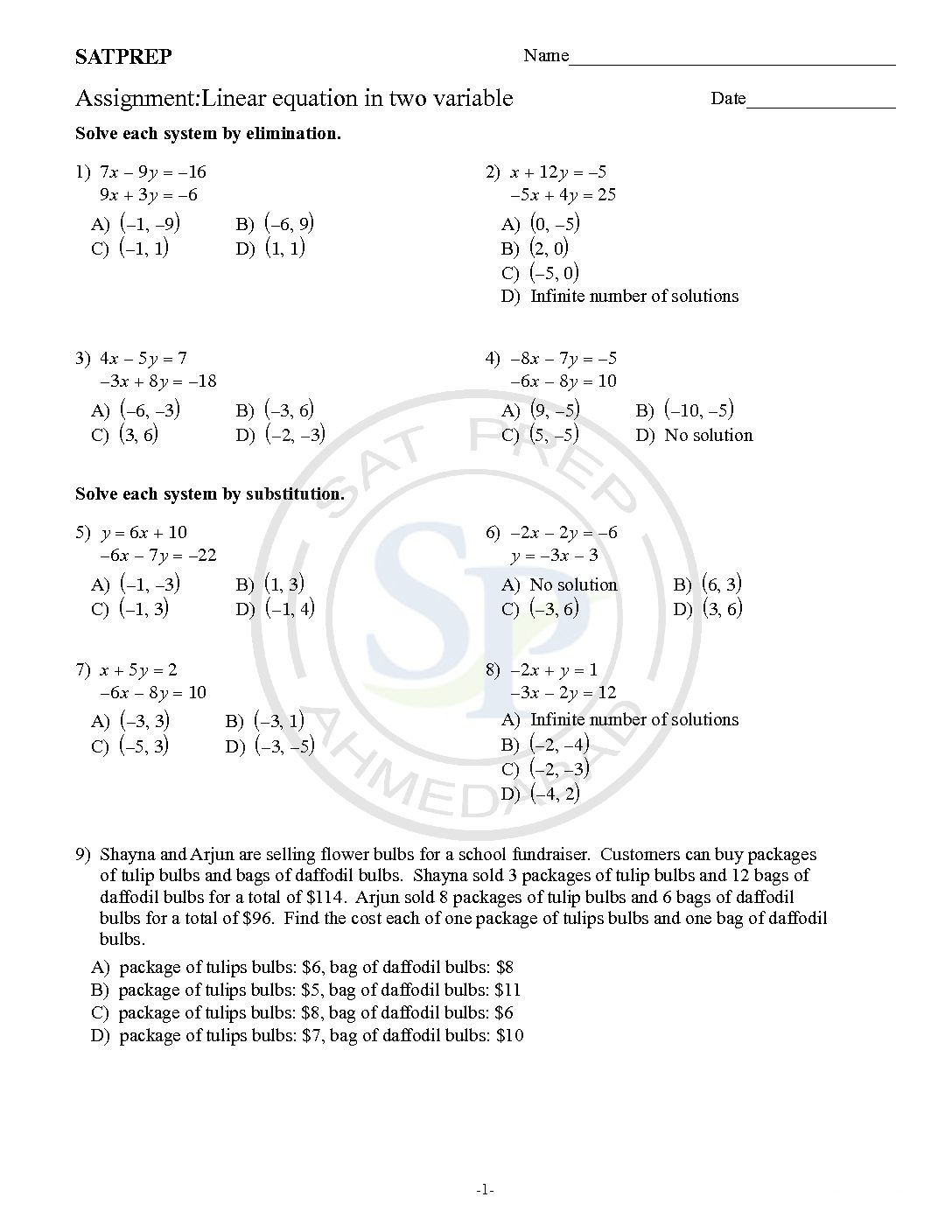
Linear equation in two variable
Two variable linear equation. The general form of Linear Equations are ax + by = c, where a is not equal to 0. Solution of a Linear Equation gives one value of x. Because line intersect axes at one point. Linear Eq.
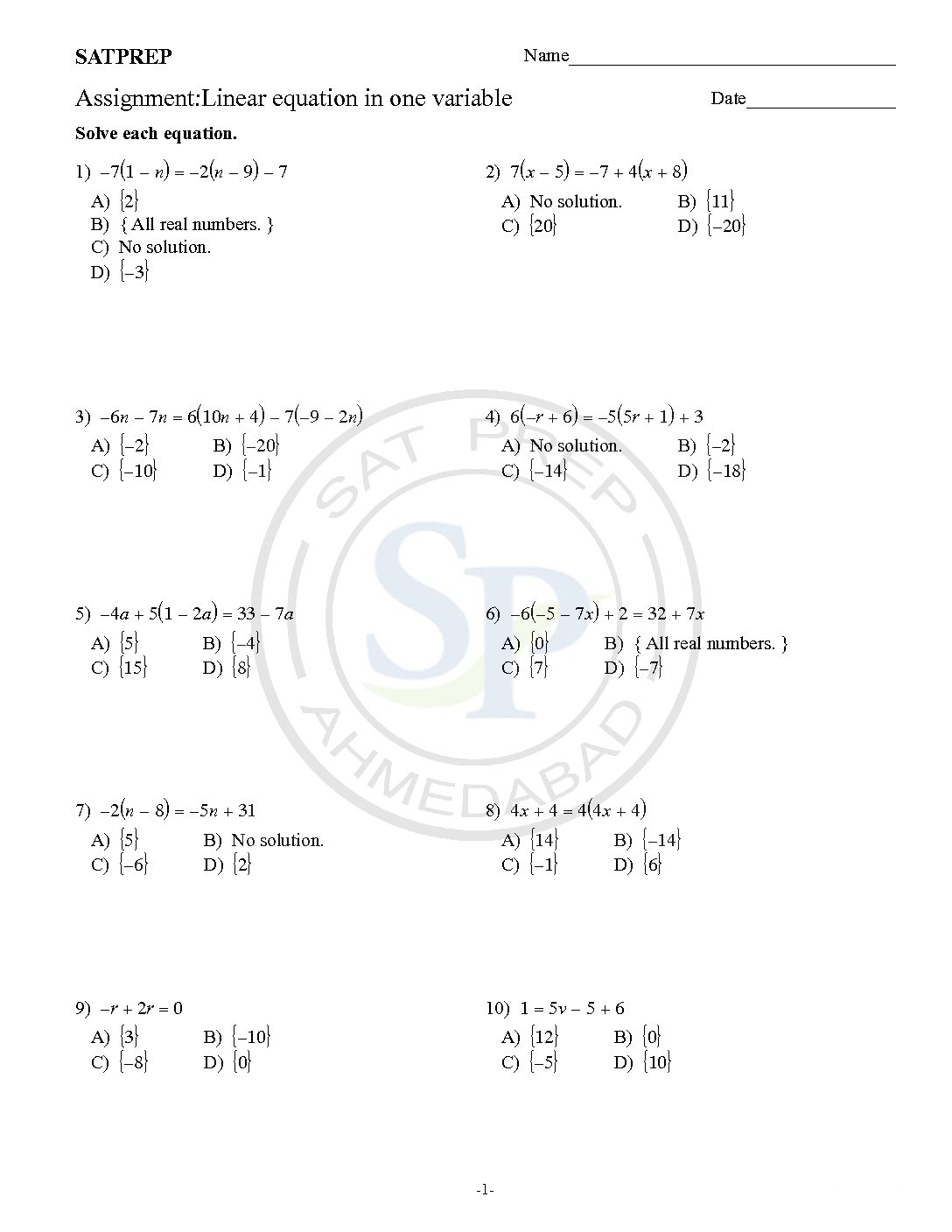
Linear equation in one variable
One variable linear equation. The general form of Linear Equations are ax +b= c, where a is not equal to 0. Solution of a Linear Equation gives one value of x. Because line intersect x-axis at one point. Two variable
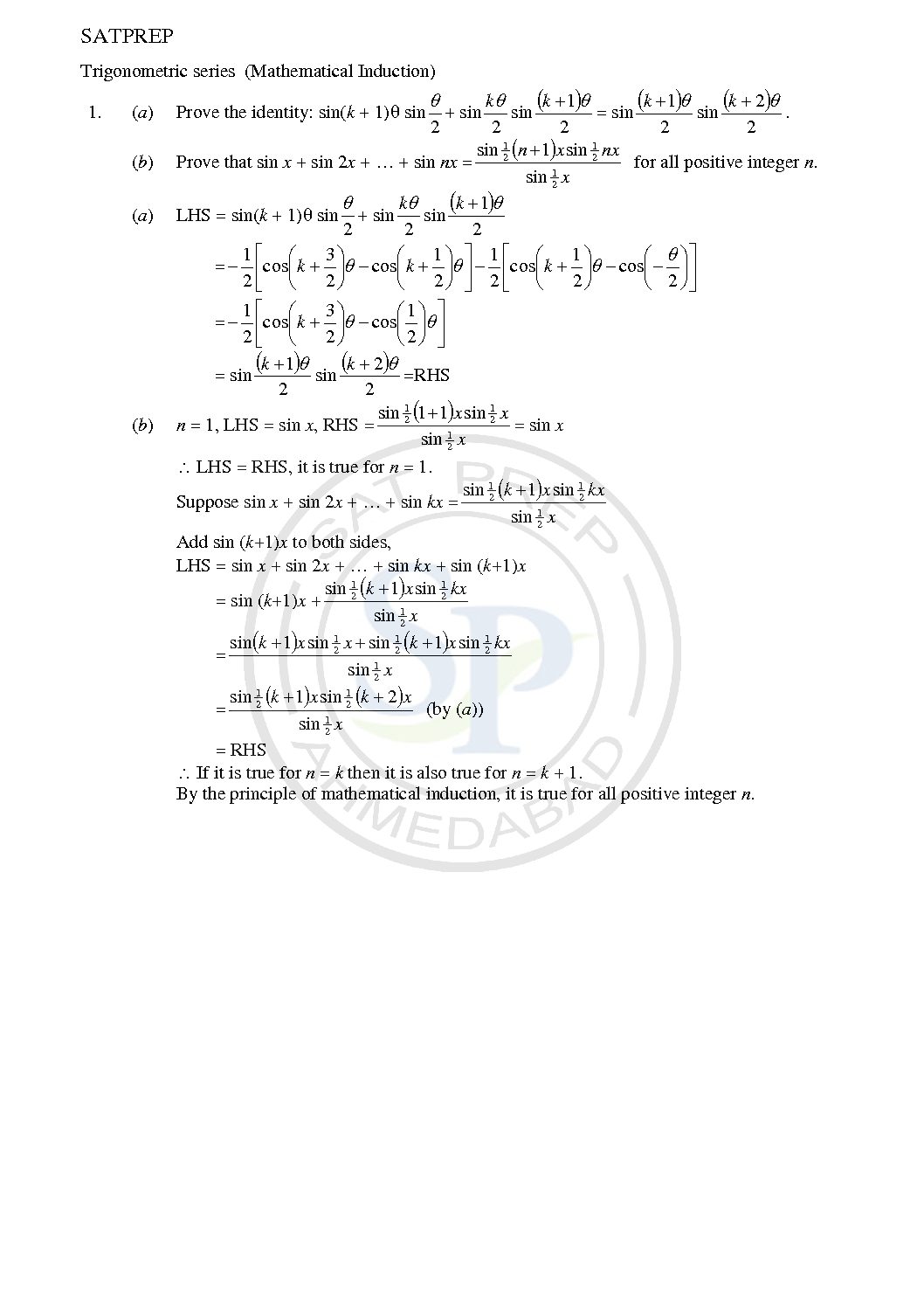
Mathematical Induction with Trigonometri...
Principle of Mathematical Induction, is a way to prove a statement is true by first making an assumption or hypothesis. There are only three steps for a Proof by Mathematical Induction before we can draw our conclusion. Once P(k+1) has been proved to be true, the statement is true for all values of the variable, by Principle of Mathematical […]
Circular Measure
The radian is a unit of measurement angle . Therefore, this unit is used in many topics likewise sector , angles . Parts of the mathematics area which include circular measure and more often, the branch of angle measurements where pi (Π) is used. Therefore, radian use to measure arc length, chord length, area of sectors of a circle and many others. […]
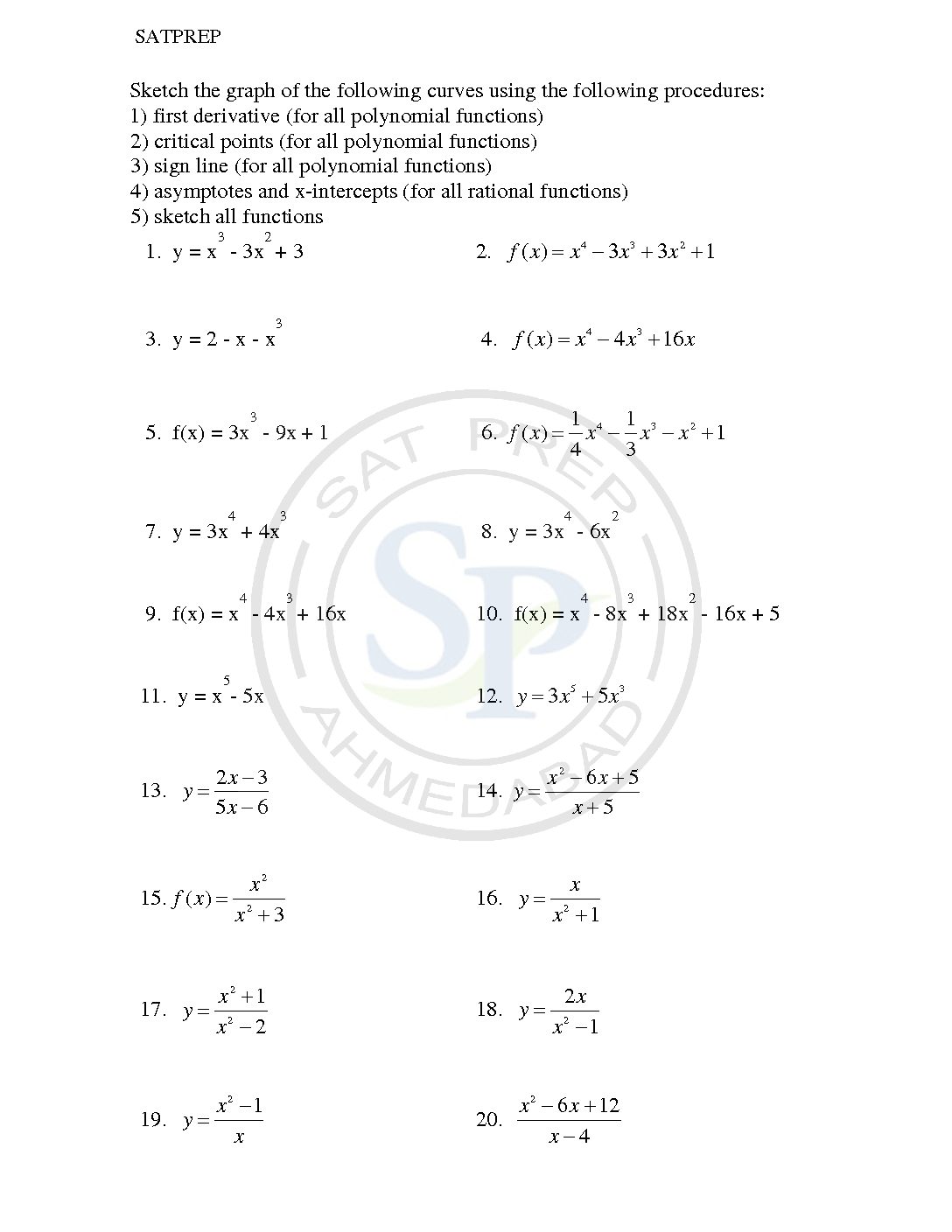
Sketching curve
This post about the sketching of curve though coordinate of turning and axes intercepts . So equation of the curve is given. Curve sketching