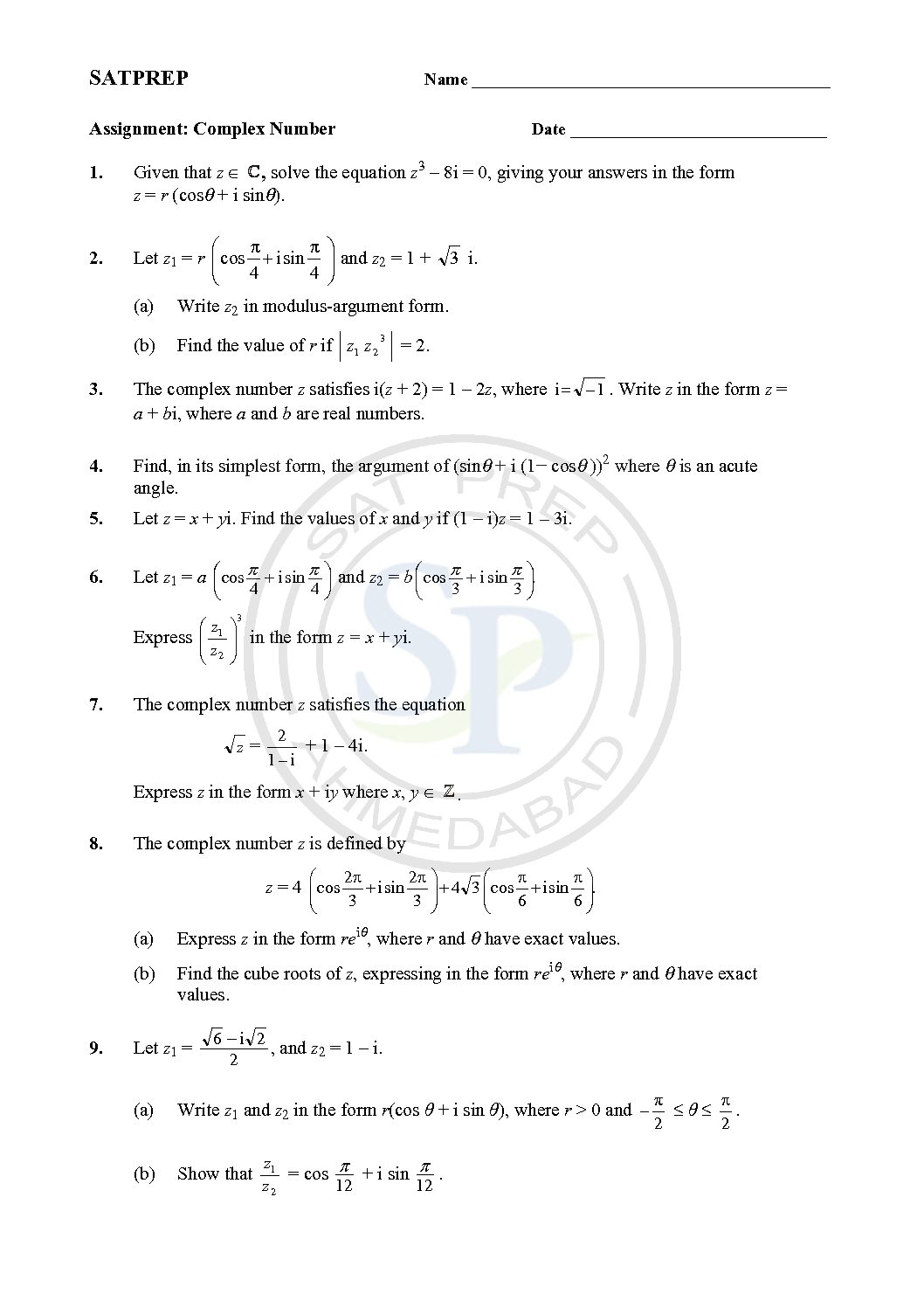
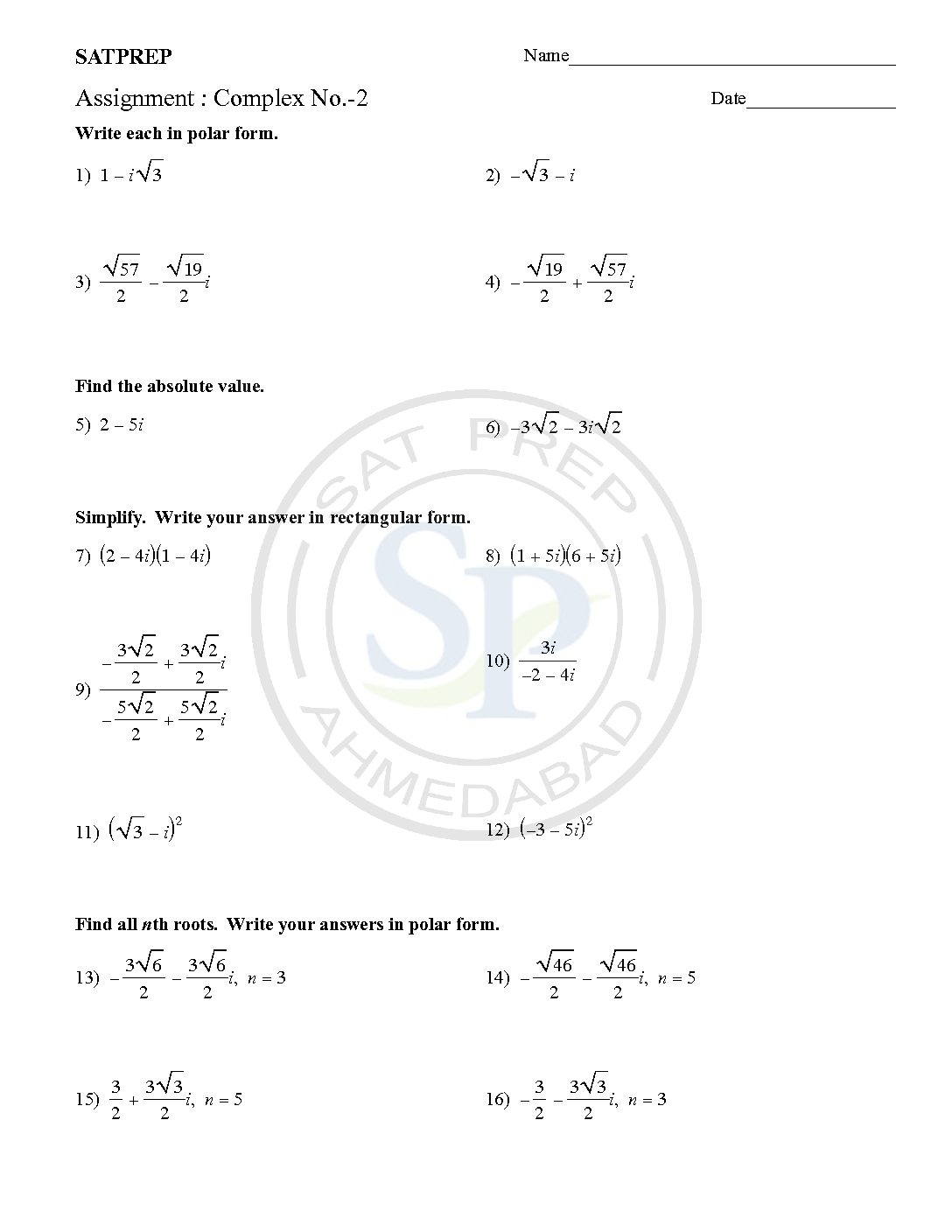
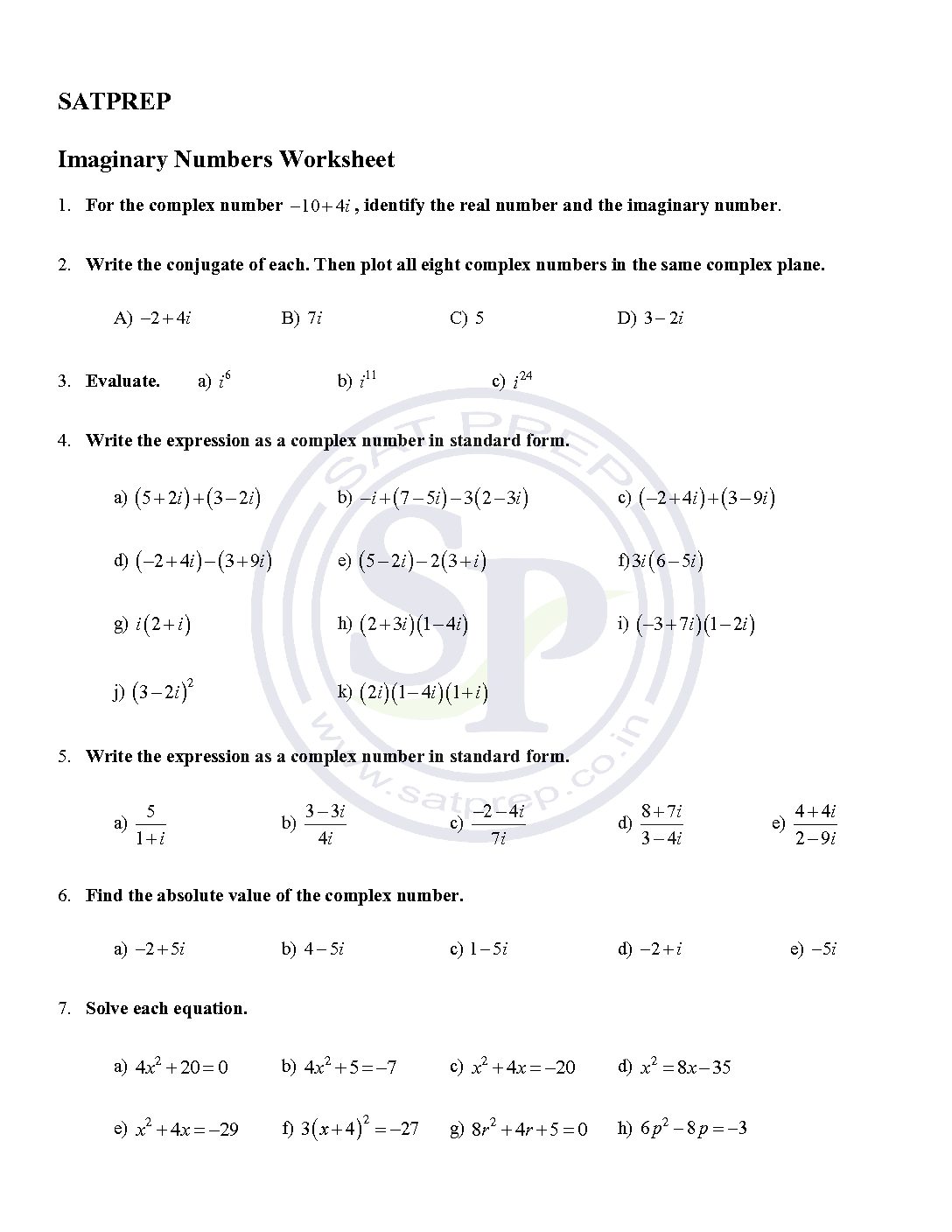
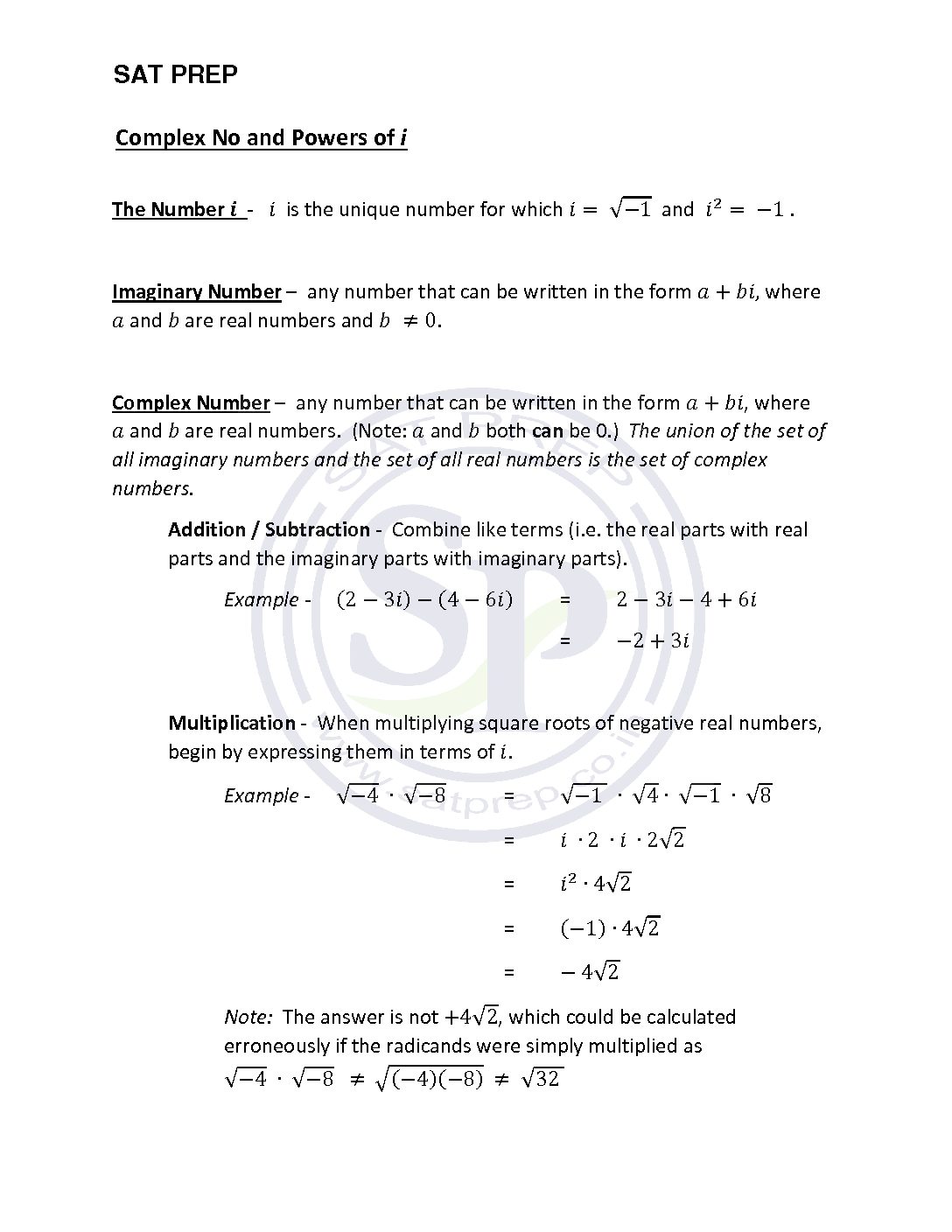
A complex number is can be expressed in the form a + bi, where a and b are real numbers . The complex number a + bi, a is called the real part, and b is called the imaginary part. So due to presence of i this part is called imaginary. Hence complex no is another number system. Therefore it obey all rules […]
You are browsing archives for
Category: Complex Number
Conversion Complex No Rectangular to Pol...
Converting from Polar Form to Rectangular Form. Either method of notation is valid for complex numbers. Rectangular form lending itself to addition and subtraction, and polar form lending itself to multiplication and division. Hence polar form of a complex number is another way to represent a complex number. The form z = a + b i is called the rectangular coordinate form of a complex number. This representation is very useful when we multiply or divide complex numbers. Therefore argand diagram use […]
Complex Number
Conversion from Polar to Rectangular Form Complex and vice versa. r cos Θ = x , r sin Θ = y Conversion of polar to Rectangular
Polynomial with complex root
nth degree polynomial with real coefficients has precisely n complex roots . Hence when all root cannot be real. Complex root with Polynomial
Arithmetics of complex no
imaginary number is any number of the form a + bi where a and b are real numbers. Addition and Subtraction of complex numbers is to add or subtract real parts and the imaginary parts. complex no
Imaginary No
A number is an imaginary number when it multiplied by square root of negative no. An imaginary number is the square root of a negative number and does not have a tangible value.”Complex” numbers have two parts, a “real” part (being any “real” number that you’re used to dealing with) and an “imaginary” part (being any number with an […]
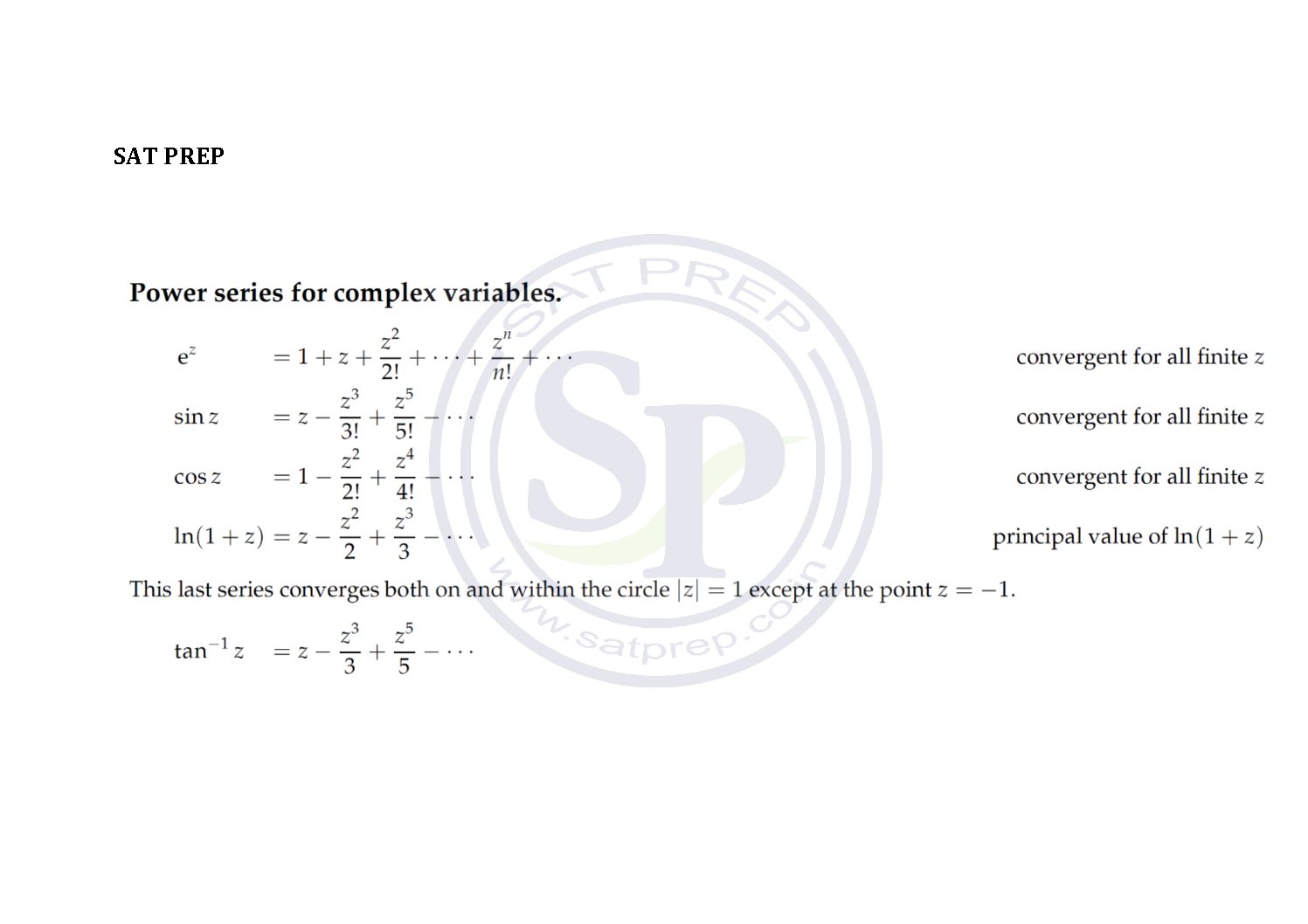
Power series with Complex Coefficient
Power series with Complex Coefficient. n represents the coefficient of the nth term and c is a constant. An is independent of x and may be expressed as a function of n . The set of all formal power series in X with coefficients in a commutative ring R form another ring that is written R [[ X ]] Also expressions for Coefficients of a Power Series. As […]