First of all this post consists of questions of factorisation of quadratics . The method use to find solutions are splitting middle term and another method is formula . We also find the nature of roots of equation. The method is use to find nature is discriminant (D= b2-4ac). Discriminant is also used in formula […]
You are browsing archives for
Category: Functions
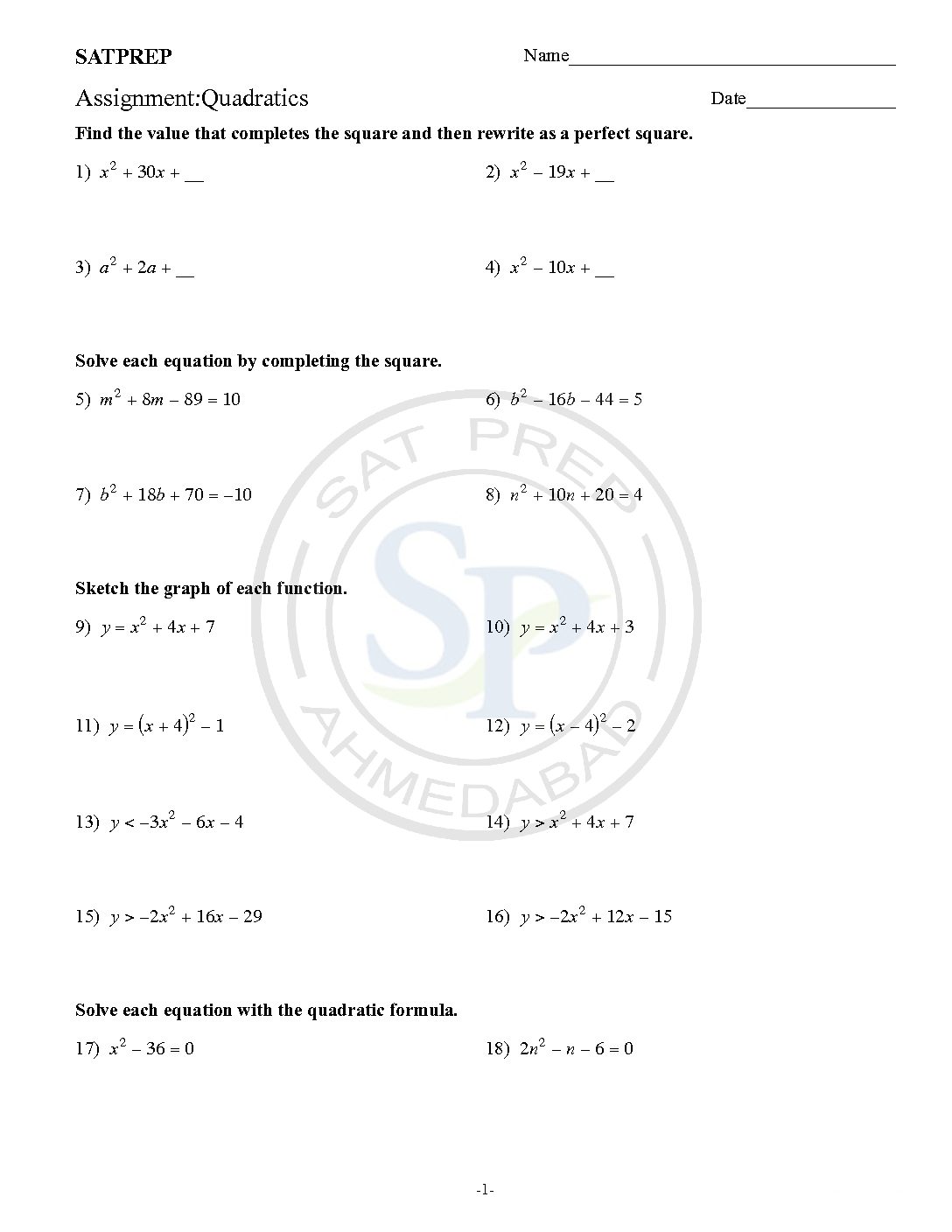
Completing Square form
Completing form is to solve a quadratic equation by changing the form of the equation so that the left side is a perfect square trinomial. Completing the square is a technique for converting a quadratic polynomial of the form ax2 + bx + c to a(x-b)2+c. Hence this method is used to convert. Similarly it use to […]
Function
A functions f(x) tells you what to do with the input, but, to be completely defined, function also need to know what type of input is permitted to go into the function. Function
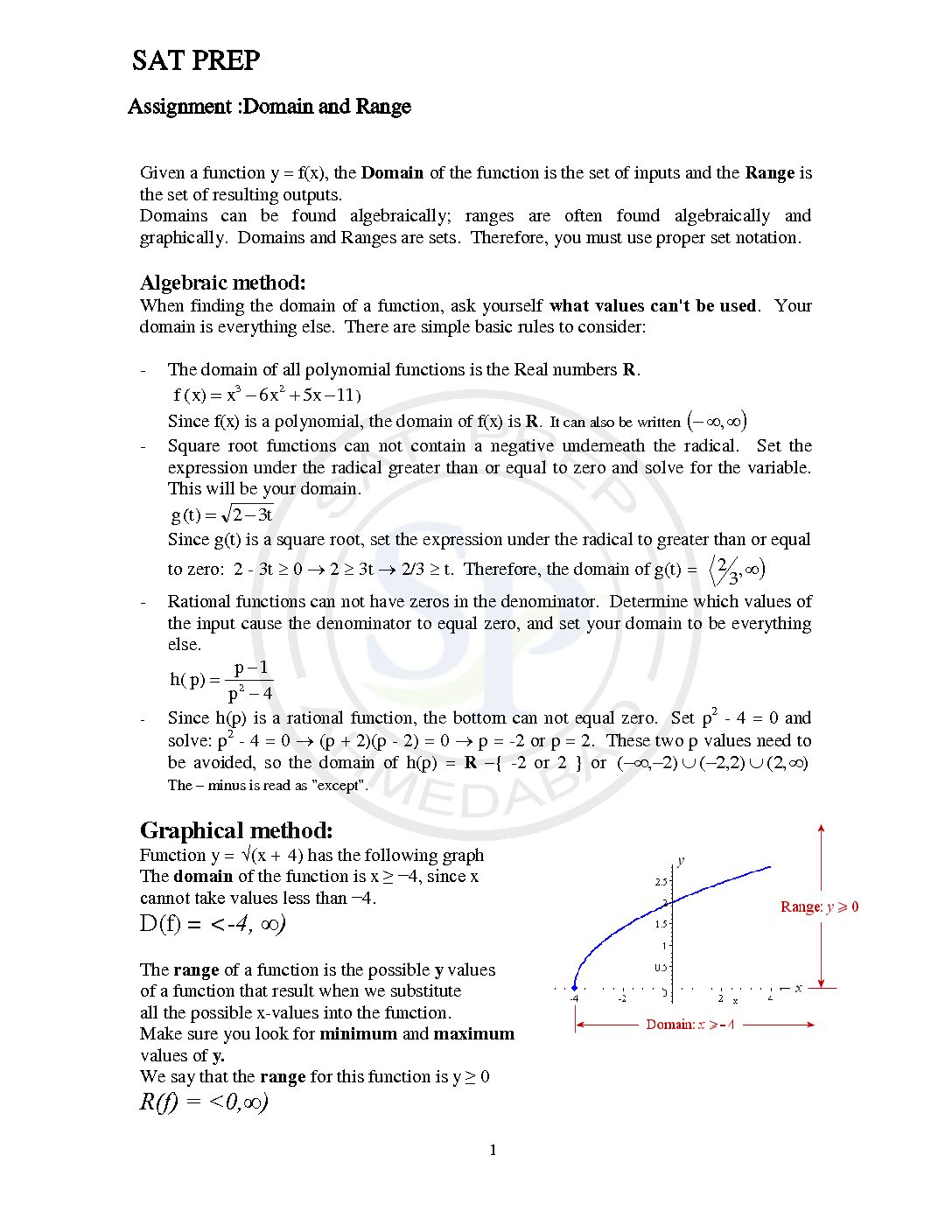
Domain and range
Domain represent values of x , while range represented by F(x) or y function. Mostly , functions are portrayed as a set of x/y coordinates. Therefore y-axis serving as a function of x. When we use function F(x) notation then one is independent variable and another dependent variable. Due to this on Y-axis F(x) represent. Domain and range
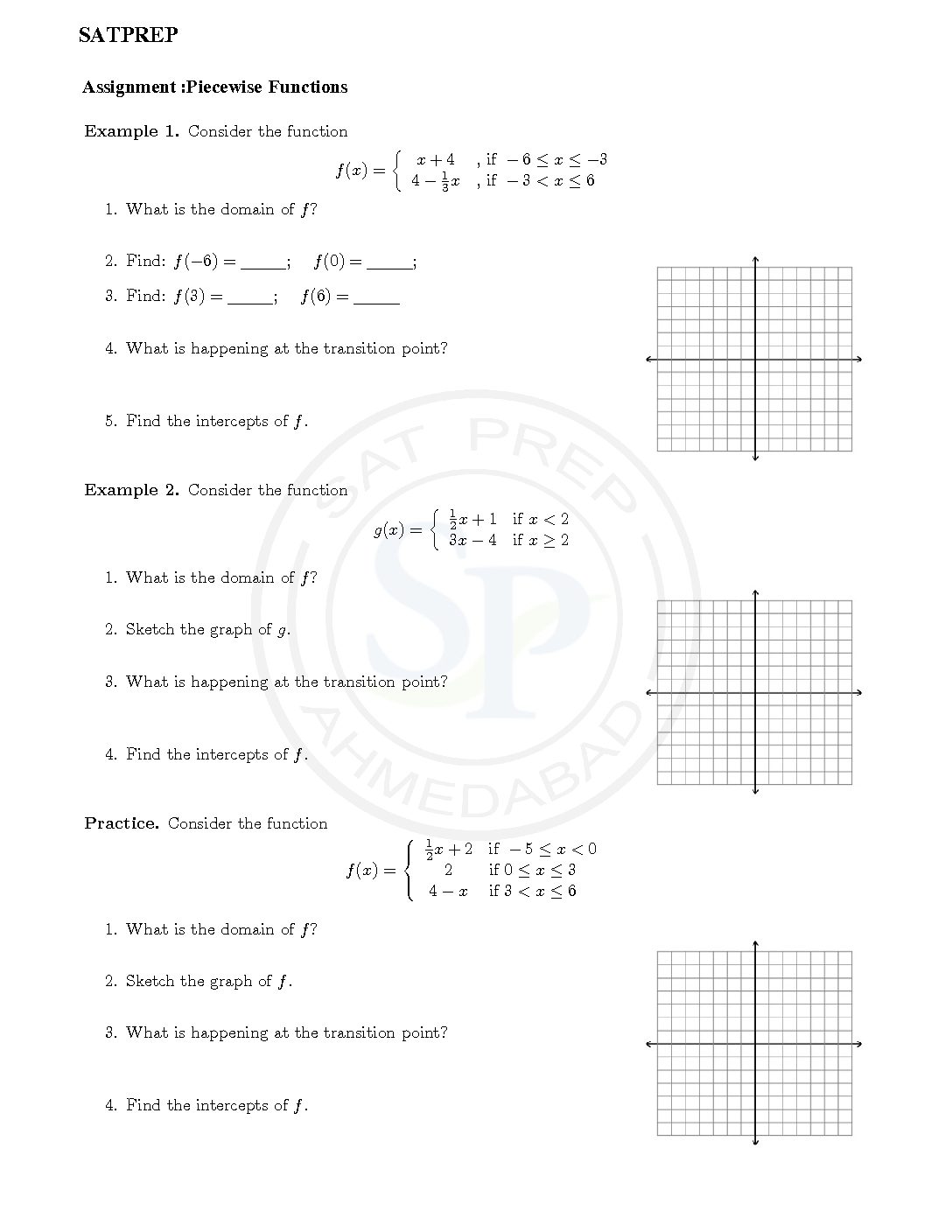
Piece wise function
Piecewise Functions. A Function Can be in Pieces. We can create functions that behave differently based on the input (x) value. Piece wise function
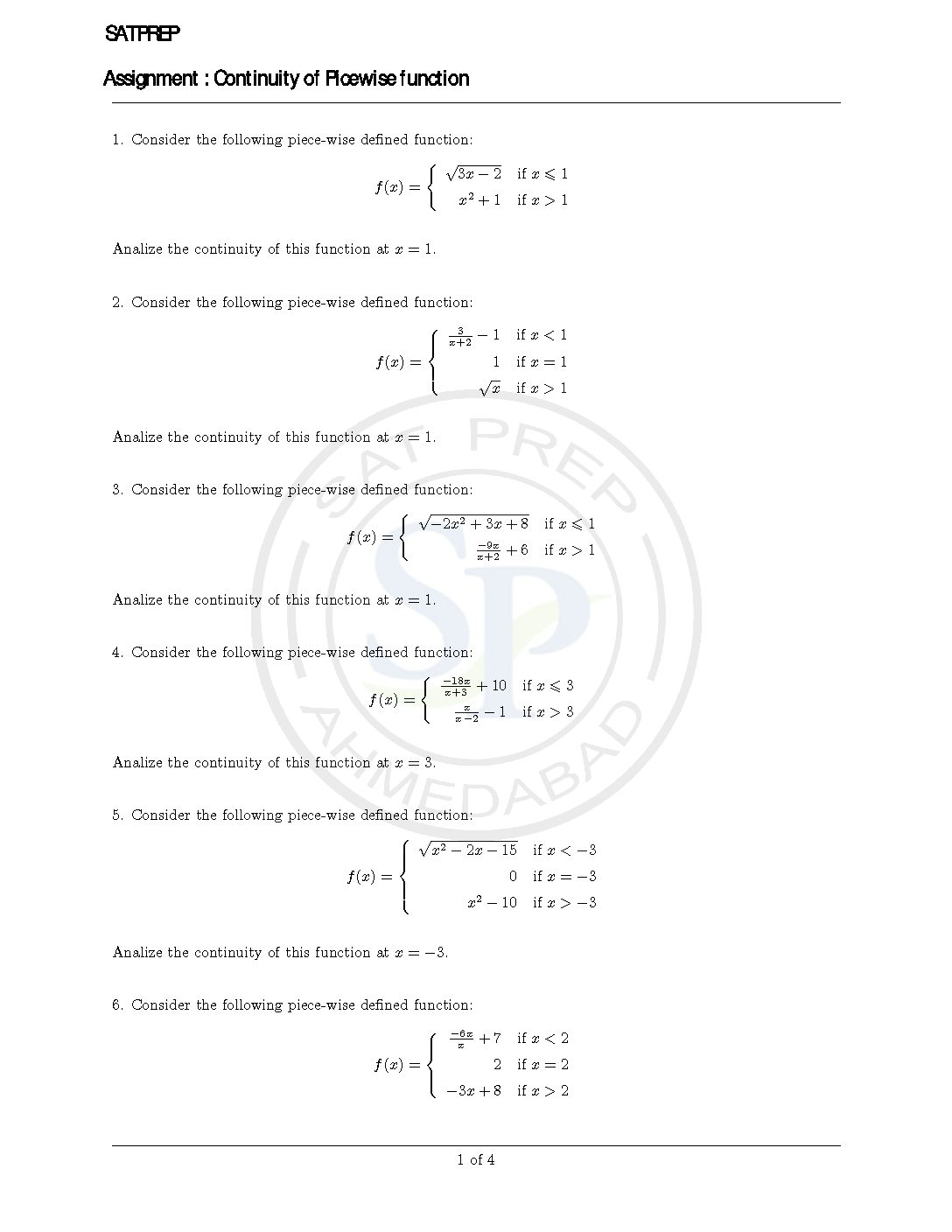
Continuity of Piecewise Function
Limit of a continuous function at a point is equal to the value of the function at that point. Piecewise Functions. The piecewise function f (x) is continuous at such a point if and only of the left- and right-hand limits of the pieces agree and are equal to the value of the f. Continuity of Piecewise function
Rules of Transformation
In this post question are from transformations of function. It basically deals with translation of functions through vector . Also it deal with reflection. Transformation