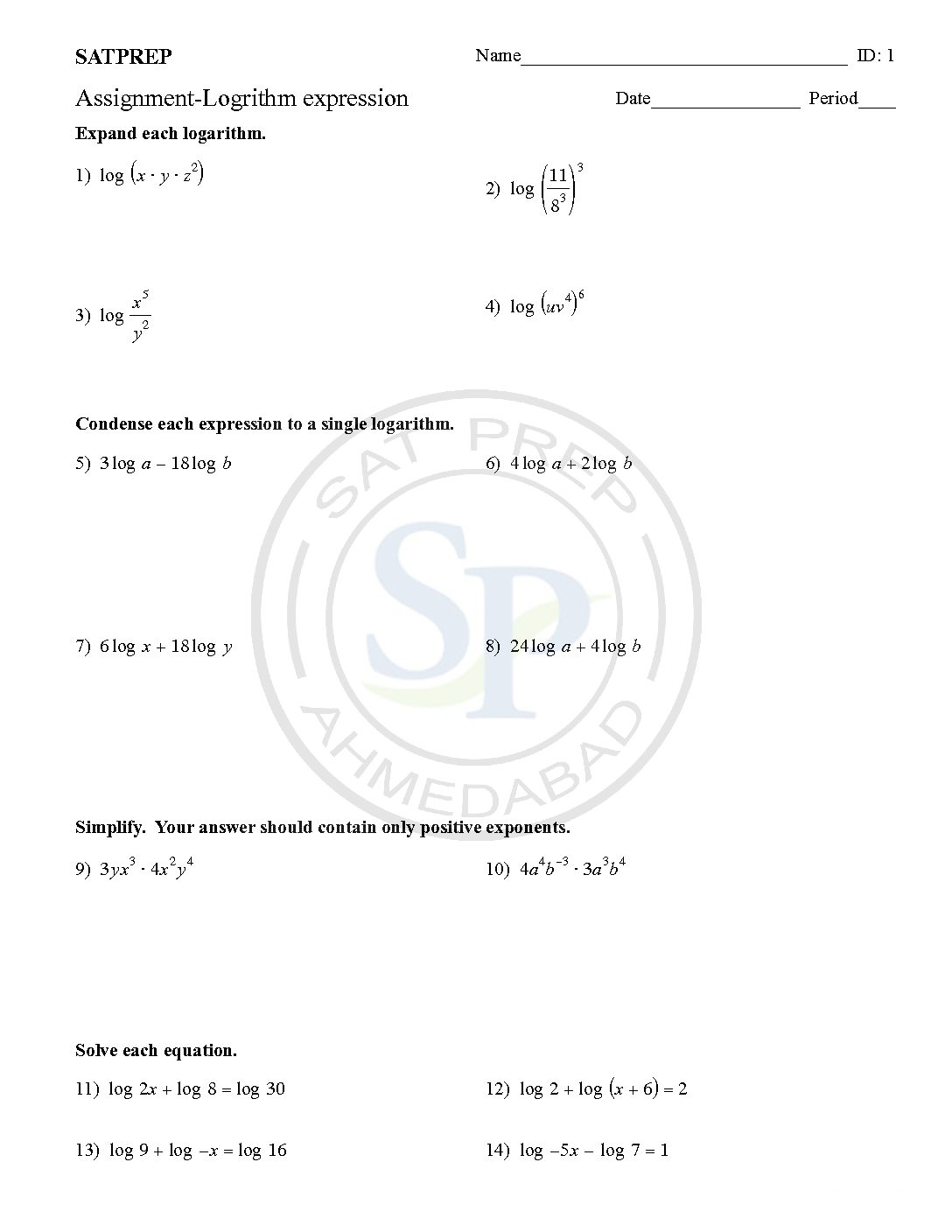
Logarithmic Expressions are simplify by Rules or Laws of Logarithms and solve by inverse of exponentiation. It is expressed by using the abbreviation “log”. Because of different base of number log notation are also different. lg for base 10 , ln for base e . Logarithm quotient rule The logarithm of the division of x and y is the difference of logarithm of x […]
You are browsing archives for
Category: Exponential and Logarithmic function
Logarithmic and exponential Function
The logarithmic function y = logax is defined to be equivalent to the exponential equation x = ay. y = logax only under the following conditions: x = ay, a > 0, and a≠1. Logarithmic functions are the inverses of exponential functions. The inverse of the exponential function y = ax is x = ay. […]
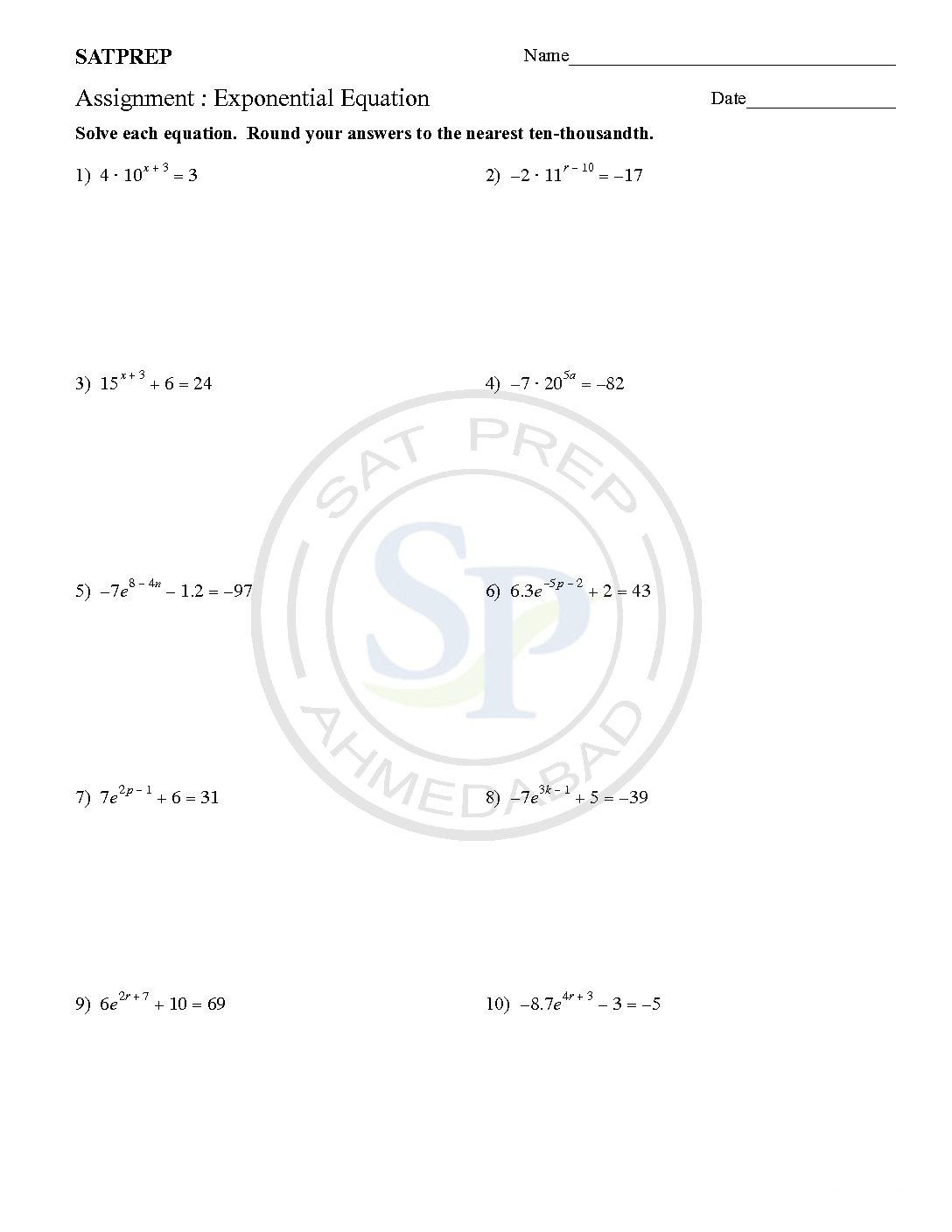
Exponential Equation
Exponential equations are one in which a variable occurs in the exponent, for example, . When both sides of the equation have the same base, the exponents on either side are equal by the property Exponential Equation
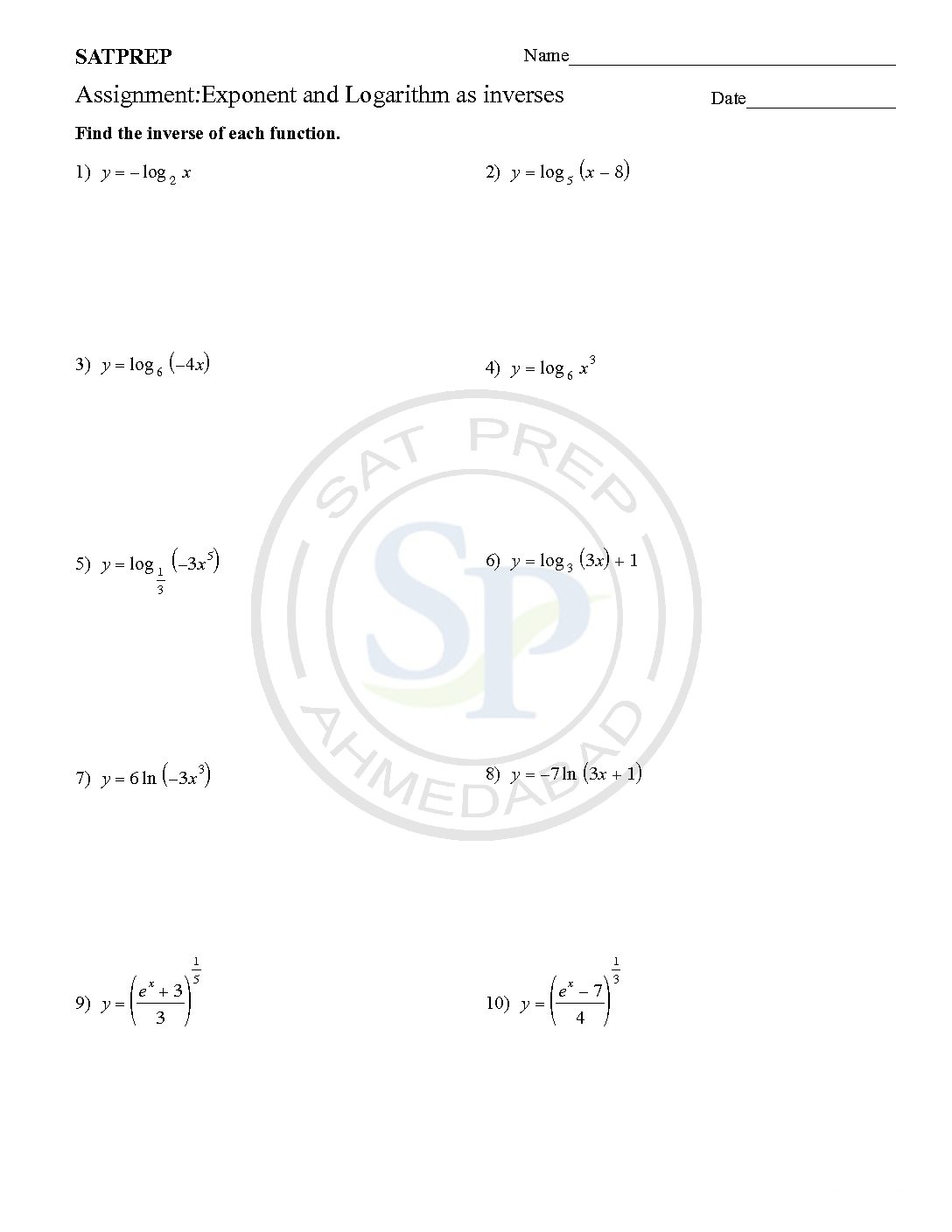
Exponent and Logarithm inverses
The inverse of the exponential function y = ax is x = ay. The logarithmic function y = logax is defined to be equivalent to the exponential equation x = ay. y = logax only under the following conditions: x = ay, a > 0, and a≠1. It is called the logarithmic function with base […]