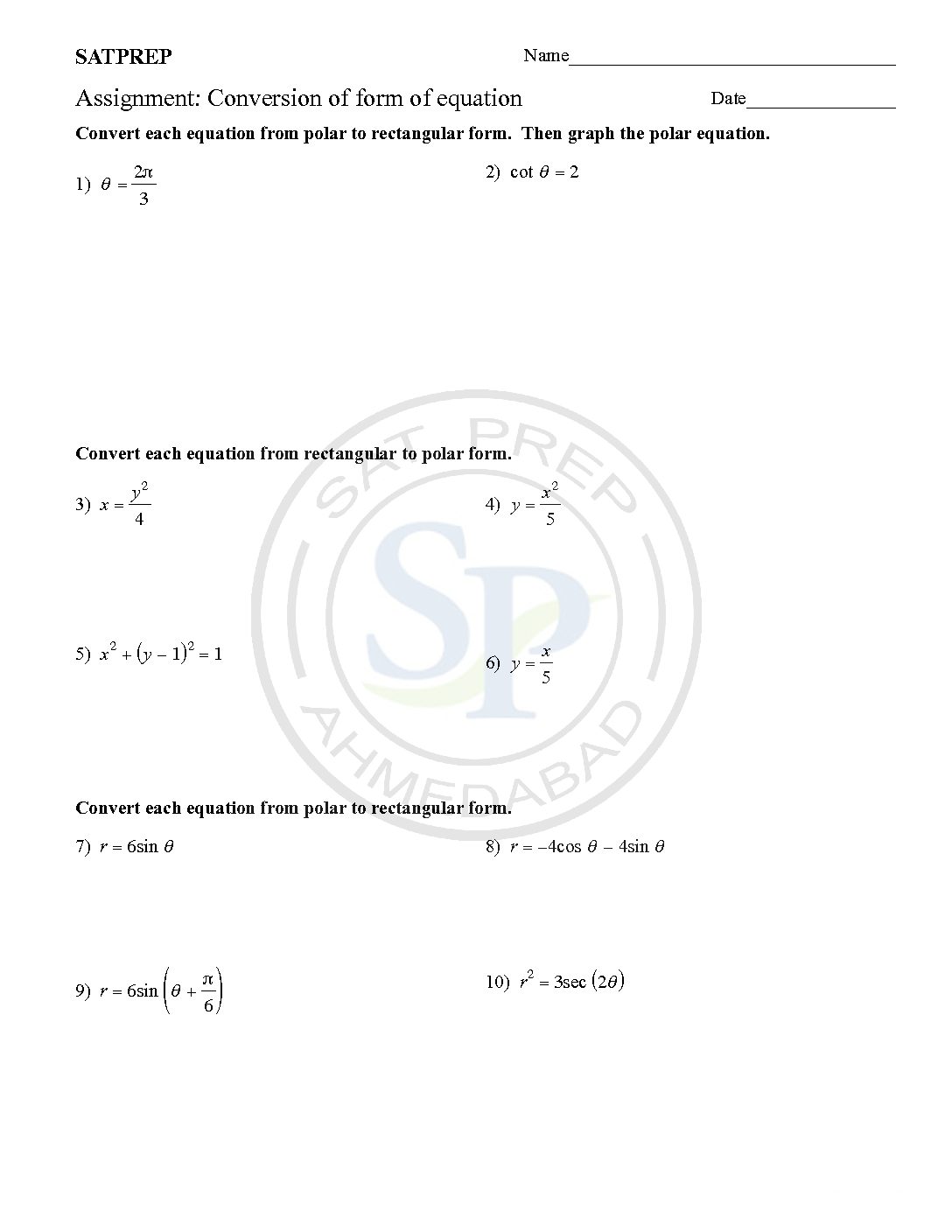
This rectangular to polar form conversion converts a number in rectangular form to its equivalent value in polar form. Rectangular forms of numbers take on the format, rectangular number= x + jy, where x and y are numbers. The x is the real number of the expression and the y represents. To Convert from Cartesian to Polar. When we know a point in Cartesian Coordinates (x,y) and we want it in Polar Coordinates […]
You are browsing archives for
Category: Placewise Function
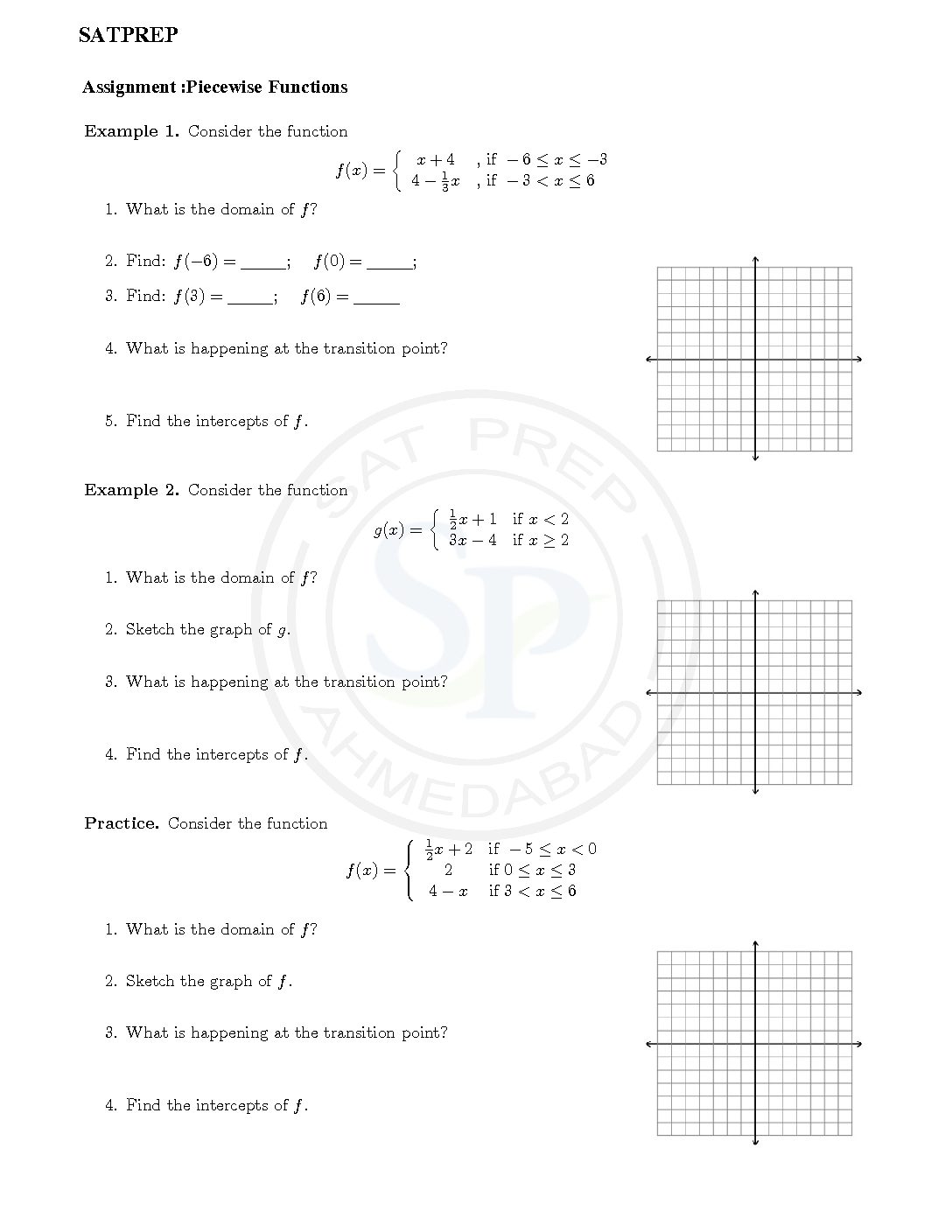
Piece wise function
Piecewise Functions. A Function Can be in Pieces. We can create functions that behave differently based on the input (x) value. Piece wise function
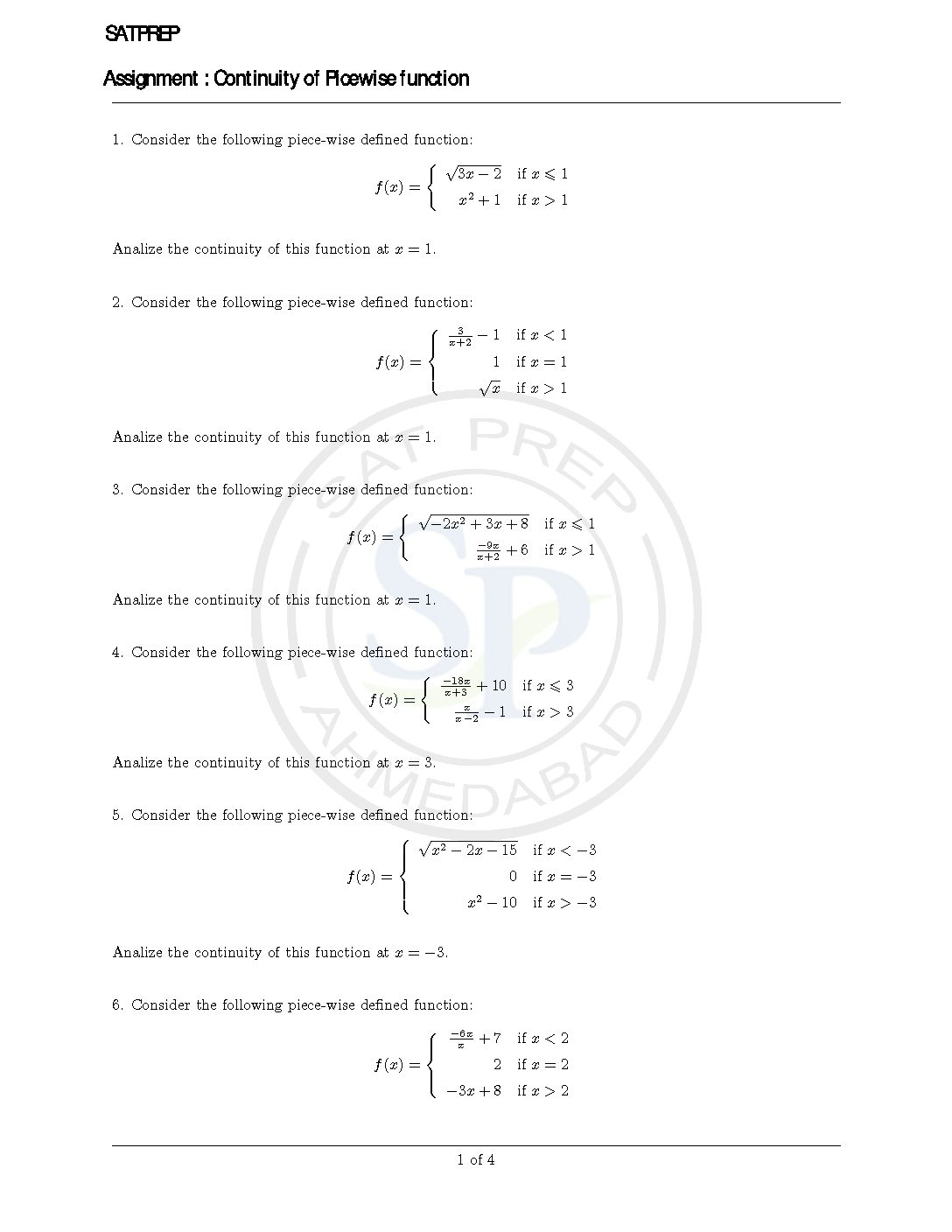
Continuity of Piecewise Function
Limit of a continuous function at a point is equal to the value of the function at that point. Piecewise Functions. The piecewise function f (x) is continuous at such a point if and only of the left- and right-hand limits of the pieces agree and are equal to the value of the f. Continuity of Piecewise function