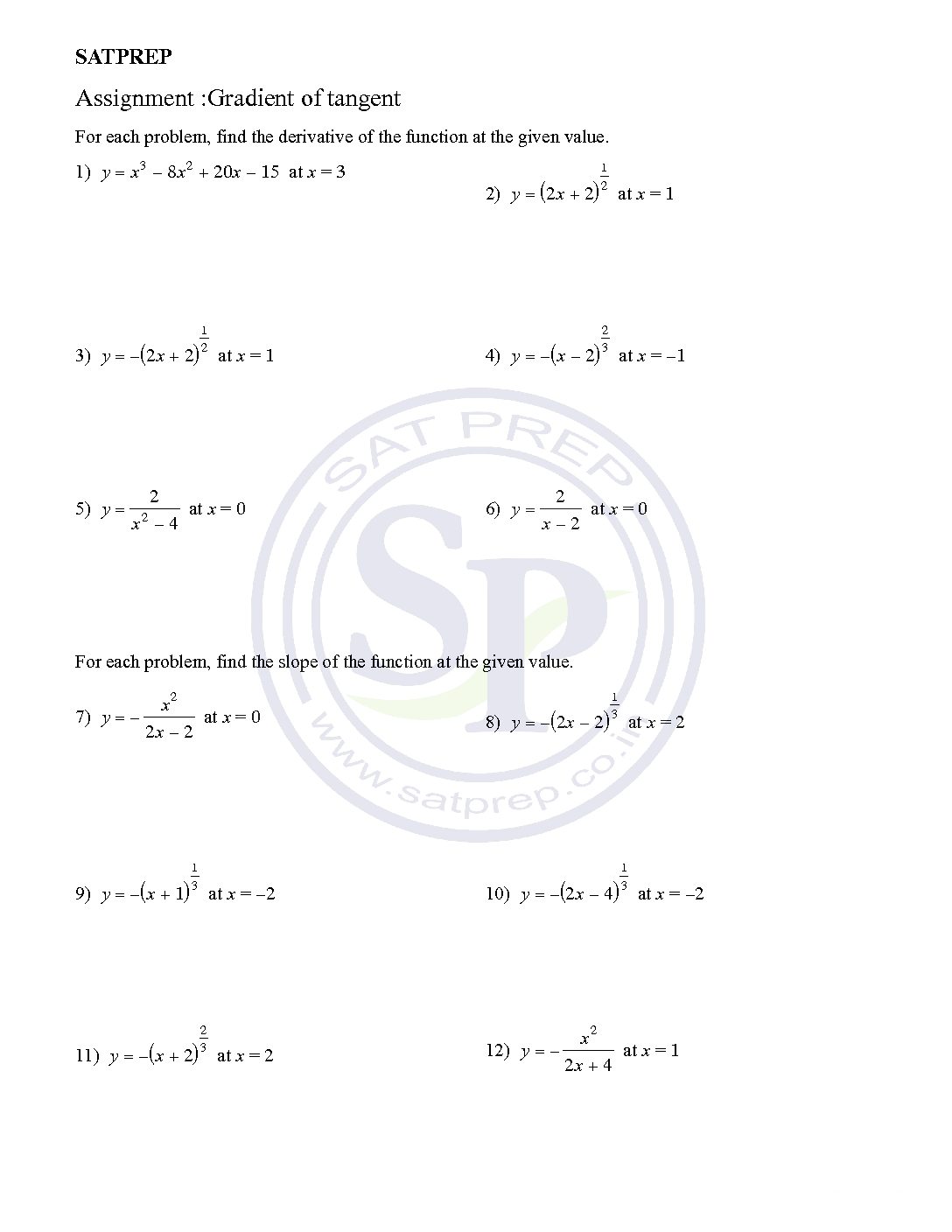
At a given point on a curve, the gradient of the curve is equal to the gradient of the tangent to the curve. The derivative (or gradient function) describes the gradient of a curve at any point on the curve. Similarly, it also describes the gradient of a tangent to a curve at any point […]
You are browsing archives for
Tag: gradient
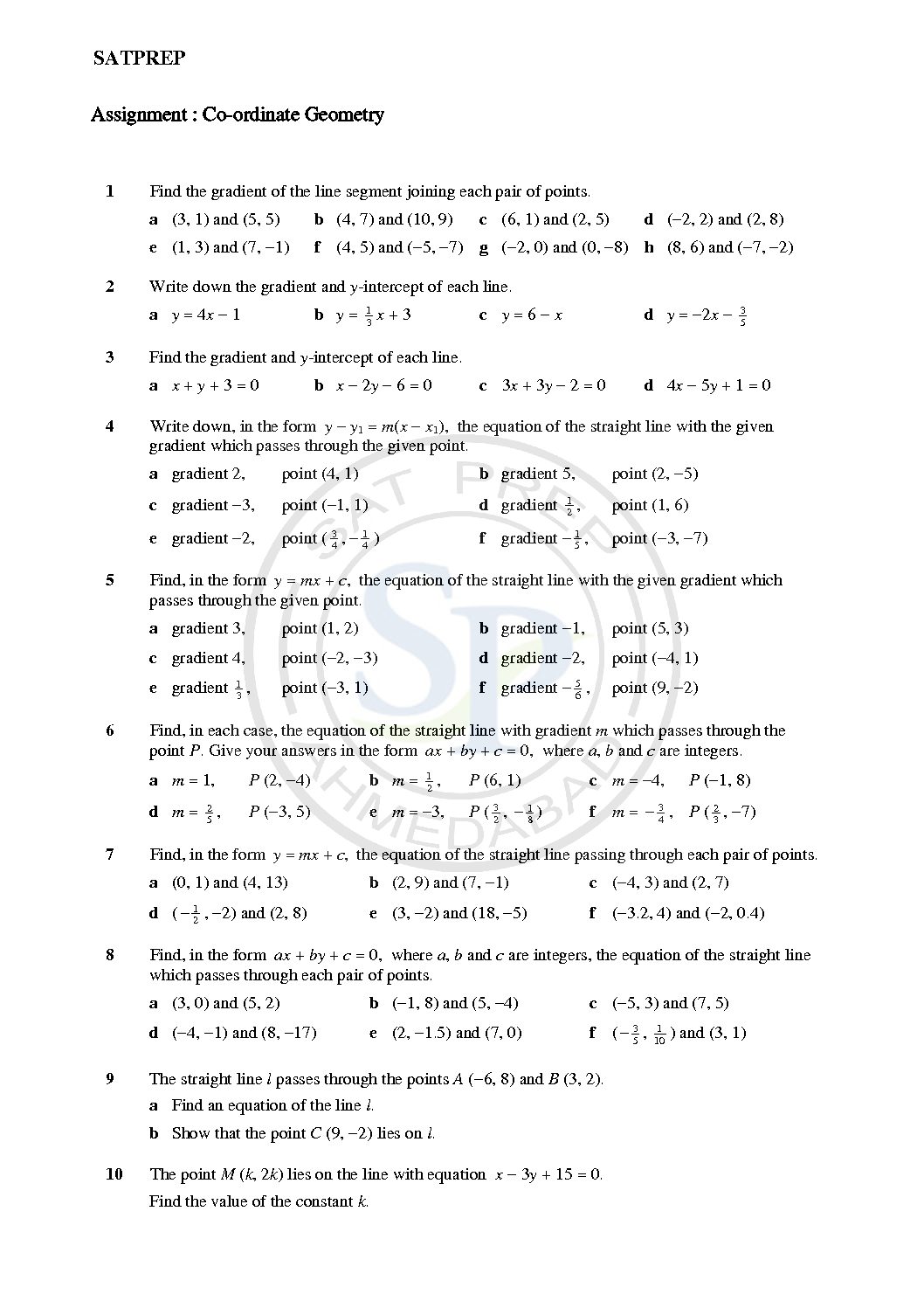
Co-ordinate geometry
A coordinate geometry is a branch of geometry. In this, the position of the points on the plane supported by an ordered pair of numbers also known as coordinates. Coordinate geometry deal about gradient , distance , mid point and equation of line. Also, condition of lines like parallel , perpendicular and inclined lines. In […]
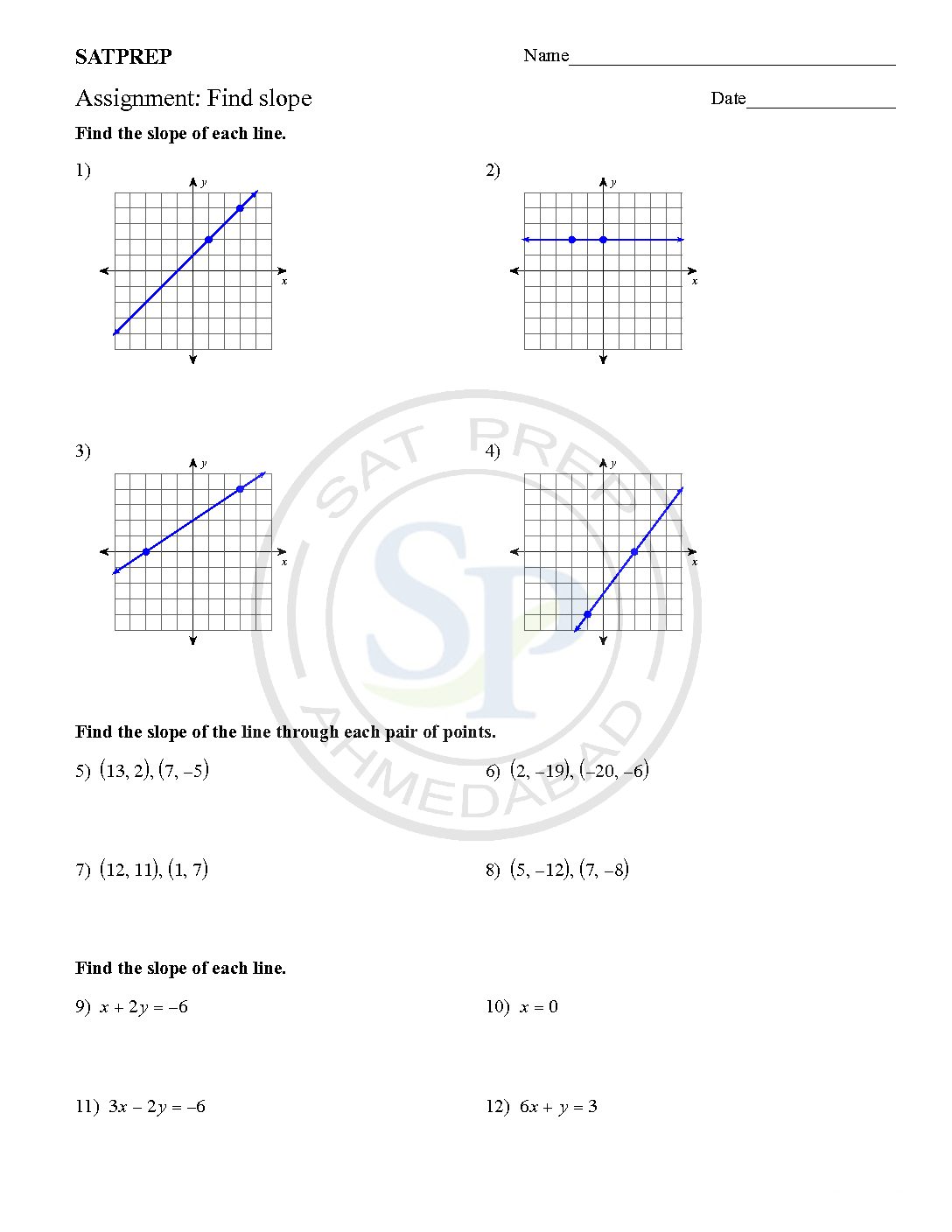
Find slope
Tangent and normal : Implicit
Tangent and Normal Lines for implicit curves are a line that touches the implicit curve at one point and has the same slope as the curve at that point. A normal to a curve is a line perpendicular to a tangent to the curve. tangent and normal
Co-ordinate geometry -2
Ordered pair of numbers also known as coordinates. Hence manipulation of coordinate such as Determine the distance between these points. Find the equation, midpoint, and slope of the line segment. Determine if the given lines are perpendicular or parallel. Also deal with graphic representation. Coordinate geometry
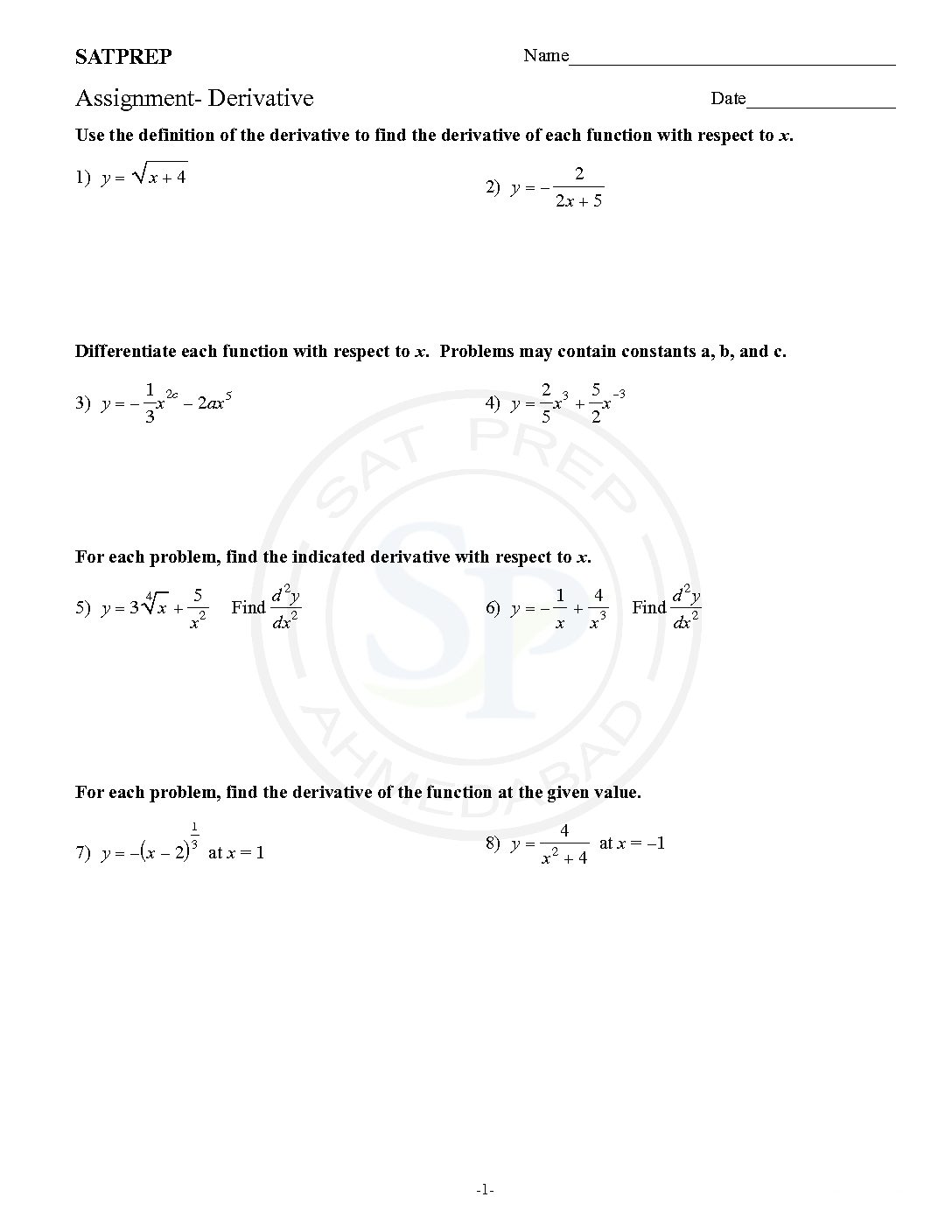
Derivative of polynomials
Derivative is product of differentiation. Differentiation has applications to nearly all quantitative disciplines. For example, in physics, the derivative of the displacement of a moving body with respect to time is the velocity of the body, and the derivative of velocity with respect to time is acceleration. Therefore differentiation is process Derivative