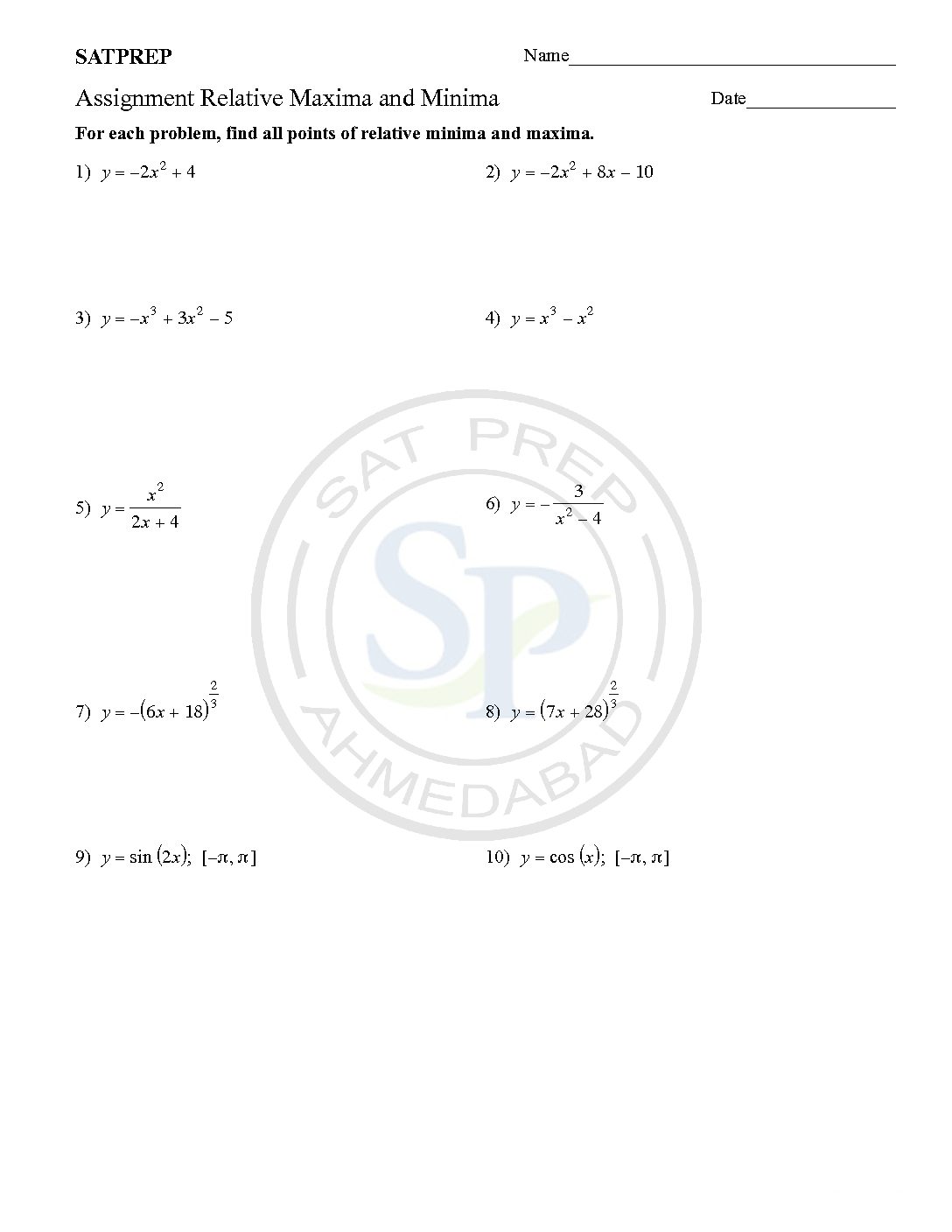
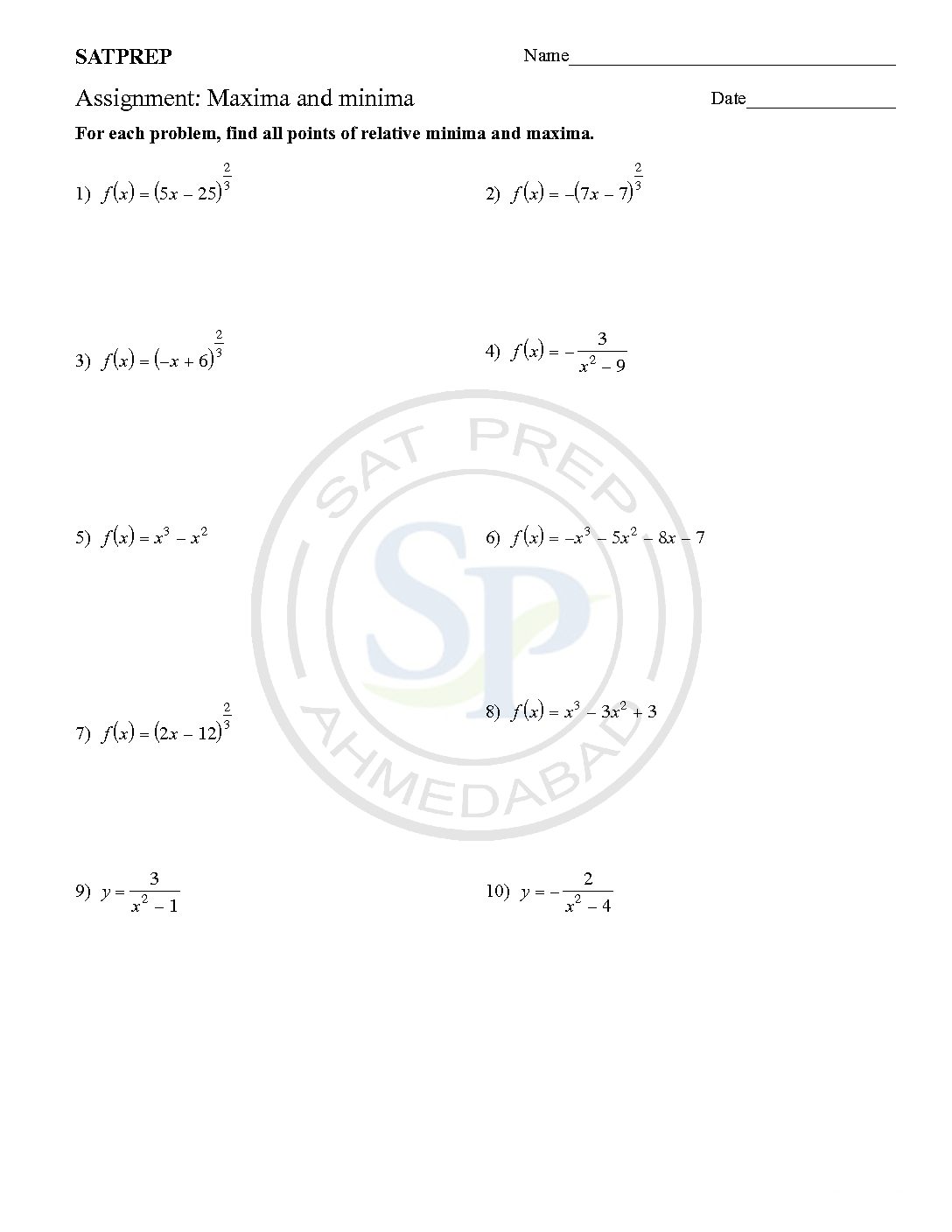
A high point of curve is called a maxima. A low point is called a minima. In the Curve only one global maxima or minima exists , while more than one local maximum or minimum. Due to curve turn on these point are called local. Hence these point also called stationary points. Maxima and minima
You are browsing archives for
Tag: optimization
Maxima and Minima
Maximum and minimum of Points of Inflection. The value f ‘(x) is the gradient at any point but often we want to find the Turning or Stationary Point (Maximum and Minimum points) or Point of Inflection These happen where the gradient is zero, f ‘(x) = 0. Critical Points include Turning points and Points where f ‘ (x) does […]
Optmization-3
Process of optimisation means optimal value of function at turning point (maximum or minimum ) value of the curve. Therefore second derivative use to find greatest or least value . Also it show greatest value. Optimization
Optimisation
Process of calculating optimal value of function at turning point (maximum or minimum )value of the curve. Hence we use second derivative. It also give greatest or least value of curve. Optimisation