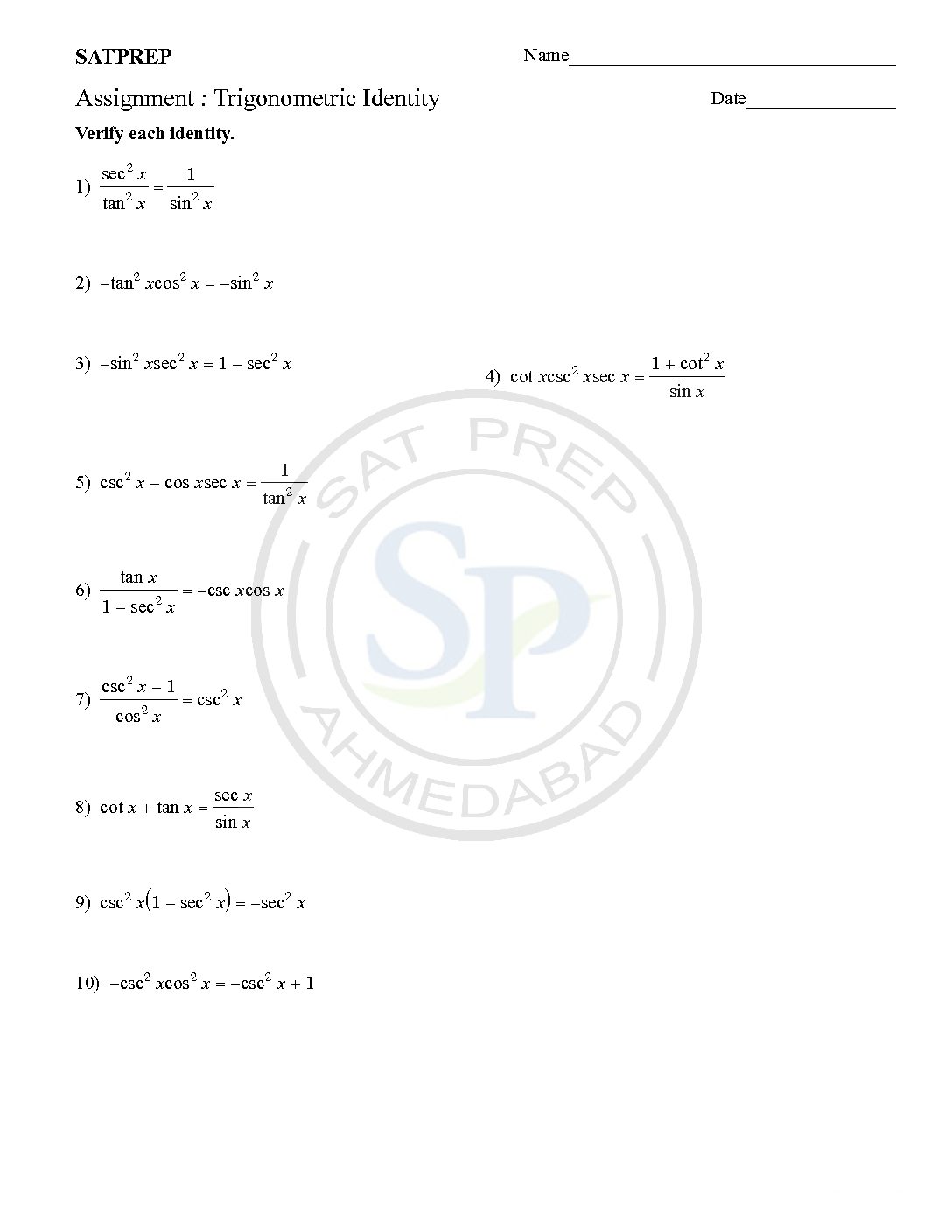
The trigonometric identity showing that the identity is always true, no matter what value of x or θ is used. Because it has to hold true for all values of x, we cannot simply substitute in a few values of x to “show” that they are equal. We have to use logical steps to show […]
You are browsing archives for
Category: Additional Maths
Trigonometric Identity
trigonometry identities showing that the identity is always true, no matter what value of x or θ is used. Because it has to hold true for all values of x, we cannot simply substitute in a few values of x to “show” that they are equal. We have to use logical steps to show that one […]
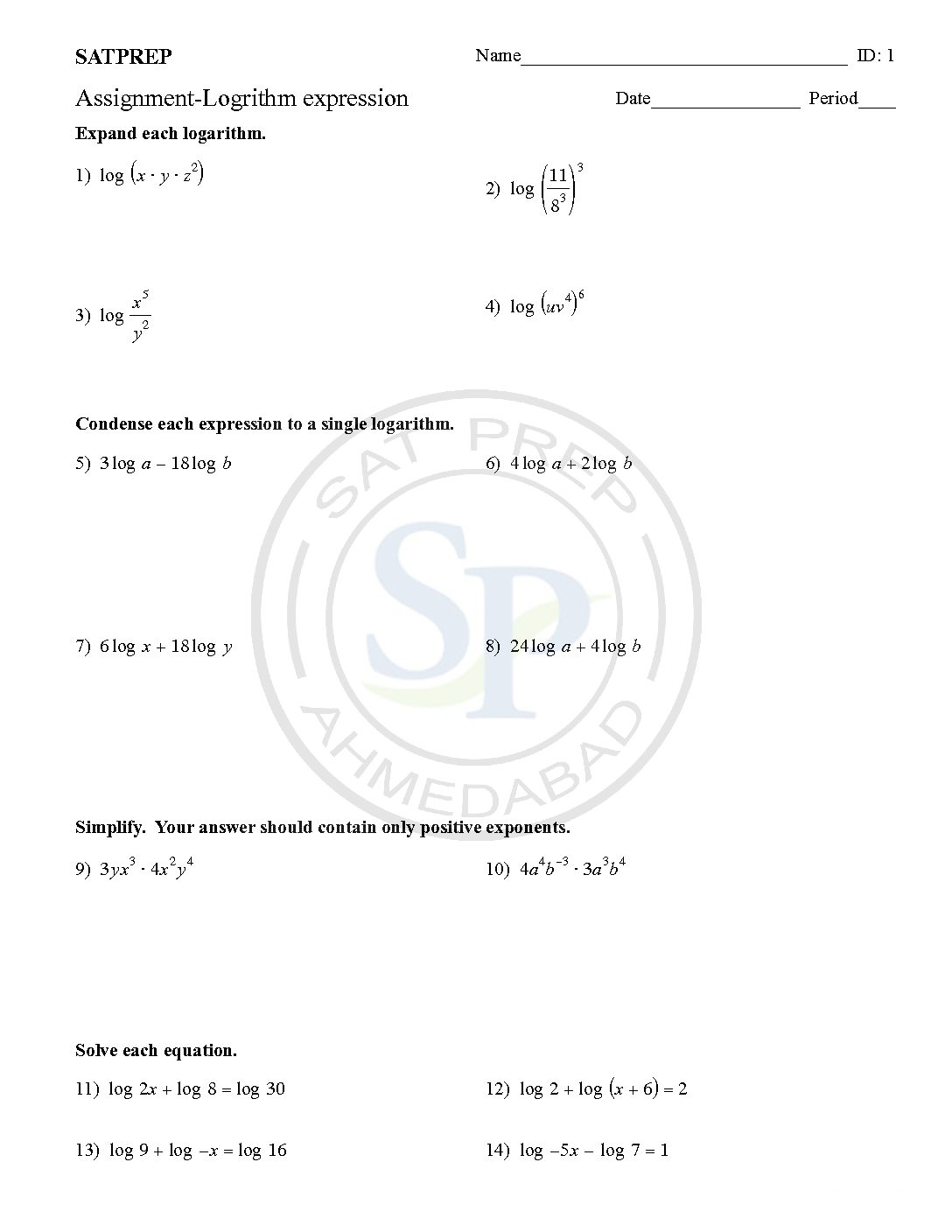
Logarithmic Expression
Logarithmic Expressions are simplify by Rules or Laws of Logarithms and solve by inverse of exponentiation. It is expressed by using the abbreviation “log”. Because of different base of number log notation are also different. lg for base 10 , ln for base e . Logarithm quotient rule The logarithm of the division of x and y is the difference of logarithm of x […]
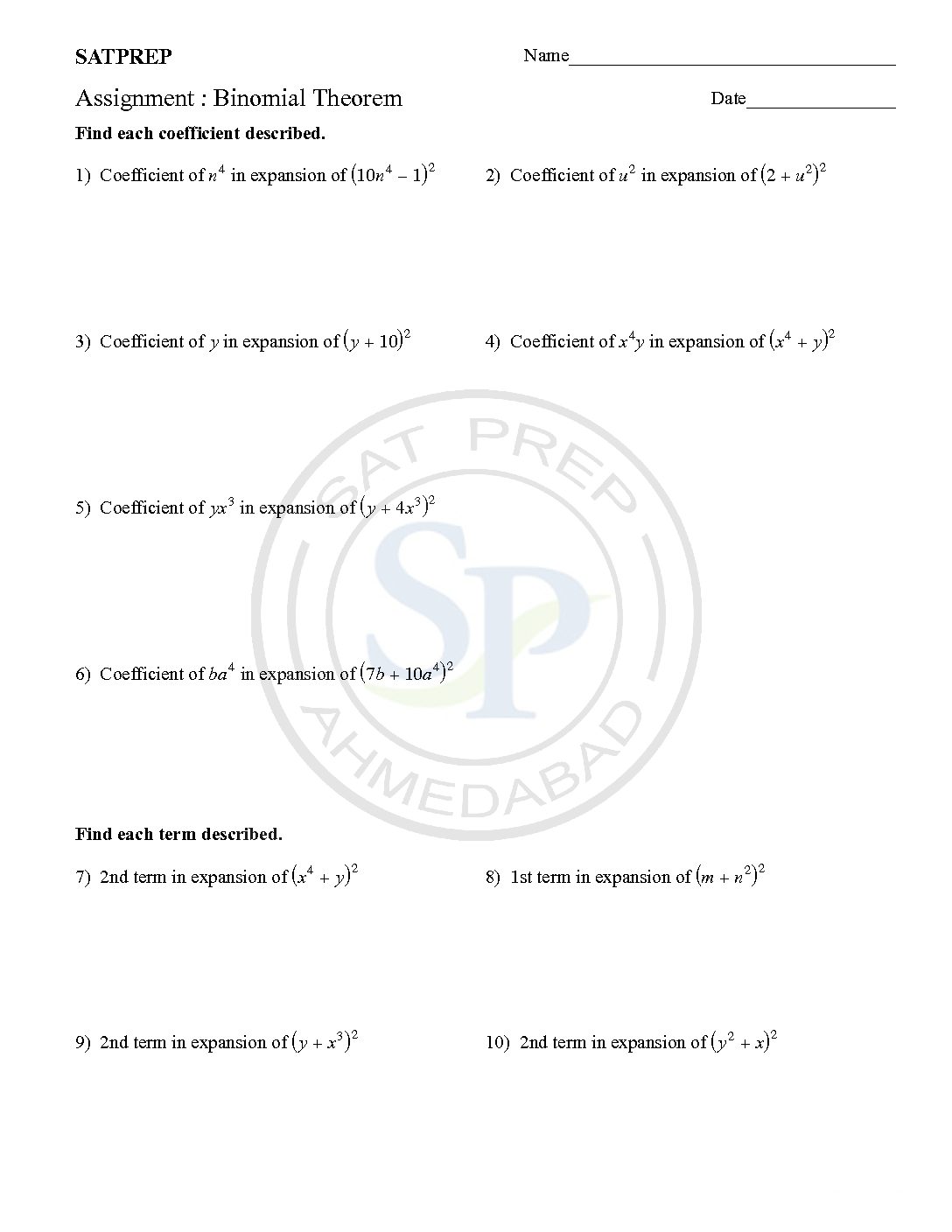
Binomial Theorem
Binomial theorems is another ways of expansion of two terms. Another way it is generalised form of expansion. Due to expansion of two term it is binomial. “What are the binomial coefficients?” . It shows how to calculate the coefficients in the expansion of (a + b) n. The symbol for a binomial coefficient nCr. As well as pascal […]
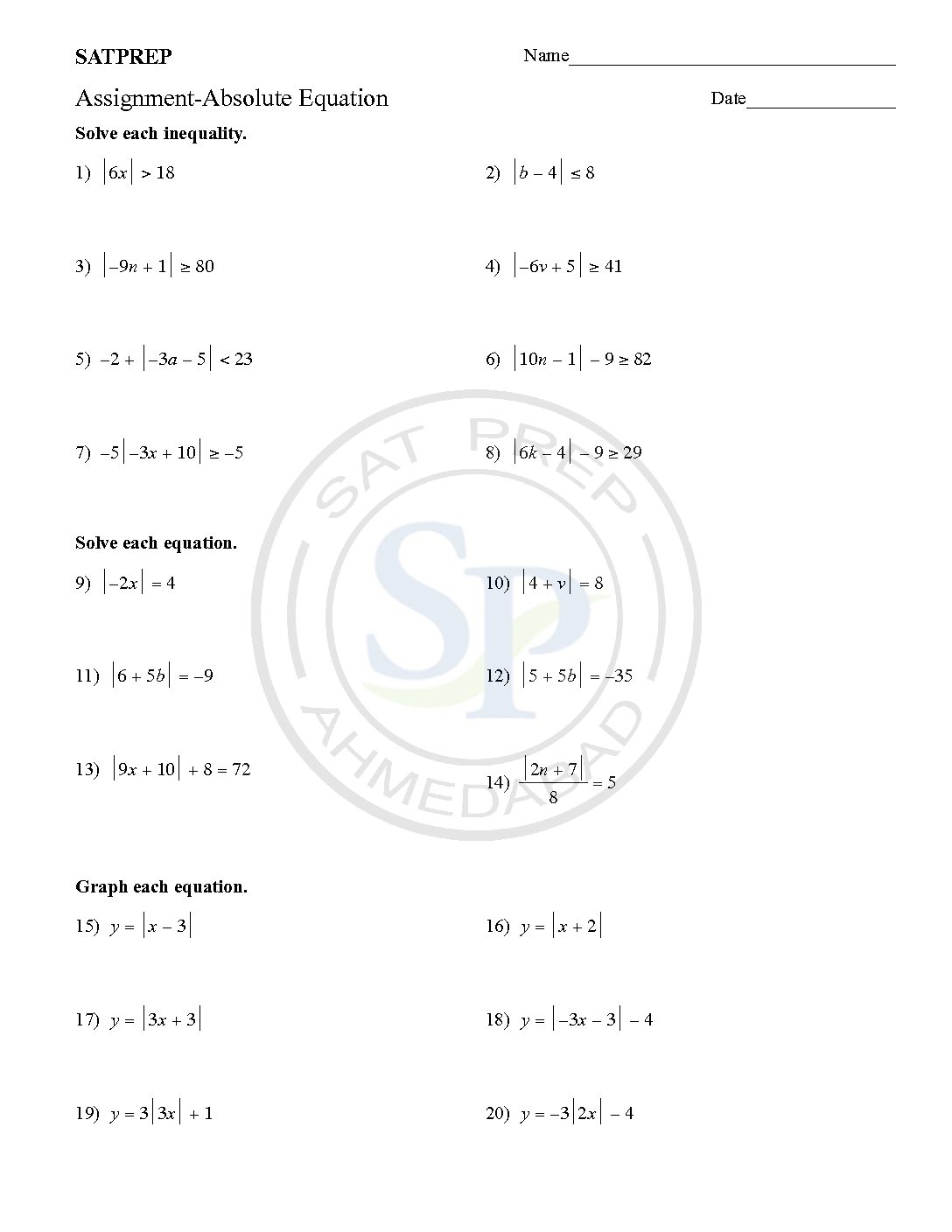
Absolute Equation
Solving absolute value equations and inequalities. And represents the distance between a and 0 on a number line. An absolute value equation is an equation that contains an absolute value expression. Has two solutions x = a and x = -a because both numbers are at the distance a from 0. Absolute Equation
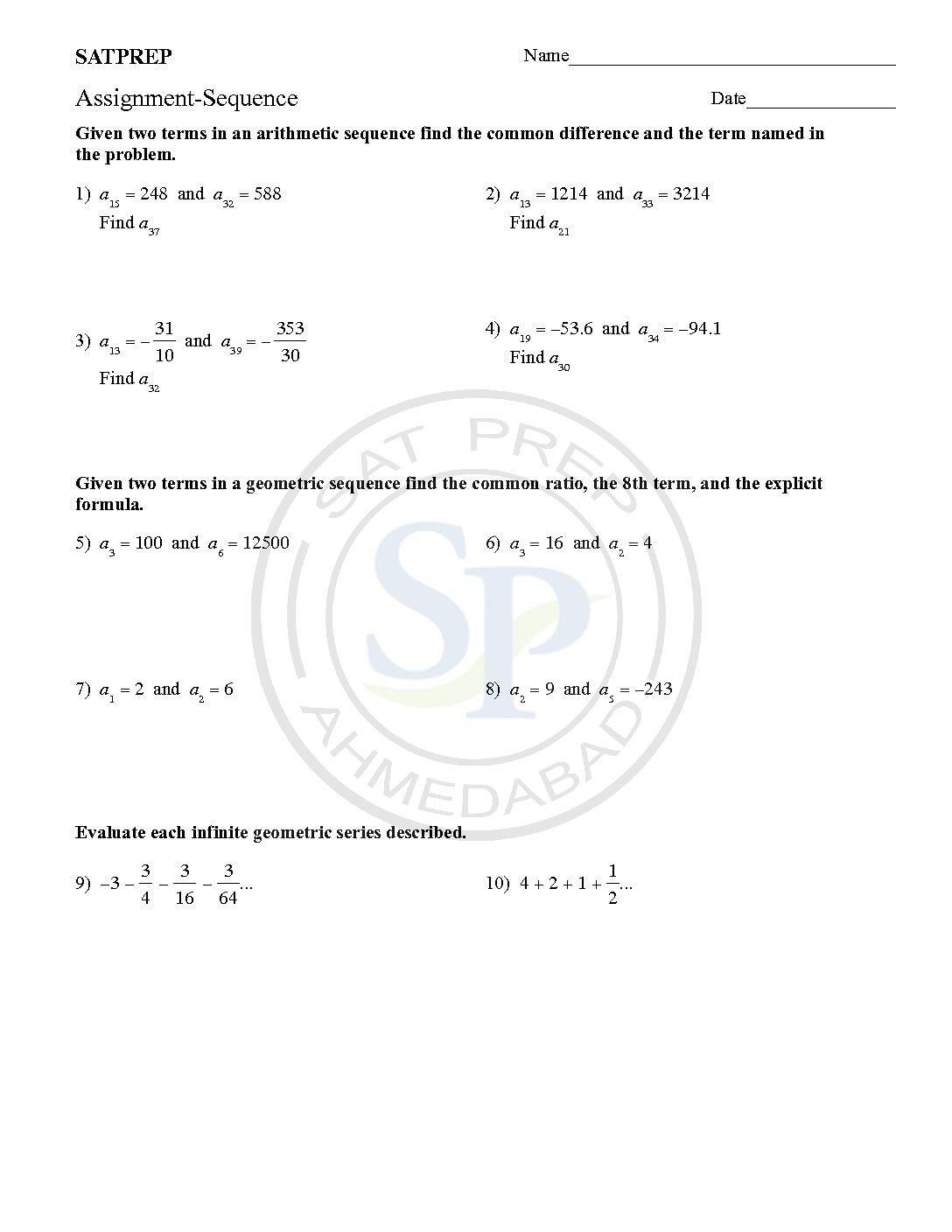
Sequence-2
Sequence and series is arrangement of term in particular pattern. Mathematical structures using the convergence properties of sequences. In particular, sequences are the basic for series Sequence and series
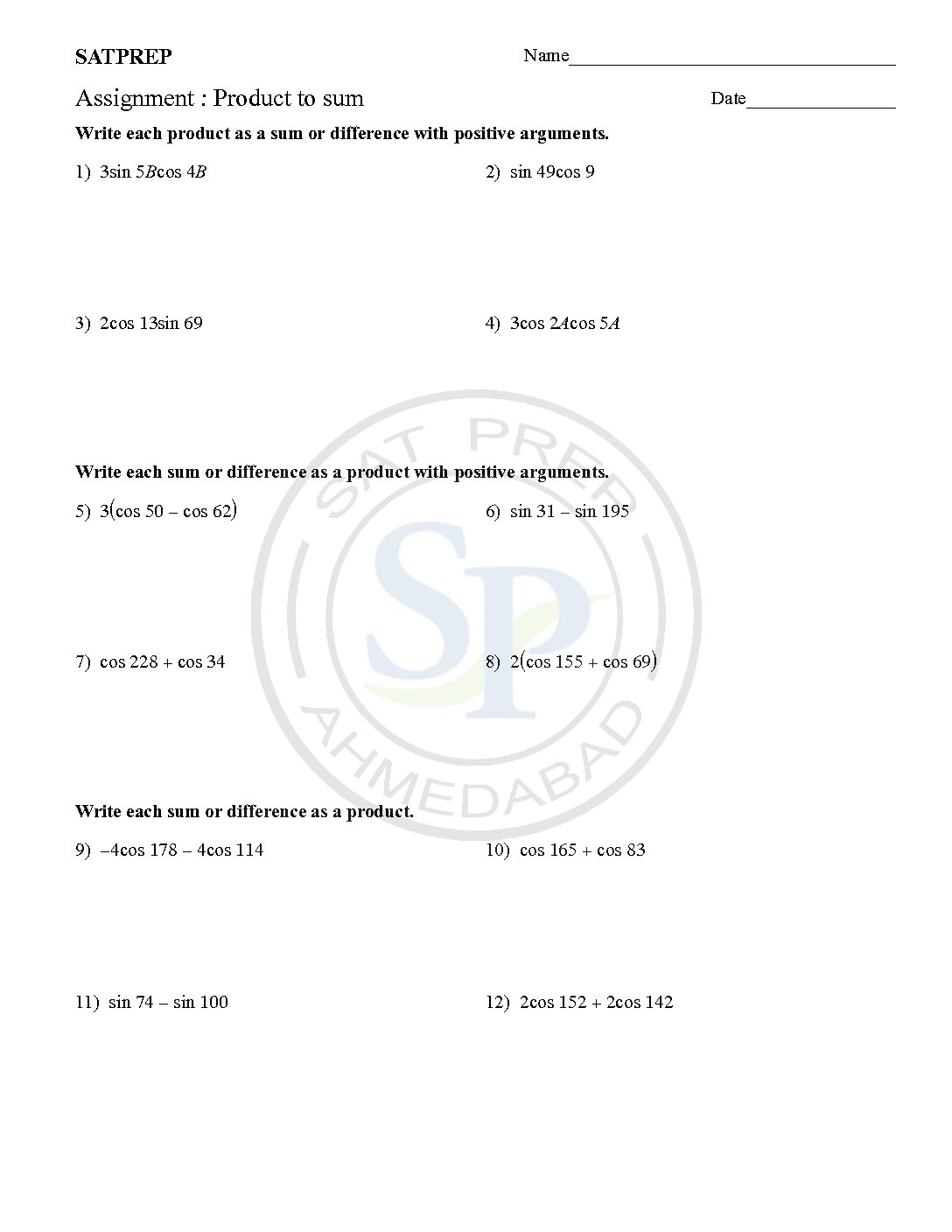
Product to Sum
Product‐Sum and Sum‐Product Identities. The process of converting products into sums can make a difference . Integrate \( \int \! \sin 3x \cos 4x \, \mathrm{d}x.\) This problem may seem tough at first, but after using the product-to-sum trigonometric formula, this integral very quickly changes into a standard form . Converting a sum of trig functions into a product. Write as and then […]
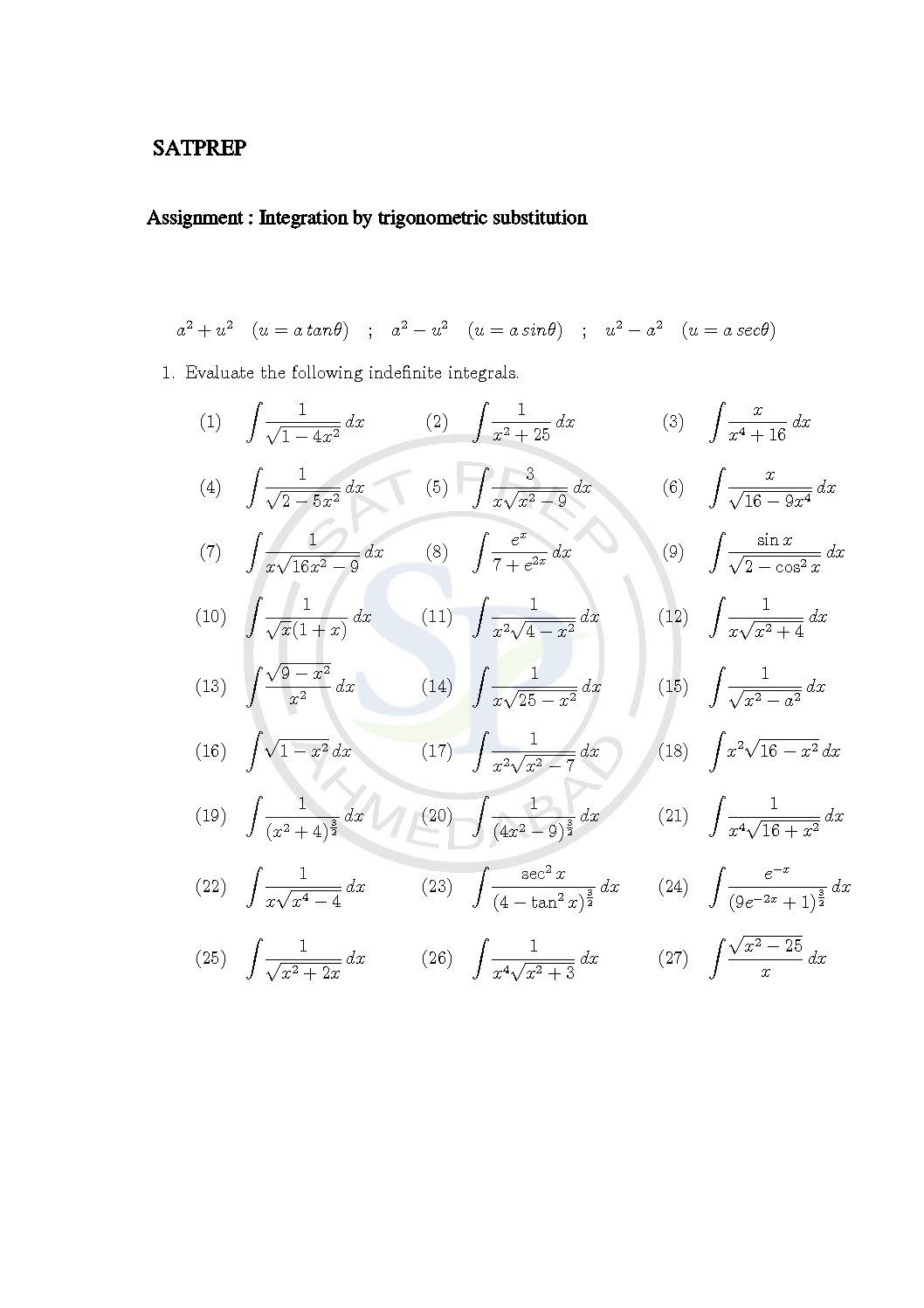
Integration by trigonometric substitutio...
This post is about worksheet of Integration by trigonometric substitution. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by substitution
Applications of Integration(Kinematics)
This post about Application of Integration into Kinematics. Solve for displacement given a velocity function in time. Solve for displacement and velocity given an acceleration function in time, & distinguish between displacement and total distance. kinematics
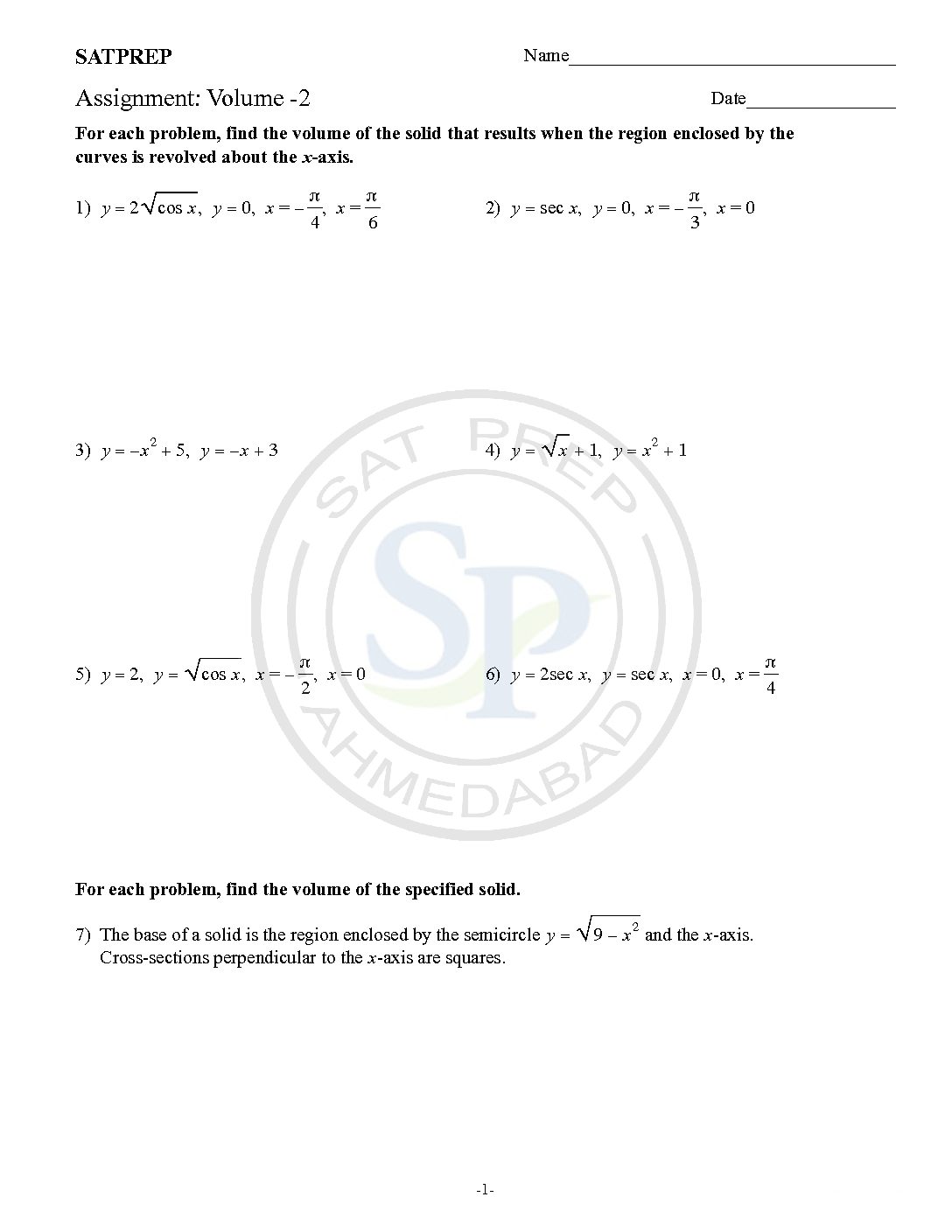
Volume -2
To get a solids of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. Volume