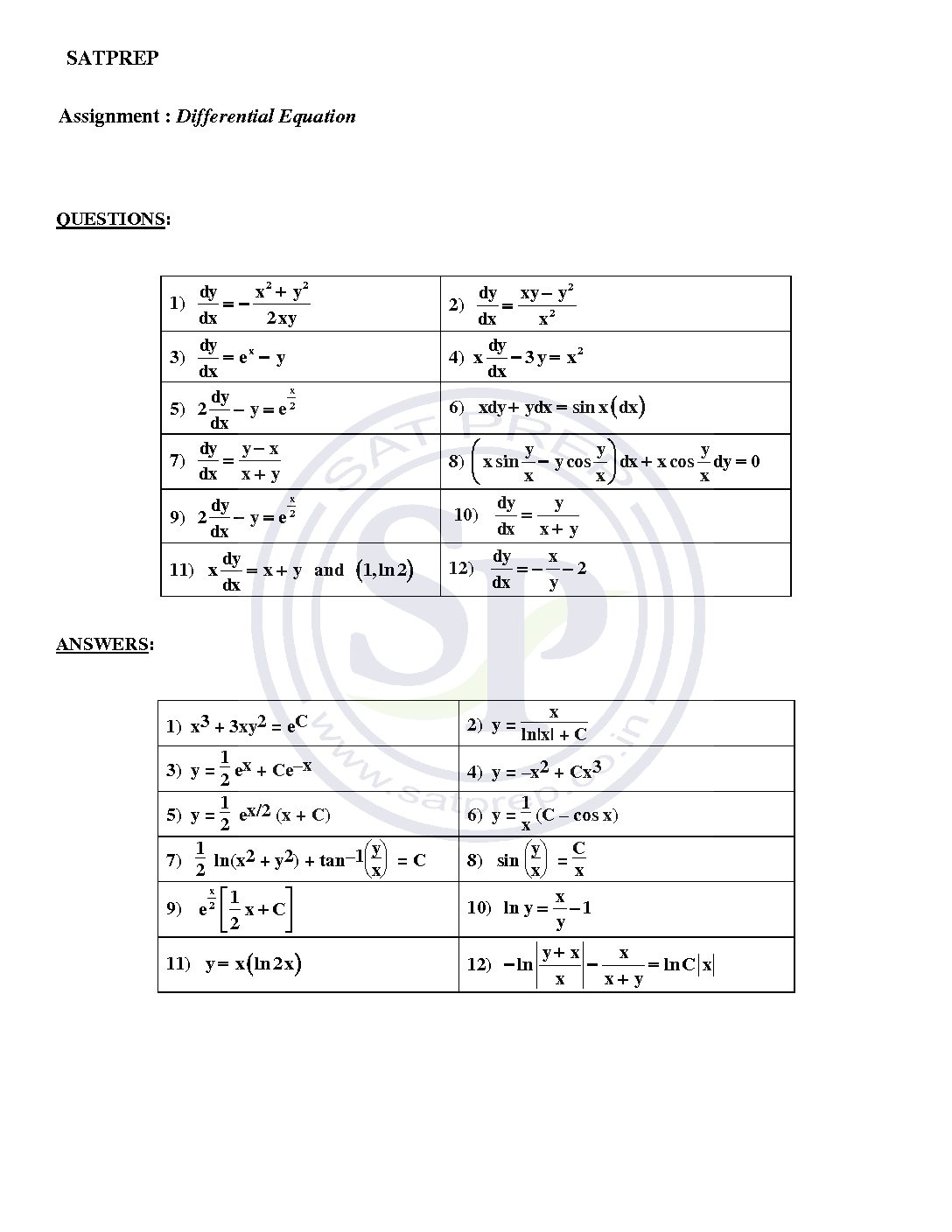
An equation that relates one or more functions and their derivatives. In applications, the functions generally represent physical quantities, the derivatives represent their rates of change. Therefore , differential equation defines a relationship between the two. Differential Eq.
You are browsing archives for
Category: Integration
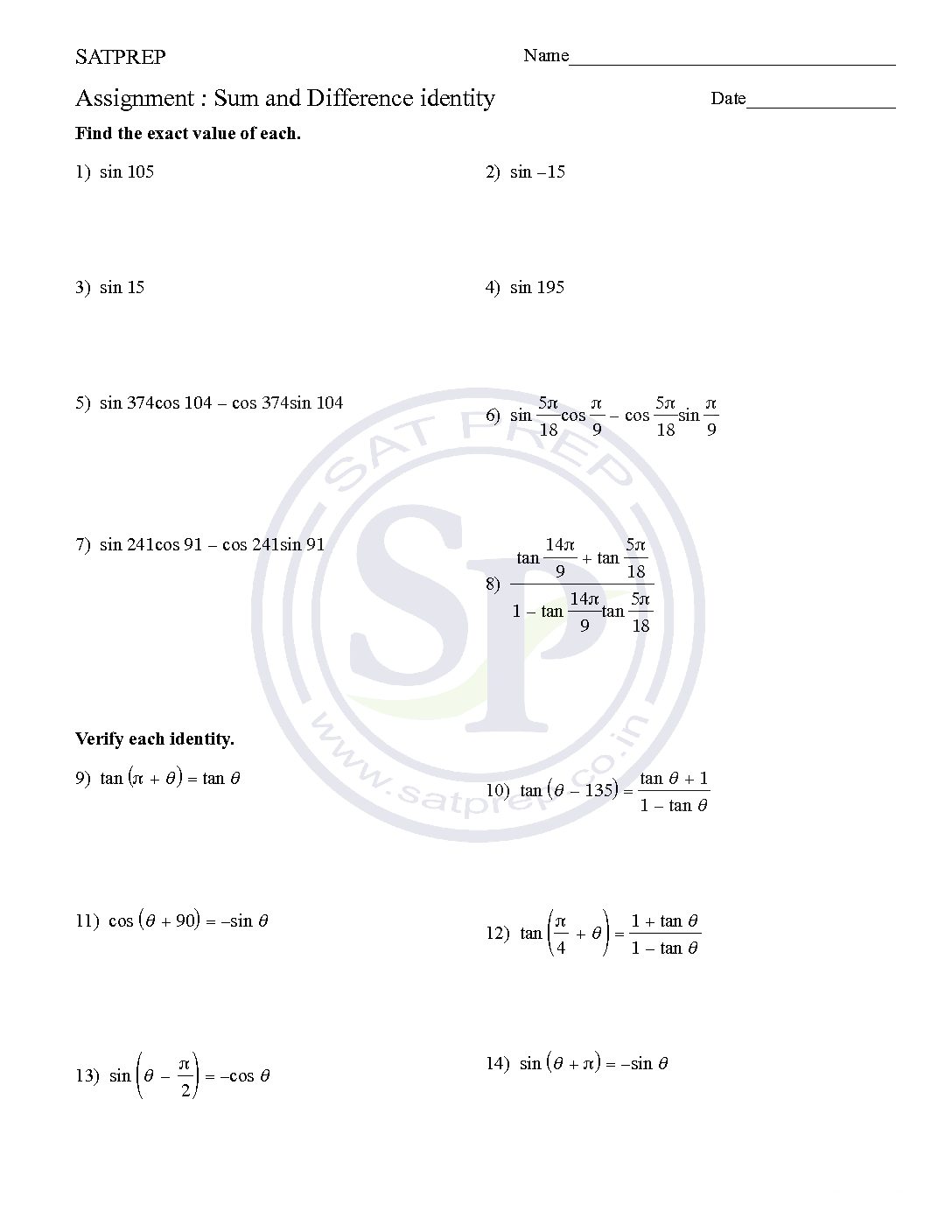
Sum and Difference identity
Sum or difference of angle is called compound angle. Similarly algebraic sum of two or more angles also called compound angle . Hence it solve . Sum and Difference identity
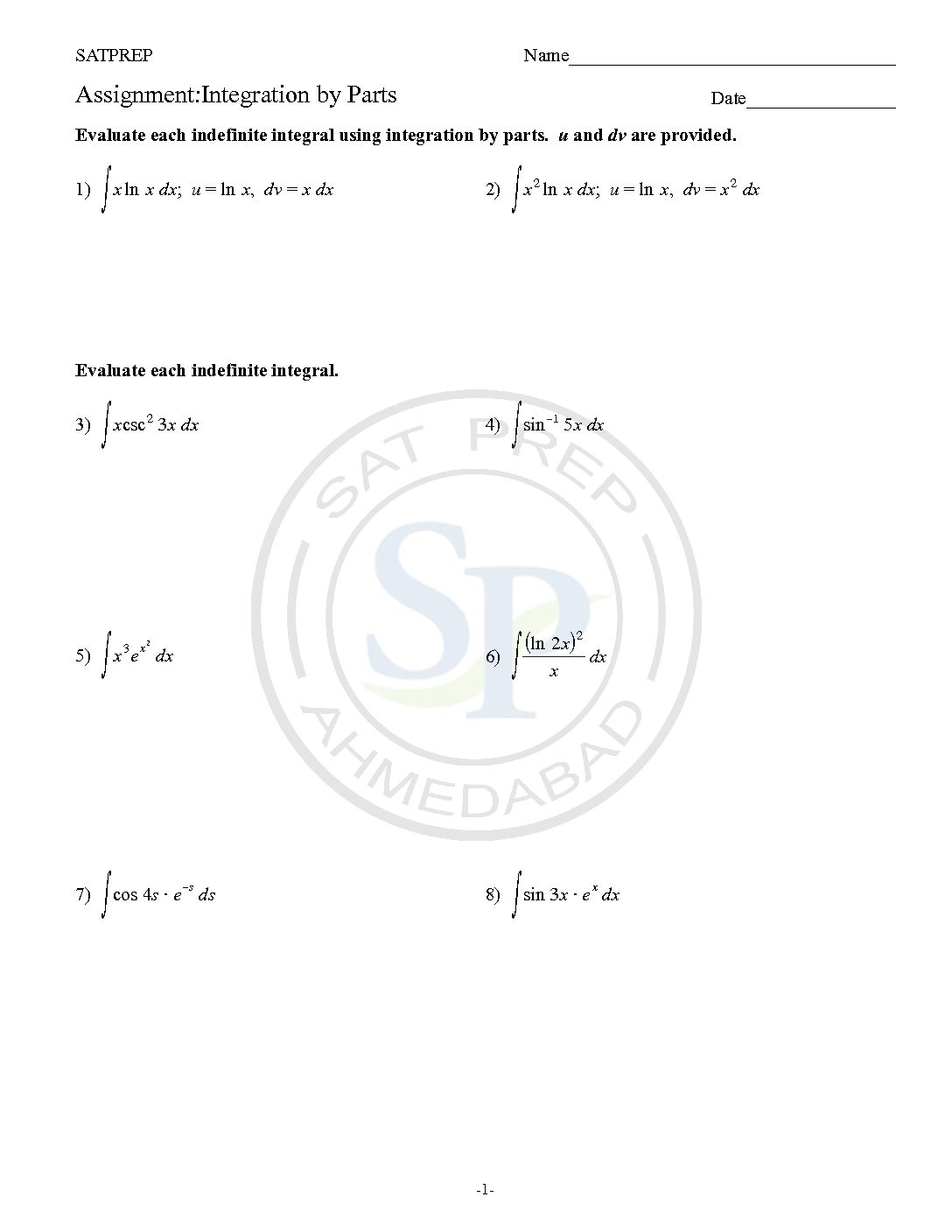
Integration by parts
Process of integration when two different functions are in product form. By part method one function get differentiate while other integrate as per formula. Integration by parts
Integration By Parts
Method of integration when two different functions are in product form. By part method one function get differentiate while other integrate as per formula. Integration by Parts
Equation of curve
Equations of curve evaluate by doing integration of derivative curve. The gradient and a point the curve passes through are given as.. Gradient: dy/dx = 6sqrt(x) Point the curve passes through: (4,1) I need to find the equation of the curve. Therefore integration is process of finding equation of the curve. Equation of curve
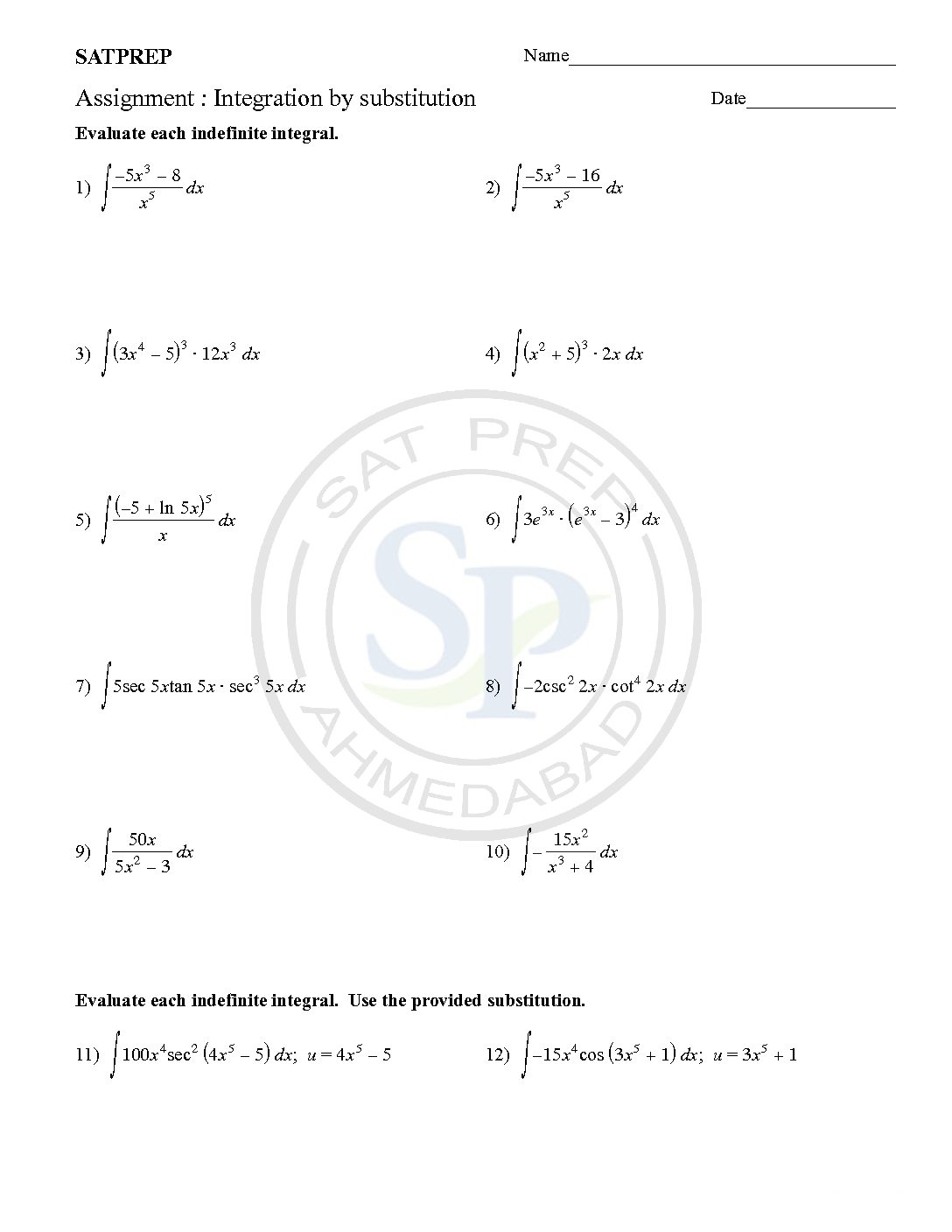
Integration by substitution
This post is about worksheet of Integration by substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by substitution
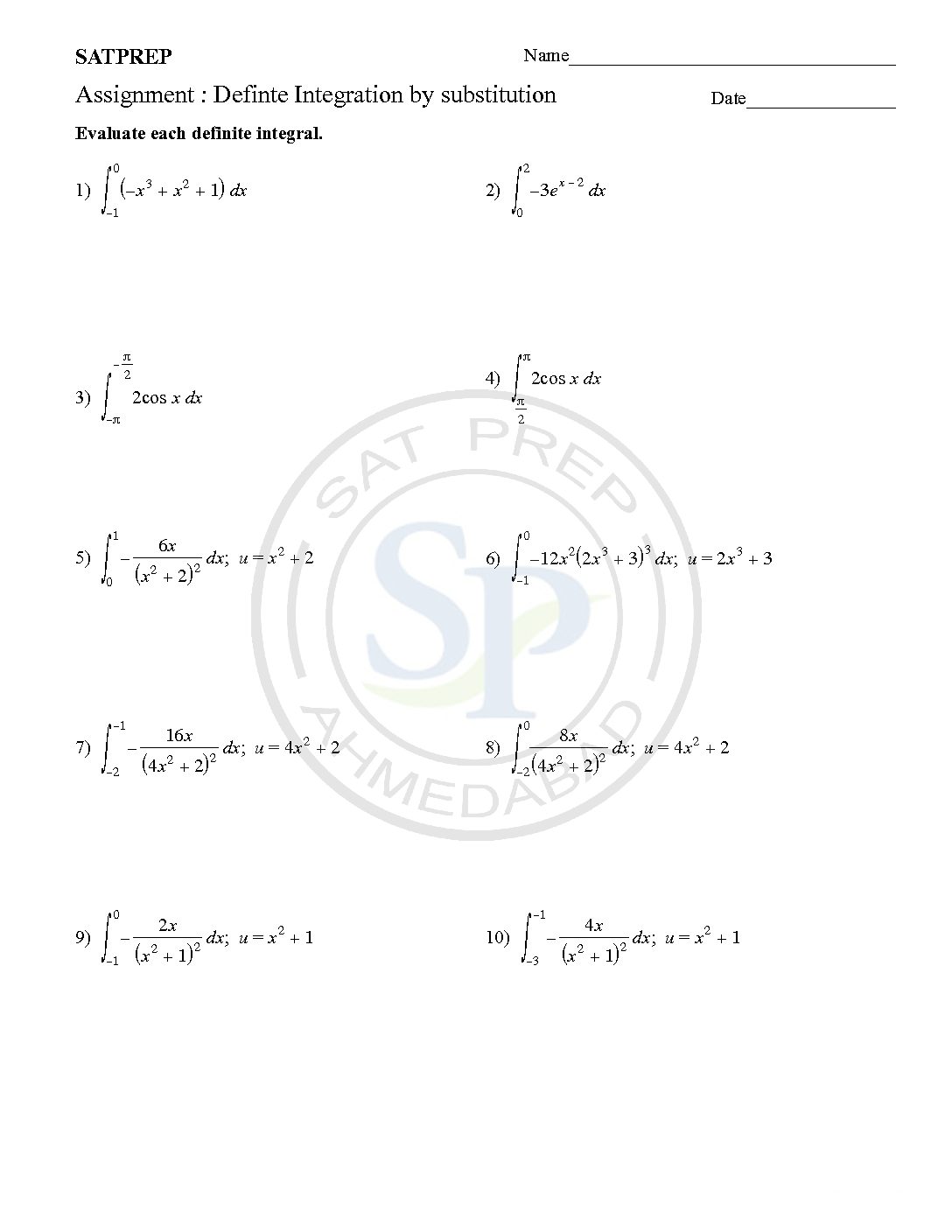
Definite integration
A Definite Integral has start and end values. In other words there is an interval [a, b]. Hence , definite integral gives particular solution. Definite Integration
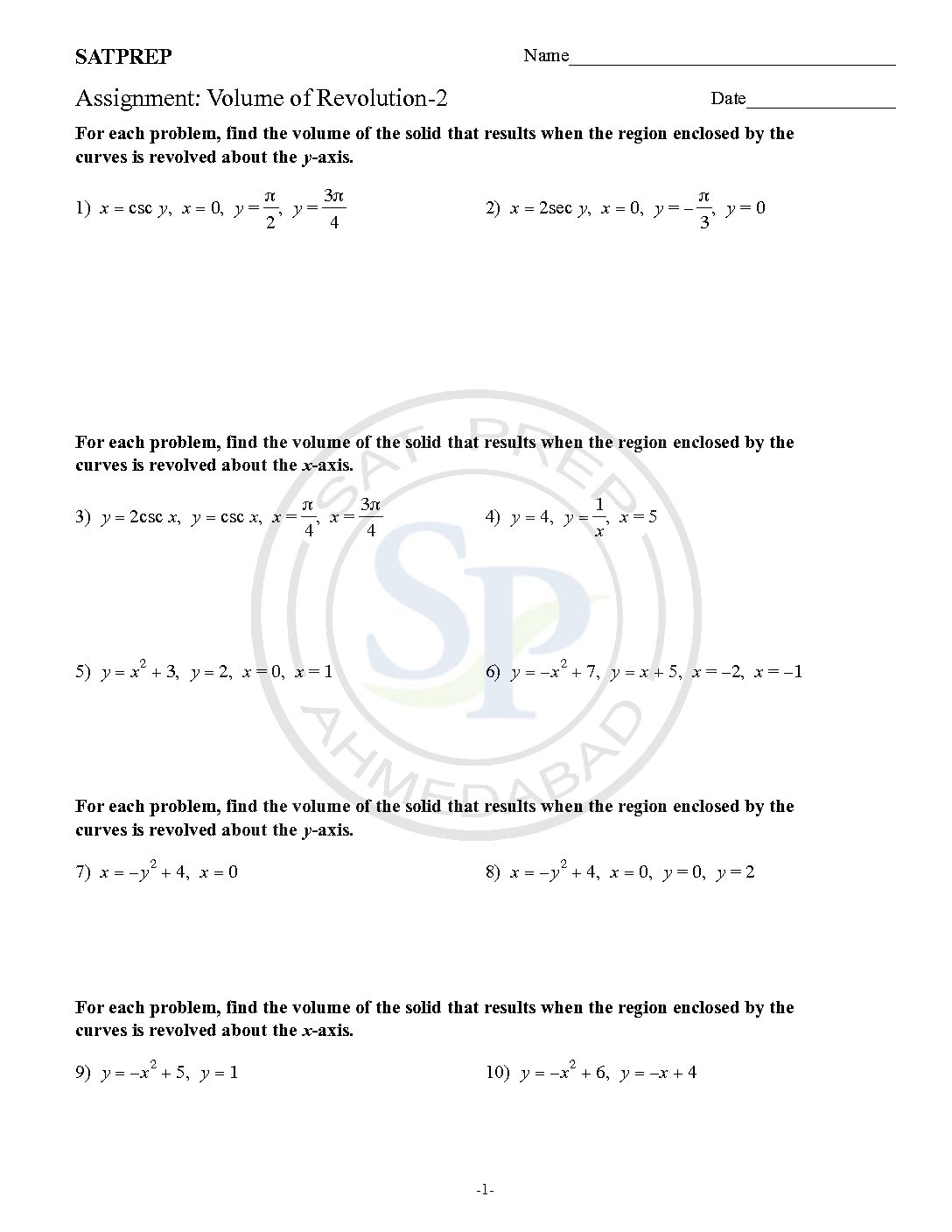
Volume of revolution of solid
Volume of revolution. To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. For purposes of this discussion let’s rotate the curve about the x -axis, although it could be any vertical […]
Volume of revolution of solid
Volume with Rings. To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. For purposes of this discussion let’s rotate the curve about the x -axis, although it could be any vertical or […]
Volume of revolution
To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution Volume of Revolution