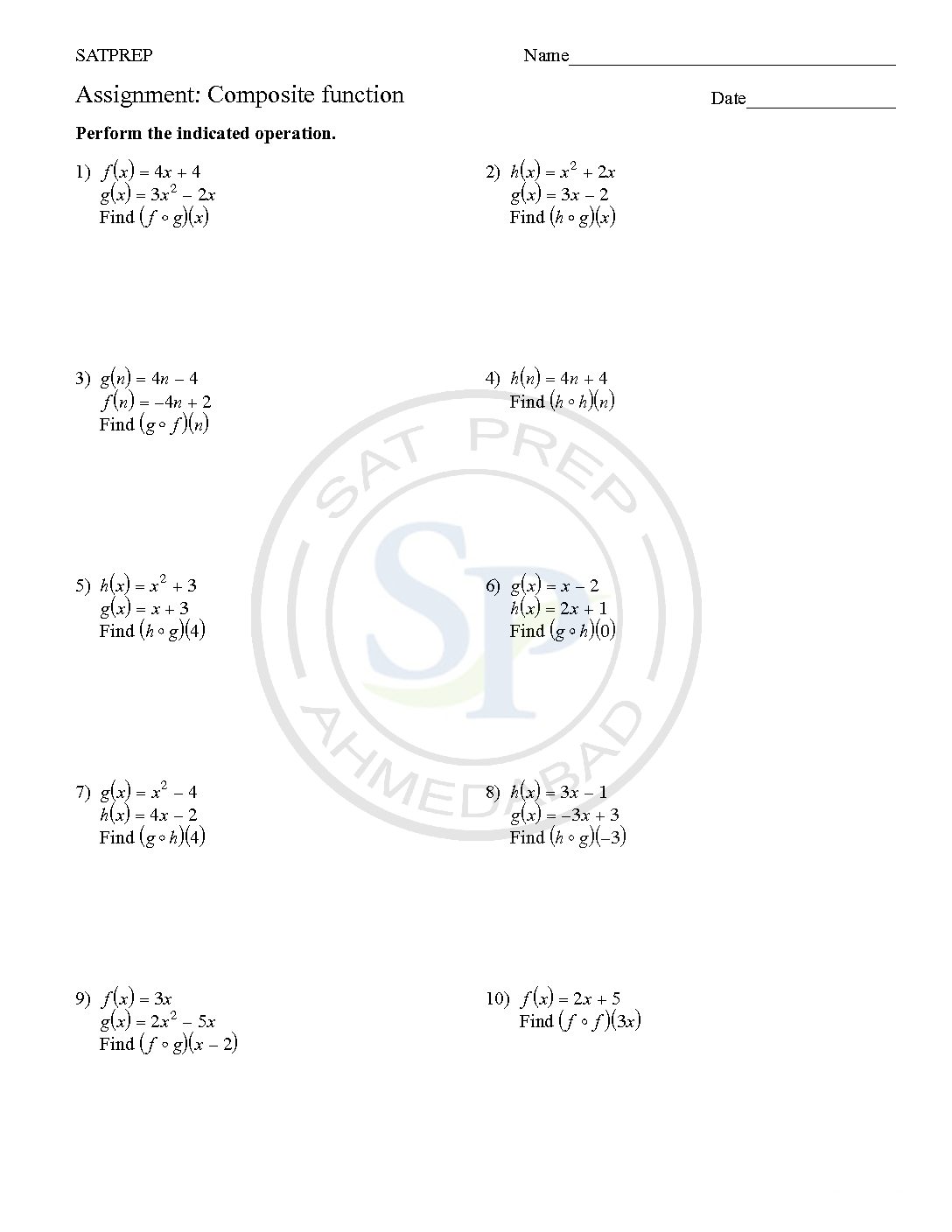
Composite functions and functional equations Composite functions Composition of two functions occurs when the output of one function becomes the input for a second function. Suppose f(x) = x2 and g(x) = 3x − 1. The composite functionf ˛g(x) = f (g(x)) Composite function
You are browsing archives for
Category: AP
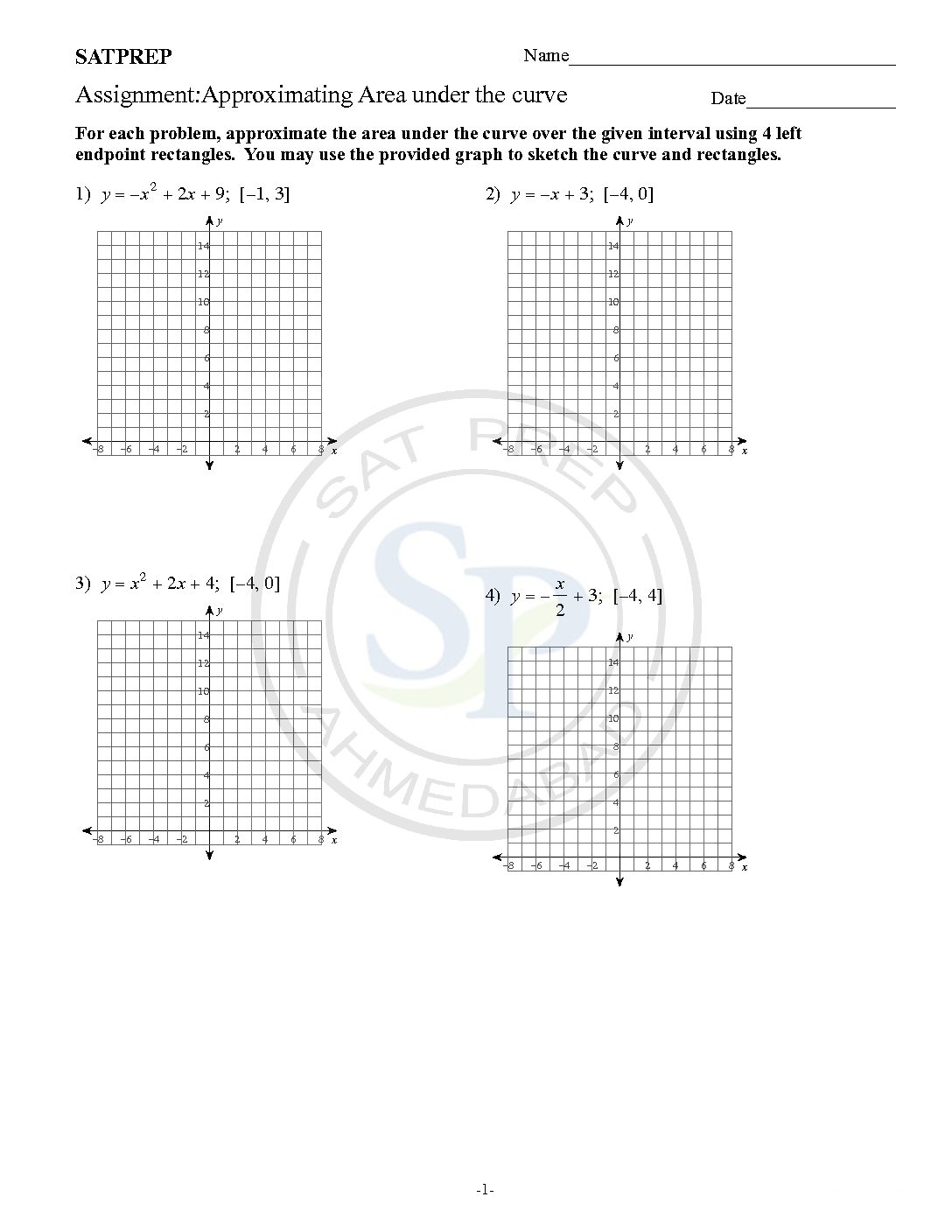
Approximating area under the curve
Approximate area of under a curve. Compute left, right, and midpoint Hence Riemann sums use with n rectangles are computed. Due to the this it approximate area. Approximate area under
Riemann sum( table)
A Riemann sums is an approximation of a region’s area. It obtained by adding up the areas of multiple simplified slices of the region. It is applied in calculus to determine the area of a region. Hence it give approximate area of region. It is also give right and left sum. Riemann sum
Composite Function
Composite functions are defined as one function defined inside another function. Like ff(x)) or f(g(x)) etc. Another function composition is an operation that takes two functions f and g and produces a function h such that h(x) = g(f(x)) Composite function
Point of inflection
Inflection Point. An inflection point is a point on a curve at which the sign of the curvature (i.e., the concavity) changes. Inflection points may be stationary points, but are not local maxima or local minima. For example, for the curve plotted above, the point is an inflection point. Point of infection
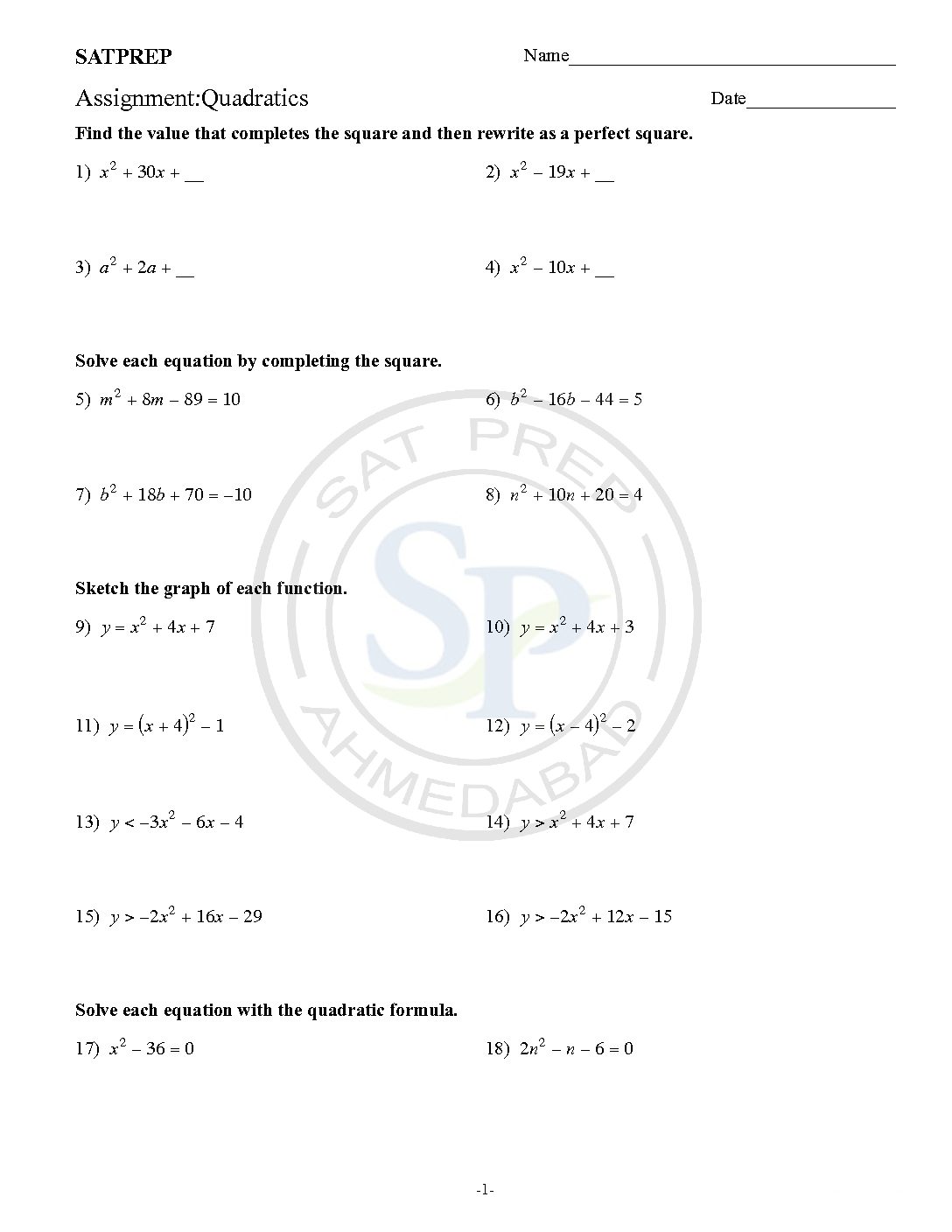
Quadratics
First of all this post consists of questions of factorisation of quadratics . The method use to find solutions are splitting middle term and another method is formula . We also find the nature of roots of equation. The method is use to find nature is discriminant (D= b2-4ac). Discriminant is also used in formula […]
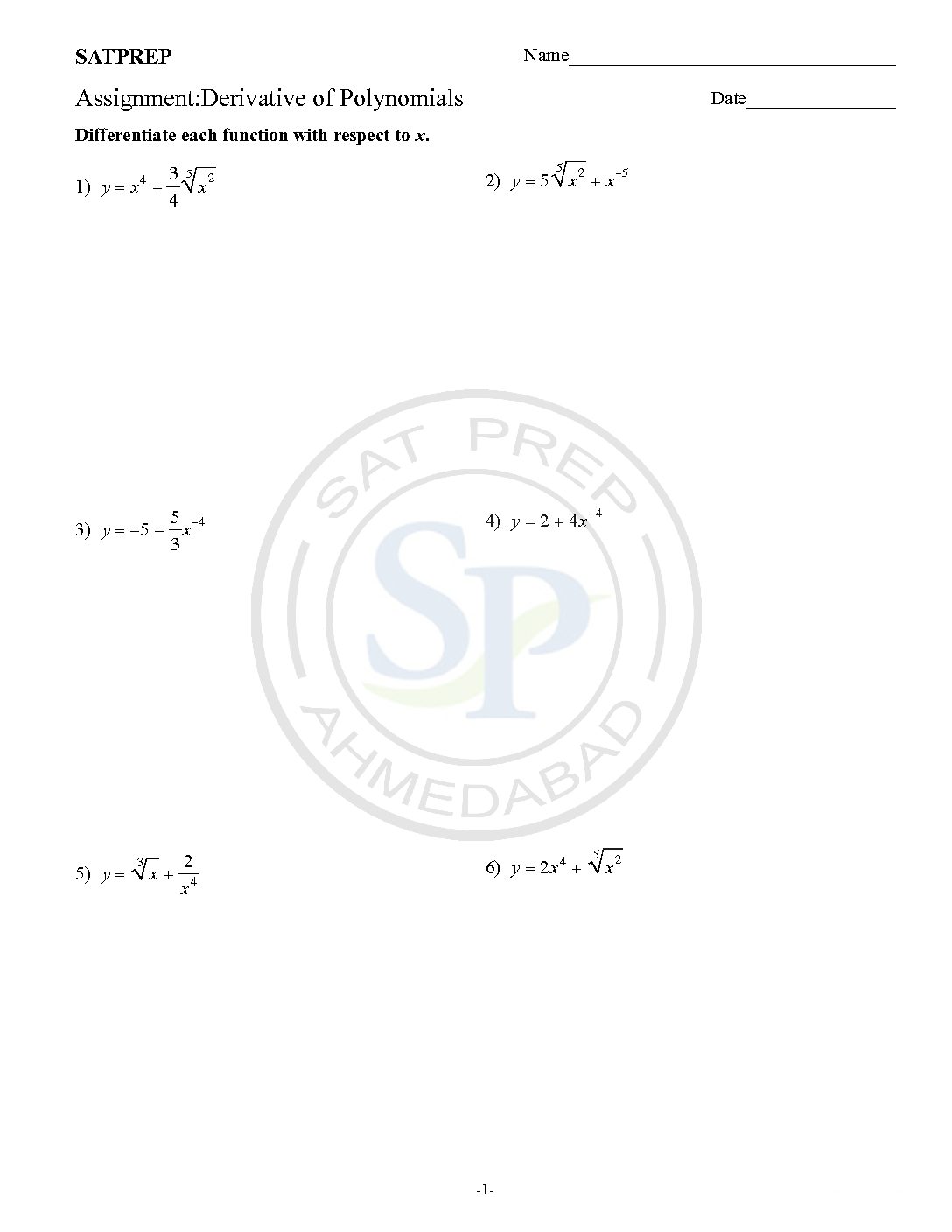
Differentiation of polynomial
Differentiation is process of getting derivative. Differentiation has applications to nearly all quantitative disciplines. For example, in physics, the derivative of the displacement of a moving body with respect to time is the velocity of the body, and the derivative of velocity with respect to time is acceleration. Similarly in chemistry as well as Economics also derivative
Formulae of Calculus
List of Calculus Formulas-basic Properties and Formulas of Integration : If f (x) and g(x) are differentiable functions . Another In basic calculus, we learn rules and formulas for differentiation, which is the method by which we calculate the derivative of a function, and integration, Differential Calculus that is concerning rates of change and slopes of curves, and Integral Calculus concerning […]
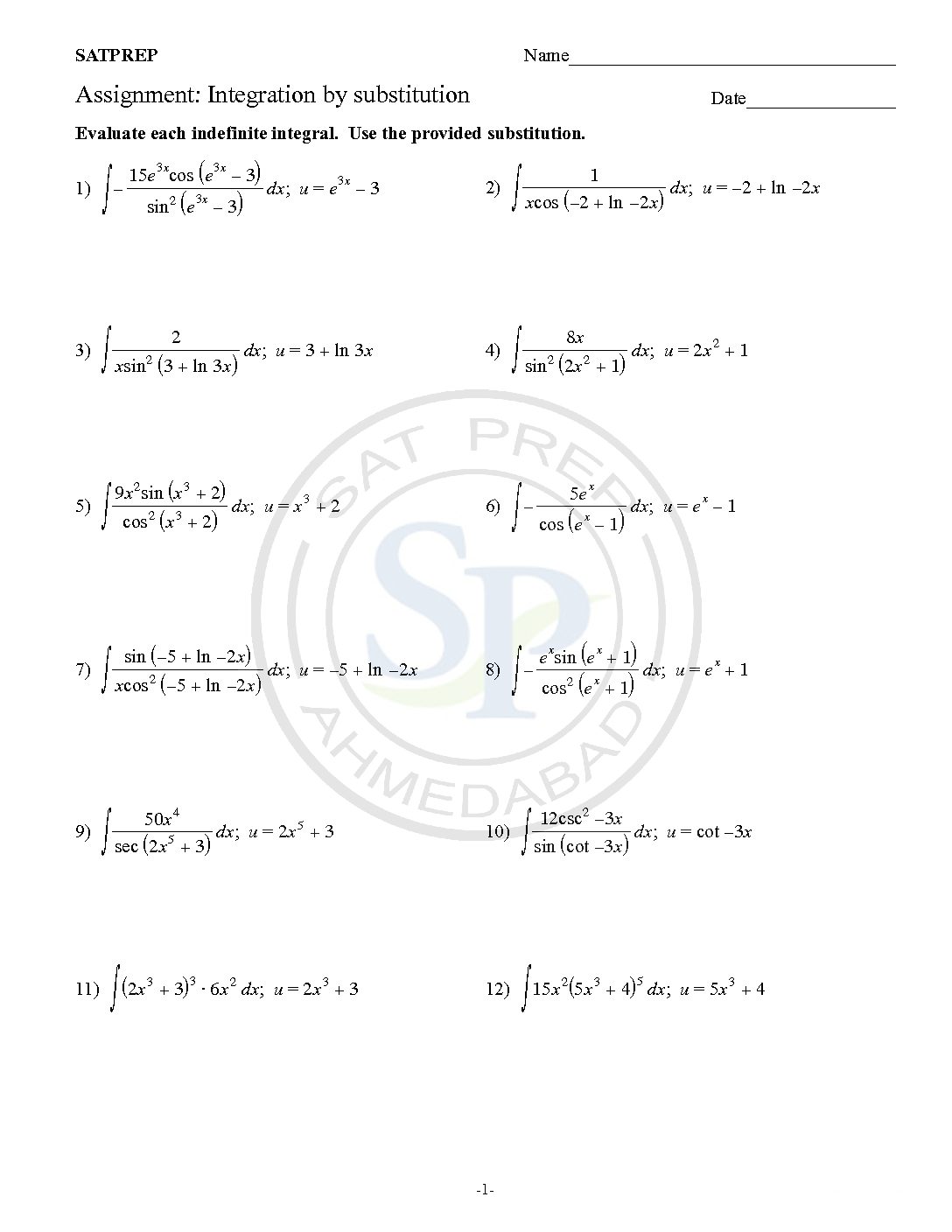
Integration by Substitution-5
This post is about worksheet of integration by substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by substitution
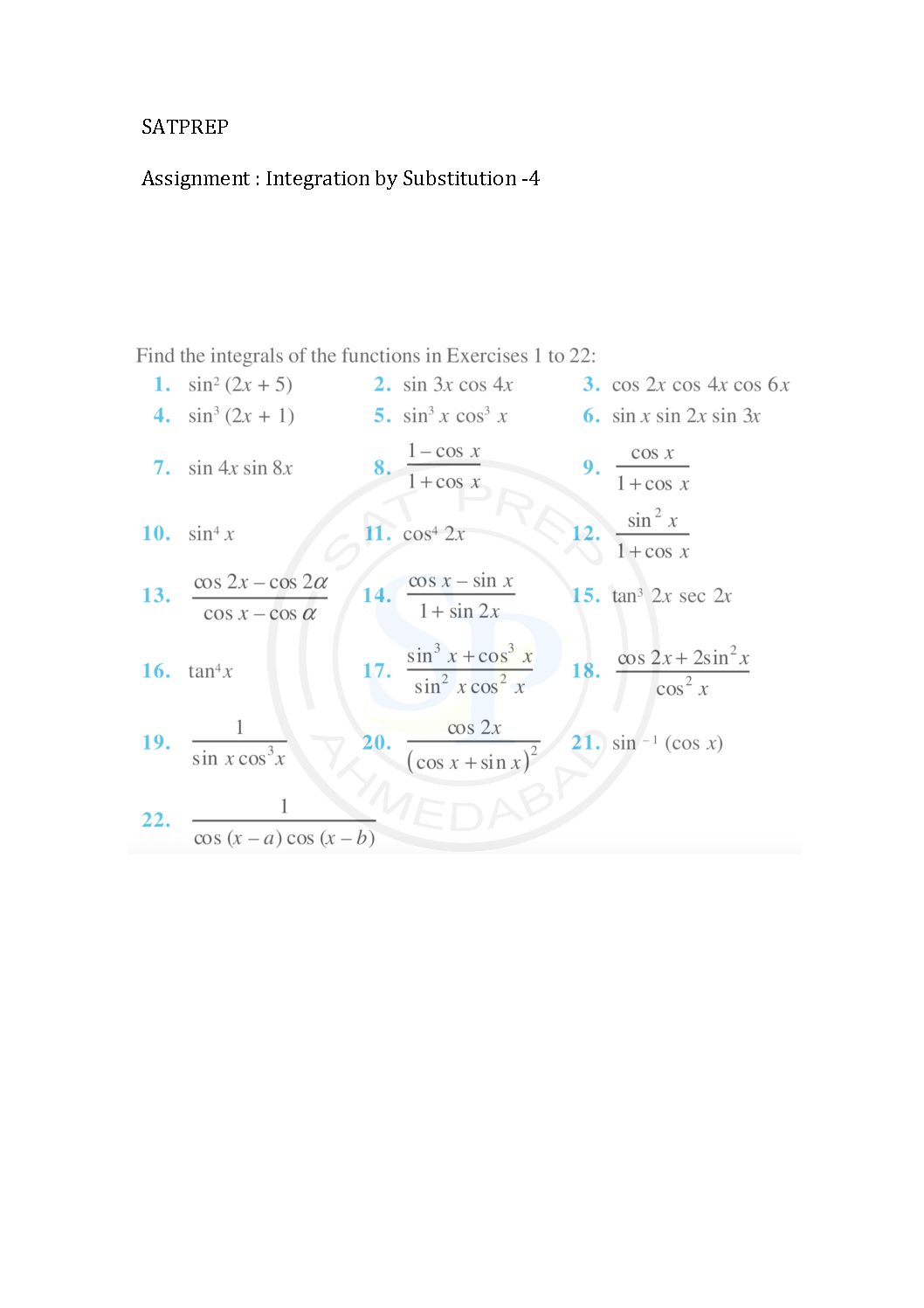
Integration by Substitution-4
This post is about worksheet of integration by substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] integration by substitution