You are browsing archives for
Category: Integration
Topic wise A-Level Pure Math Past Paper ...
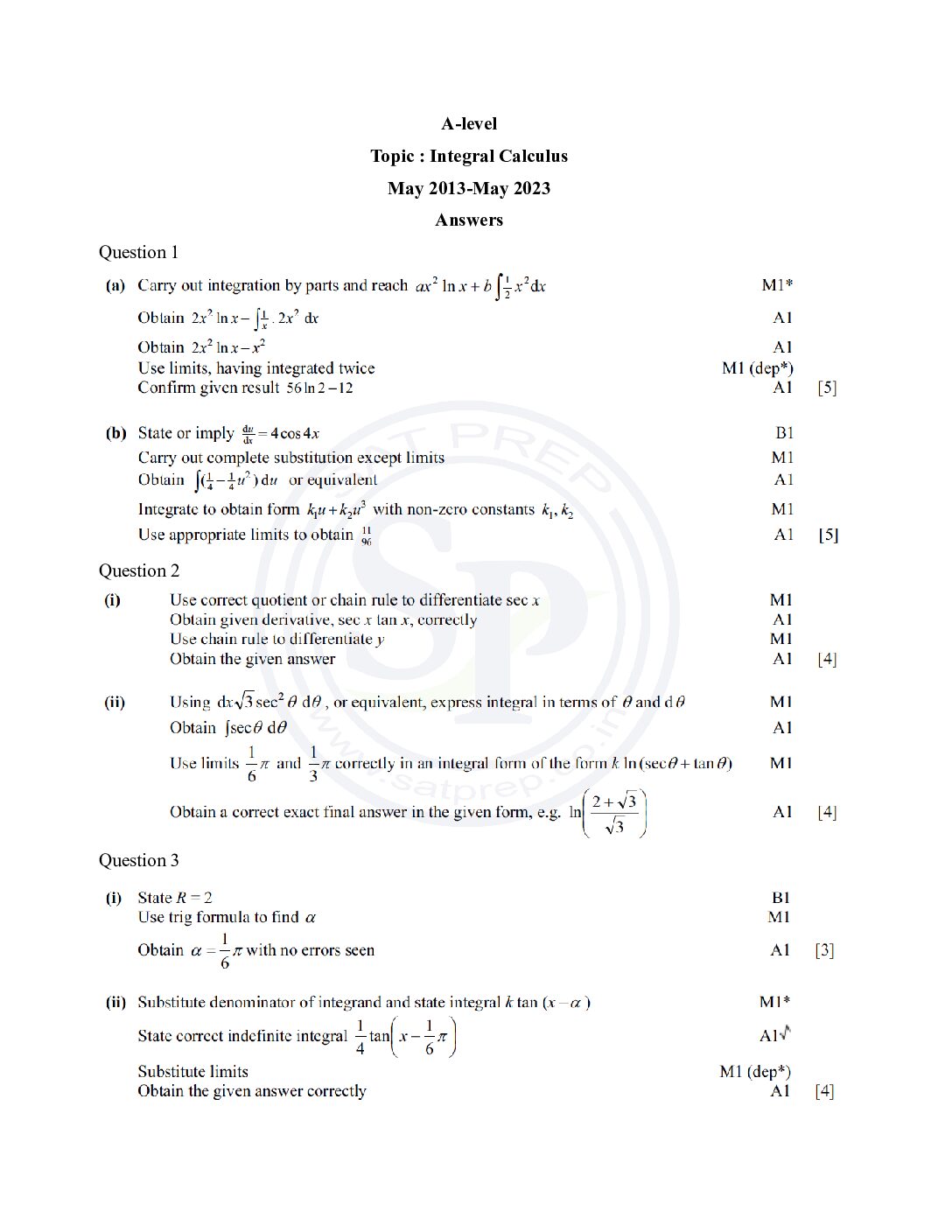
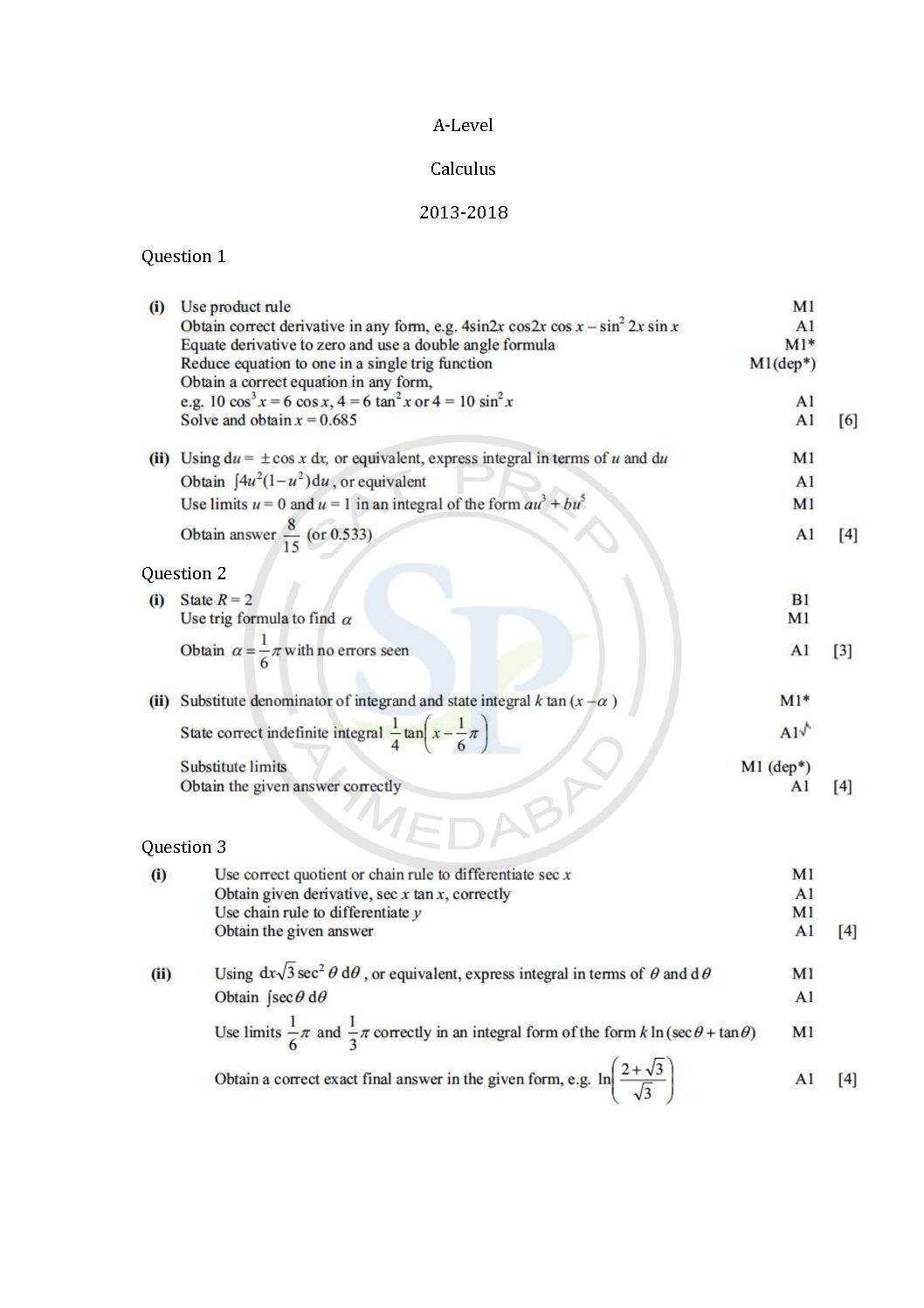
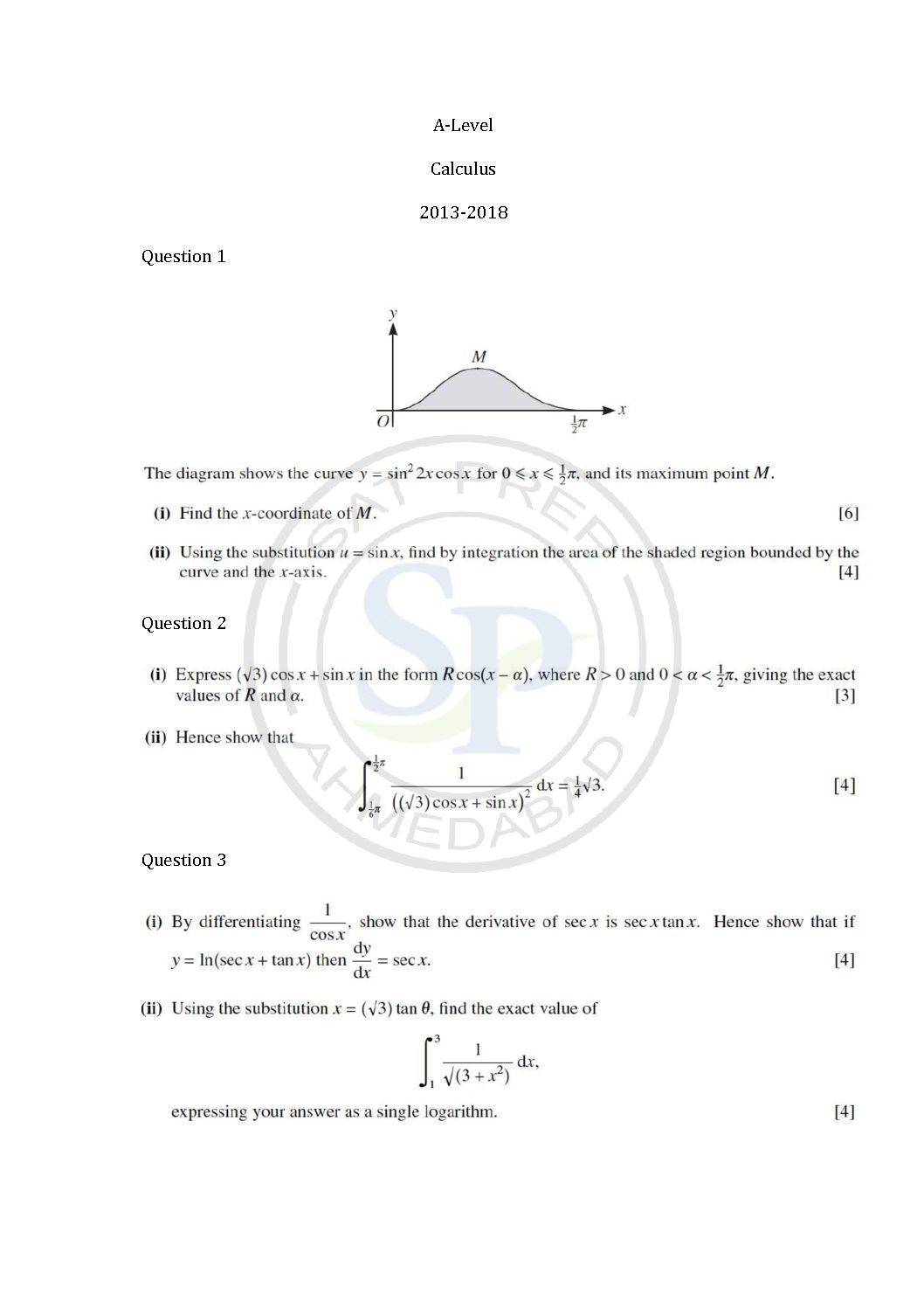
Topic wise A-Level Math Past Paper Cal...
This post is about solution of question of calculus from AS-level Math past paper. In this post questions are from different paper from May 2013 to Nov 2017. By this post students will come to know variety of questions asked in previous year papers. The questions type in this post is calculator . www.cie.org.uk
Topic wise A-Level Math Past Paper Ca...
This post is about question of calculus from A-level Math past paper. In this post questions are from different paper from May 2013 to Nov 2017. By this post students will come to know variety of questions asked in previous year papers. The questions type in this post is calculator . www.cie.org.uk
Equation of curve
Equations of curve evaluate by doing integration of derivative curve. The gradient and a point the curve passes through are given as.. Gradient: dy/dx = 6sqrt(x) Point the curve passes through: (4,1) I need to find the equation of the curve. Therefore integration is process of finding equation of the curve. Equation of curve
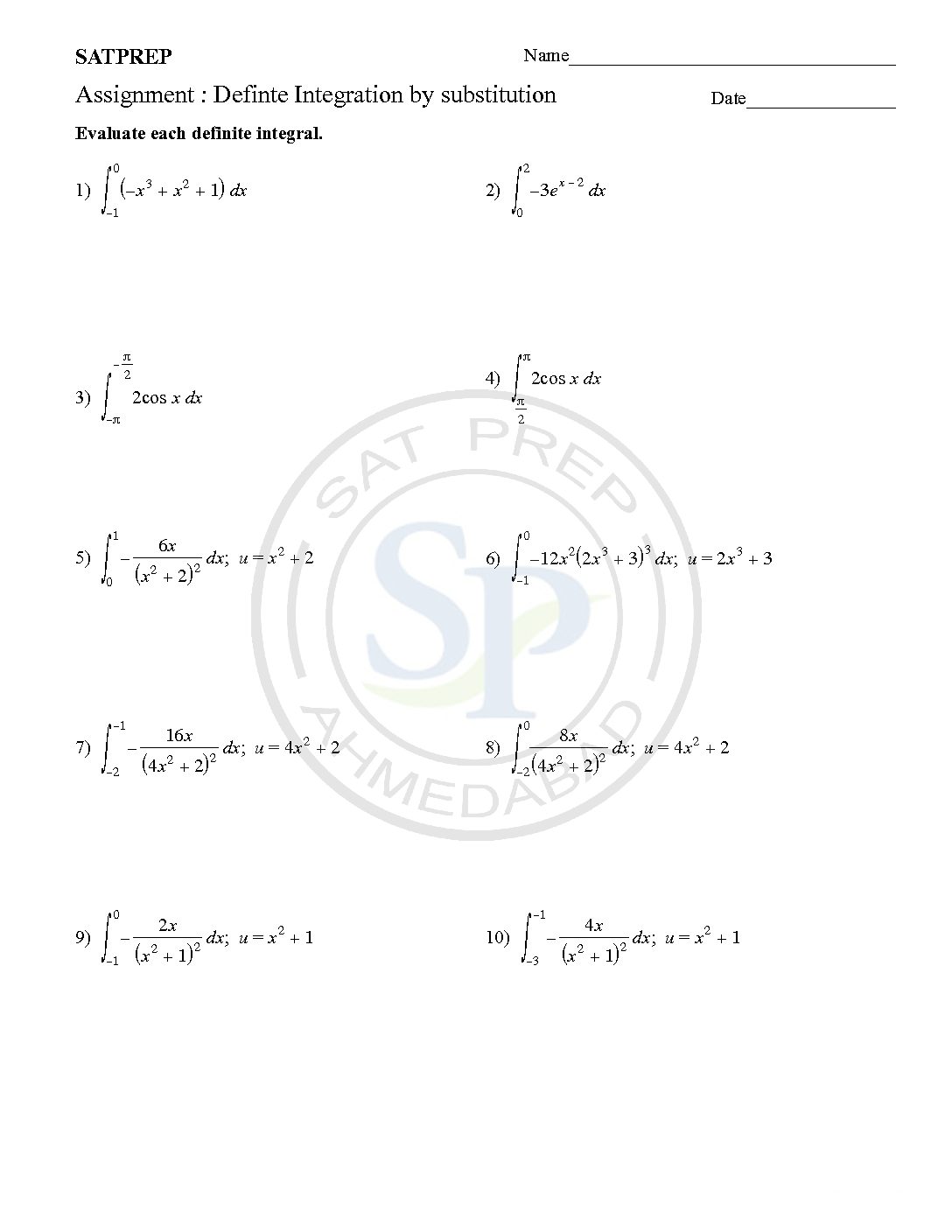
Definite integration
A Definite Integral has start and end values. In other words there is an interval [a, b]. Hence , definite integral gives particular solution. Definite Integration
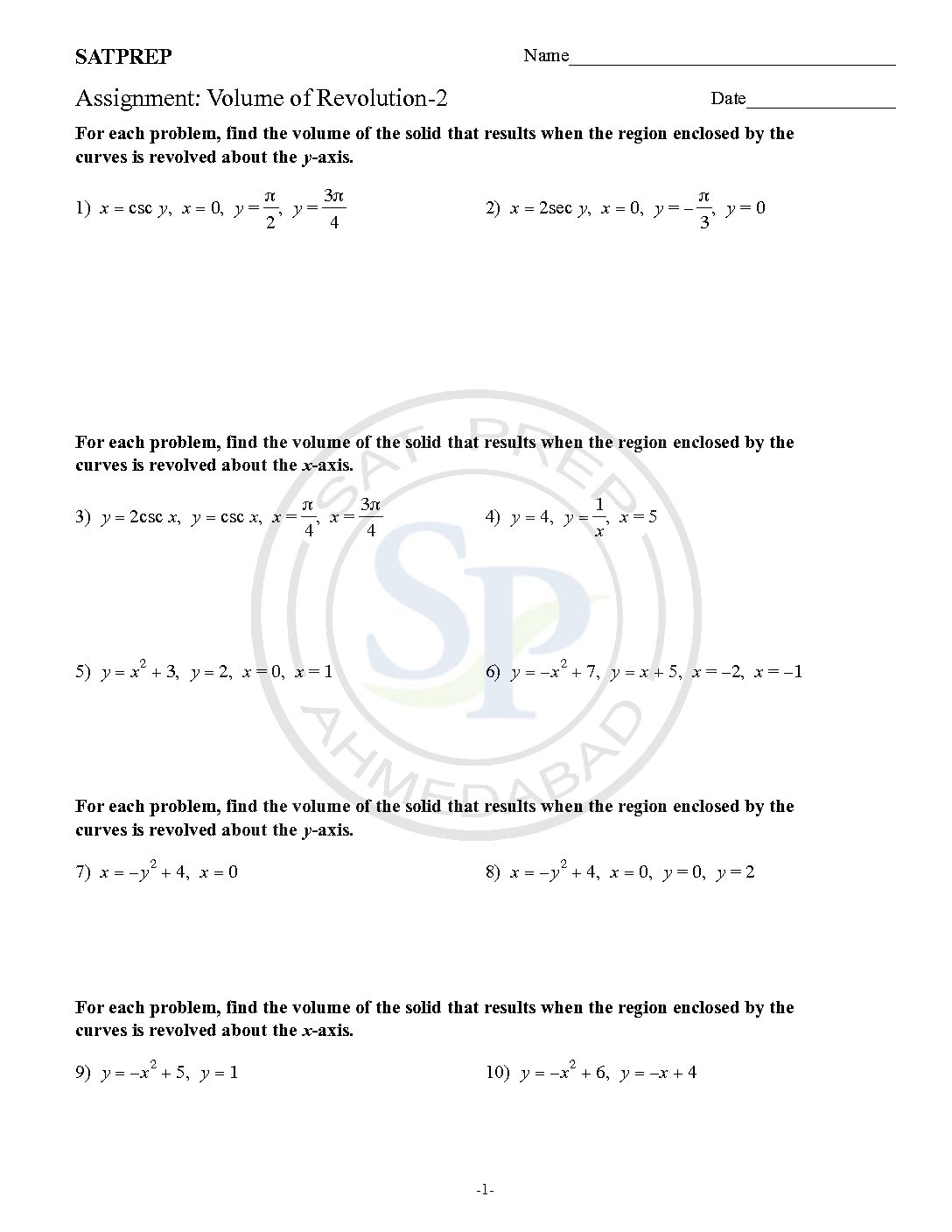
Volume of revolution of solid
Volume of revolution. To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. For purposes of this discussion let’s rotate the curve about the x -axis, although it could be any vertical […]
Volume of revolution
To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution Volume of Revolution
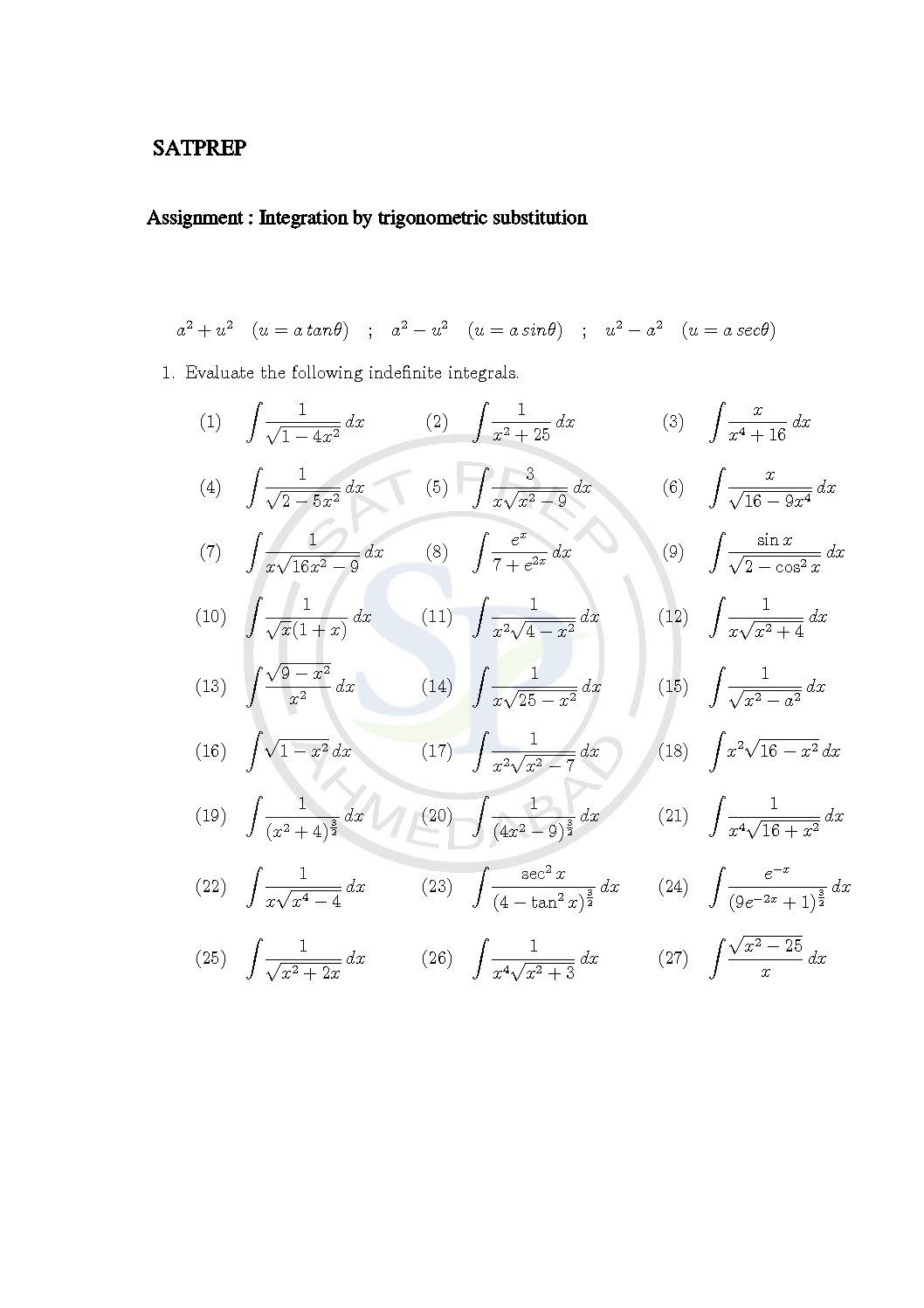
Integration by trigonometric substitutio...
This post is about worksheet of Integration by trigonometric substitution. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by substitution
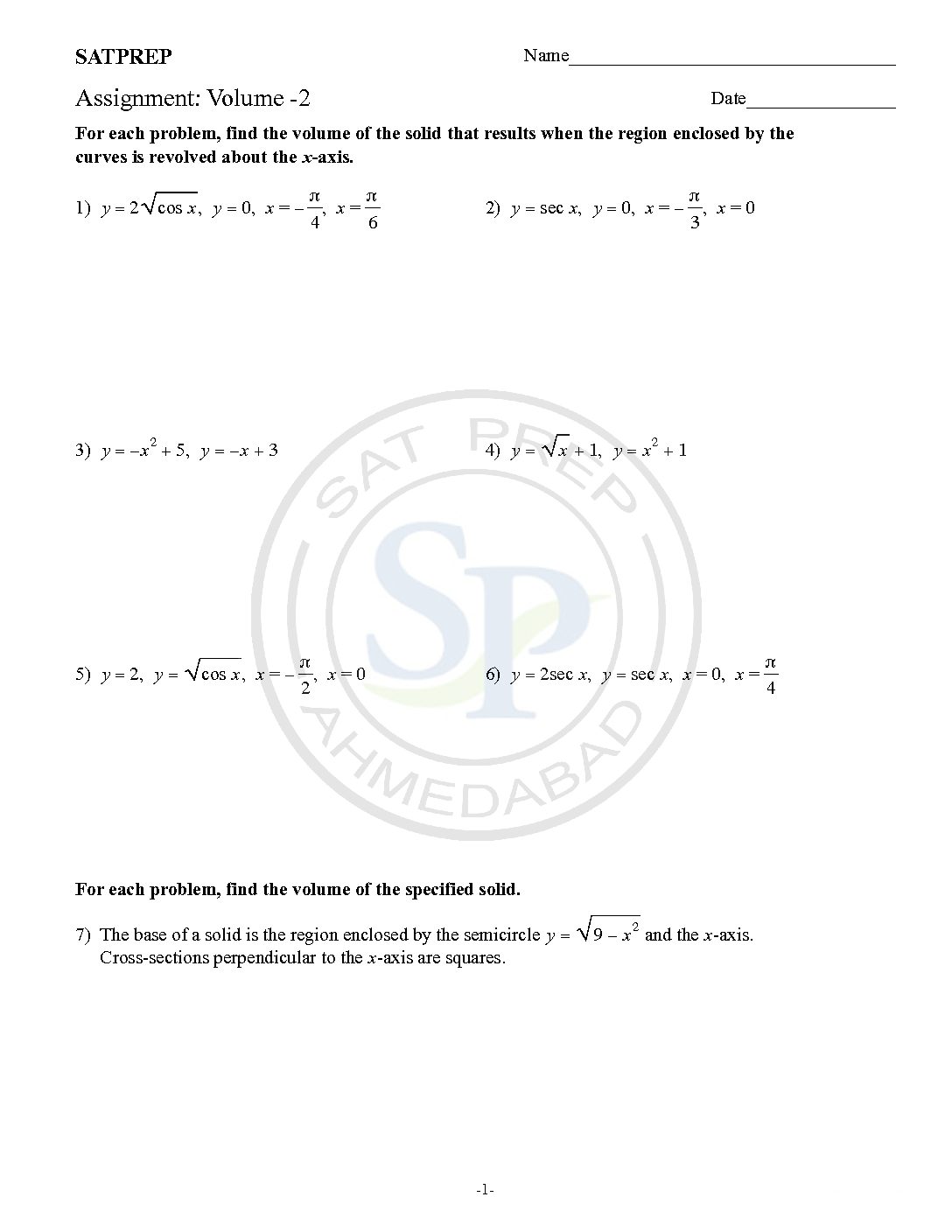
Volume -2
To get a solids of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. Volume