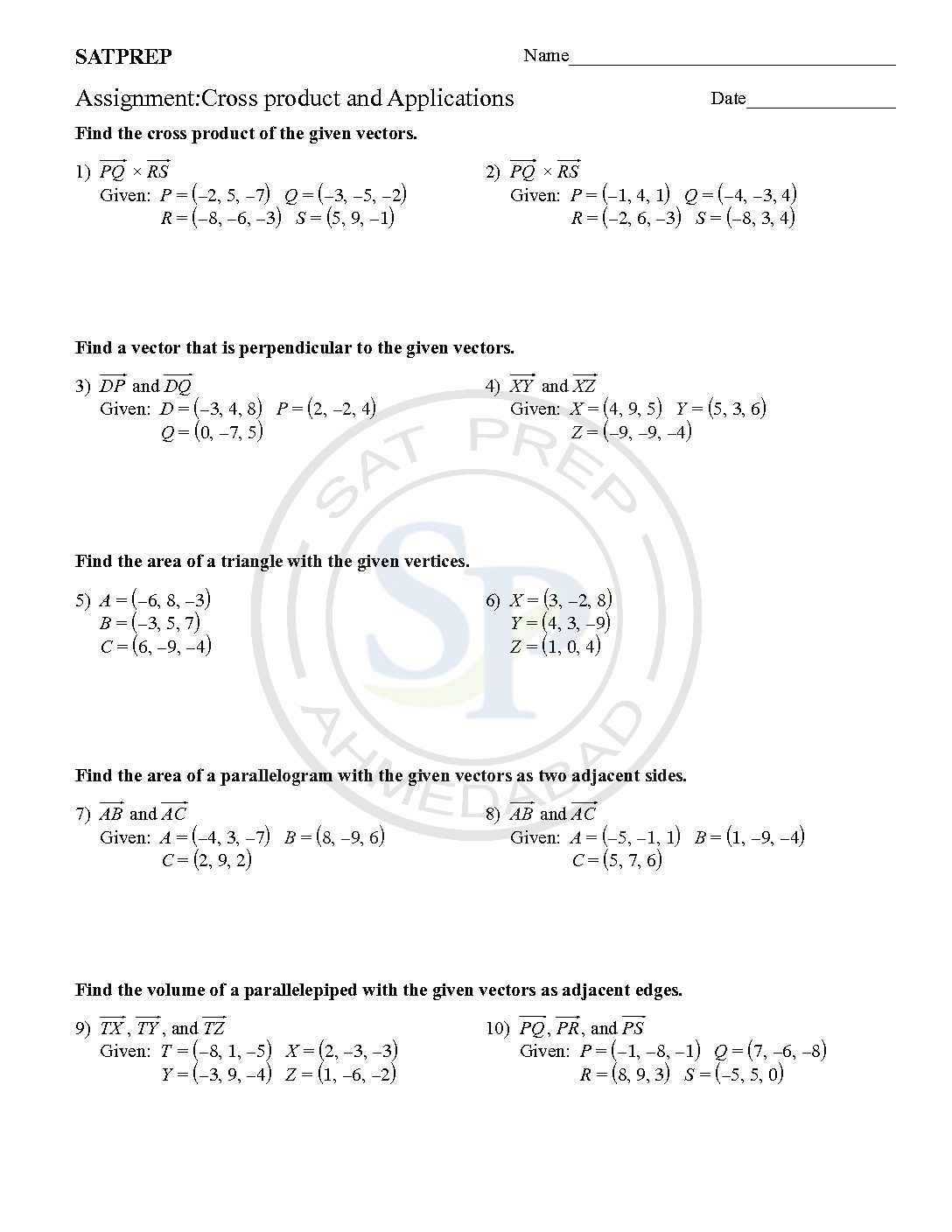
Cross Product vs Dot Product . The significant difference between finding a dot product and cross product is the result. The dot product of any two vectors is a number (scalar), whereas the cross product of any two vectors is a vector. Cross product and application
You are browsing archives for
Category: Pure Maths
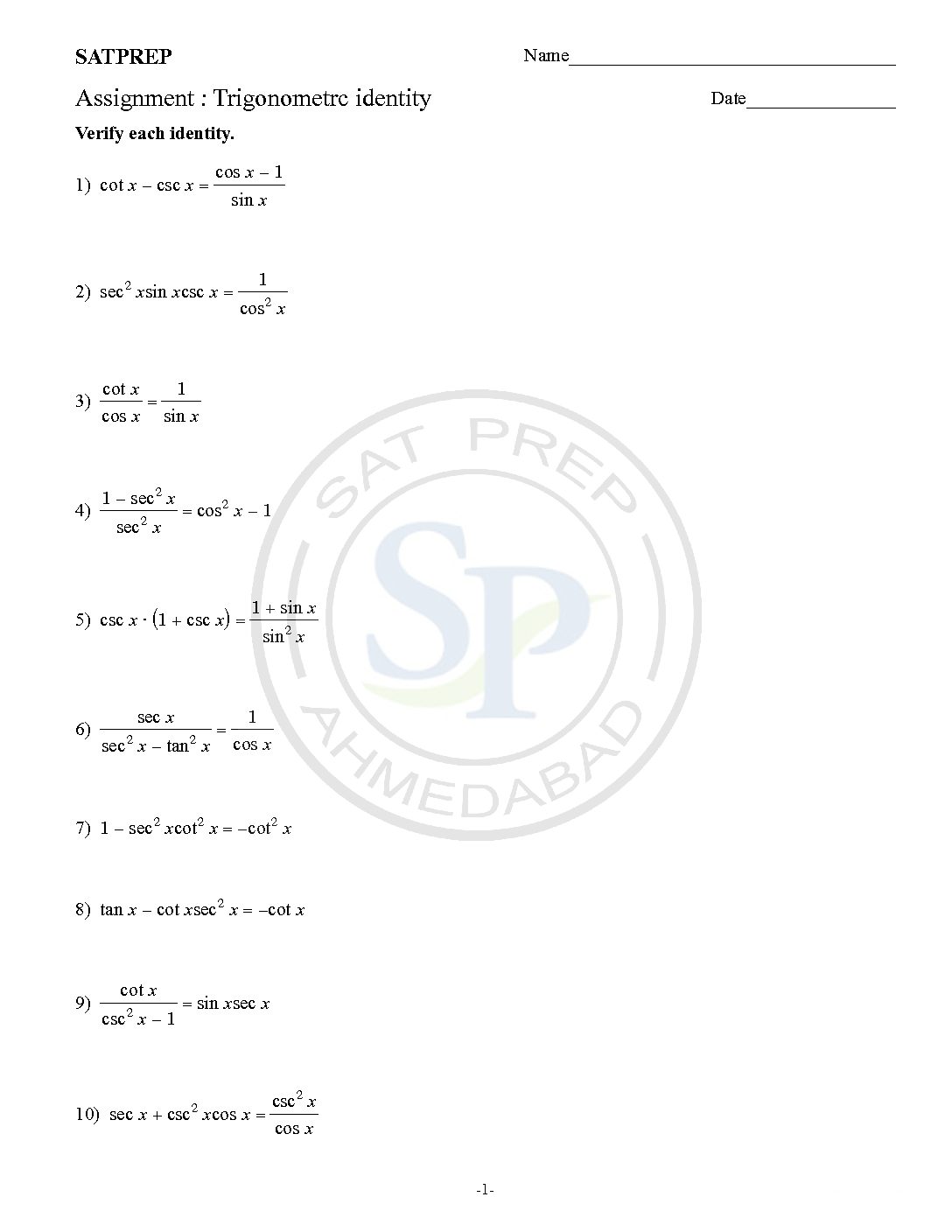
Trigonometric identity
Trig identity is equations that are true for Right Angled Triangles. D1: sin 2A+ cos 2A=1 D2: 1+ tan 2A= sec 2A D3: 1+ cot 2A= csc 2A Hence trigonometric identities are prove by above D1,D2 and D3. Similarly also using reciprocal identity. Trigonometric Identity
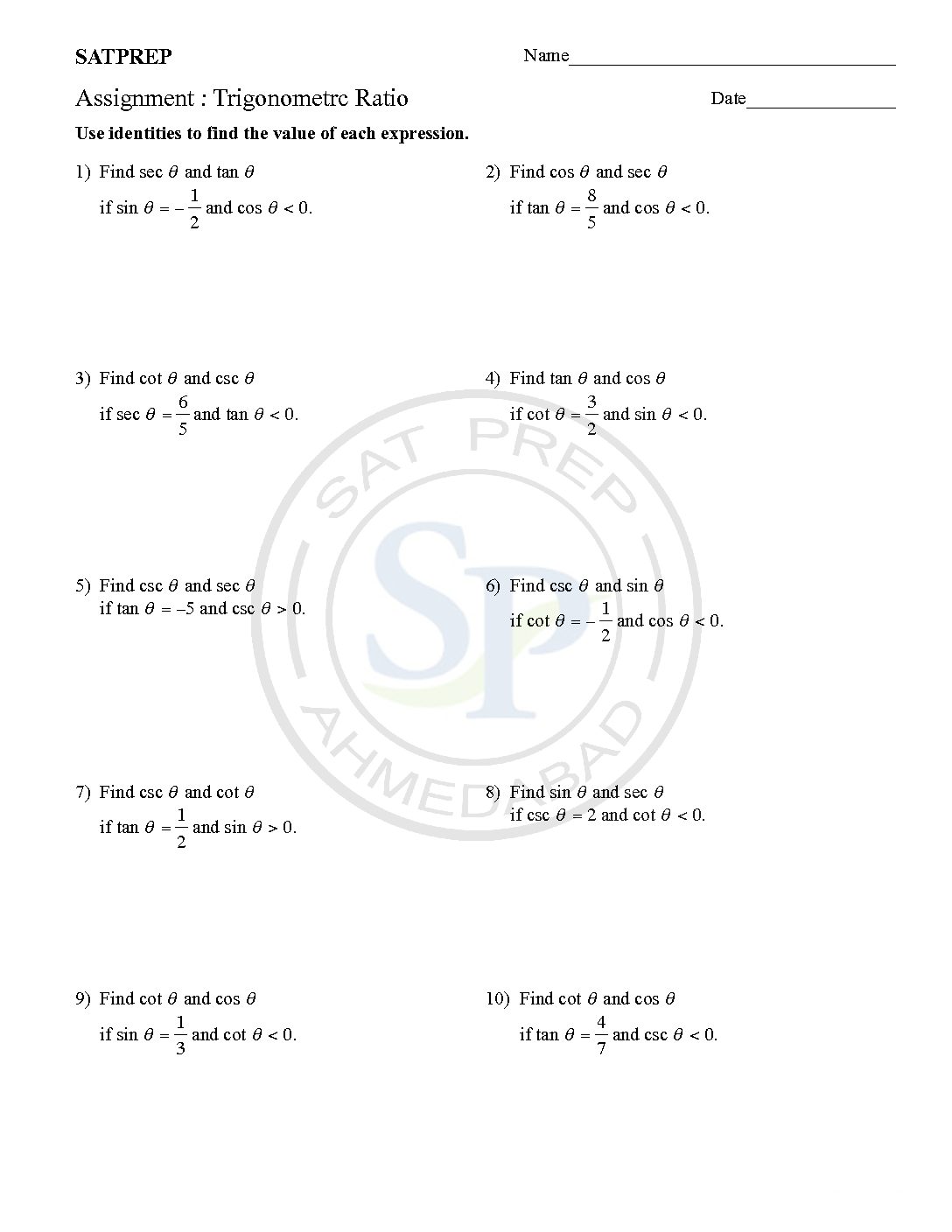
Trigonometric ratio-2
The ratios of the sides of a right triangle are called trigonometric ratios. Sine, Cosine and Tangent are main ratio while rest three reciprocal. Hence Sine and Cosine are the trigonometric ratios, whose values are less that 1 for an acute angle. Because they are periodic. www.kutasoftware.com
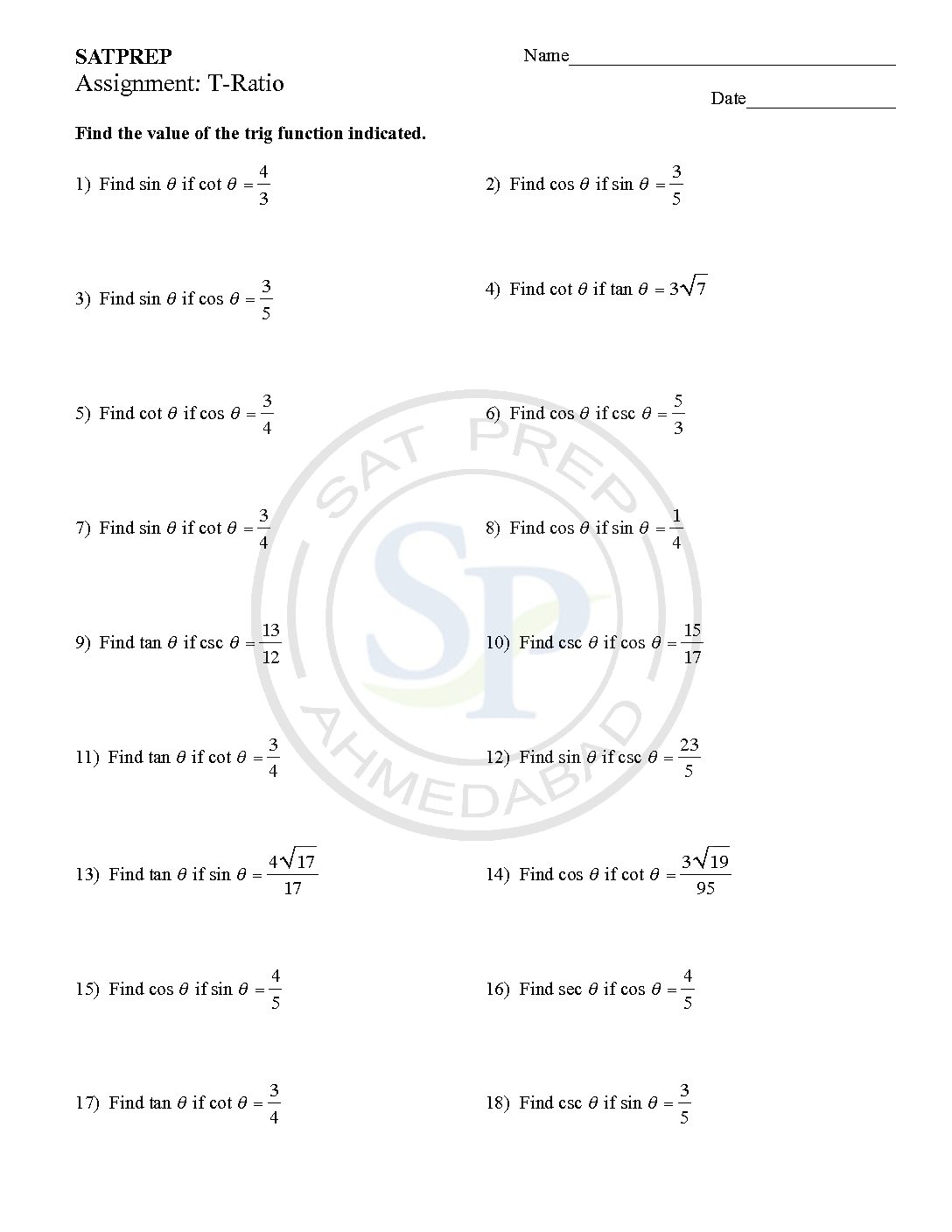
Trigonometric ratio
The ratios of the sides of a right triangle are called trigonometric ratios. Sine, Cosine and Tangent are main ratio while rest three reciprocal. Hence Sine and Cosine are the trigonometric ratios, whose values are less that 1 for an acute angle. Because they are periodic. Trigonometric ratio
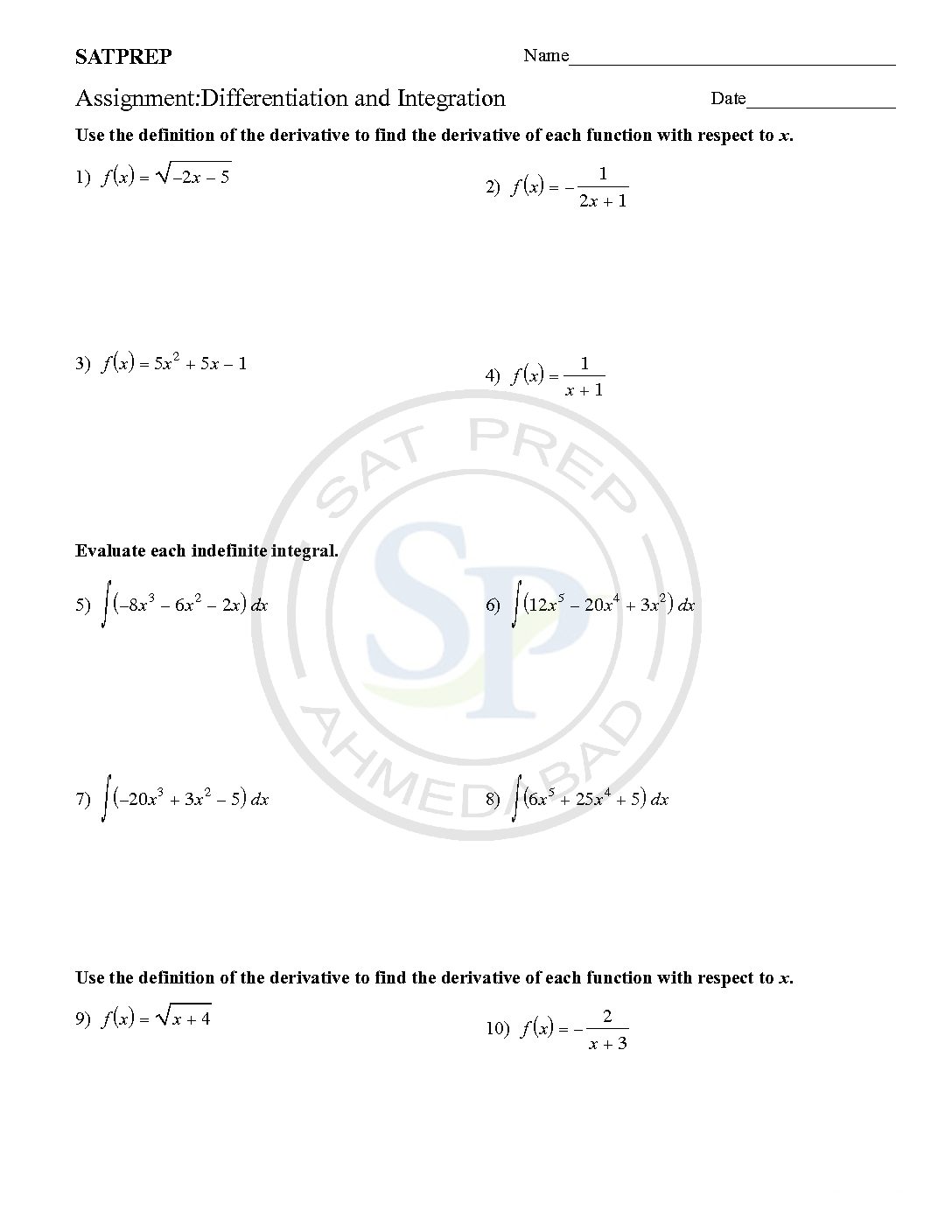
Differentiation and Integration
First of all differentiation and Integration are process of calculus. Due to differentiation we get derivative, while integration of derivative we get function back. Integration also called derivative. Differentiation and Integration
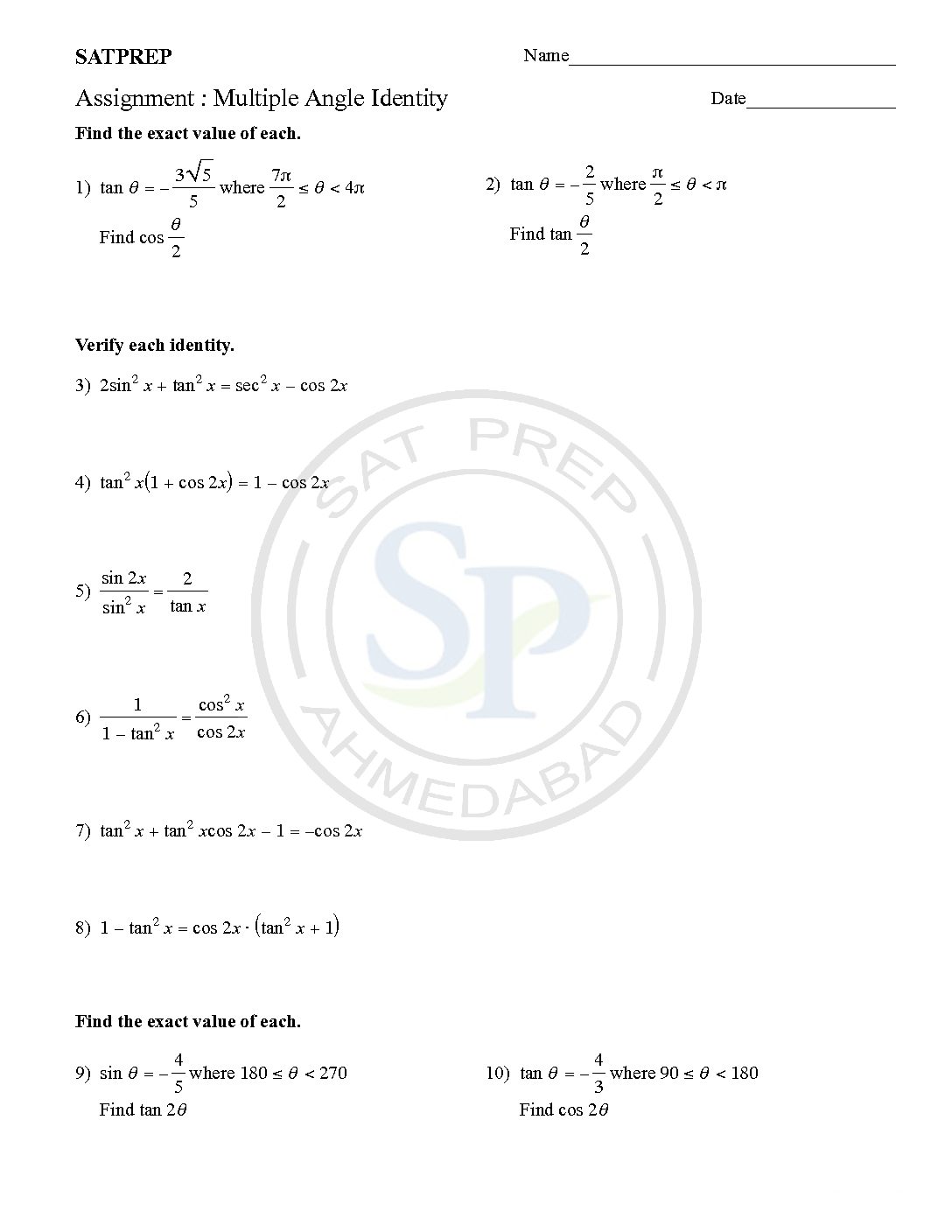
Multiple angle identity
Multiple angles identity are nothing but the trigonometric identity of multiple angles. Use for Proof of the double-angle and half-angle formulas. Solving Trigonometric Equations and Identities using Double–Angle and Half- Angle Formulas, examples and step by step solutions. Hence double angle formulae for sin 2A, cos 2A and tan 2A use for solving identity. Also to solve […]
Tangent and normal : Implicit
Tangent and Normal Lines for implicit curves are a line that touches the implicit curve at one point and has the same slope as the curve at that point. A normal to a curve is a line perpendicular to a tangent to the curve. tangent and normal
Trapezoidal Rule-2
trapezium rules is a way of estimating the area under a curve. so the trapezium rule gives a method of estimating integrals. Due to this we get approximate answer . Also we can get area using integration. trapezium rule
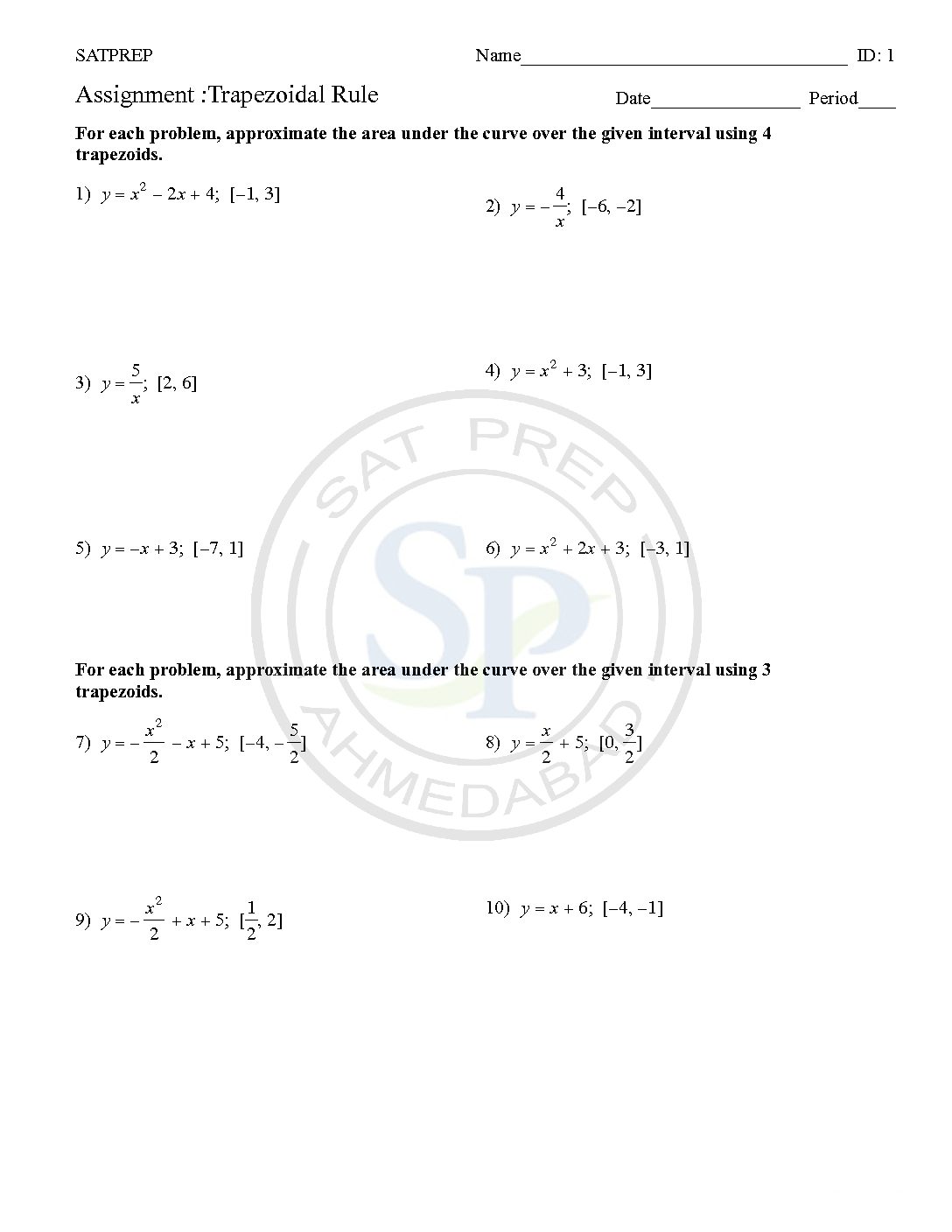
Trapezoidal Rule
The trapezium rules is a way of estimating the area under a curve. so the trapezium rule gives a method of estimating integrals. Due to this we get approximate answer . Also we can get area using integration. trapezium Rule
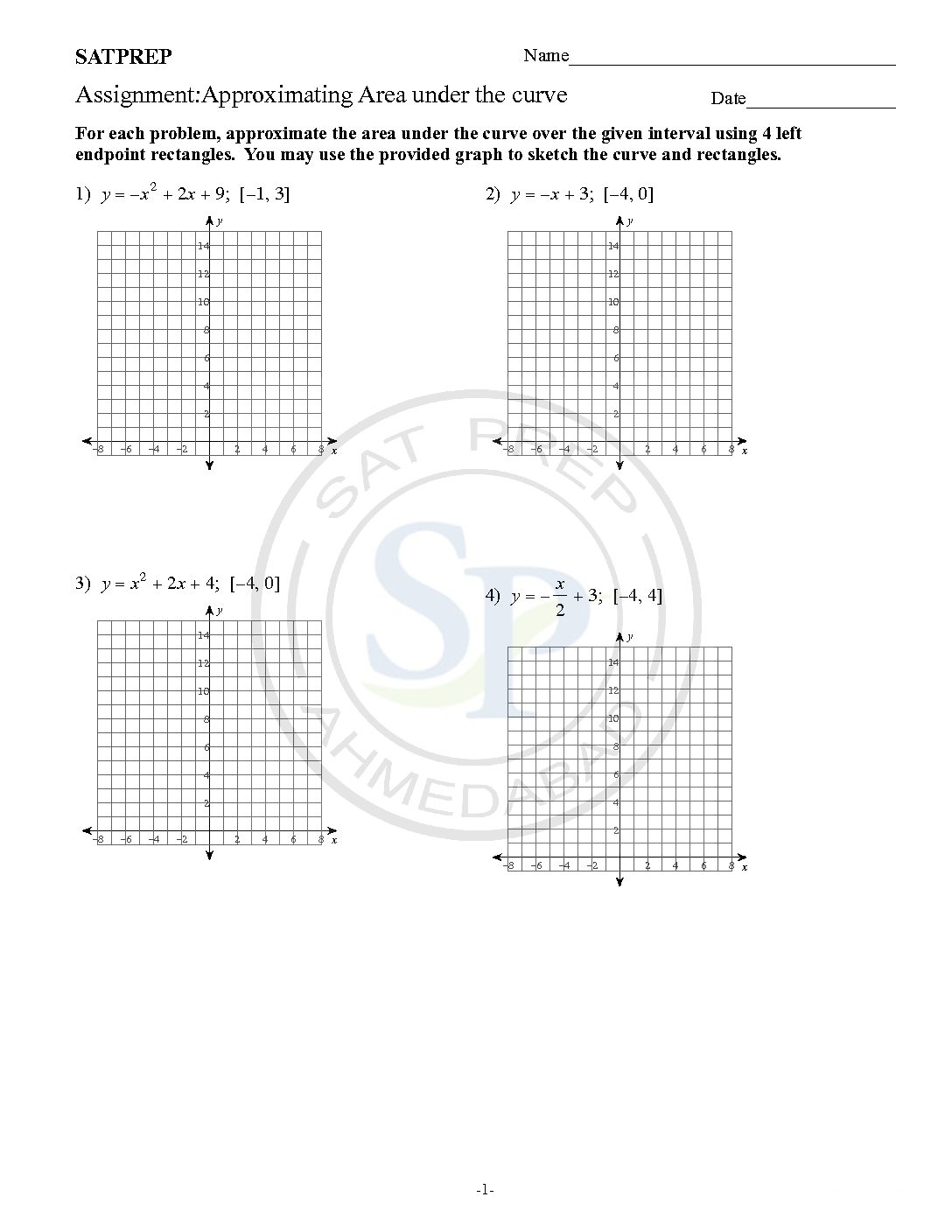
Approximating area under the curve
Approximate area of under a curve. Compute left, right, and midpoint Hence Riemann sums use with n rectangles are computed. Due to the this it approximate area. Approximate area under