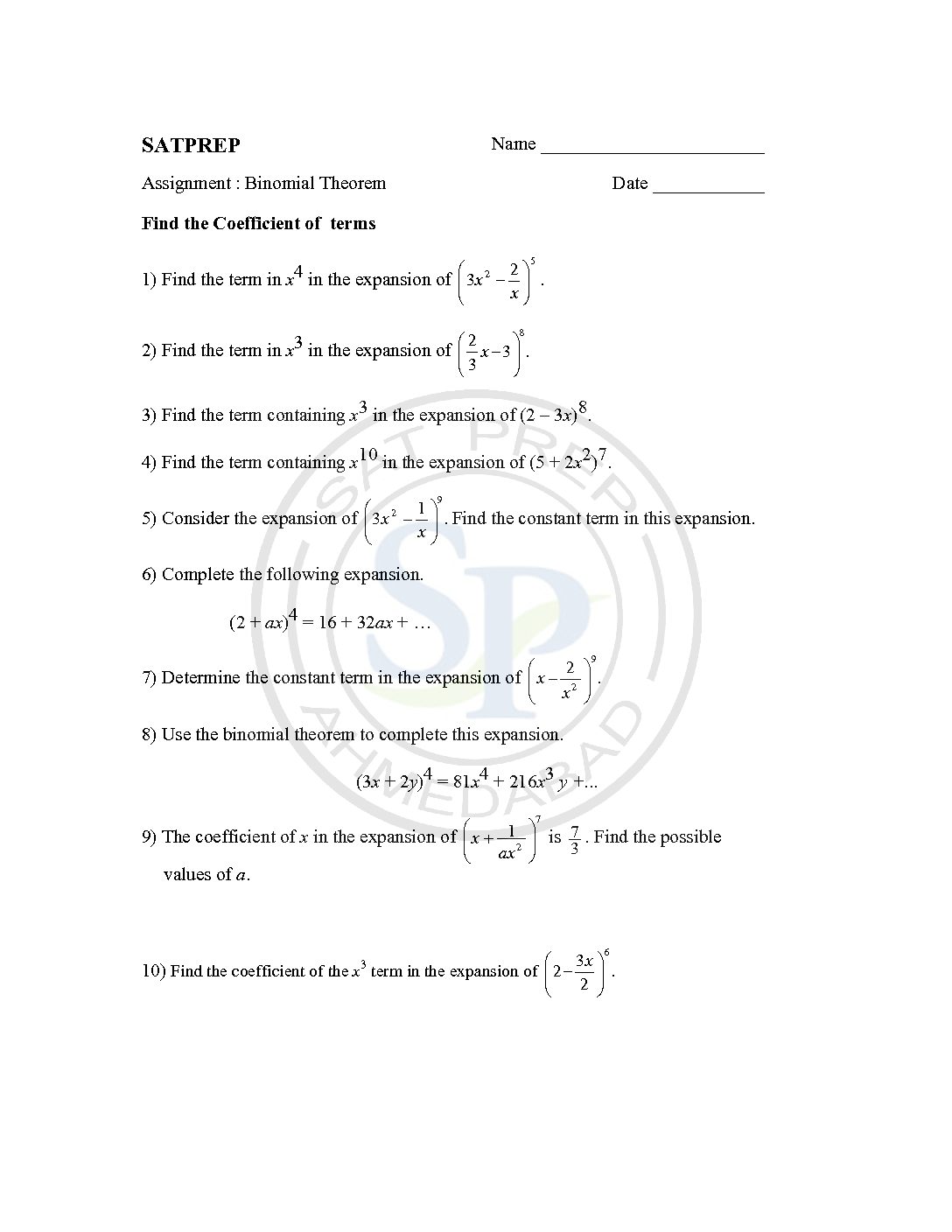
When a binomial is raised to whole number powers, the coefficients of the terms in the expansion form a pattern. Each expansion has one more term than the power on the binomial. The sum of the exponents in each term in the expansion is the same as the power on the binomial. www.kutasoftware.com
You are browsing archives for
Category: Cambridge Maths A
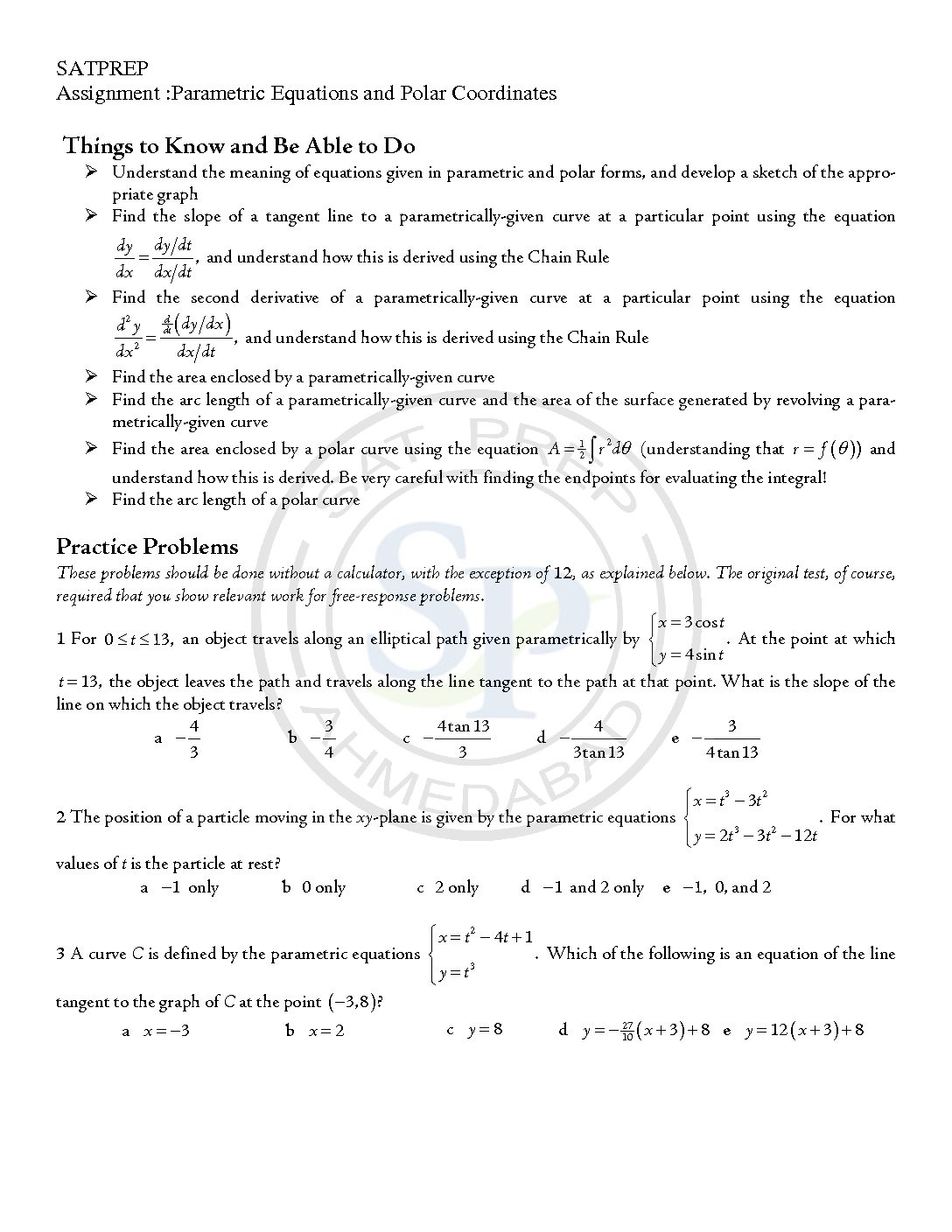
Parametric and Polar Equation
Parametric and Polar Equations , a function with two variables, x and y. In some cases, though it is useful to introduce a third variable, called a parameter, and express x and y in terms of the parameter. Polar equations ,work. Coordinates in polar equations are of the form (r,θ), where r represents radius and θ represents angle. Hence both are same type […]
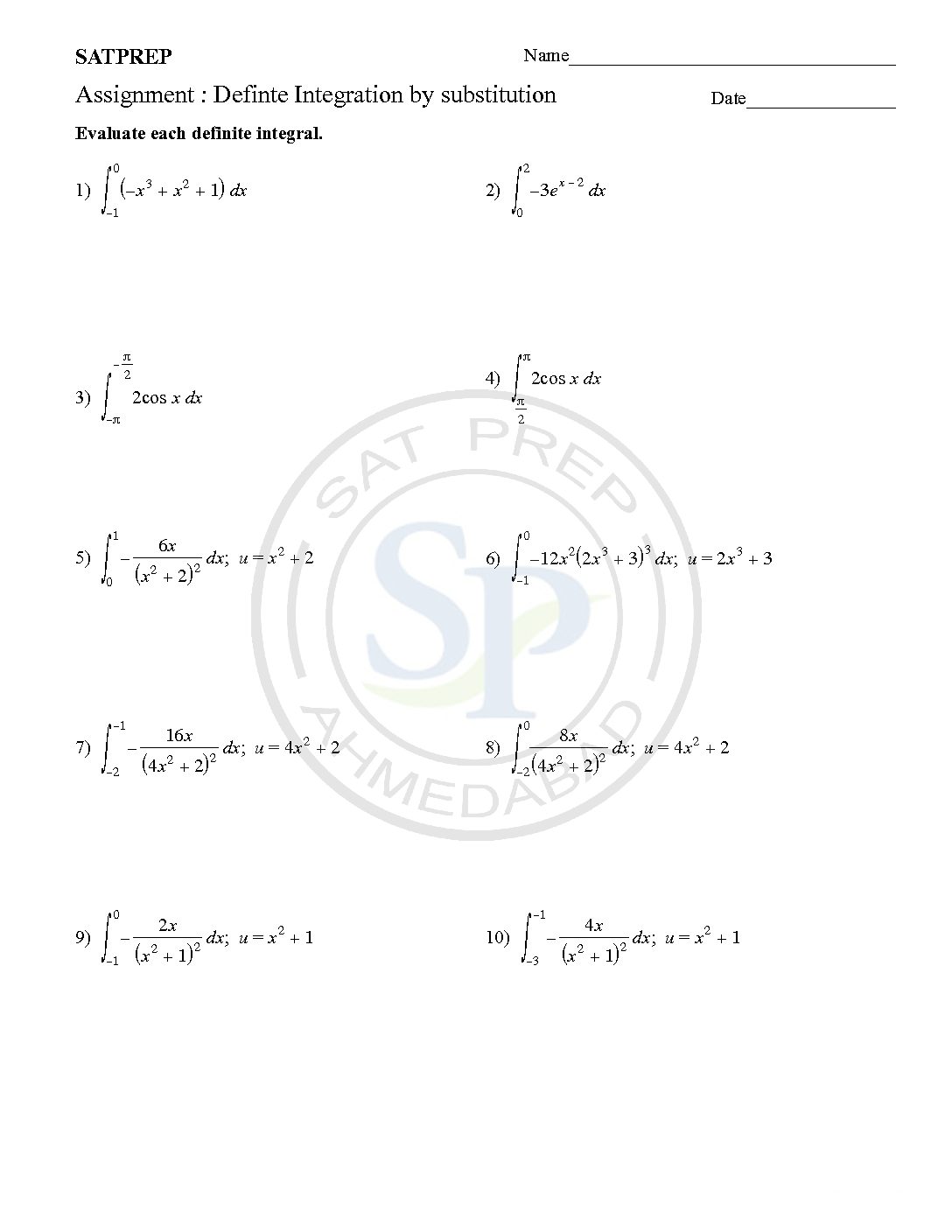
Definite integration
A Definite Integral has start and end values. In other words there is an interval [a, b]. Hence , definite integral gives particular solution. Definite Integration
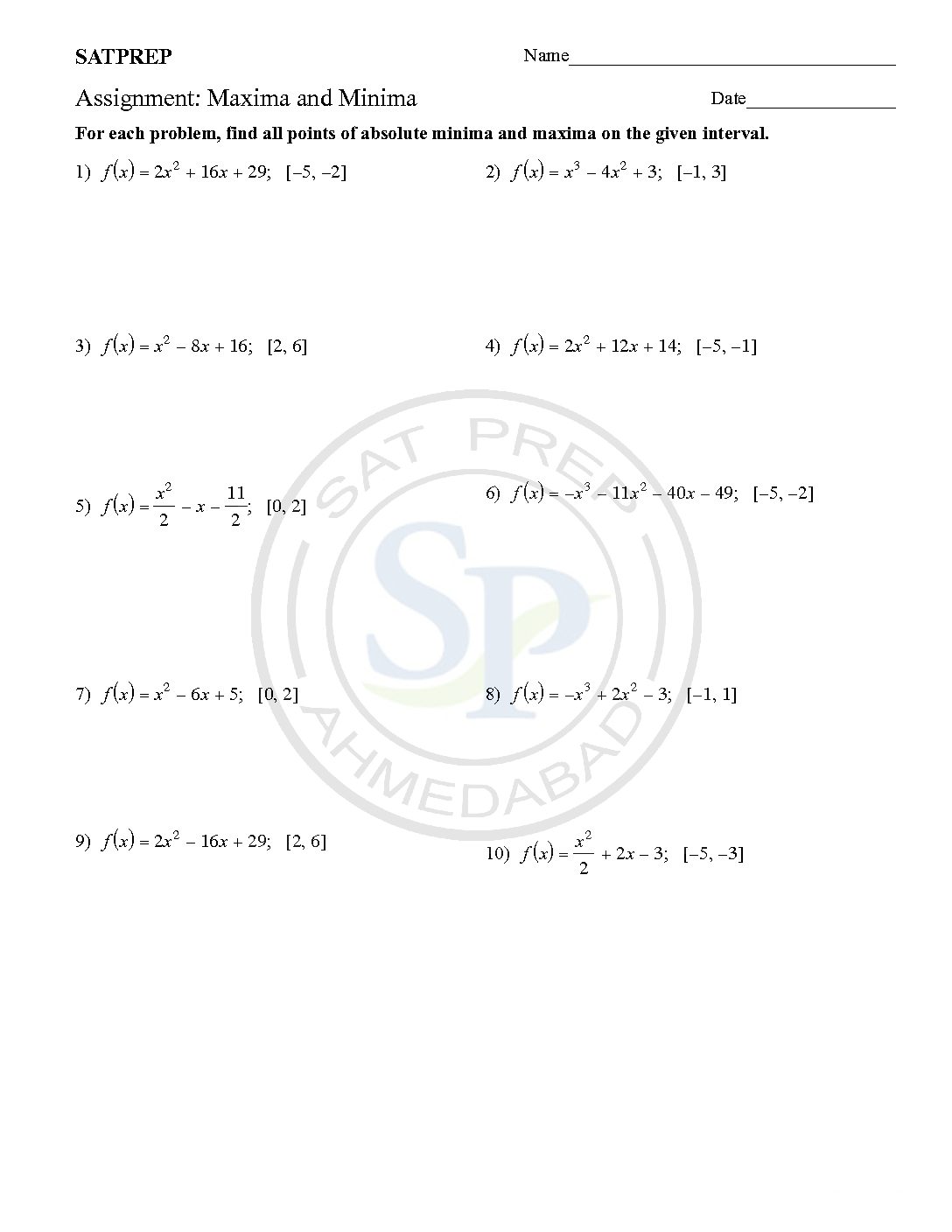
Maxima and minima
Maximum and Minima of Points of Inflection. The value f ‘(x) is the gradient at any point but often we want to find the Turning or Stationary Point (Maximum and Minimum points) or Point of Inflection These happen where the gradient is zero, f ‘(x) = 0. Critical Points include Turning points and Points where f ‘ (x) does not exist. […]
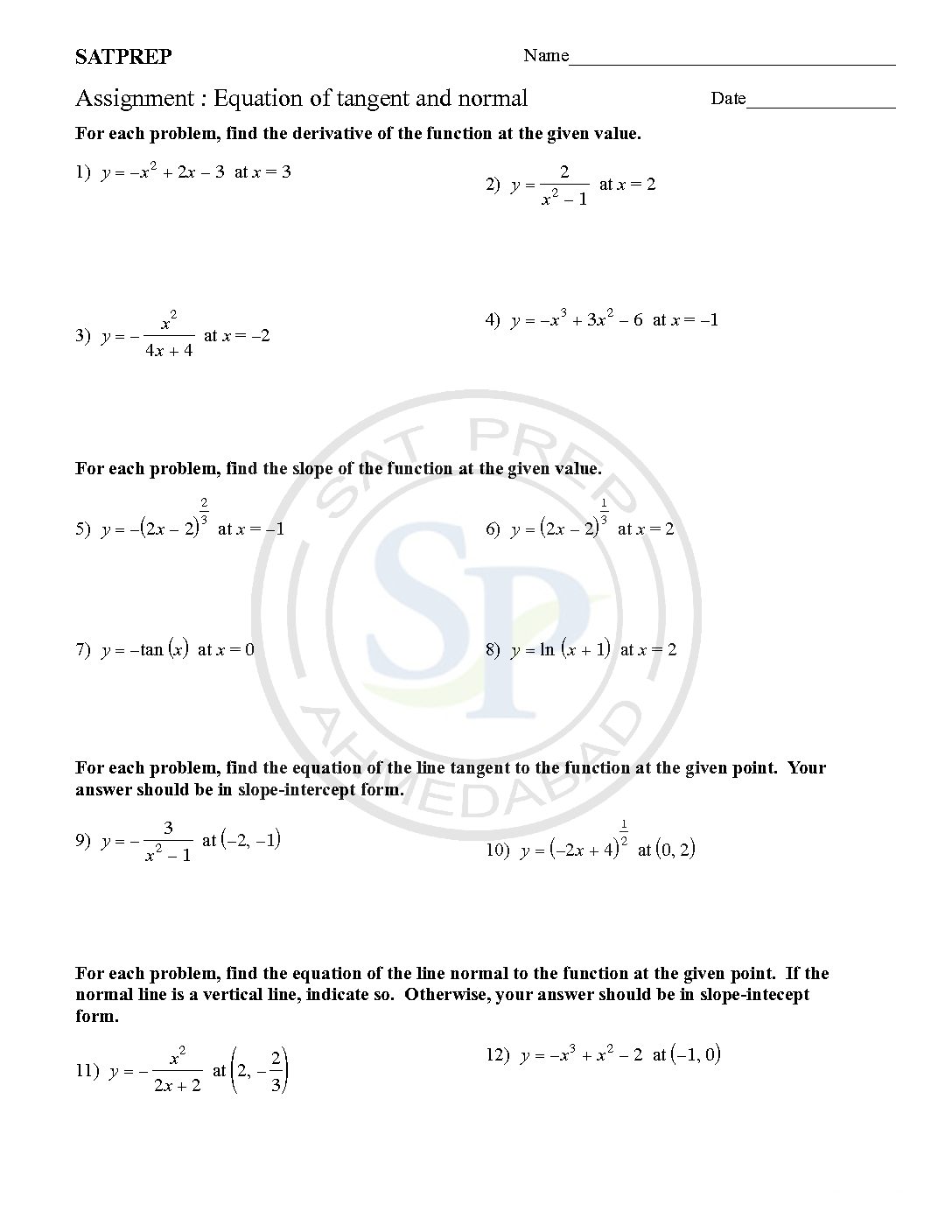
Equation of Tangent and Normal
A tangent to a curves are a line that touches the curve at one point and has the same slope as the curve at that point. A normal to a curve is a line perpendicular to a tangent to the curve. Tangent and normal
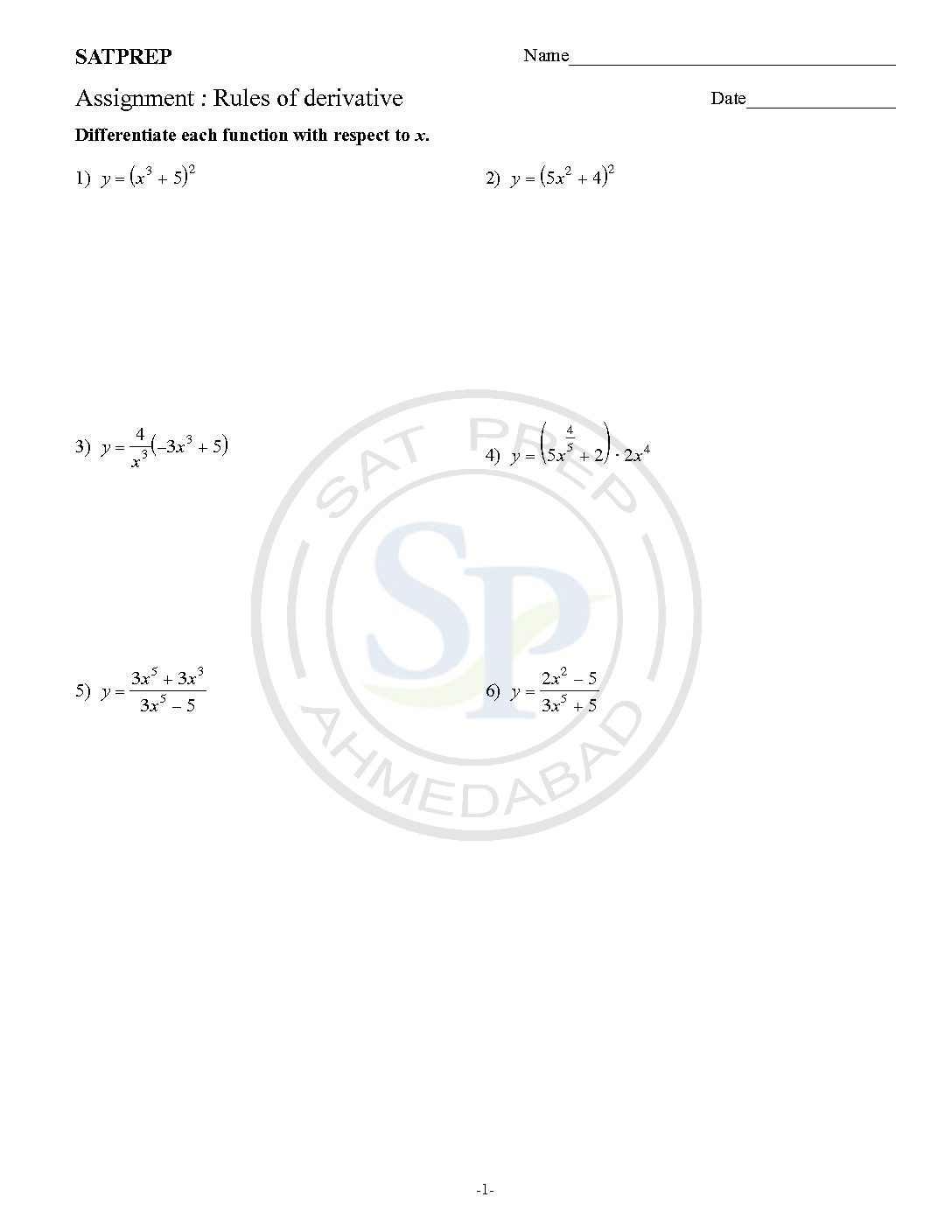
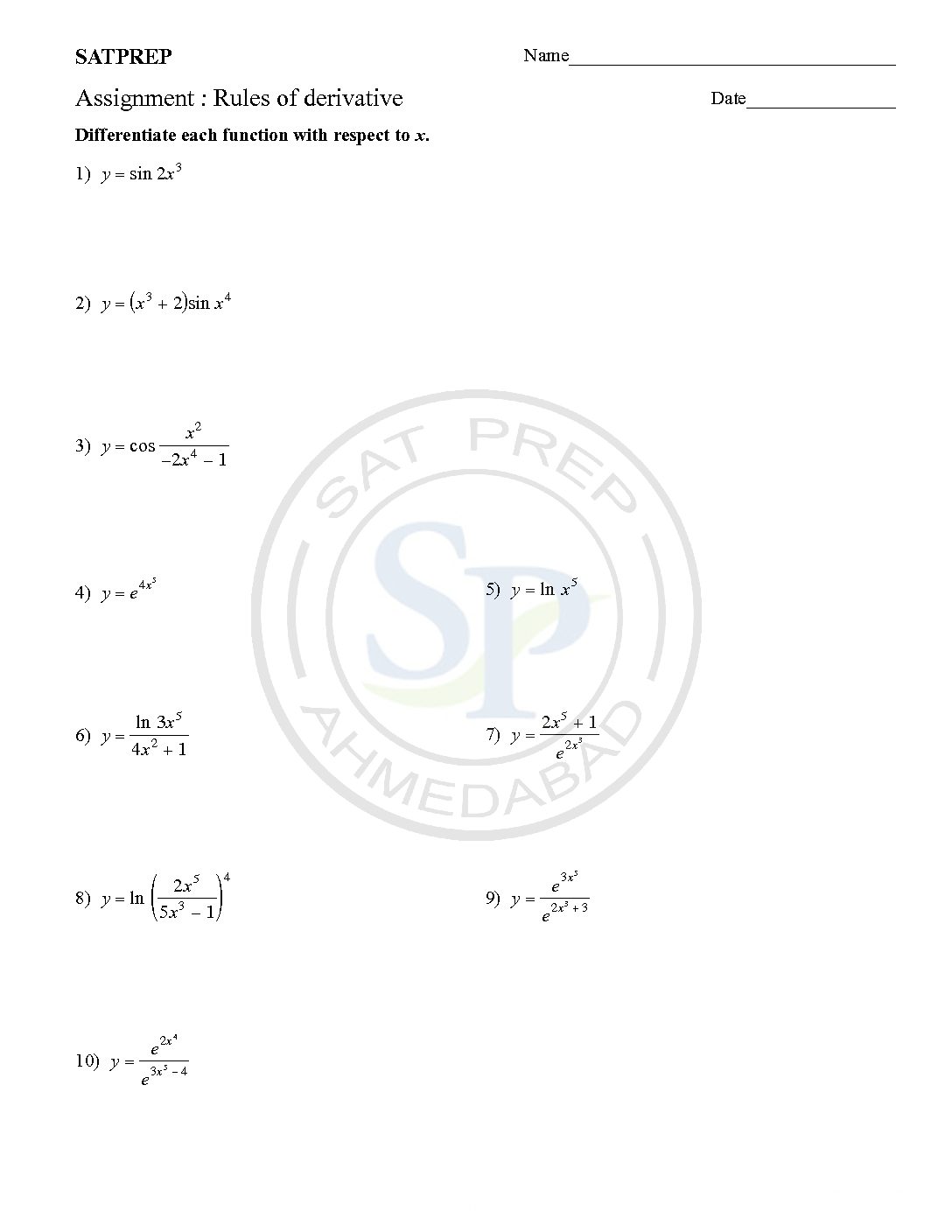
Rules of derivative
Rules for derivatives. Rules for derivatives. Sum rule: The derivative of the sum or difference of two functions is the sum or difference of their derivatives. (u + v)’ = u’ + v’ Constant multiple: The derivative of a constant times a function is the constant times the derivative of the function. (ku)’ = ku’ Rules for derivative
Rules of derivative
Rule for derivatives. Rules for derivatives. Sum rule: The derivative of the sum or difference of two functions is the sum or difference of their derivatives. (u + v)’ = u’ + v’ Constant multiple: The derivative of a constant times a function is the constant times the derivative of the function. (ku)’ = ku’ Rules of derivative
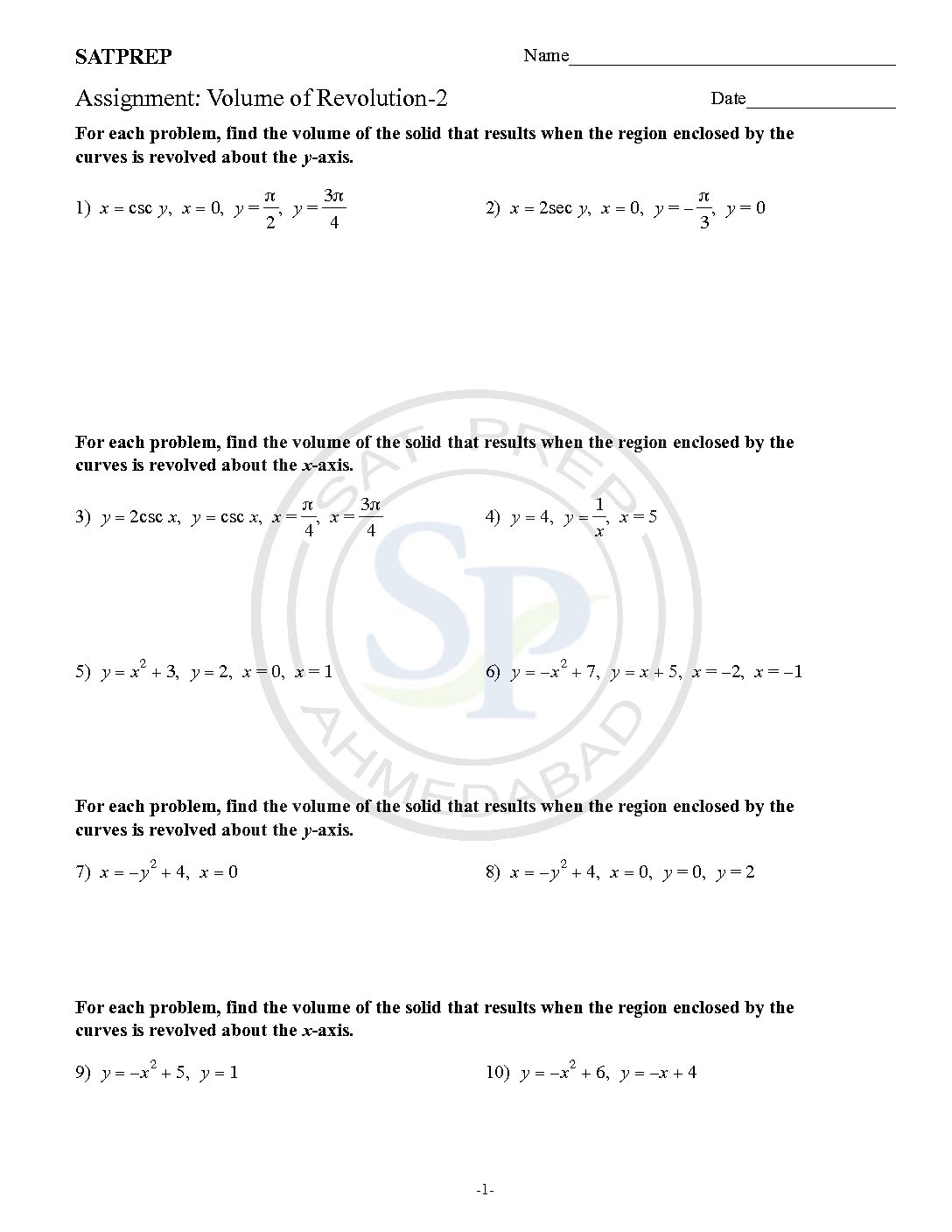
Volume of revolution of solid
Volume of revolution. To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. For purposes of this discussion let’s rotate the curve about the x -axis, although it could be any vertical […]
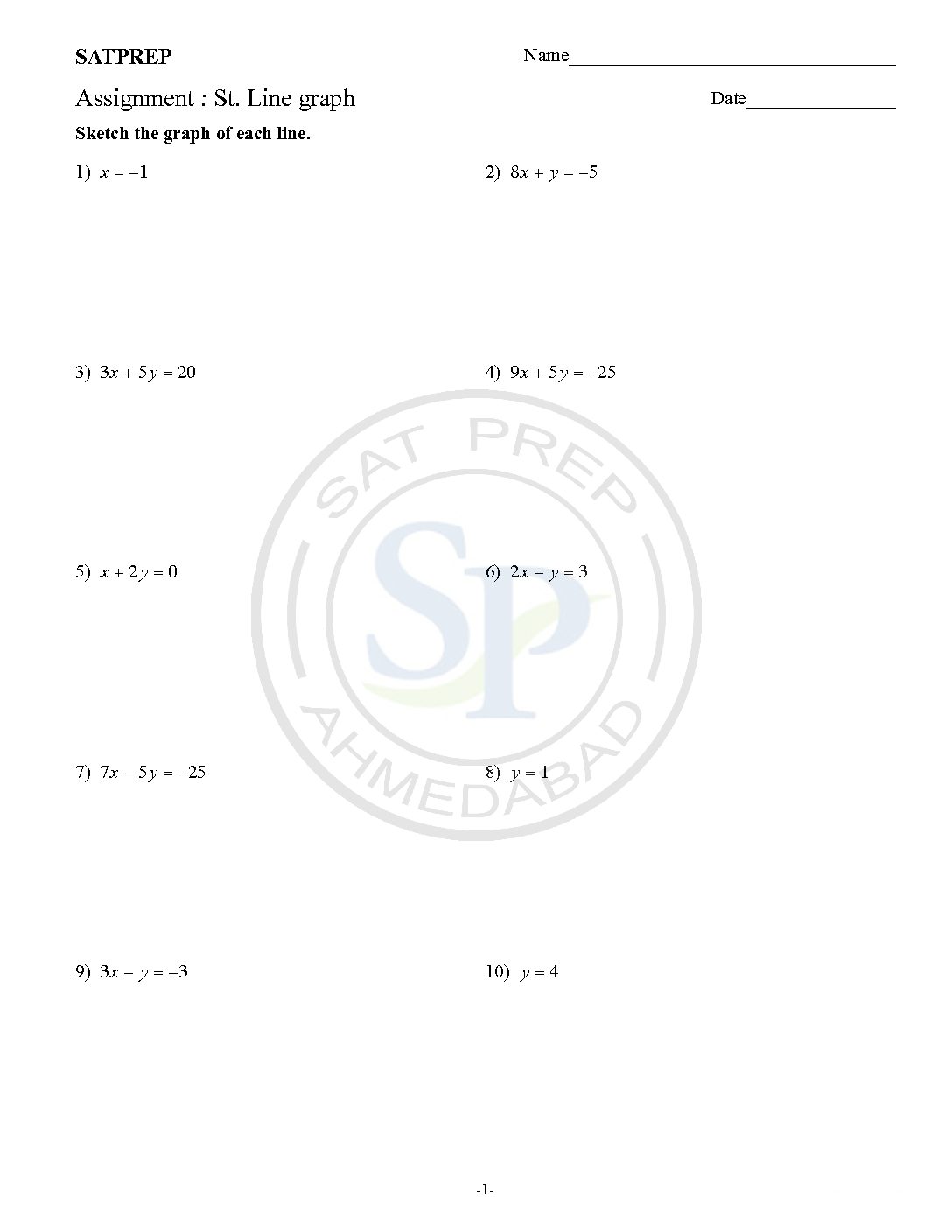
St. Line Graph
Straight line graphs. The graph of each of equations is a straight line: If an equation can be rearranged into the form y = mx +c, then its graph will be a straight line. ax +by= c can be rearranged as (which can be re-written as y = mx+c). Therefore vertical lines have equations have slope undefined form x =k . Hence graph depend on […]
Volume of revolution
To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution Volume of Revolution