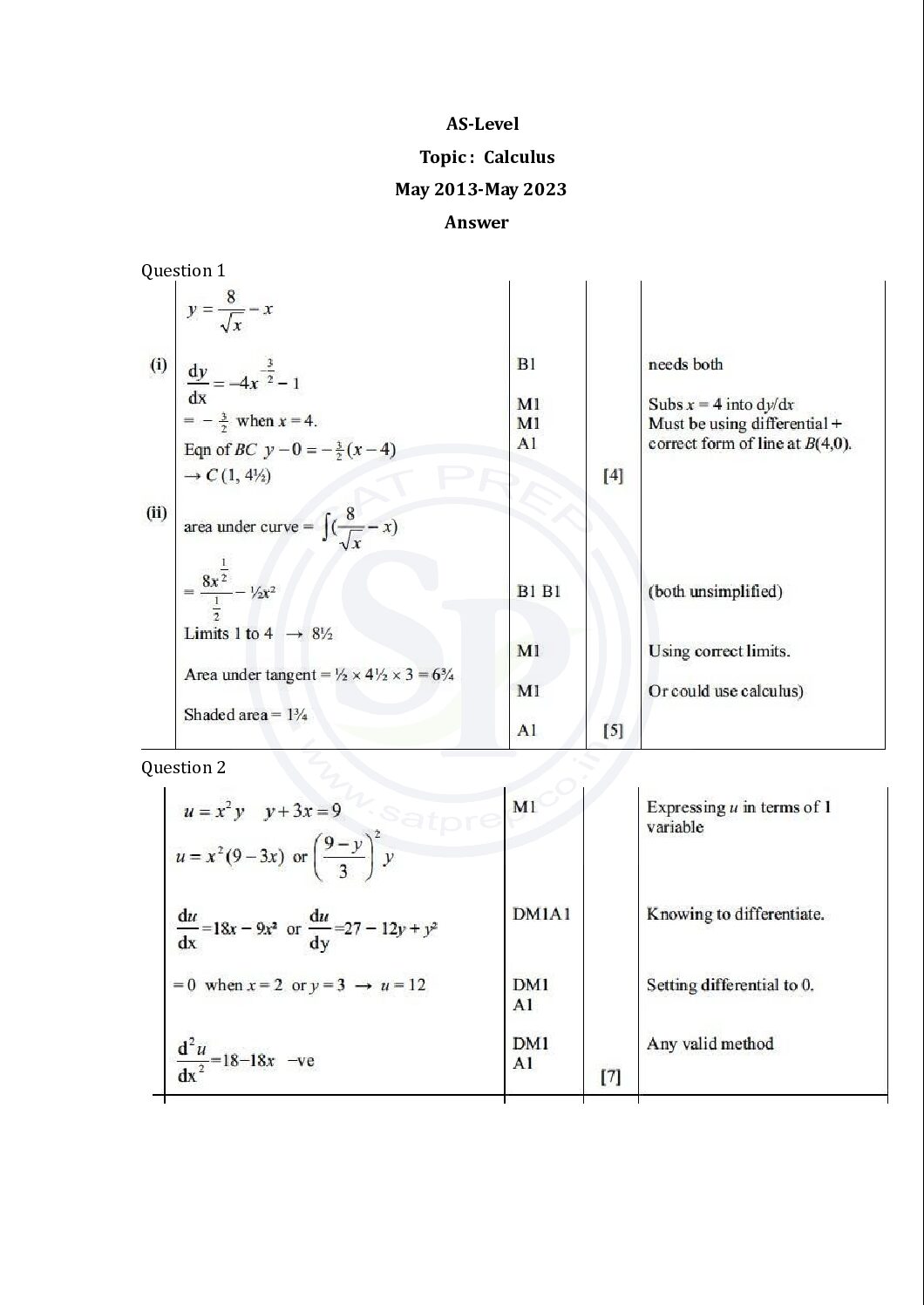
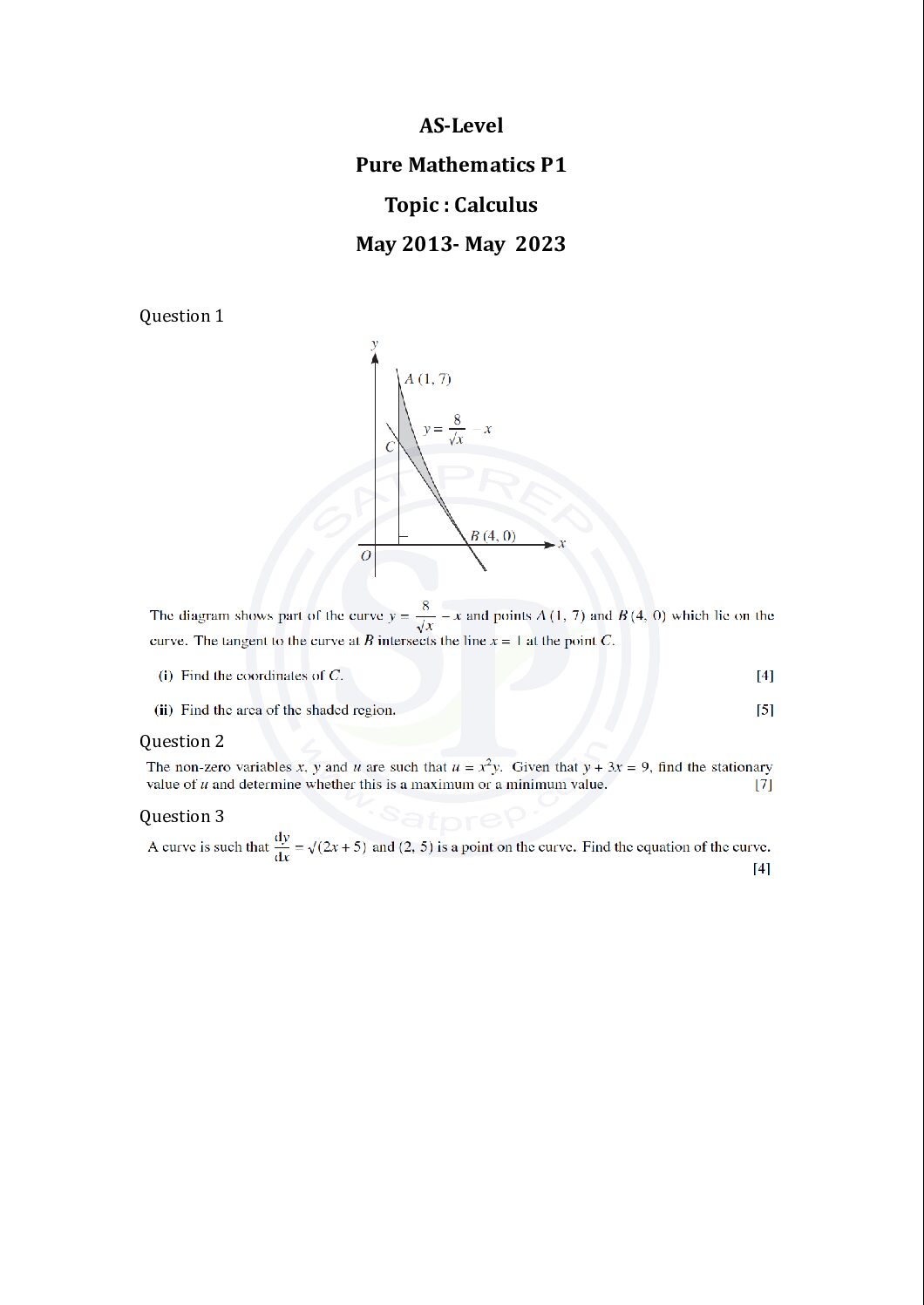
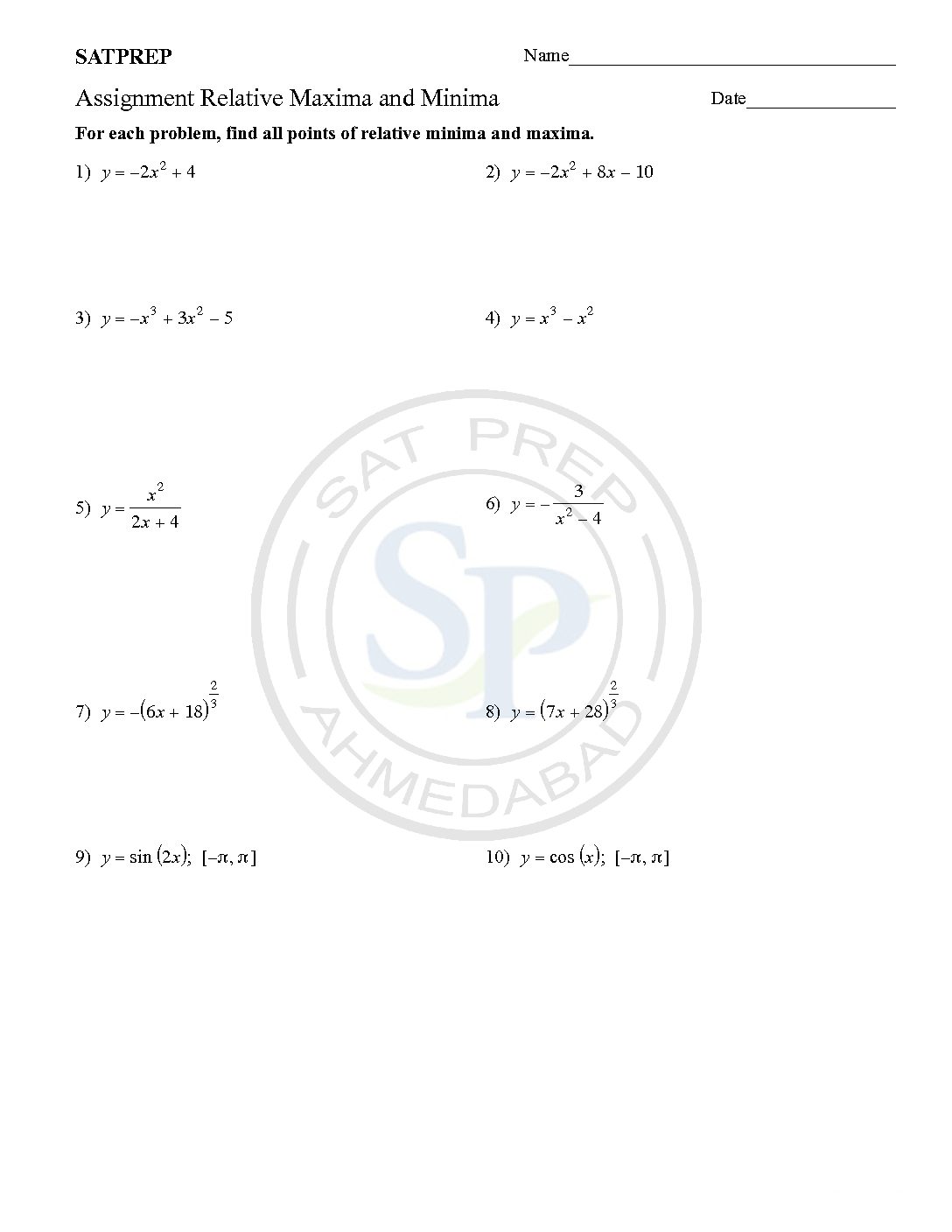
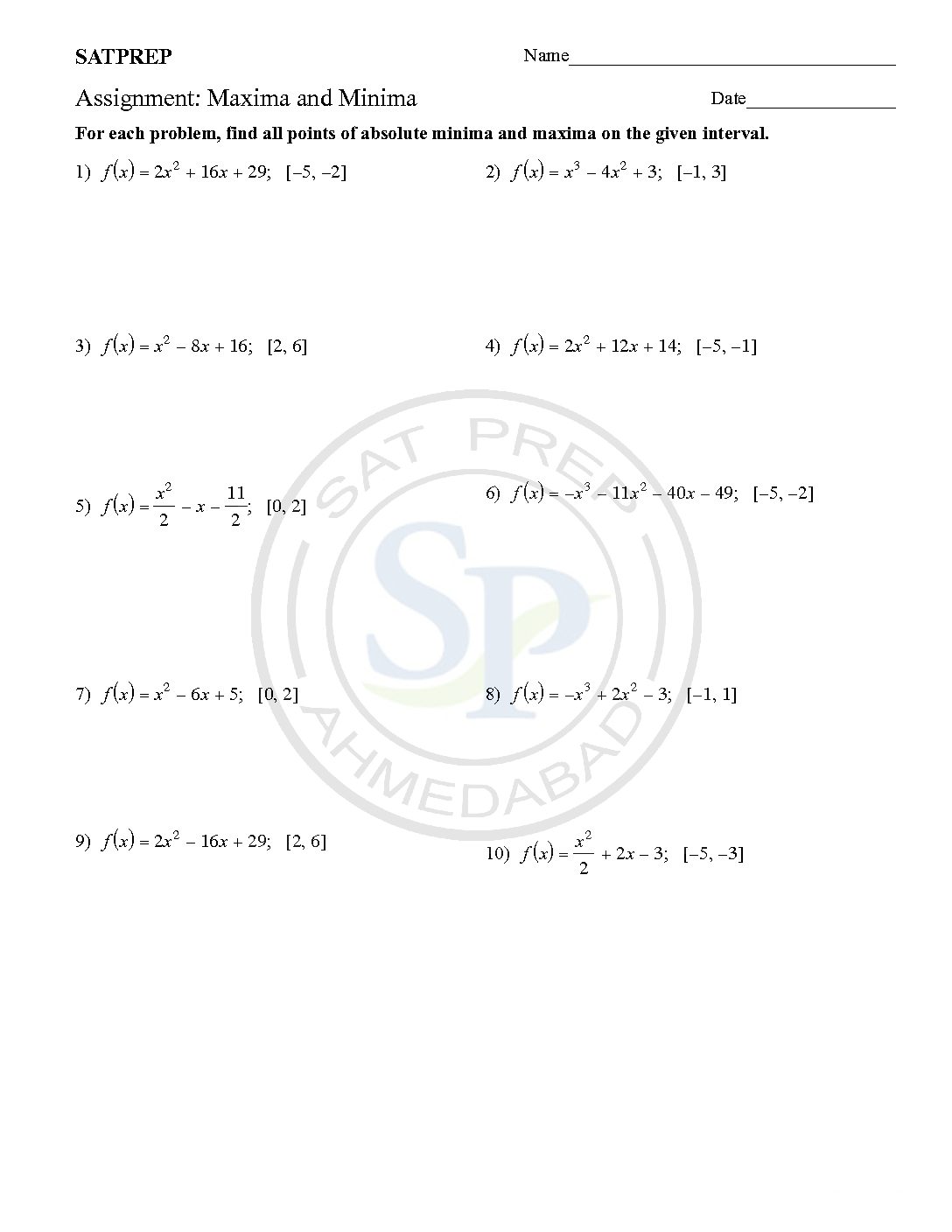
This post is about Solution of Calculus AS-level questions of past papers. In this post questions are from May 2013 to May 2023 papers . By this post students will come to know variety of questions asked in previous year papers. www.cie.org.uk f t u
You are browsing archives for
Category: Calculus
Topic wise AS-Level Pure Math Past Paper...
This post is about Questions of calculus AS-level from past paper. In this post questions are from different paper from May 2013 to May 2023. By this post students will come to know variety of questions asked in previous year papers. The questions type in this post is calculator . www.cie.org.uk
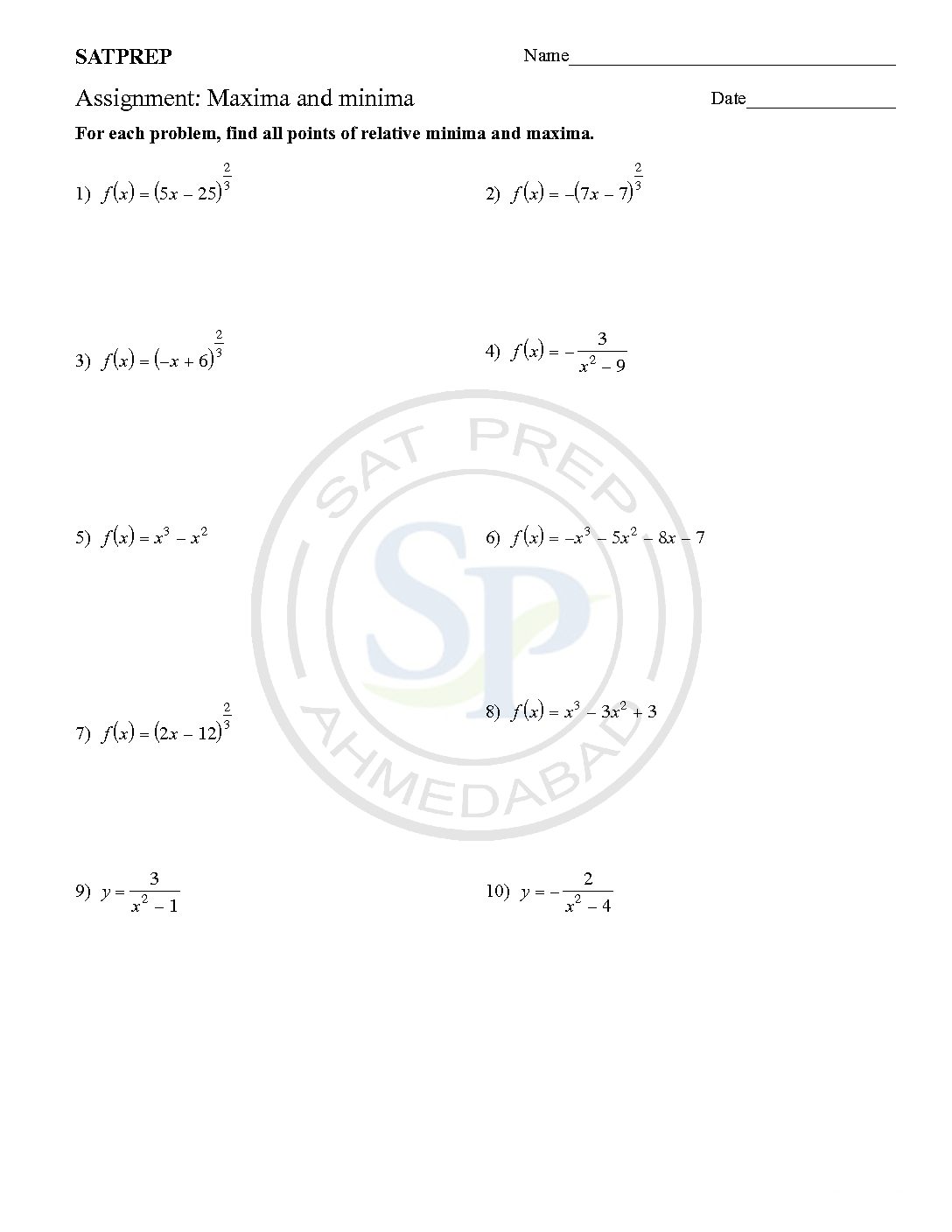
Maxima and minima
A high point of curve is called a maxima. A low point is called a minima. In the Curve only one global maxima or minima exists , while more than one local maximum or minimum. Due to curve turn on these point are called local. Hence these point also called stationary points. Maxima and minima
Maxima and minima
Maximum and Minima of Points of Inflection. The value f ‘(x) is the gradient at any point but often we want to find the Turning or Stationary Point (Maximum and Minimum points) or Point of Inflection These happen where the gradient is zero, f ‘(x) = 0. Critical Points include Turning points and Points where f ‘ (x) does not exist. […]
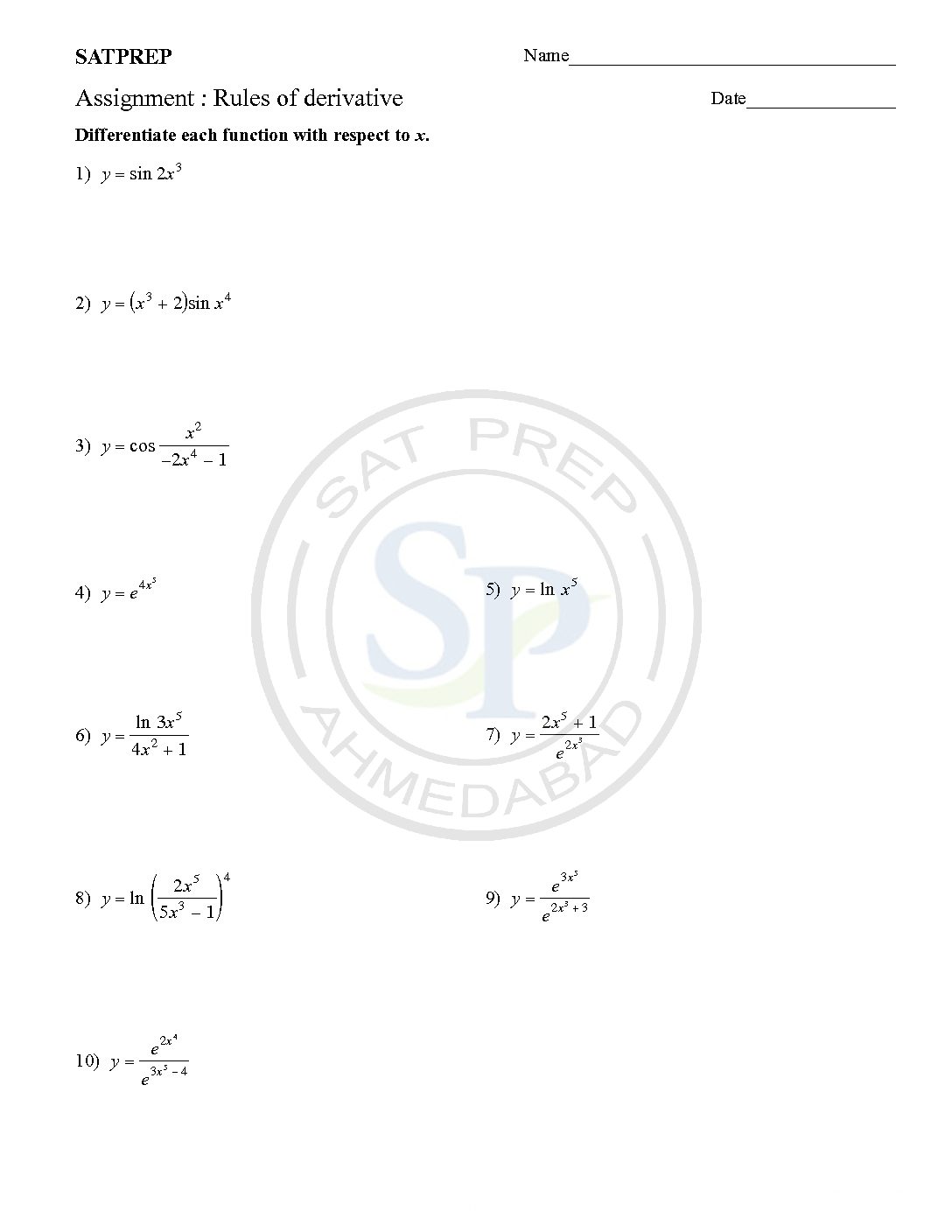
Rules of derivative
Rule for derivatives. Rules for derivatives. Sum rule: The derivative of the sum or difference of two functions is the sum or difference of their derivatives. (u + v)’ = u’ + v’ Constant multiple: The derivative of a constant times a function is the constant times the derivative of the function. (ku)’ = ku’ Rules of derivative
Maxima and Minima
Maximum and minimum of Points of Inflection. The value f ‘(x) is the gradient at any point but often we want to find the Turning or Stationary Point (Maximum and Minimum points) or Point of Inflection These happen where the gradient is zero, f ‘(x) = 0. Critical Points include Turning points and Points where f ‘ (x) does […]
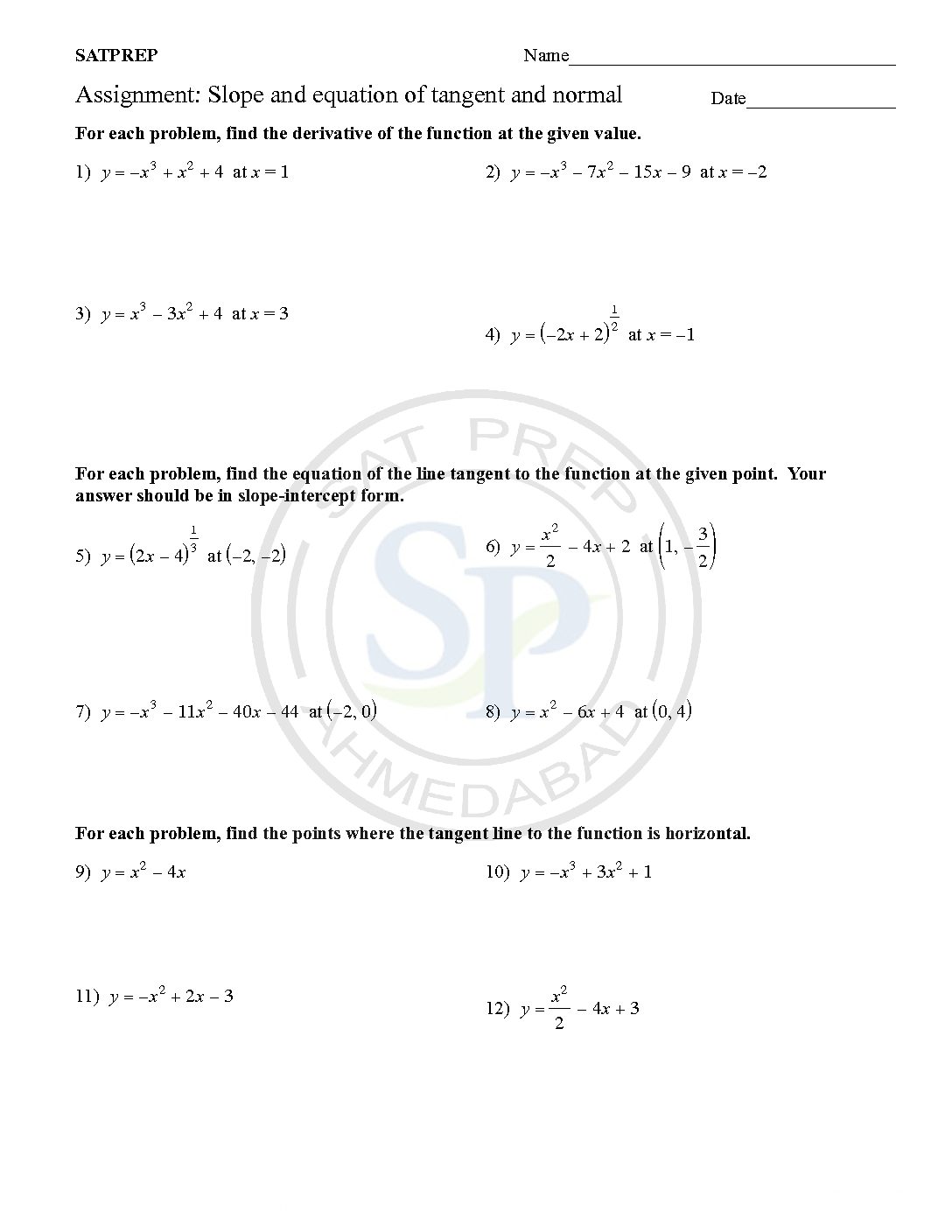
Equation of Tangent and Normal
Tangents to a curve are a line that touches the curve at one point and has the same slope as the curve at that point. A normal to a curve is a line perpendicular to a tangent to the curve. Tangent and Normal
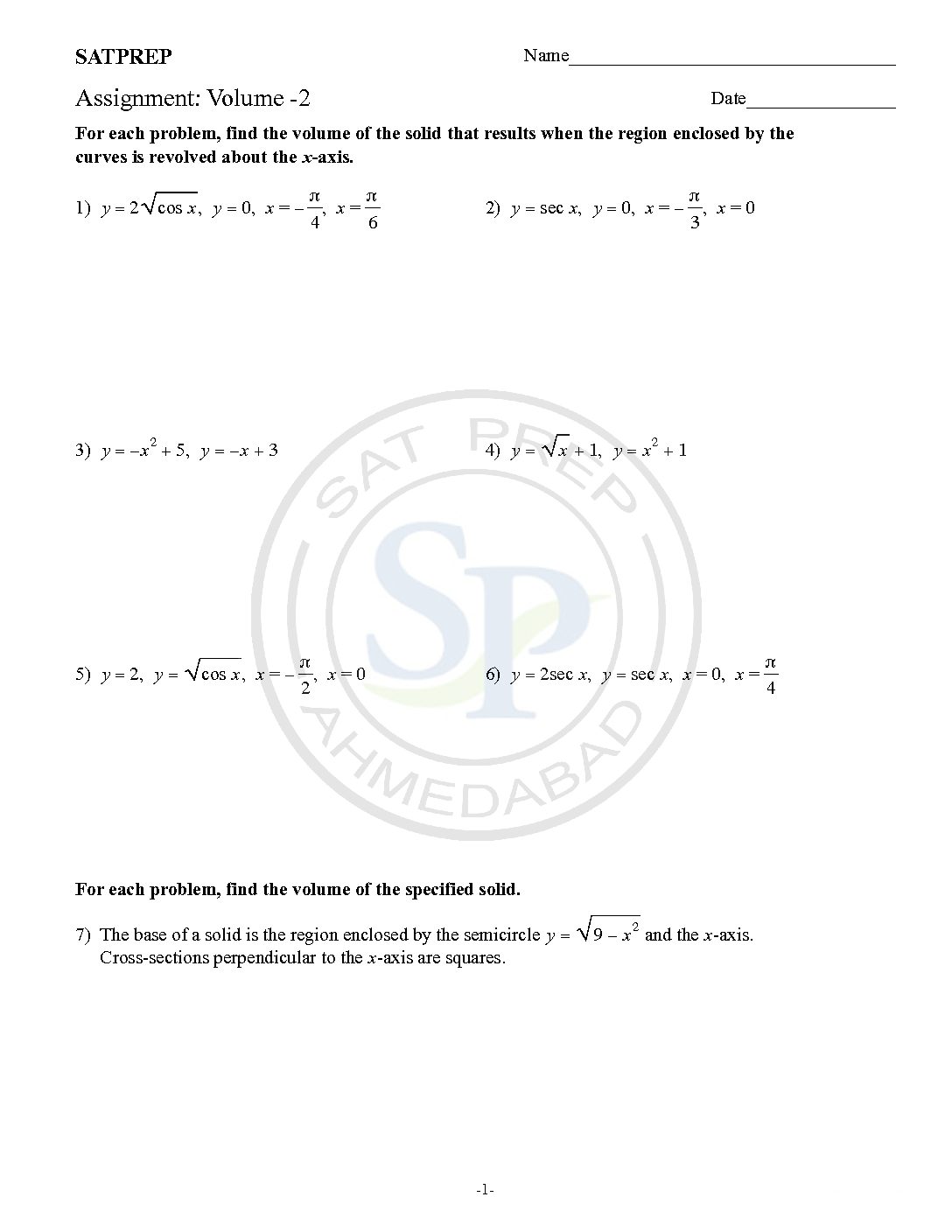
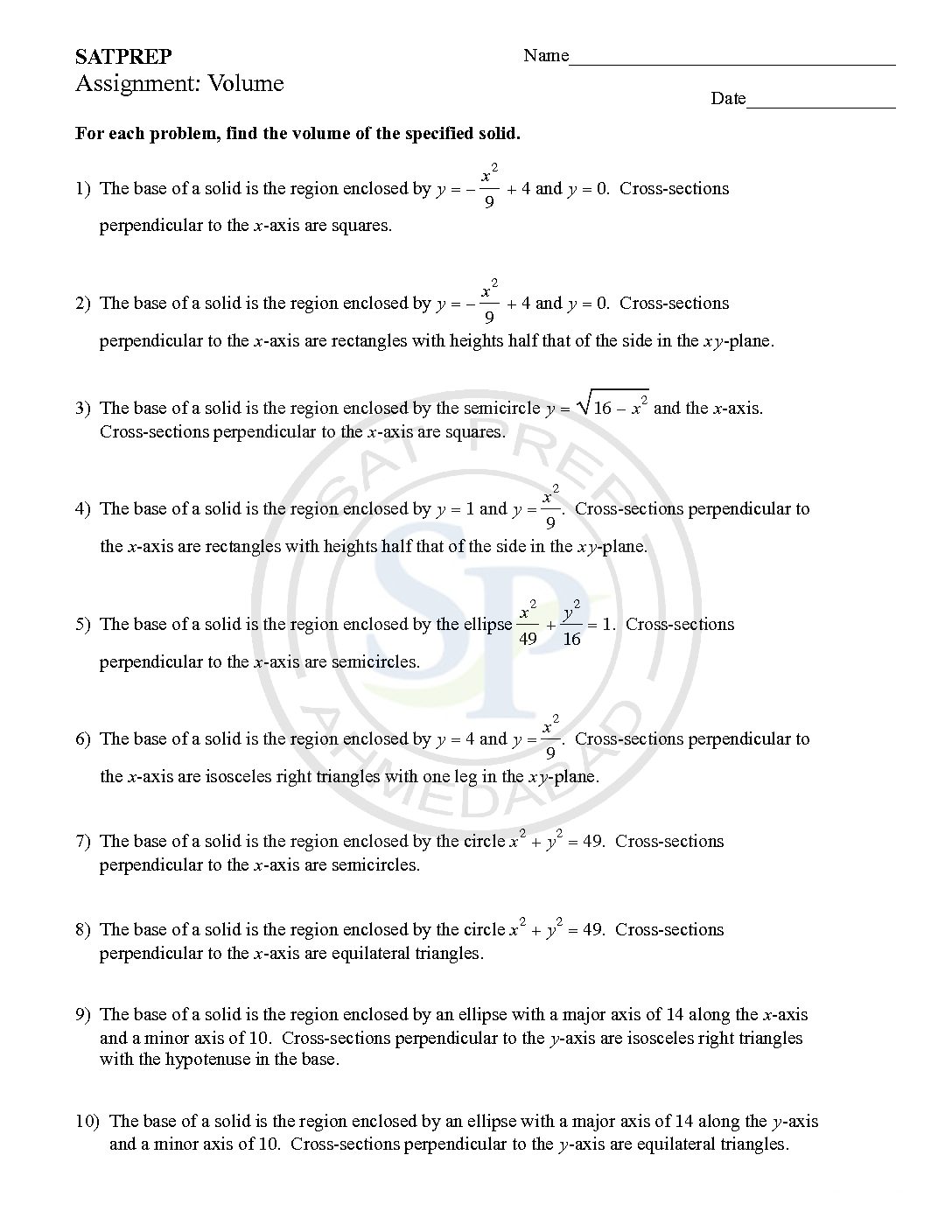
Volume -2
To get a solids of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. Volume
Volume
To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. volume of revolution
Tangent and normal : Implicit
Tangent and Normal Lines for implicit curves are a line that touches the implicit curve at one point and has the same slope as the curve at that point. A normal to a curve is a line perpendicular to a tangent to the curve. tangent and normal