Function notations are method of writing equation. Mostly , functions are portrayed as a set of x/y coordinates. Therefore y-axis serving as a function of x. When we use function F(x) notation then one is independent variable and another dependent variable. Due to this on Y-axis F(x) represent. Function notation
You are browsing archives for
Category: Pure Maths
This is pure maths category under cambridge as level
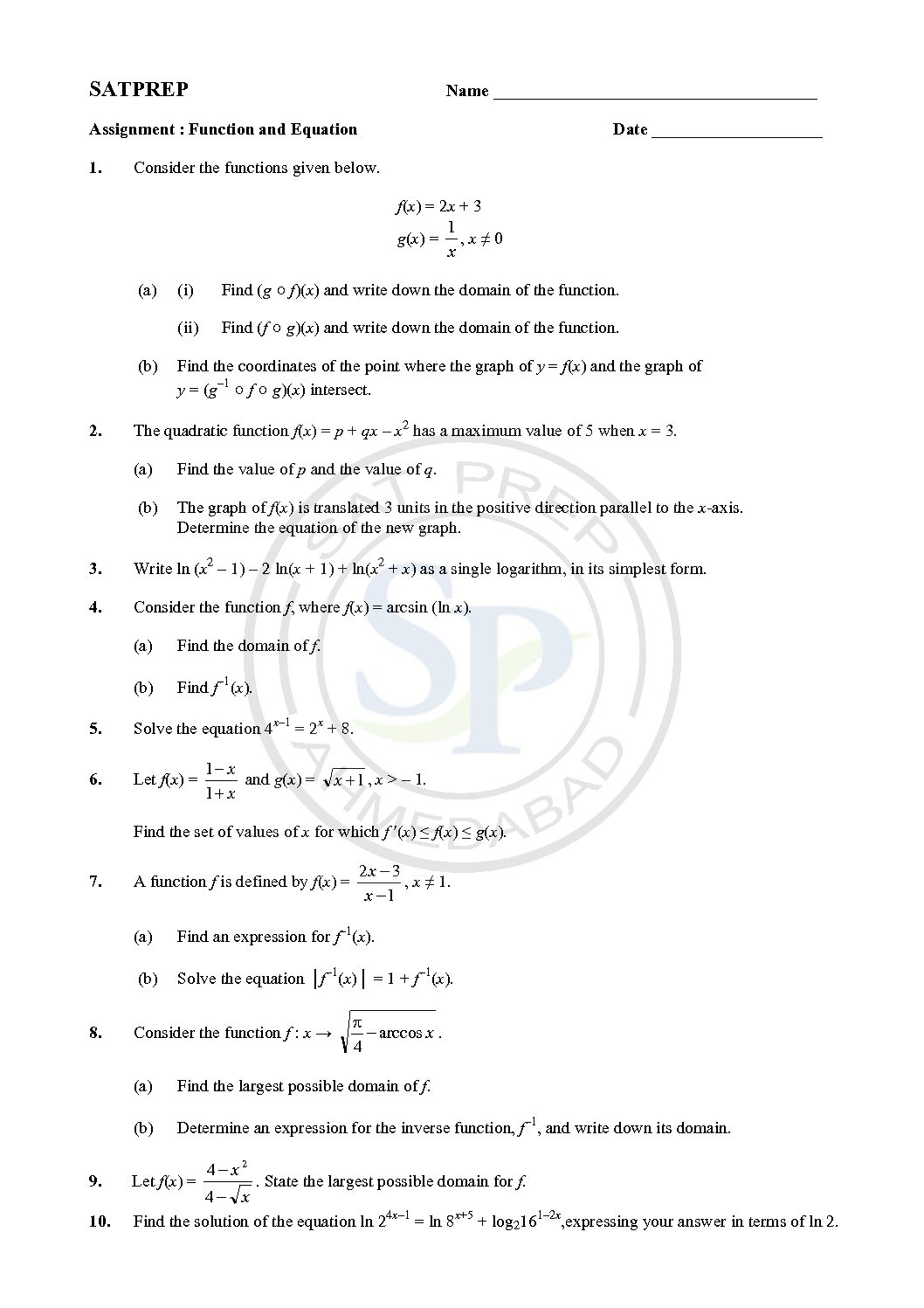
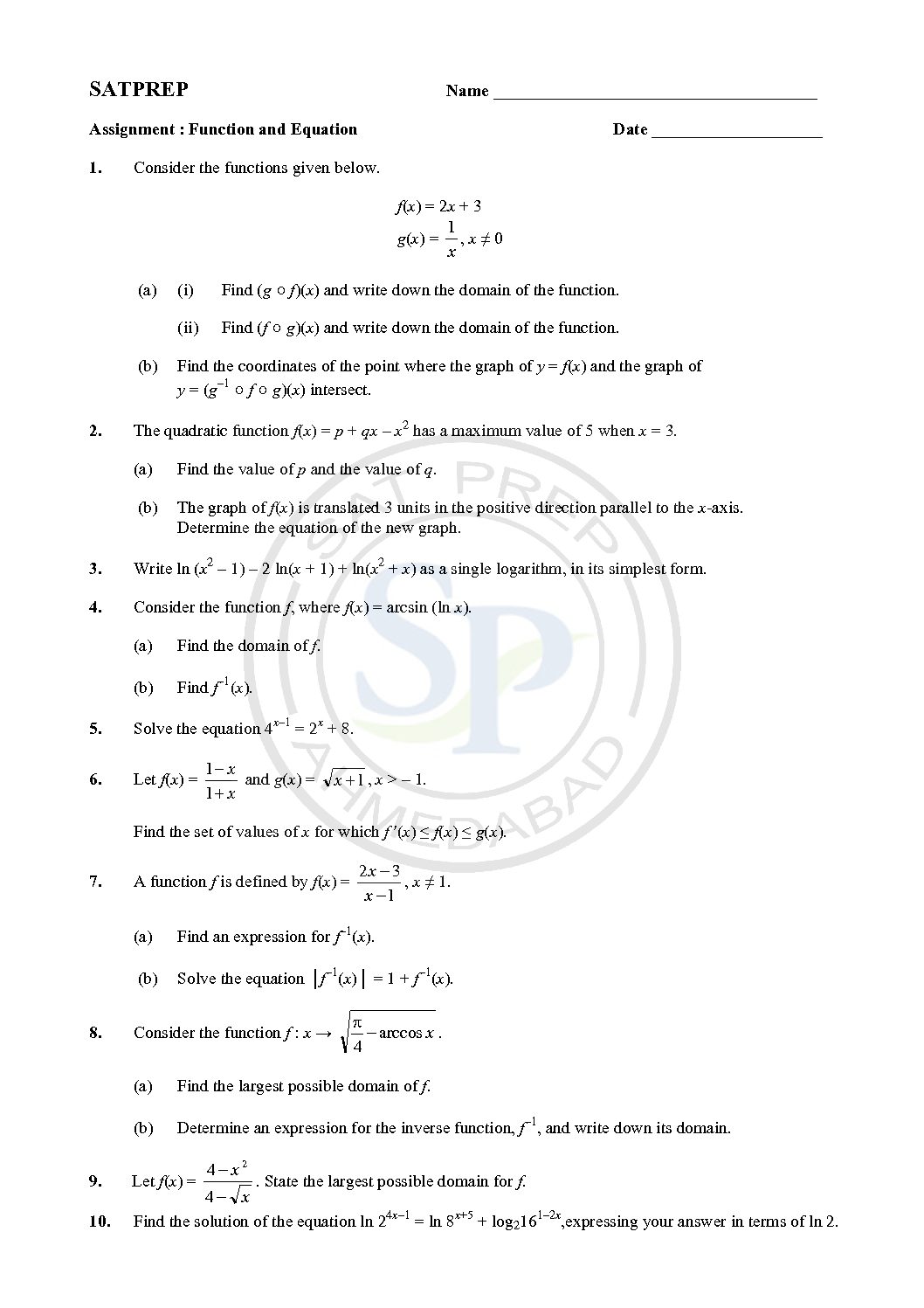
Function and Equation
Function notations are method of writing equation. Mostly , functions are portrayed as a set of x/y coordinates. Therefore y-axis serving as a function of x. When we use function F(x) notation then one is independent variable and another dependent variable. Due to this on Y-axis F(x) represent. Function Notation
Function and Equation
F(x) represent function. Mostly , functions are portrayed as a set of x/y coordinates. Therefore y-axis serving as a function of x. When we use function F(x) notation then one is independent variable and another dependent variable. Due to this on Y-axis F(x) represent. Functions
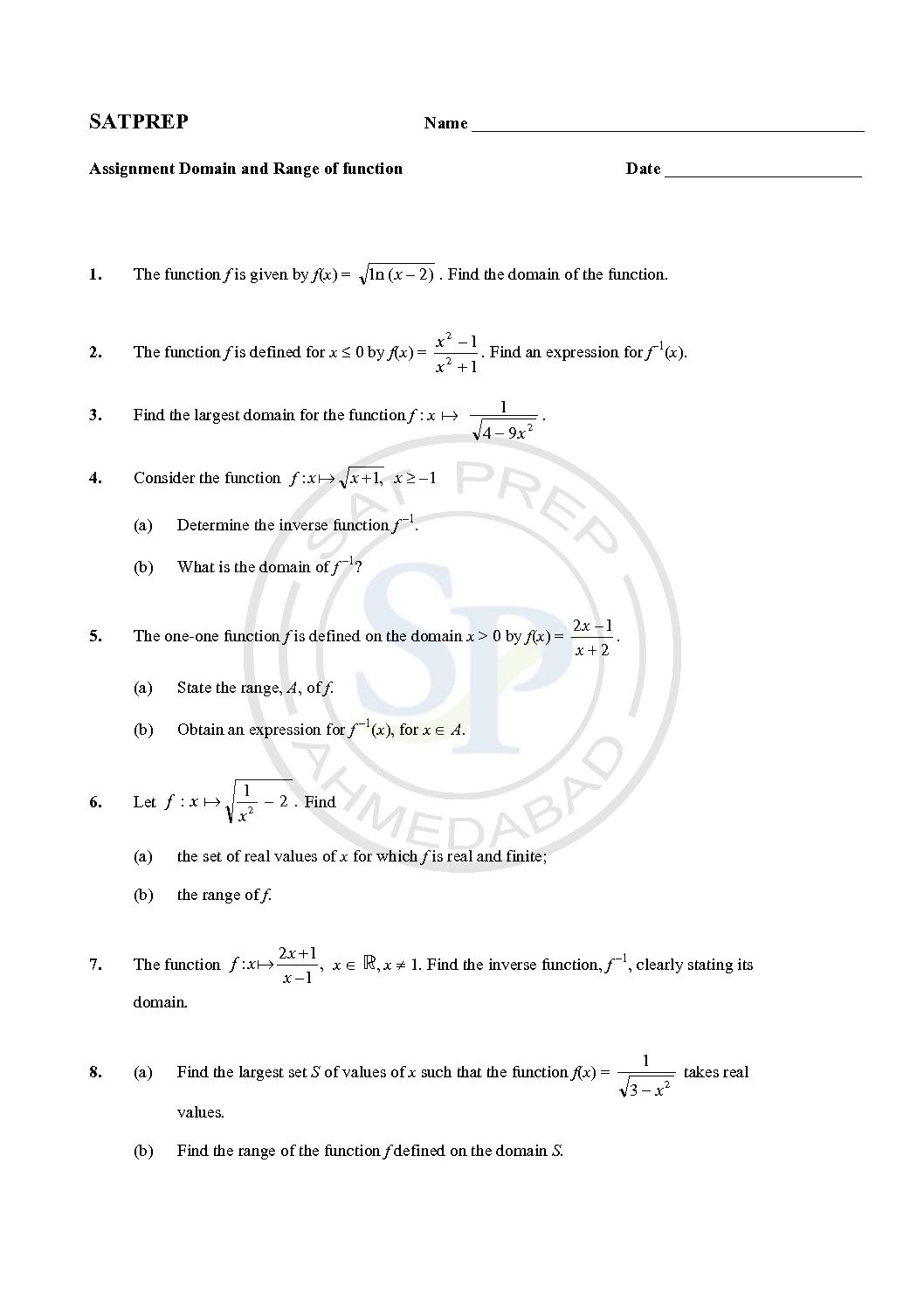
Domain and Range
Domain represent value of x , while range represented by F(x) or y function. Mostly , functions are portrayed as a set of x/y coordinates. Therefore y-axis serving as a function of x. When we use function F(x) notation then one is independent variable and another dependent variable. Due to this on Y-axis F(x) represent. Domain and range
Equation of plane and applications
A plane is determined by a point P in the plane and a normal vector n perpendicular to the plane. Since each vector in the plane must be orthogonal to the normal vector n. Equations is called a vector equation of the plane. Vector equation of a plane. a plane may be characterized by a point contained in the plane and a vector that is perpendicular, or normal, to the plane. Therefore equation plane also called cartesian equation. Plane […]
Trigonometric Equation
Solving trig equations use both the reference angles and trigonometric identities The general method of solving an equation is to convert it into the form of one ratio only. Then, using these results, hence, we can obtain solutions. Trigonometric equation
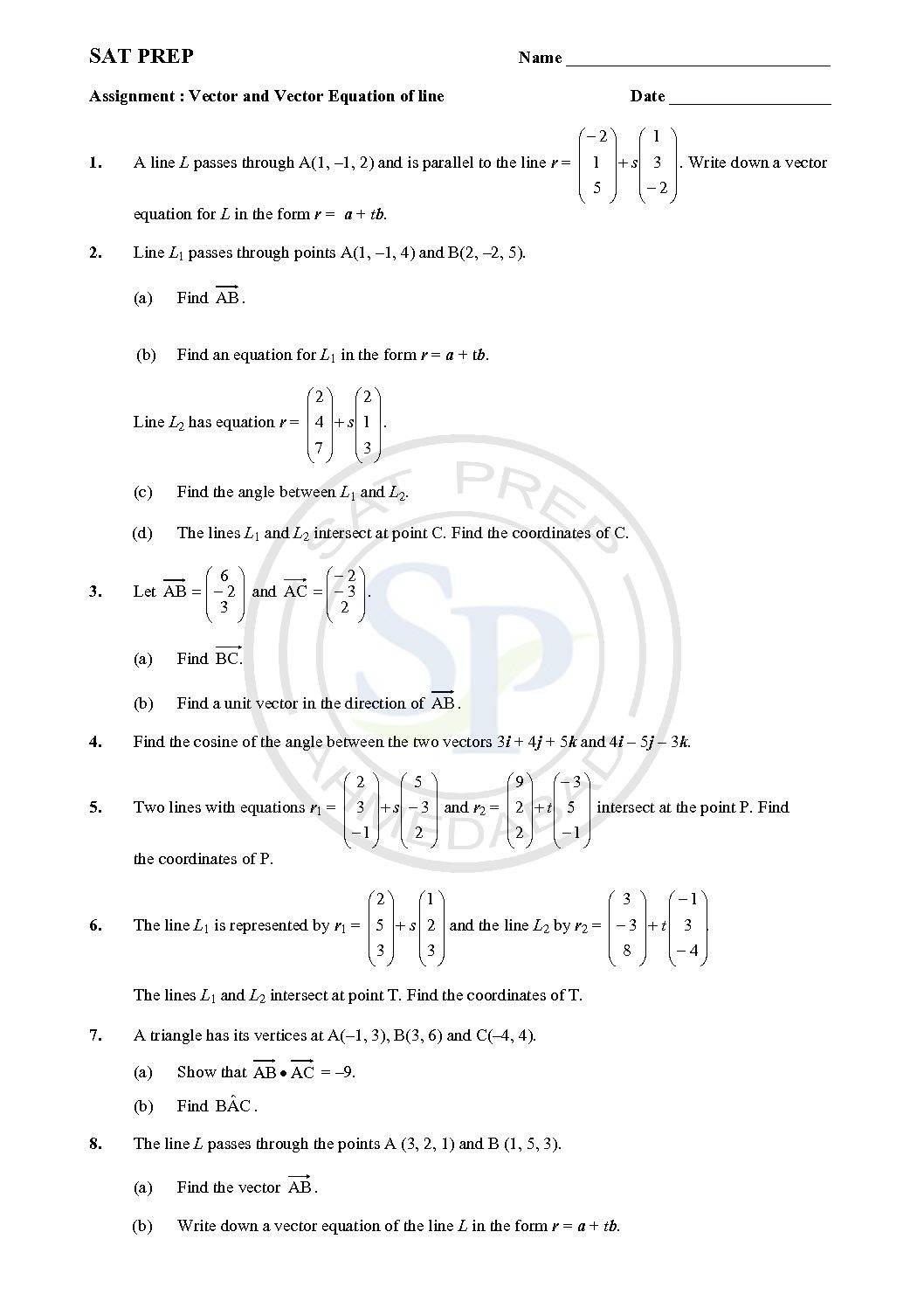
Vector and vector equation of line
The vector is direction one point to another point. The vector equation of a line is r = a + tb. In this equation, “a” represents position vector and “b” represents a direction vector of the line. Moreover “r” represents the vector of any general point on the line and “t” is constant. Hence it is similar to equation of line vector
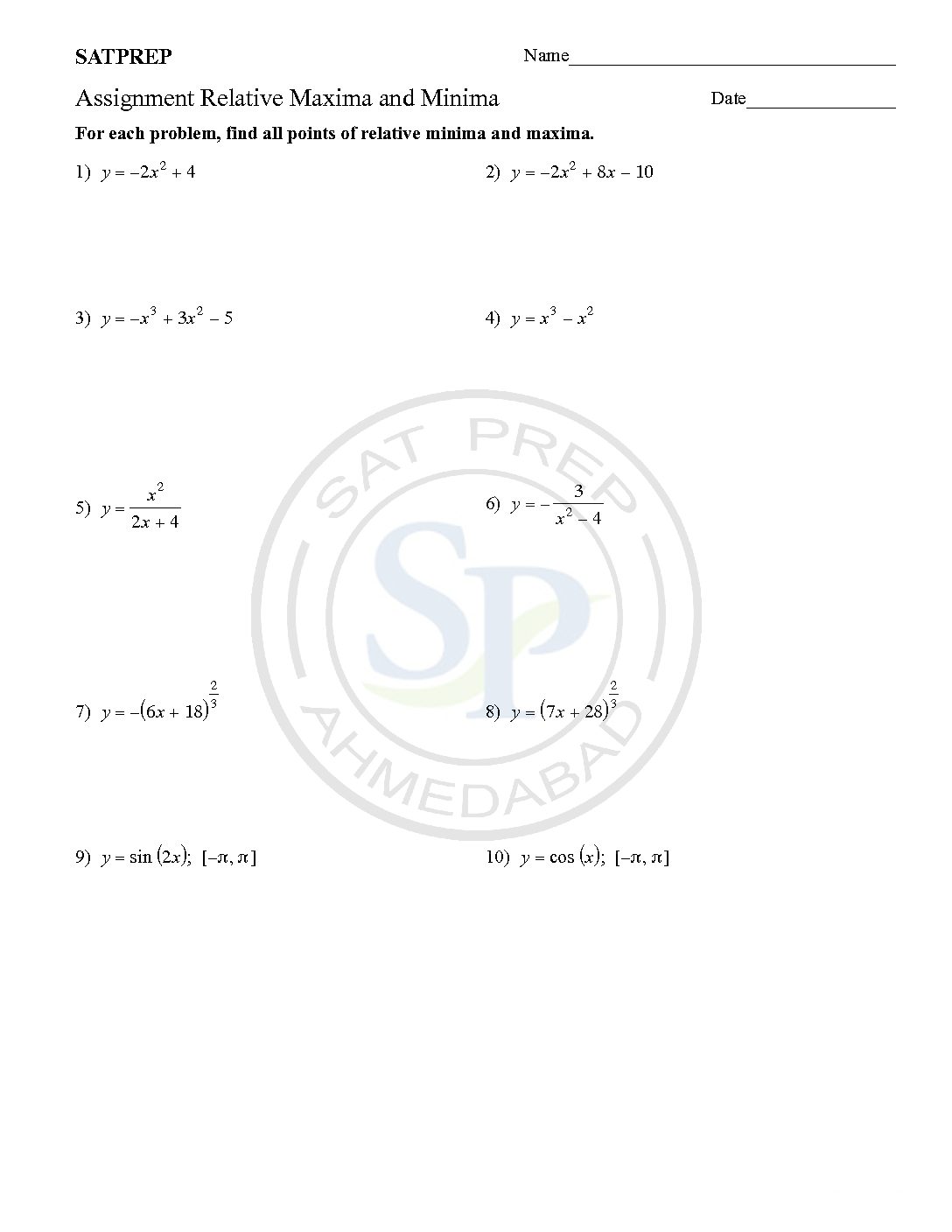
Maxima and minima
A high point of curve is called a maxima. A low point is called a minima. In the Curve only one global maxima or minima exists , while more than one local maximum or minimum. Due to curve turn on these point are called local. Hence these point also called stationary points. Maxima and minima
Equation of curve
Equations of curve evaluate by doing integration of derivative curve. The gradient and a point the curve passes through are given as.. Gradient: dy/dx = 6sqrt(x) Point the curve passes through: (4,1) I need to find the equation of the curve. Therefore integration is process of finding equation of the curve. Equation of curve
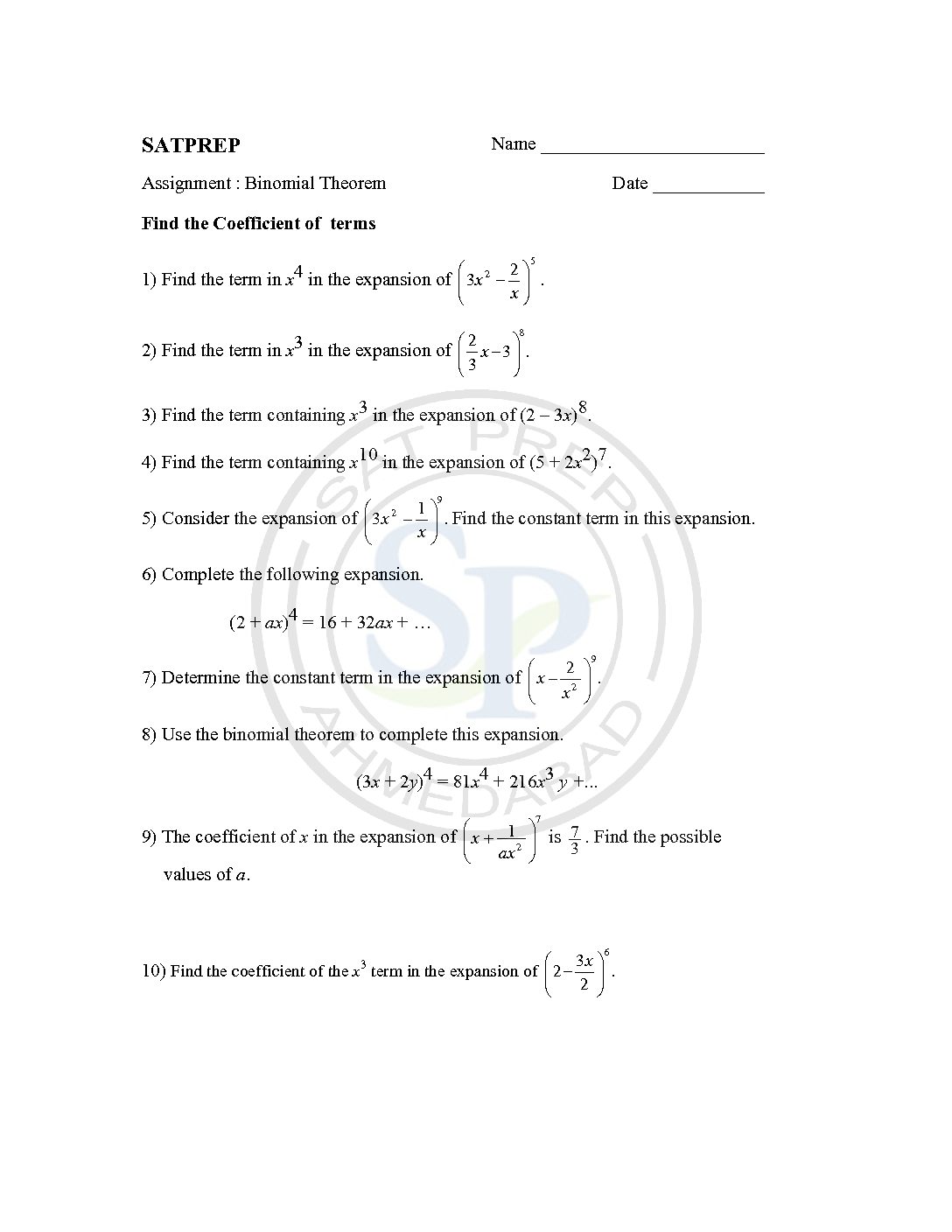
Binomial Theorem
When a binomial is raised to whole number powers, the coefficients of the terms in the expansion form a pattern. Each expansion has one more term than the power on the binomial. The sum of the exponents in each term in the expansion is the same as the power on the binomial. www.kutasoftware.com