trigonometry identities showing that the identity is always true, no matter what value of x or θ is used. Because it has to hold true for all values of x, we cannot simply substitute in a few values of x to “show” that they are equal. We have to use logical steps to show that one […]
You are browsing archives for
Category: Cambridge Maths AS
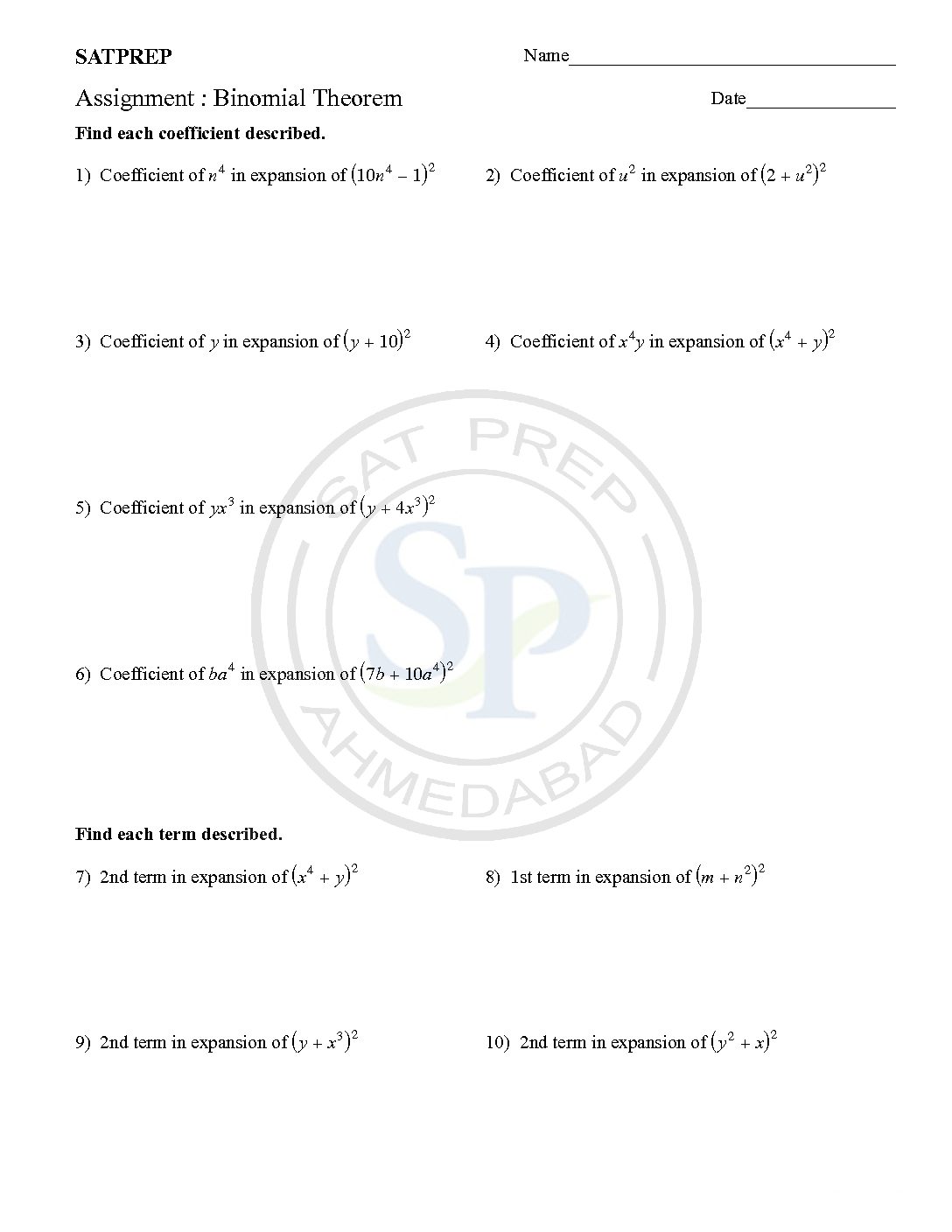
Binomial Theorem
Binomial theorems is another ways of expansion of two terms. Another way it is generalised form of expansion. Due to expansion of two term it is binomial. “What are the binomial coefficients?” . It shows how to calculate the coefficients in the expansion of (a + b) n. The symbol for a binomial coefficient nCr. As well as pascal […]
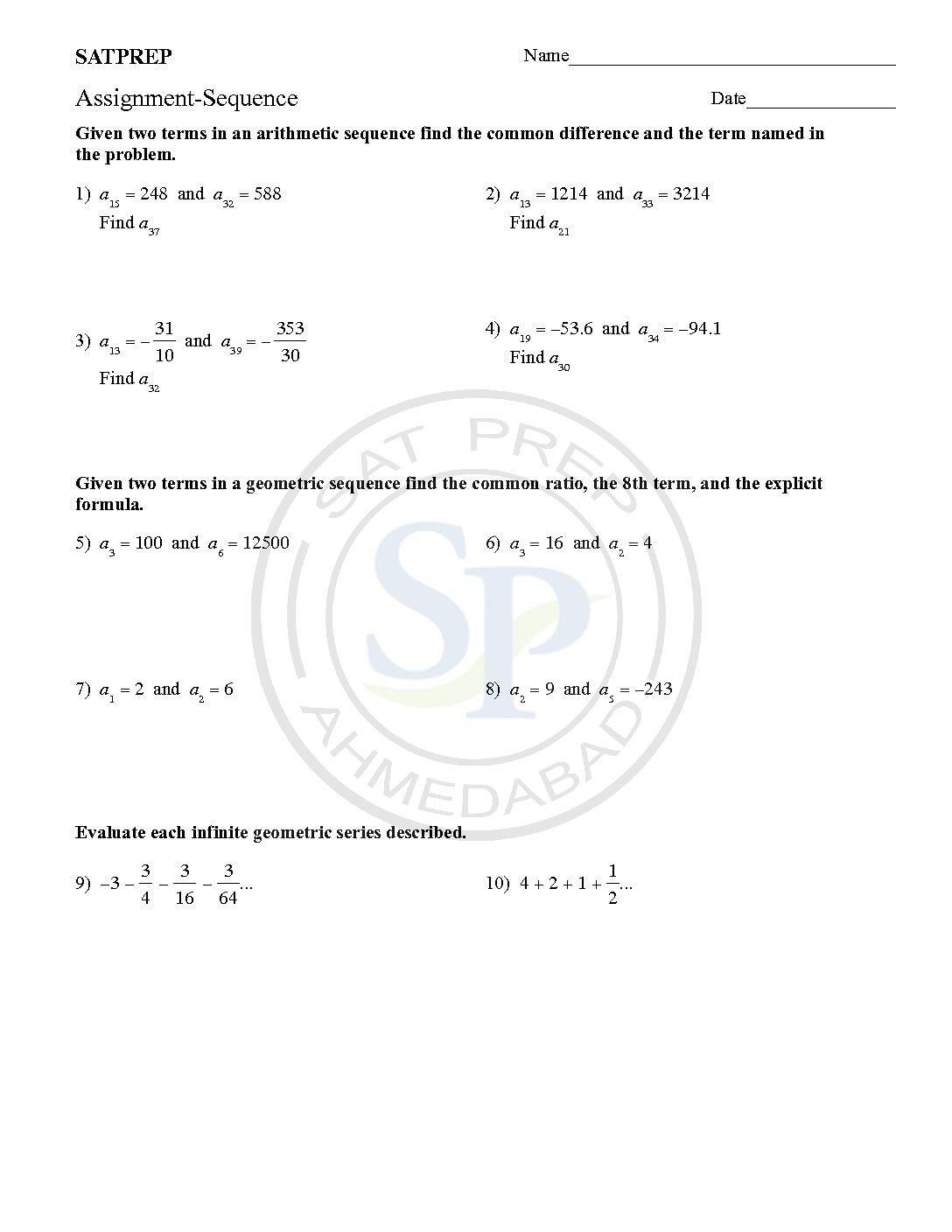
Sequence-2
Sequence and series is arrangement of term in particular pattern. Mathematical structures using the convergence properties of sequences. In particular, sequences are the basic for series Sequence and series
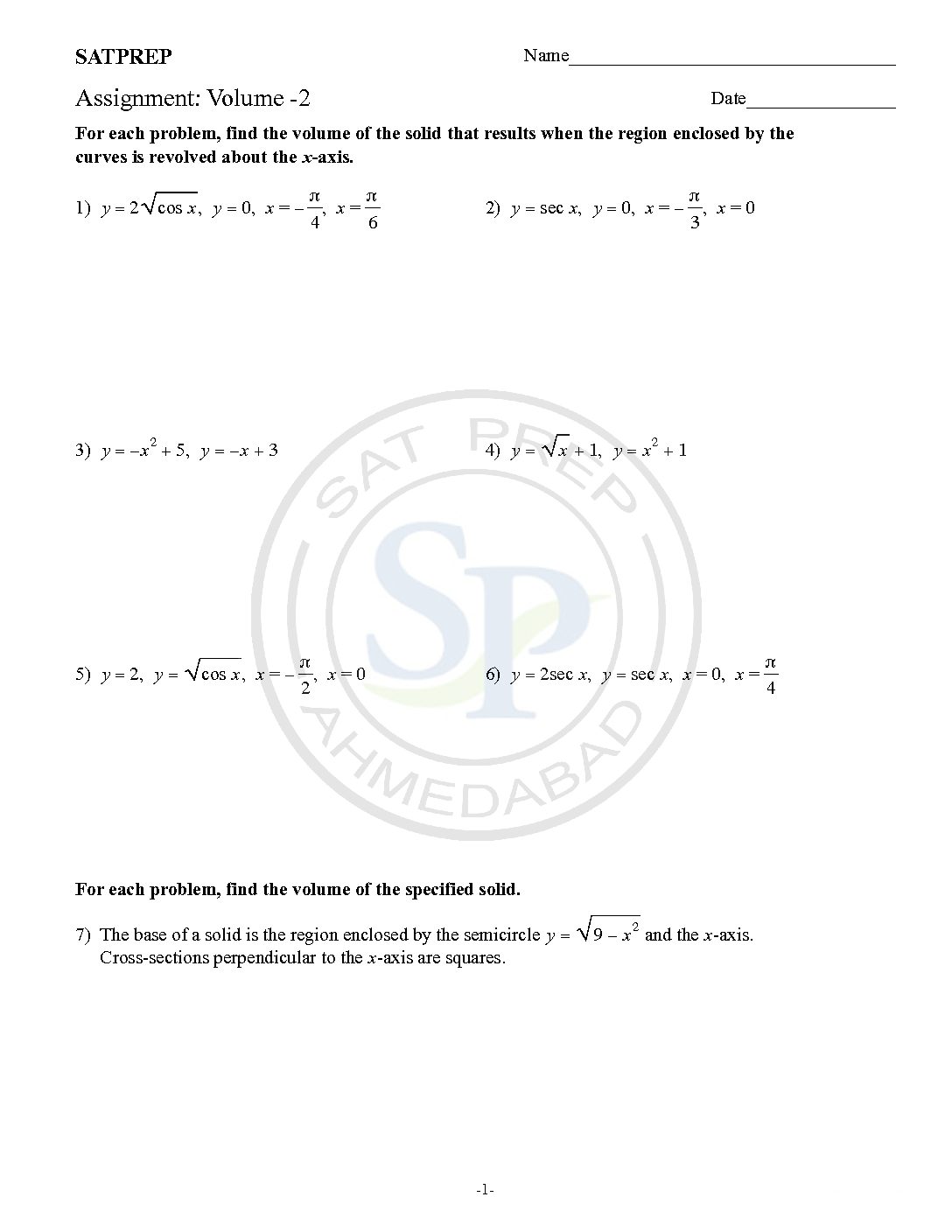
Volume -2
To get a solids of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. Volume
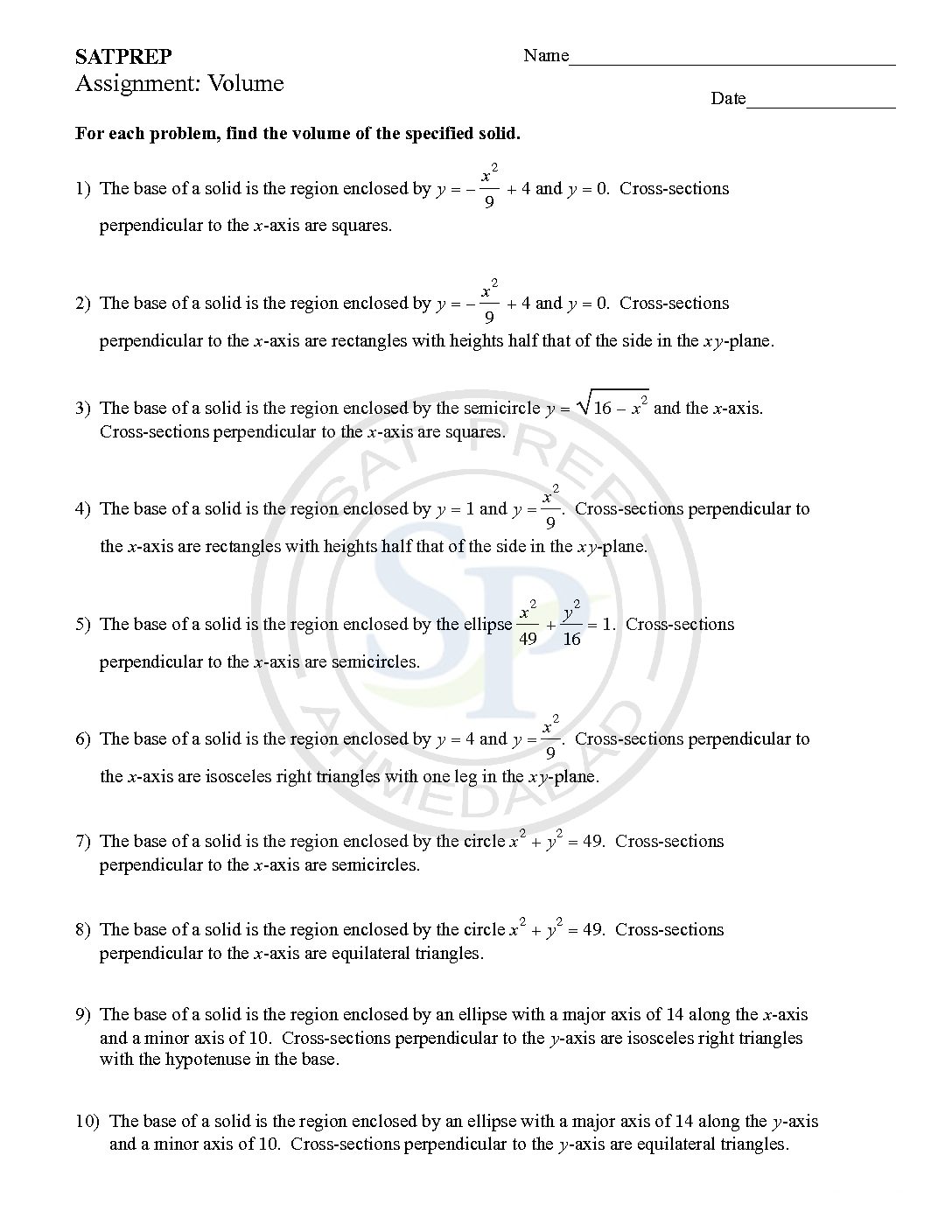
Volume
To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution. volume of revolution
Integration by substitution
This post is about worksheet of integration by trigonometric substitution. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by trigonometric substitutions
Factorisation
Factorisation process we applied bracket to take common term out. Also we apply difference of square of variable. Hence we reduce no of terms in expression. Therefore factorisation and expansion are reverse process. Factorisation
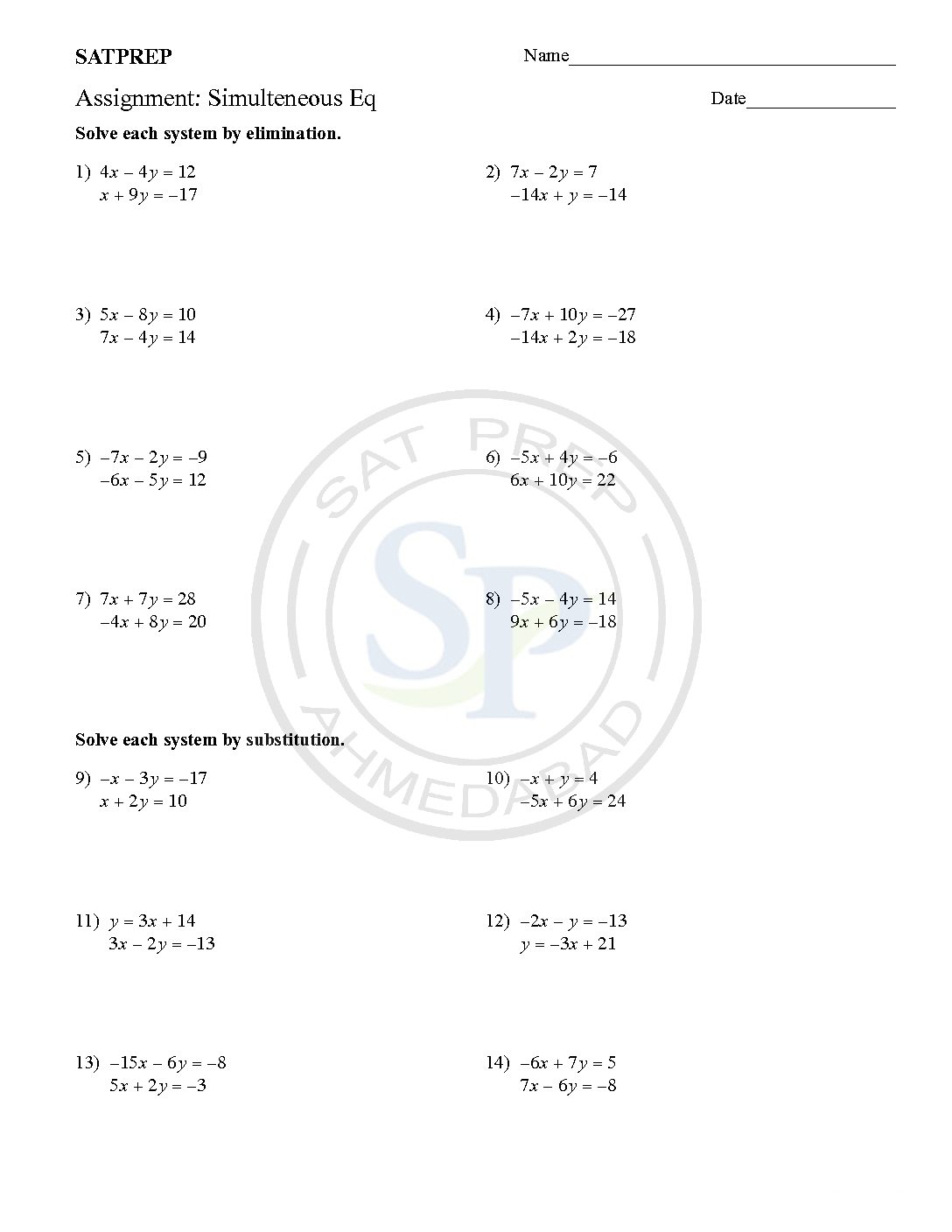
Simultaneous Eq.
simultaneous equations are solve by the elimination. These equations are also solve by substitution as well as graphic method. Due to variable solution give coordinate. simultaneous equation
Further Probability
to calculate Further Probability. Further Probability. An event ( E) is a subset of the sample space. That is, an event is a subset of all possible outcomes. We refer to this subset of outcomes as favourable outcomes. further probabilty
Sequence
Arrangement of term in particular pattern is known as sequence. A series is simply adding the terms in a sequence. An arithmetic series involves adding the terms of an arithmetic sequence. Similarly when terms are in ratio and addition , sequence as well as series will be geometric. Also when ratio is less than one than geometric series will be convergent. […]