This post is about worksheet of integration by algebraic substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by substitution
You are browsing archives for
Category: Calculus
Integration of Trigonometric Function
This post is about worksheet of Integration of trigonometric functions. It also one of most important concept of integral calculus . The function ƒ(φ(t)) is also integrable on [a,b] Integration of trigonometric function
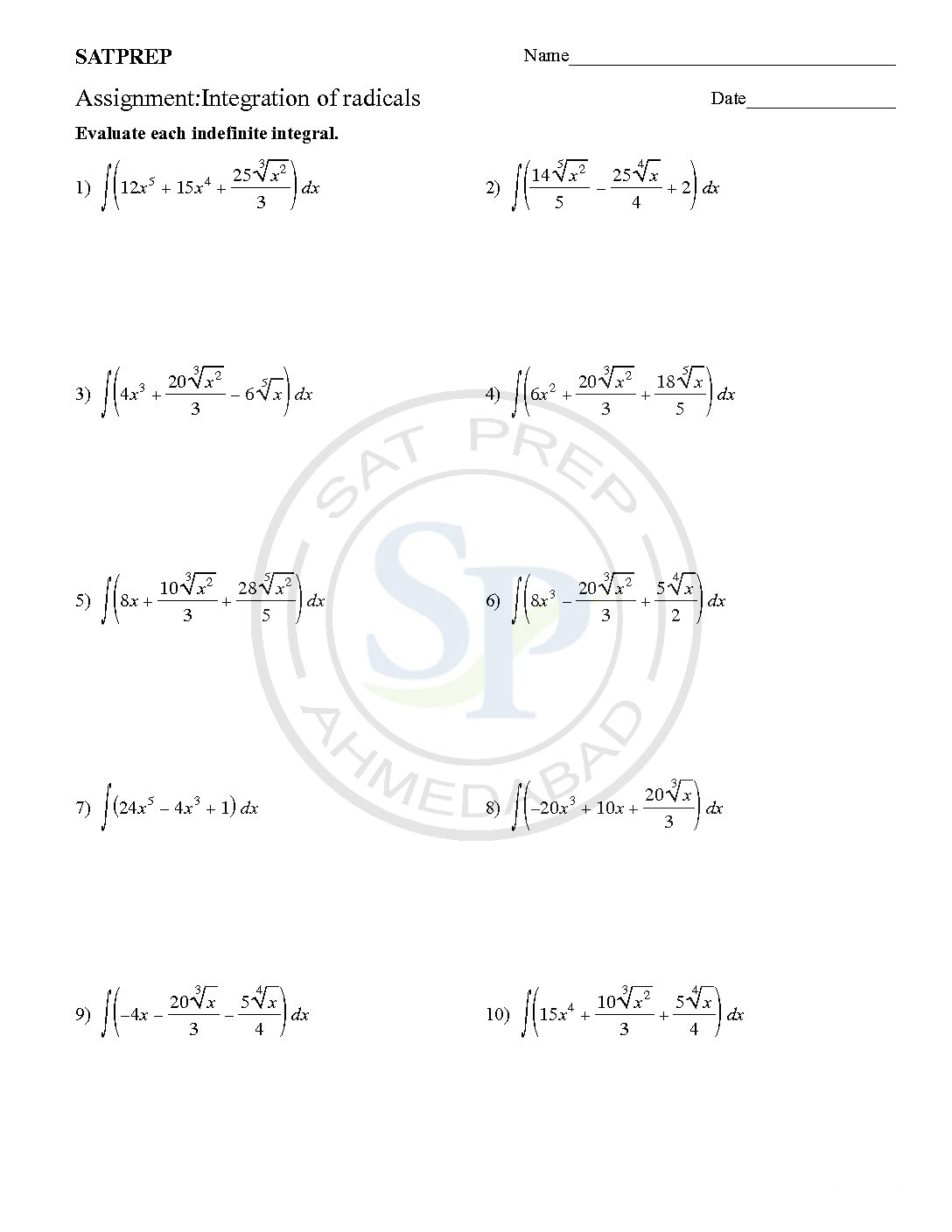
Integration of Radicals
This post about integration of polynomial with fractional power. First of all simplify expression also surds and then apply integration rule. Due to presence of constant it will give general solution. Integration
Integration of Polynomials
In integration the power of polynomials increase like quadratic become cubic etc. As well as get integration constant . It always give general solution due to integration constant. Integration
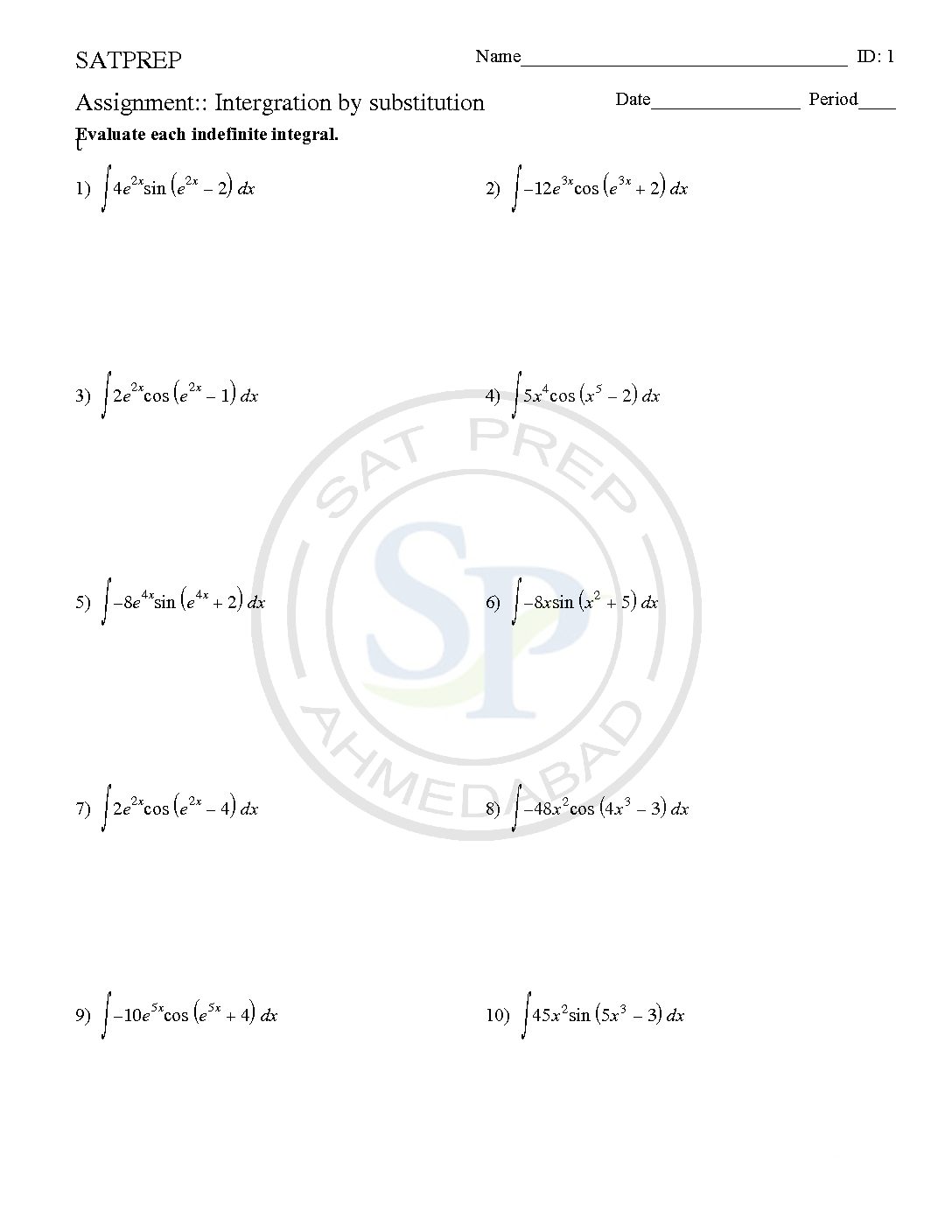
Integration by substitution -1
This post is about worksheet of Integration by exponential substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by exponential substitution
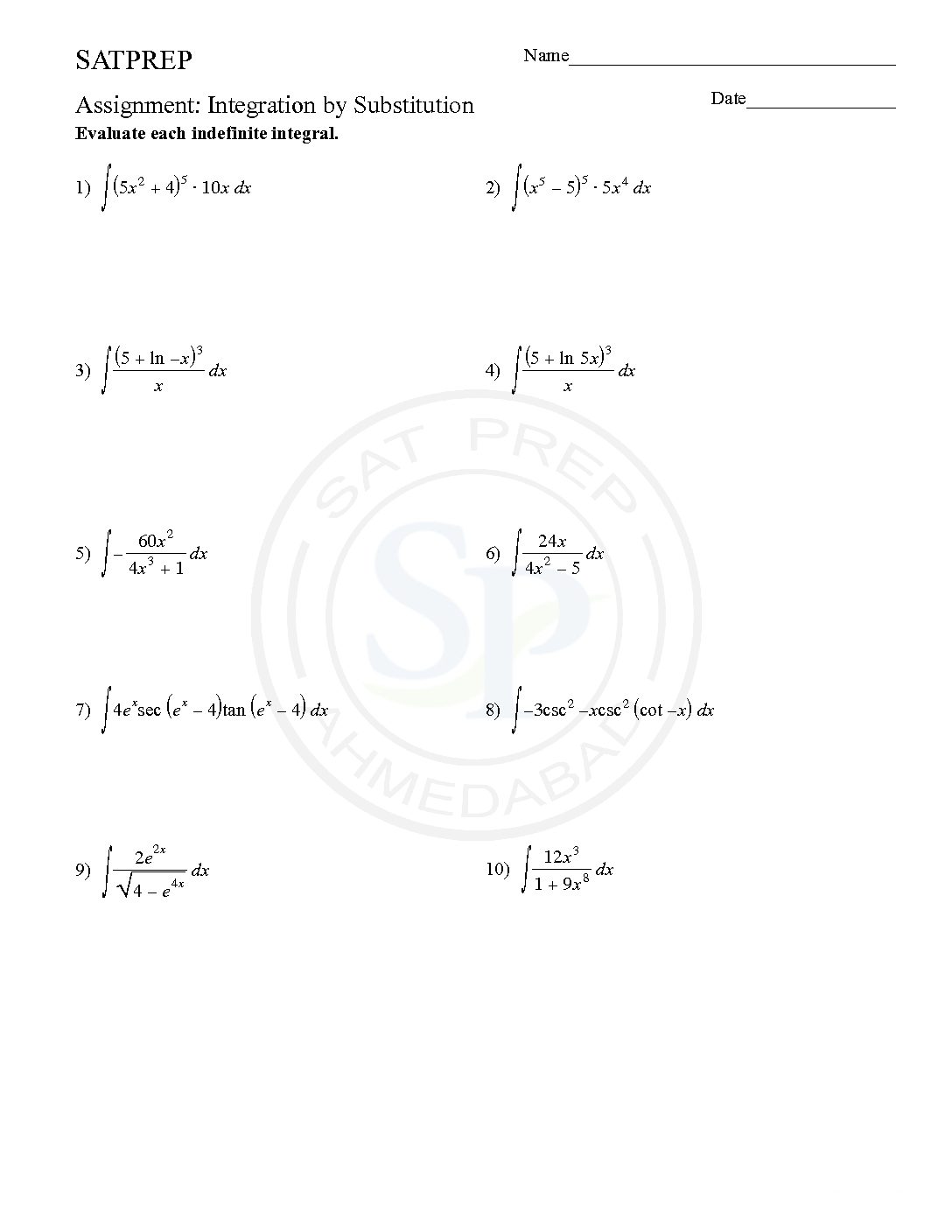
Integration by substitution -2
This post is about worksheet of integration by logarithmic substitution. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by logarithmic substitutions
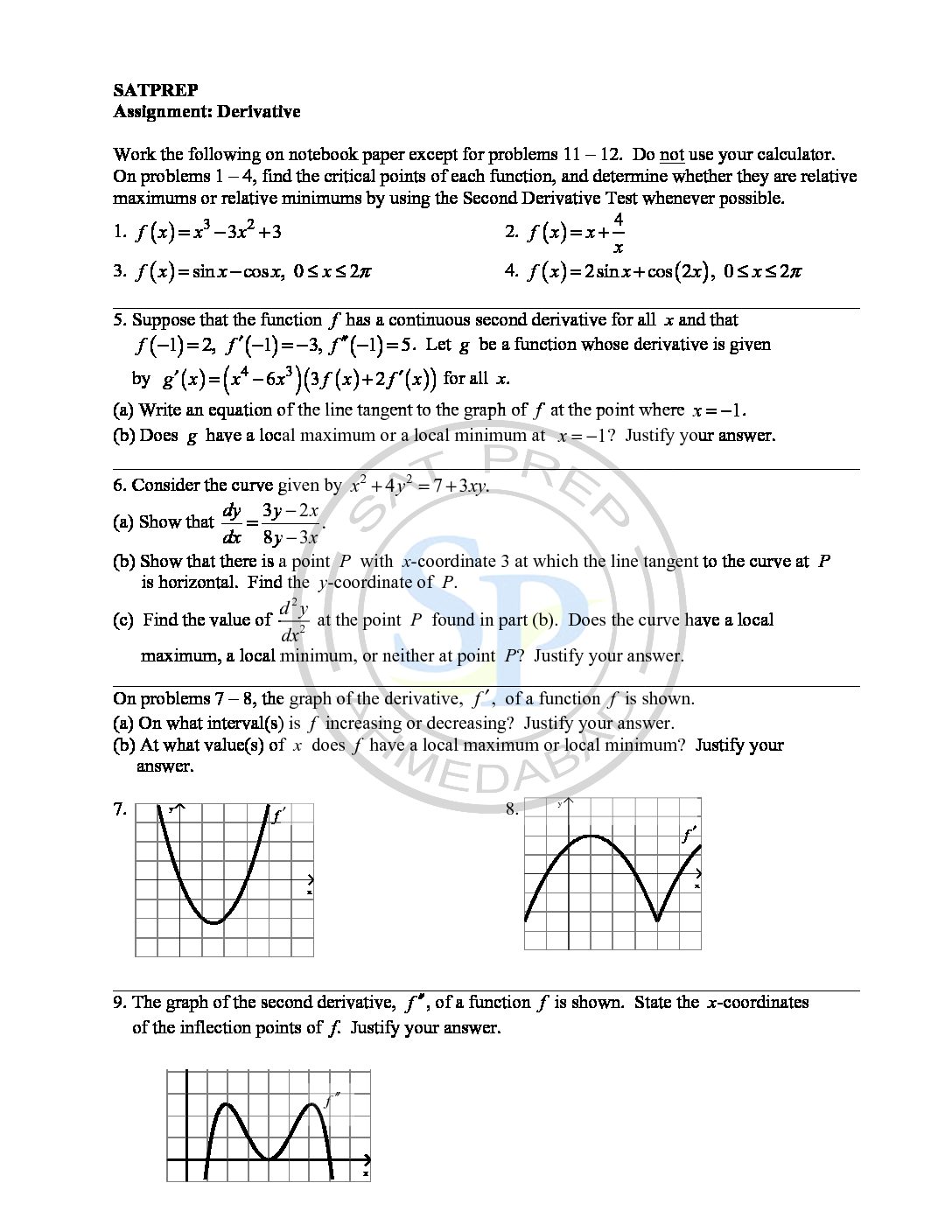
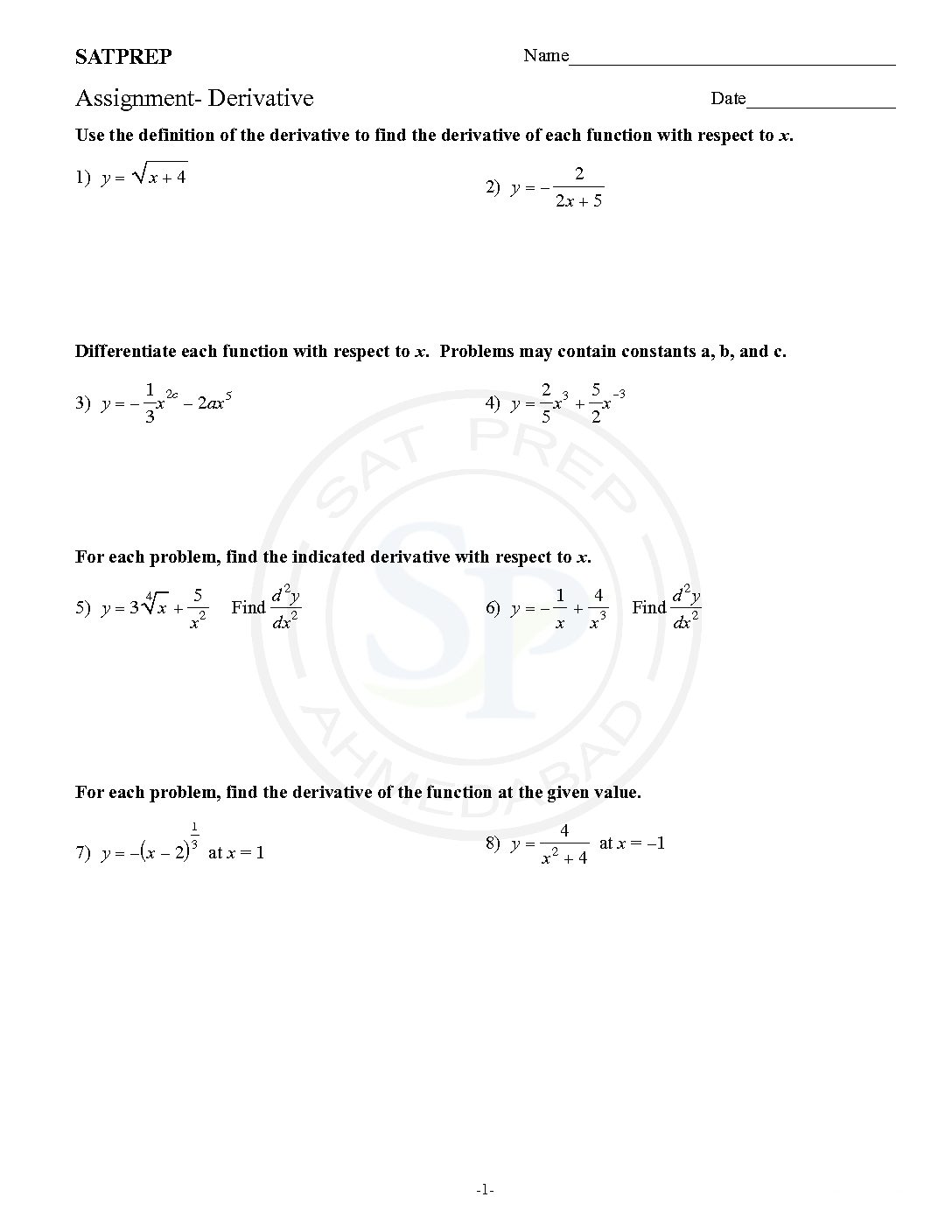
Derivative
The derivative of a function of a single variable at a chosen input value. Derivative is the slope of the tangent line to the graph of the function at that point. Hence derivative of a function y = f(x) of a variable x is a measure of the rate at which the value y of the function changes with respect to the change […]
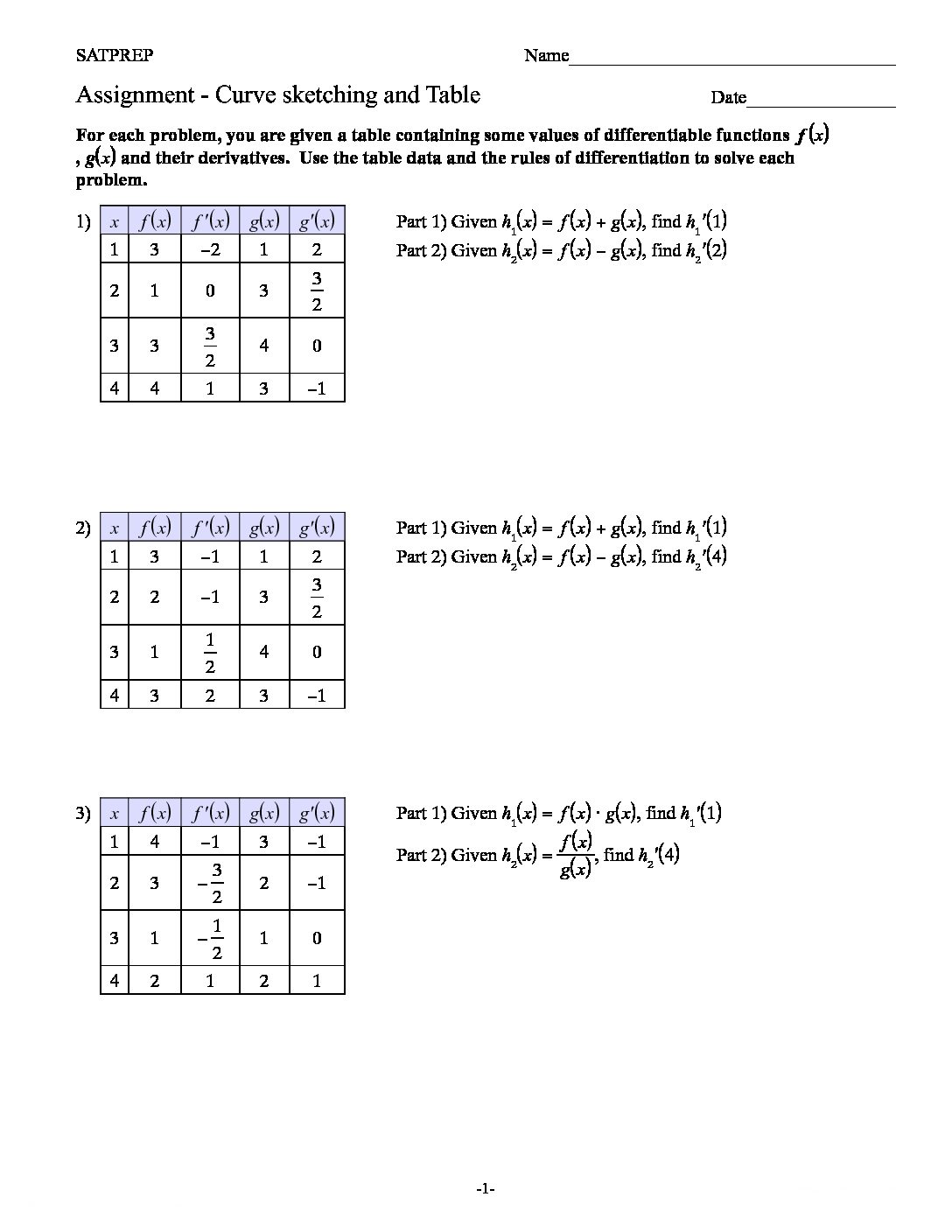
Curve sketching
Curve Sketching. If f (-x) = -f (x) for all x in the domain, then f is odd and symmetric about the origin. d) Asymptotes: Find the asymptotes of the function using the methods described above. First attempt to find the vertical and horizontal asymptotes of the function. Curve sketching
Derivative of polynomials
Derivative is product of differentiation. Differentiation has applications to nearly all quantitative disciplines. For example, in physics, the derivative of the displacement of a moving body with respect to time is the velocity of the body, and the derivative of velocity with respect to time is acceleration. Therefore differentiation is process Derivative
Optimization-2
Process of optimisation means optimal value of function at turning point (maximum or minimum ) value of the curve. Therefore second derivative use to find greatest or least value . Also it show greatest value. Optimization