Inflection Point. An inflection point is a point on a curve at which the sign of the curvature (i.e., the concavity) changes. Inflection points may be stationary points, but are not local maxima or local minima. For example, for the curve plotted above, the point is an inflection point. Point of infection
You are browsing archives for
Category: IB Maths SL
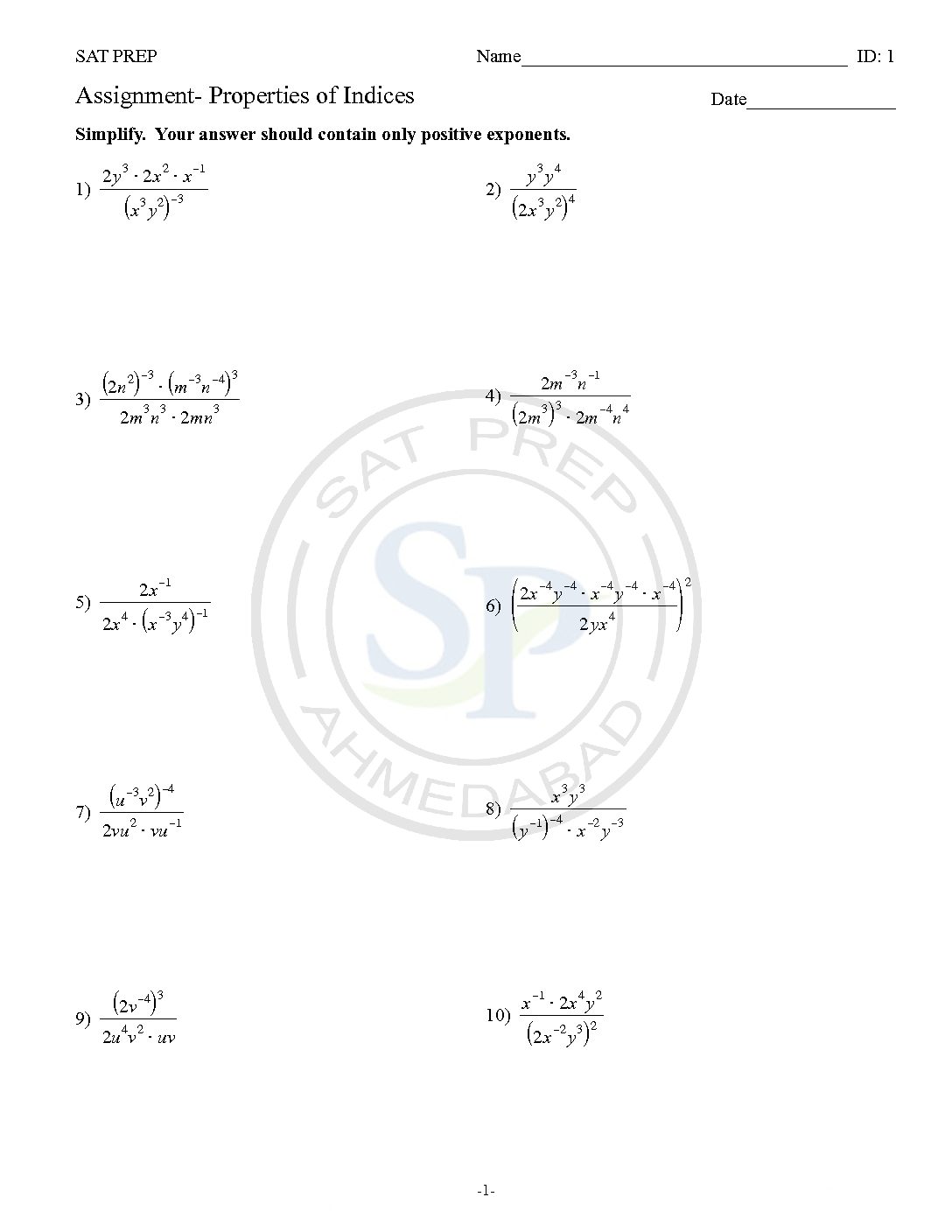
Properties of indices
Laws of Exponents. Exponents are also called Powers or Indices. The exponent of a number says how many times to use the number in a multiplication. Law of Indices. To manipulate expressions, we can consider using the Law of Indices. These laws only apply to expressions with the same base, for example, 3 4 and 3 2 can be manipulated […]
Formulae of Calculus
List of Calculus Formulas-basic Properties and Formulas of Integration : If f (x) and g(x) are differentiable functions . Another In basic calculus, we learn rules and formulas for differentiation, which is the method by which we calculate the derivative of a function, and integration, Differential Calculus that is concerning rates of change and slopes of curves, and Integral Calculus concerning […]
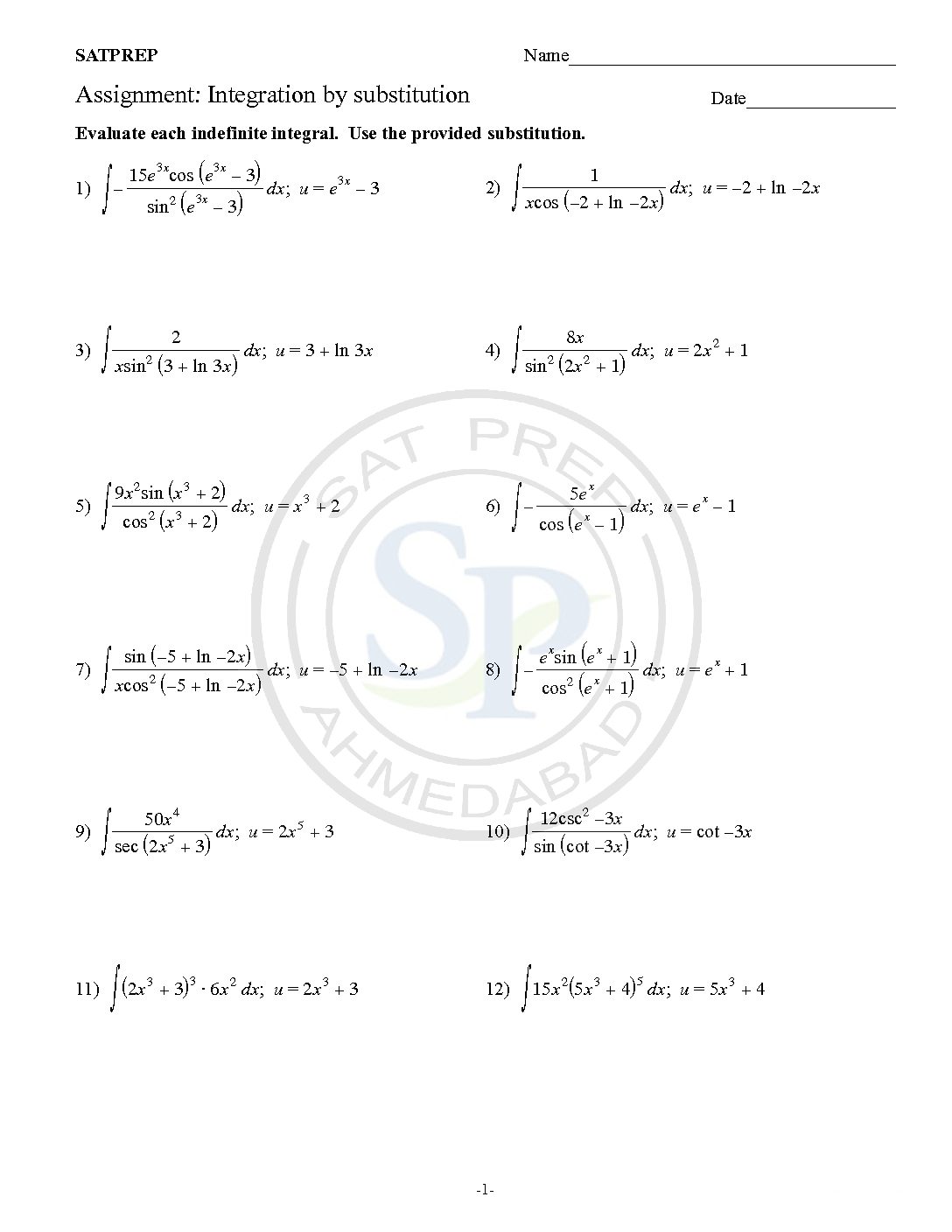
Integration by Substitution-5
This post is about worksheet of integration by substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by substitution
Integration by Substitution-4
This post is about worksheet of integration by substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] integration by substitution
integration by algebraic substitutions
This post is about worksheet of integration by algebraic substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by substitution
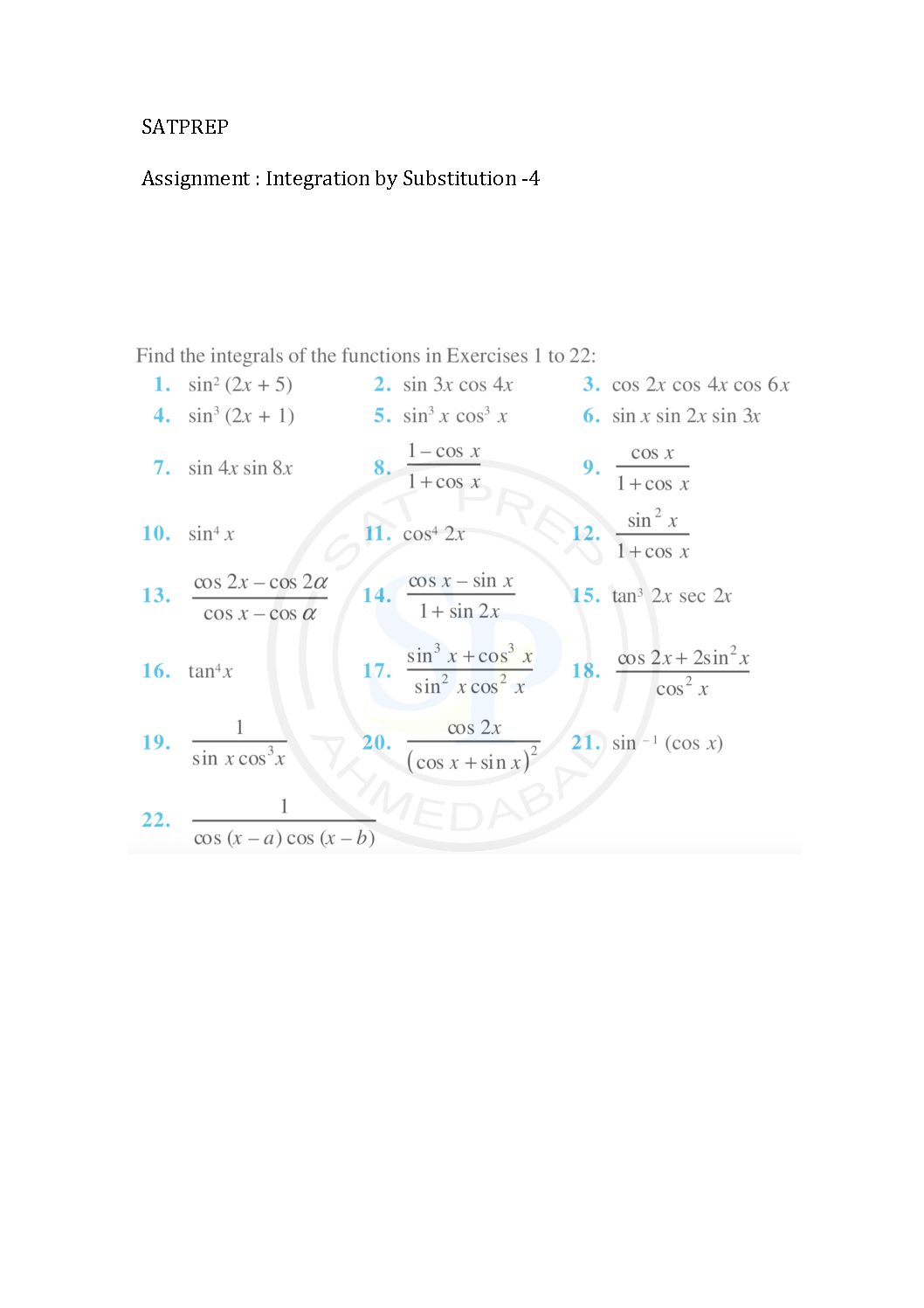
Integration of Trigonometric Function
This post is about worksheet of Integration of trigonometric functions. It also one of most important concept of integral calculus . The function ƒ(φ(t)) is also integrable on [a,b] Integration of trigonometric function
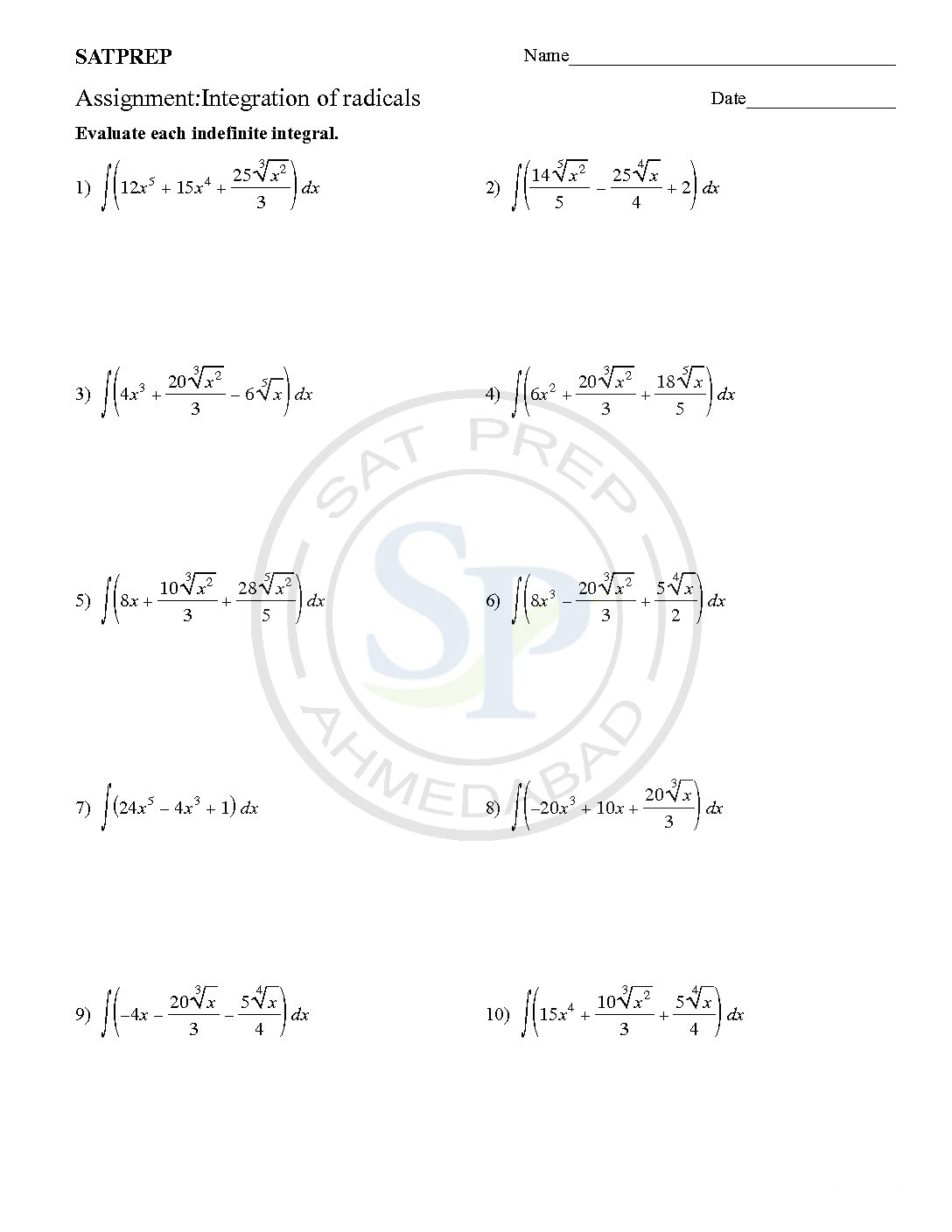
Integration of Radicals
This post about integration of polynomial with fractional power. First of all simplify expression also surds and then apply integration rule. Due to presence of constant it will give general solution. Integration
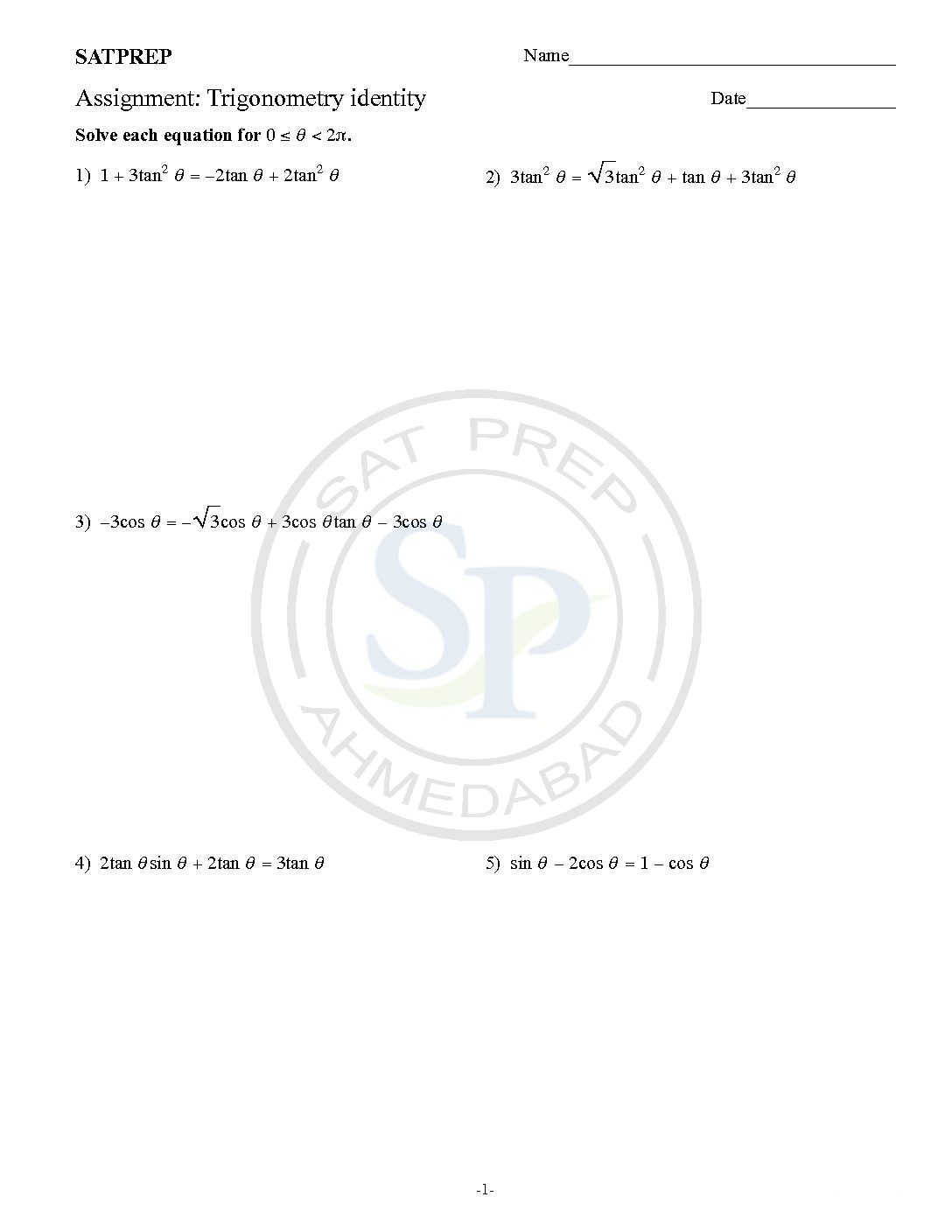
Trigonometric Identity
Trigonometric identities showing that the identity is always true, no matter what value of x or θ is used. Because it has to hold true for all values of x, we cannot simply substitute in a few values of x to “show” that they are equal. We have to use logical steps to show that one […]
Trigonometric Equation
Solving trigonometric equations use both the reference angles and trigonometric identities The general method of solving an equation is to convert it into the form of one ratio only. Then, using these results, hence, we can obtain solutions. Trigonometric Equation