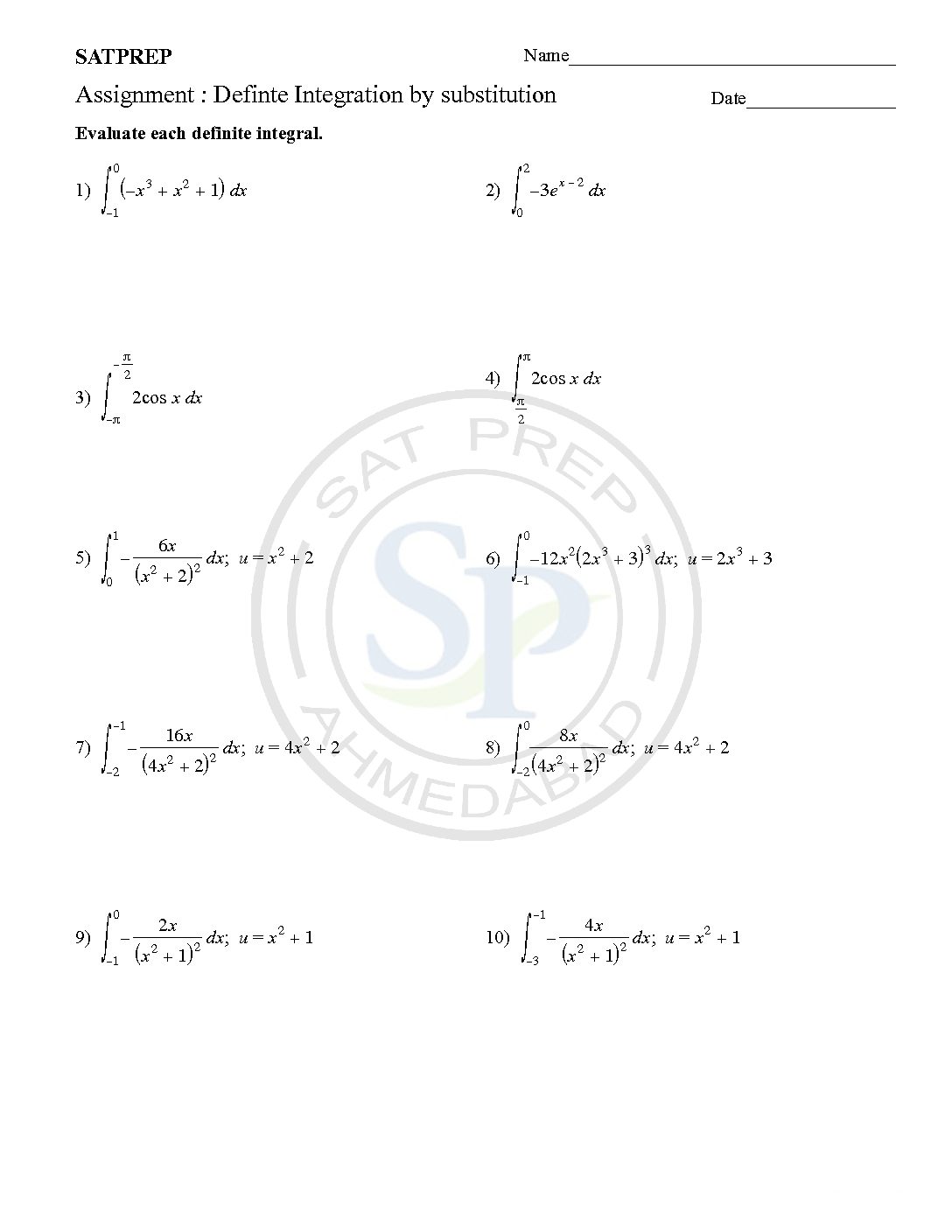
A Definite Integral has start and end values. In other words there is an interval [a, b]. Hence , definite integral gives particular solution. Definite Integration
You are browsing archives for
Tag: Definite Integration
Volume of revolution
To get a solid of revolution we start out with a function, y=f (x), on an interval [a,b]. We then rotate this curve about a given axis to get the surface of the solid of revolution Volume of Revolution
Area Under the Curve
This post about Area under a Curve. The area between the graph of y = f ( x ) and the x -axis is given by the definite integral. This formula gives a positive result for a graph above the x -axis, and a negative result for a graph below the x -axis. Because of enclosed region by limit. Hence , we use definite integration. Similarly for volume […]
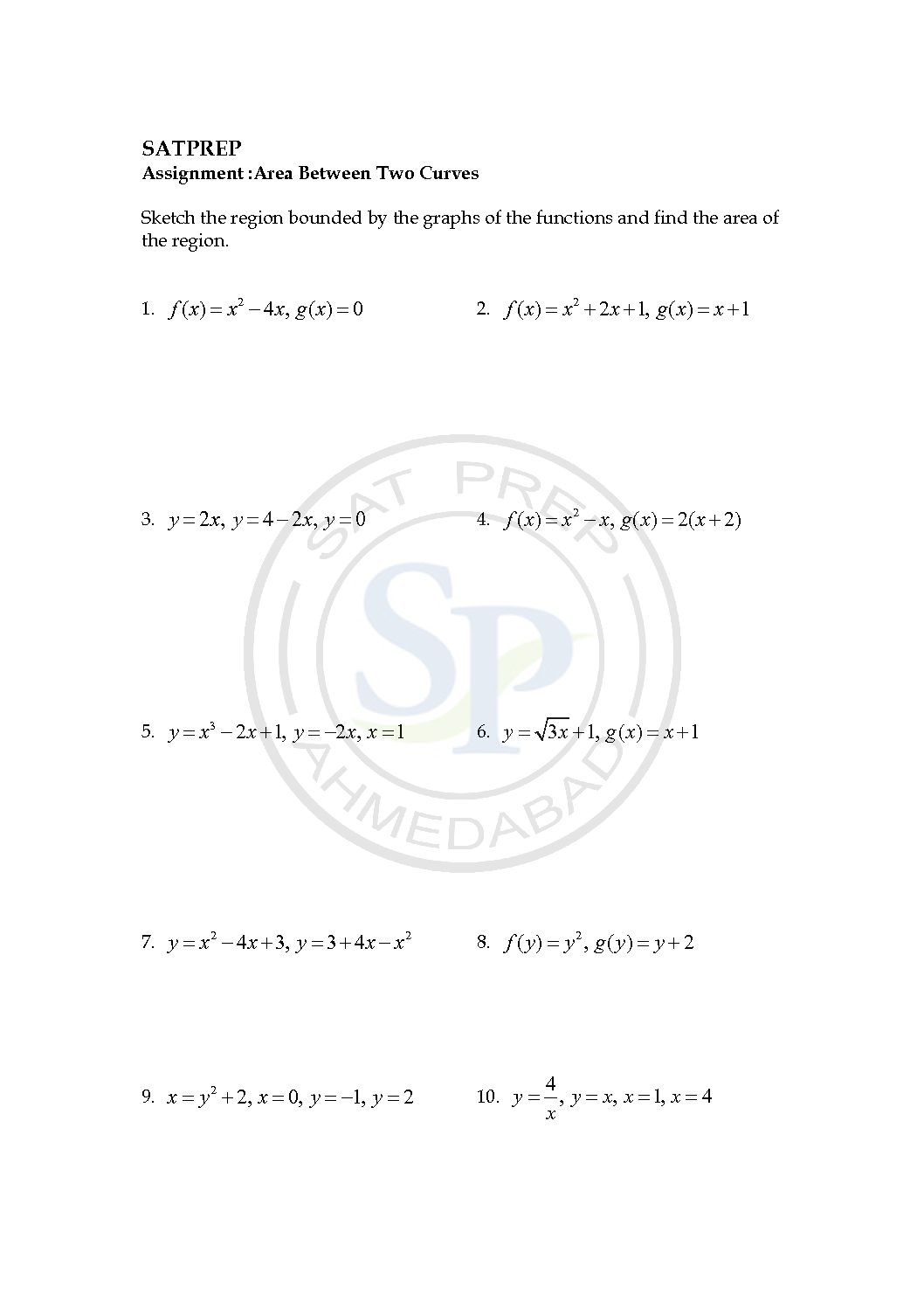
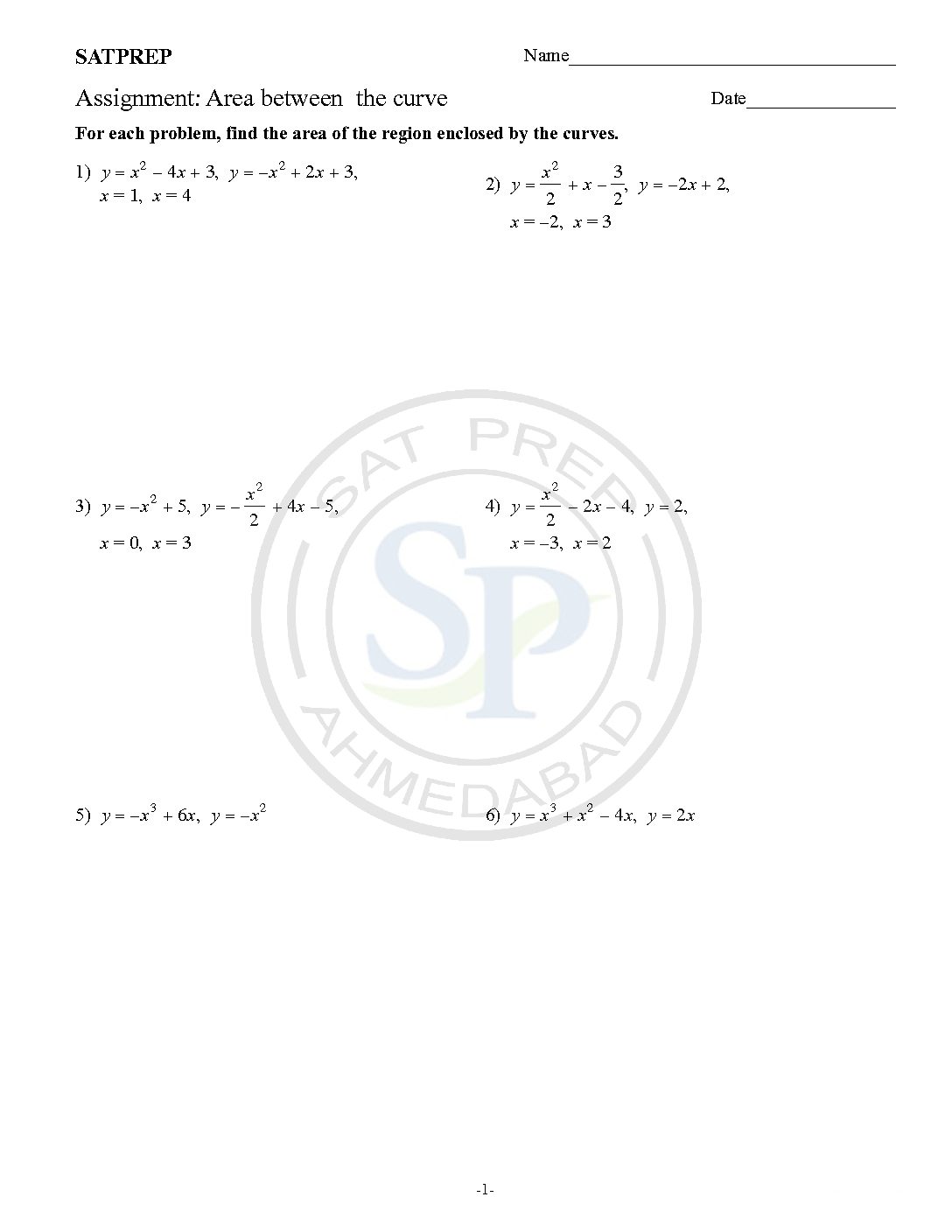
Area between the curve
area between curves y = f (x) between x = a and x = b, integrate y = f (x) between the limits of a and b. Areas under the x-axis will come out negative and areas above the x-axis will be positive. Area
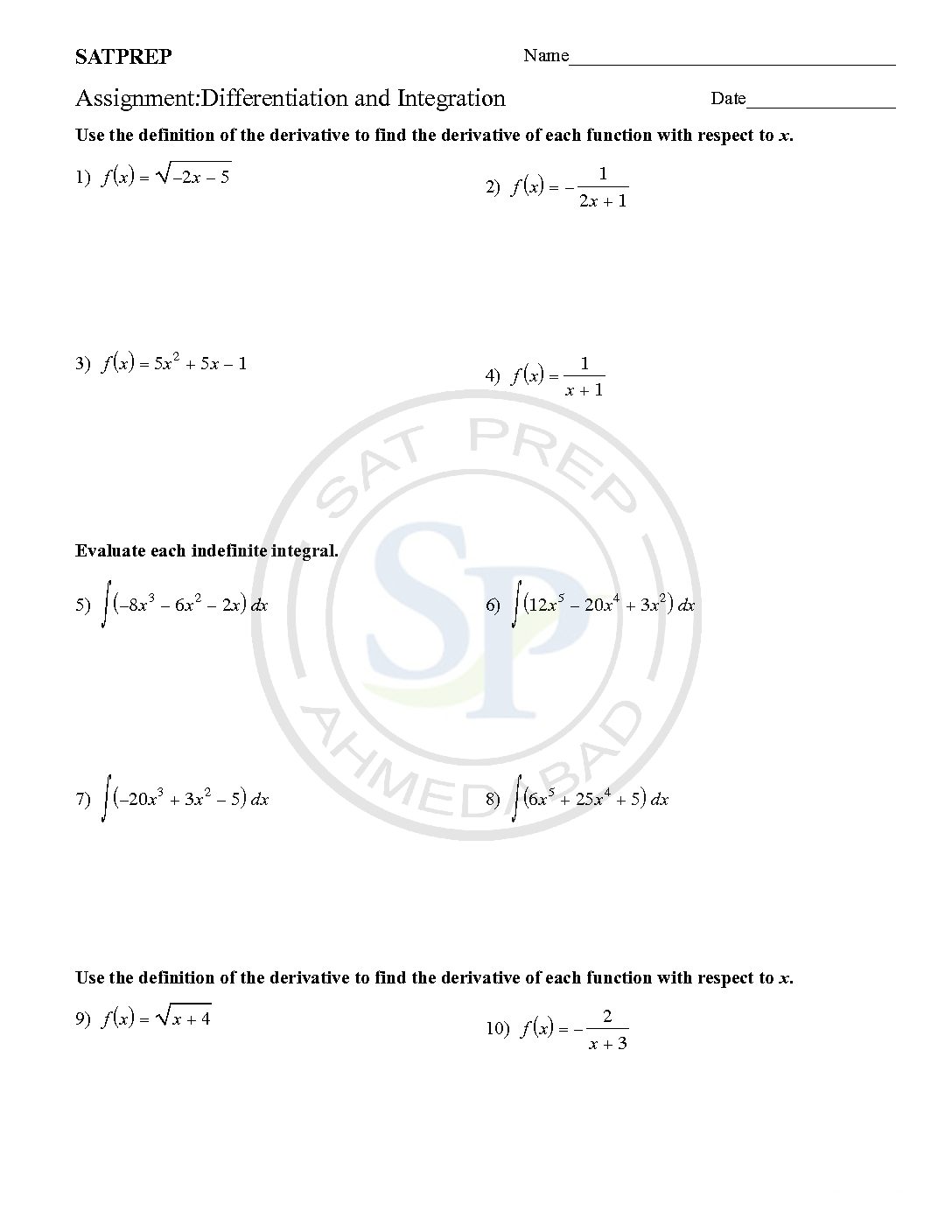
Differentiation and Integration
First of all differentiation and Integration are process of calculus. Due to differentiation we get derivative, while integration of derivative we get function back. Integration also called derivative. Differentiation and Integration