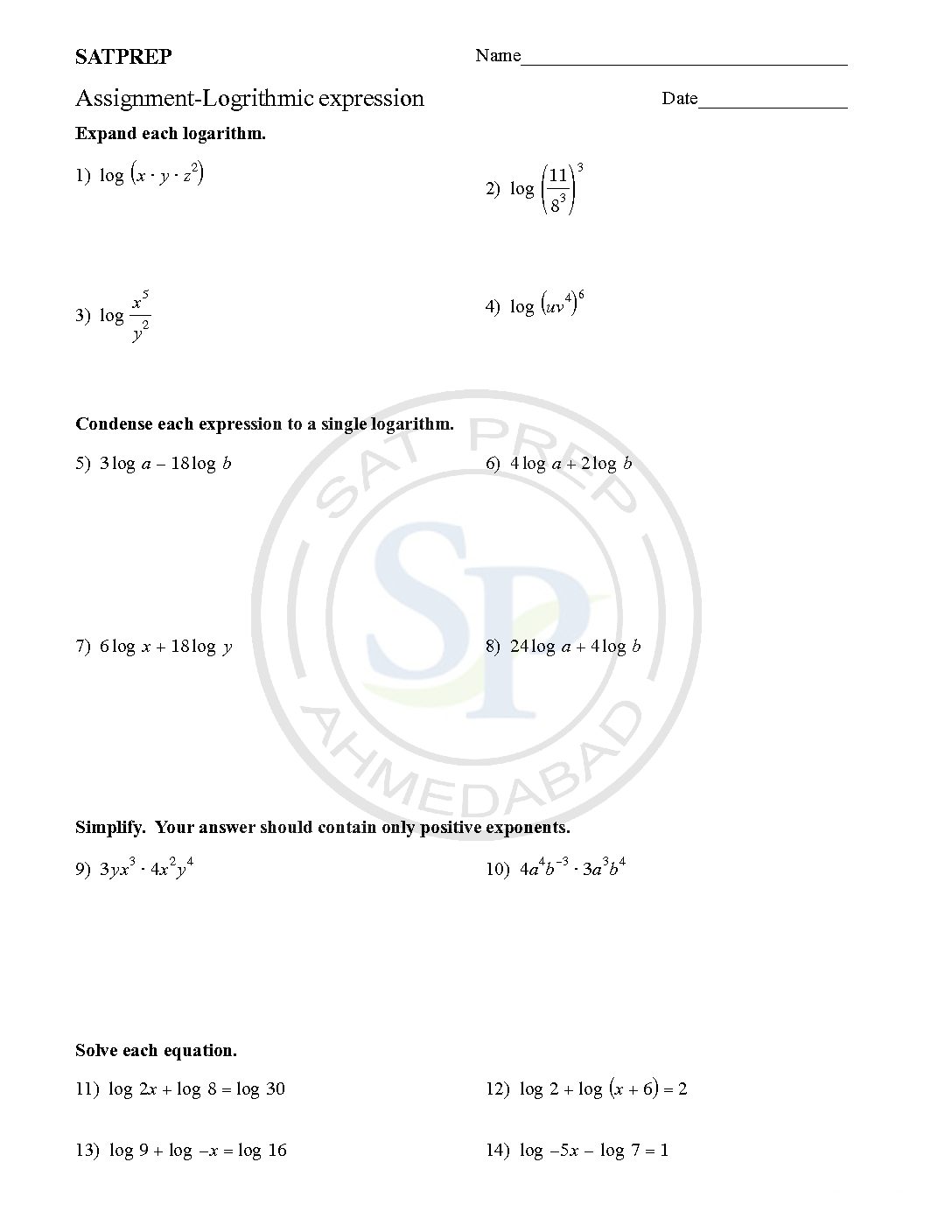
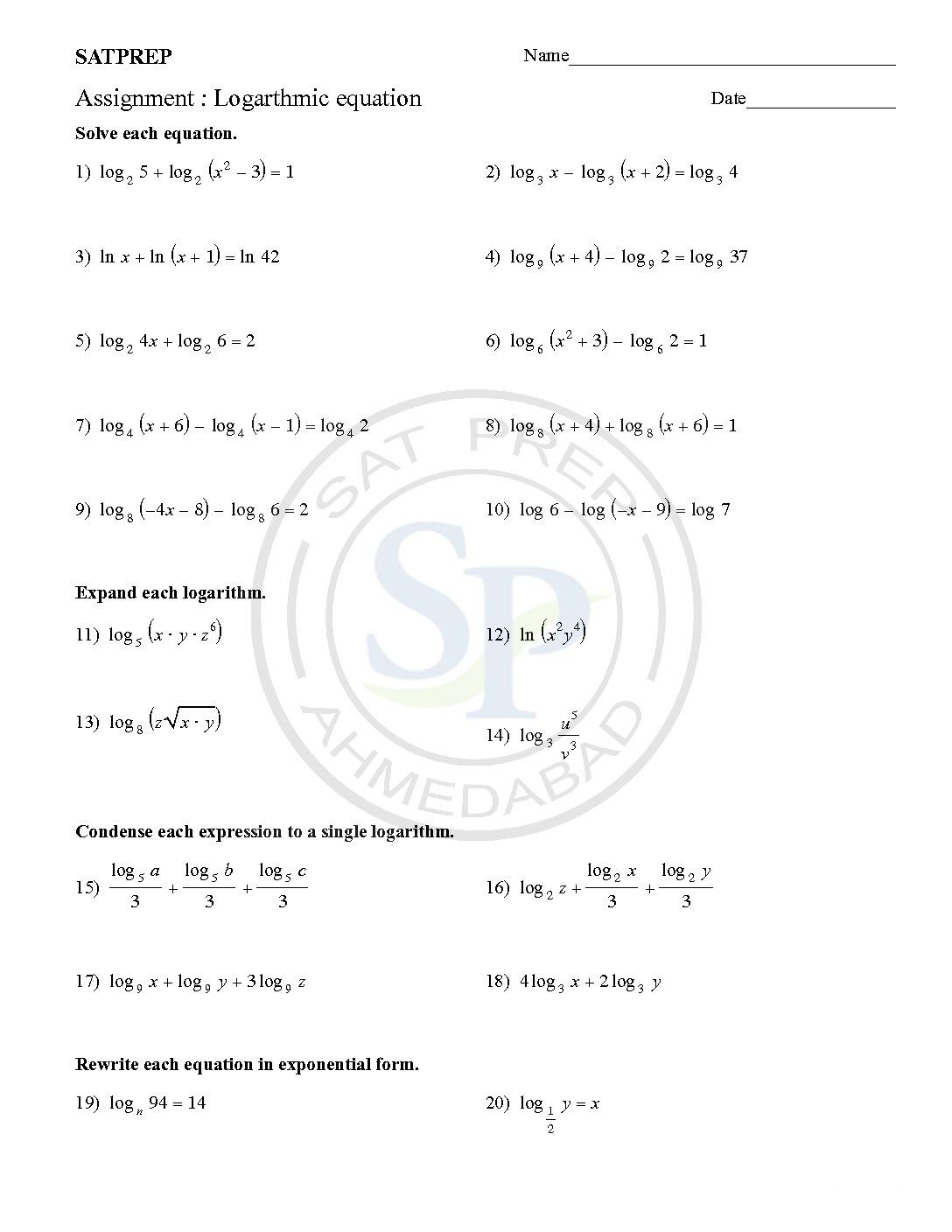
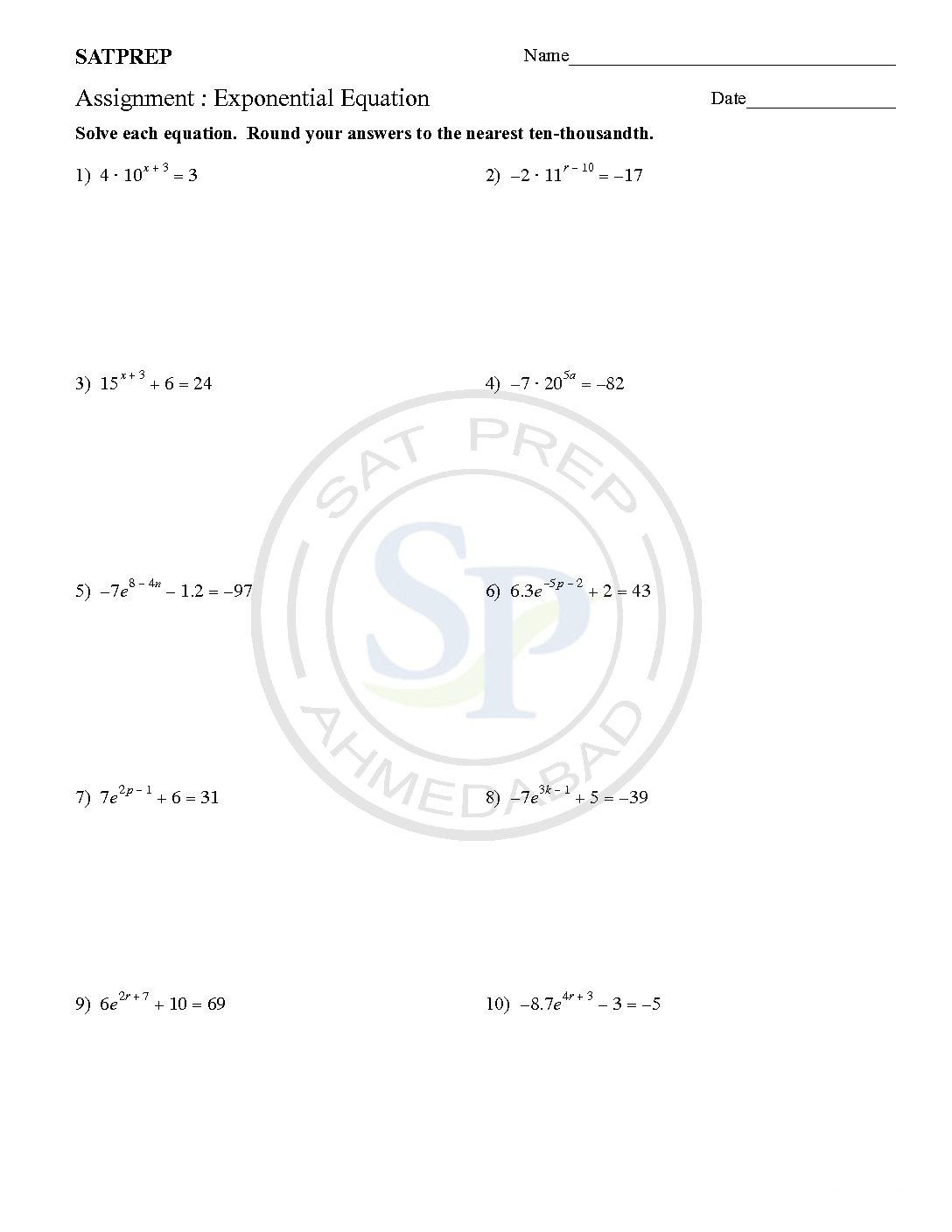
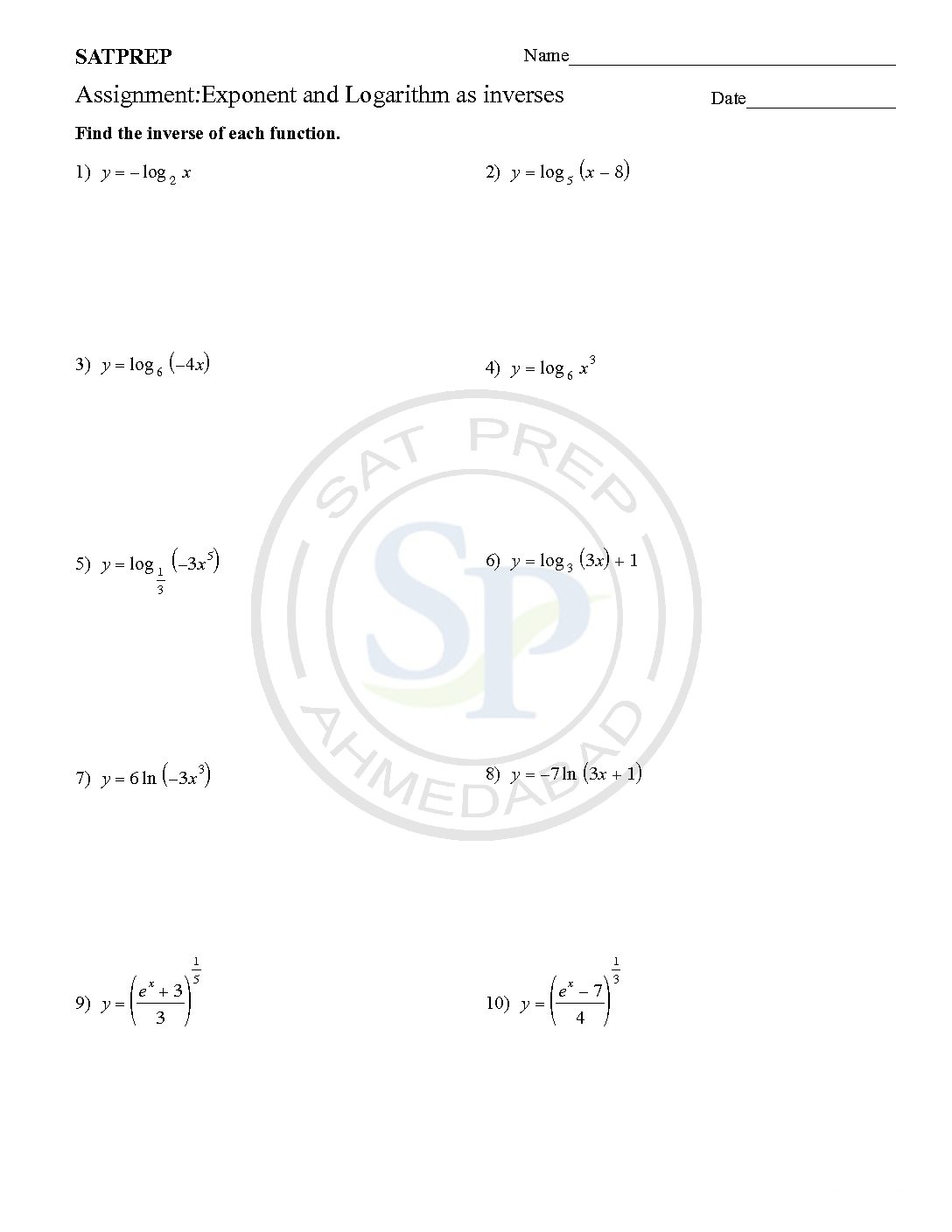
Solve the logarithmic equation. This problem involves the use of the symbol “ln” instead of “log” to mean logarithm. Logarithmic expression
You are browsing archives for
Tag: exponential
Logarthmic Equation
Logarithms equation can be solved using the properties of logarithms. So equation either are solve by log or exponent. Because both inverse of each other hence are either way exponent or log.In both apply properties of log or exponent. Also we need to factorised to solve. Logarithmic eq
Exponential Equation
Exponential equations are one in which a variable occurs in the exponent, for example, . When both sides of the equation have the same base, the exponents on either side are equal by the property Exponential Equation
Exponent and Logarithm inverses
The inverse of the exponential function y = ax is x = ay. The logarithmic function y = logax is defined to be equivalent to the exponential equation x = ay. y = logax only under the following conditions: x = ay, a > 0, and a≠1. It is called the logarithmic function with base […]
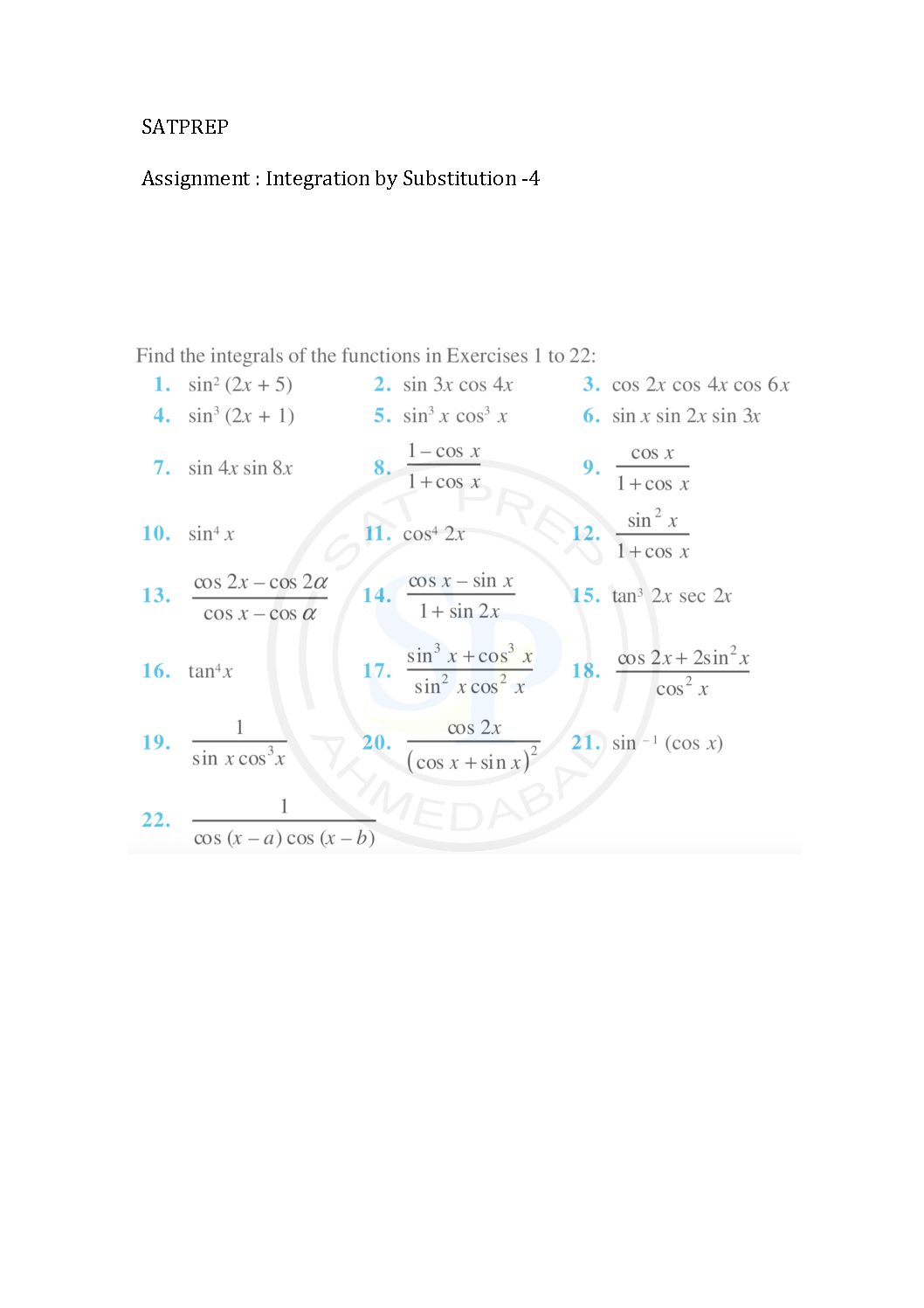
Integration by Substitution-4
This post is about worksheet of integration by substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] integration by substitution
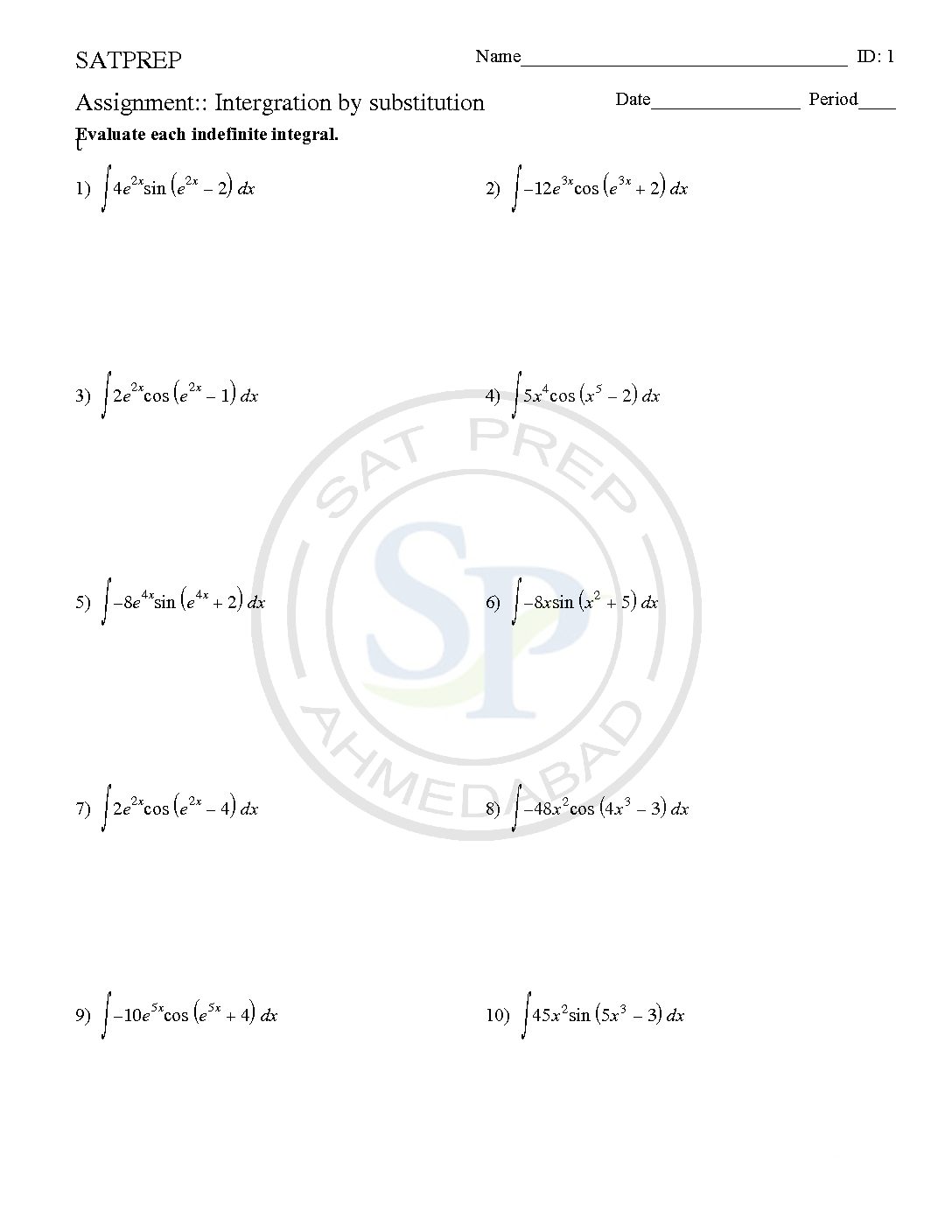
Integration by substitution -1
This post is about worksheet of Integration by exponential substitutions. It also one of most important concept of integral calculus . The function ƒ(φ(t))φ′(t) is also integrable on [a,b] Integration by exponential substitution
Limits- Trigonometric and Exponential
exponential and trigonometric functions without the use of limits. Hence Some critical trigonometric limits Sinning by degrees is costly. Further exponential functions also Limit – trigonometric and exponential
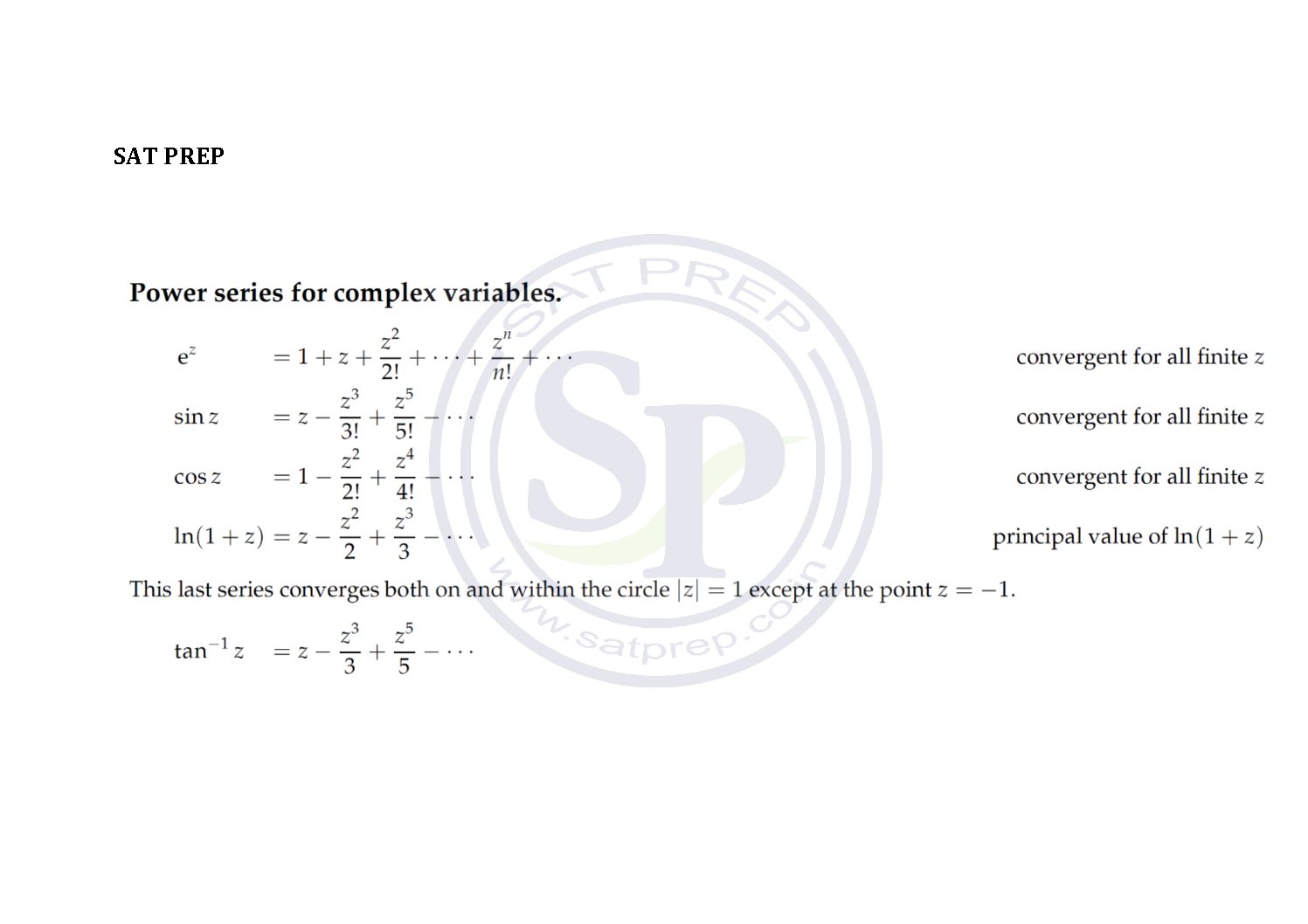
Power series with Complex Coefficient
Power series with Complex Coefficient. n represents the coefficient of the nth term and c is a constant. An is independent of x and may be expressed as a function of n . The set of all formal power series in X with coefficients in a commutative ring R form another ring that is written R [[ X ]] Also expressions for Coefficients of a Power Series. As […]
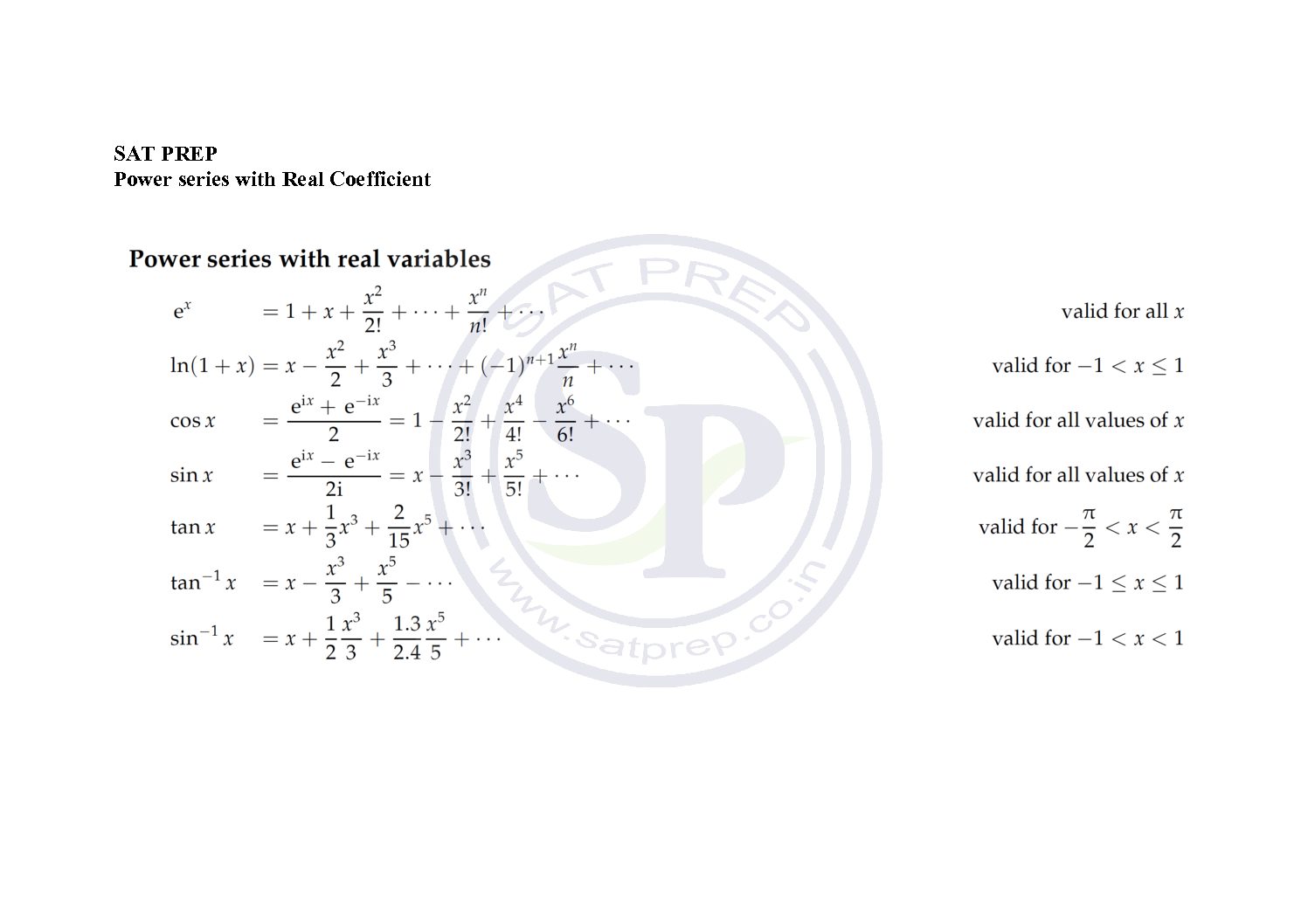
Power series with Real Coefficient
Power series with Real Coefficients. The exponential function (in blue), and the sum of the first n + 1 terms… Radius of convergence. A power series will converge for some values of the variable x… Operations on power series. Power Series